Calculus - The laws of limits

MySecretMathTutor

23 Sept 201209:57

EducationalLearning

32 Likes 10 Comments

TLDRThis video script introduces the fundamental laws of limits in calculus, explaining how they simplify the process of finding limits by breaking down complex problems into manageable parts. It covers basic addition, subtraction, and multiplication by a constant, as well as more advanced rules like product, quotient, power, and root laws. The script also highlights the importance of understanding basic limits of identity and constant functions, demonstrating their application in solving a sample limit problem step by step.

Takeaways

📚 The video discusses the basic laws of limits in calculus.
➕ The limit of the sum of two functions is the sum of their limits.
➖ The limit of the difference of two functions is the difference of their limits.
🔢 The limit of a function multiplied by a constant is the constant multiplied by the limit of the function.
✖️ The limit of the product of two functions is the product of their limits.
➗ The limit of the quotient of two functions is the quotient of their limits, provided the denominator's limit is not zero.
🔝 The limit of a function raised to a power is the limit of the function raised to that power.
🌿 The limit of a function inside a root is the root of the limit of the function.
🔍 The laws of limits simplify complex problems by breaking them into smaller parts.
📊 Basic limits like the identity function and constant function help solve more complicated limits.

Q & A

What are the basic laws of limits discussed in the video?
-The basic laws of limits discussed in the video include the addition, subtraction, and constant multiple laws. These laws allow for the combination of functions and their limits in an intuitive way, such as adding or subtracting the limits of functions and multiplying the limit of a function by a constant.
How do the laws of limits help in simplifying the process of finding limits?
-The laws of limits help simplify the process by breaking down a complex problem into smaller, more manageable pieces. Instead of finding the limit of an entire function, you can find the limits of individual components and then apply the laws to combine these results.
What is the product rule for limits as mentioned in the video?
-The product rule for limits states that if you are looking for the limit of two functions being multiplied, you can take the limit of each function individually and then multiply these limits together.
Can you explain the quotient rule for limits?
-The quotient rule for limits allows you to find the limit of two functions being divided by taking the limit of the numerator and the limit of the denominator, and then dividing these two limits. However, this can only be done if the limit of the denominator is not zero to avoid division by zero.
What is the power rule for limits and when can it be applied?
-The power rule for limits states that if you are looking for the limit of a function raised to a power, you can take the limit of the function and then raise that result to the power. This rule can be applied as long as the power or index, n, is a positive integer.
What is the root rule for limits and how does it work?
-The root rule for limits allows you to find the limit of a function inside a root by taking the limit of the function and then applying the root to that result.
What are the basic limits of the identity function and the constant function?
-The basic limit of the identity function as x approaches a value 'a' is 'a'. For a constant function, the limit as x approaches 'a' is the constant value 'K' itself.
How can the basic limits and limit laws be used to solve more complicated limits?
-The basic limits and limit laws can be used to break down a complicated limit into simpler parts. By applying the laws to these parts, you can find the individual limits and then use the basic limits to evaluate the final result.
Can you provide an example of applying the limit laws to a complex function?
-The video provides an example where the limit of (x^2 - 1) / (4x) as x approaches 4 is calculated. The quotient law is first applied, then the difference and root laws, and finally the basic limits of the identity and constant functions are used to find the final result.
What is the final result of the example limit calculation provided in the video?
-The final result of the example limit calculation is 15/4, which is obtained by applying the limit laws and basic limits to the function (x^2 - 1) / (4x) as x approaches 4.
Why is it important to understand the assumptions and restrictions of the limit laws?
-Understanding the assumptions and restrictions of the limit laws is important to ensure that the mathematical rules are being followed correctly. For example, the quotient rule requires that the limit of the denominator is not zero, and the power and root rules require that the power or index is a positive integer.

Outlines

00:00

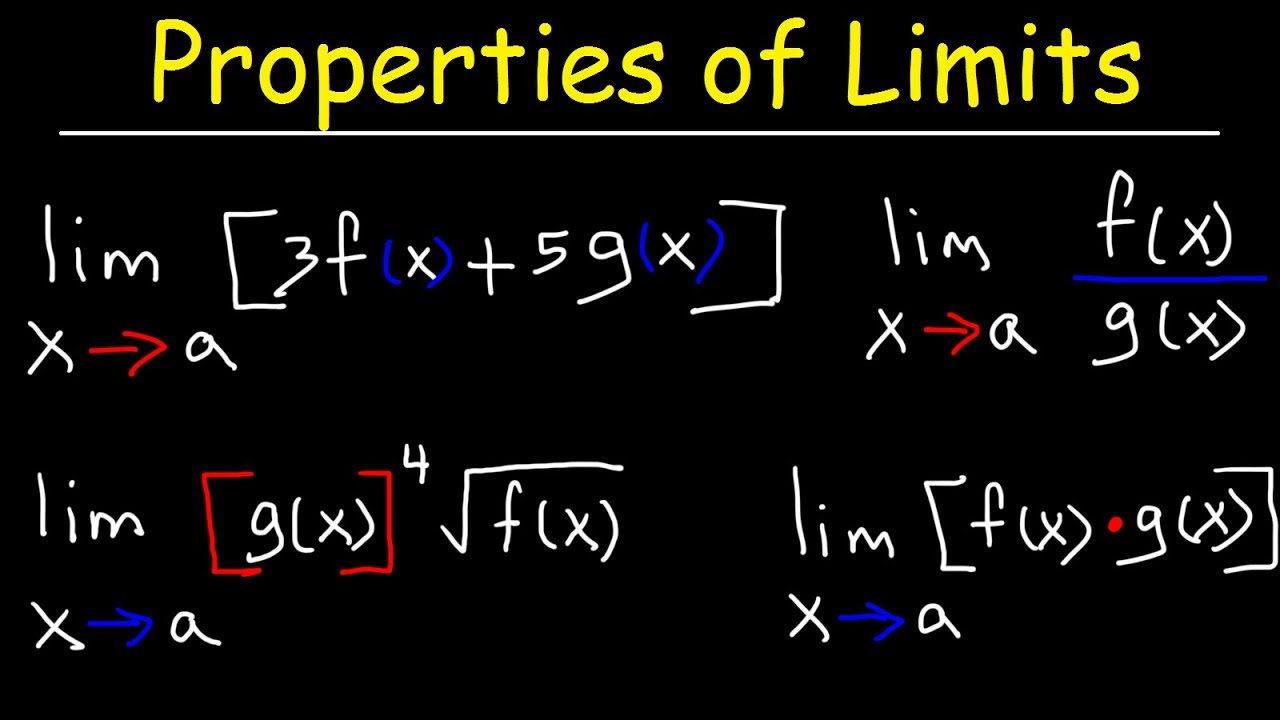
🔍 Understanding Basic Laws of Limits

This paragraph introduces the video, which focuses on the basic laws of limits. It outlines the plan to explore these laws and demonstrate how they can be used to compute limits of various functions. The importance of these laws in simplifying complex limit problems by breaking them into manageable parts is emphasized.

05:01

➕ Adding and Subtracting Limits

This section explains the laws for adding and subtracting limits. If you know the limits of two functions f and g, you can add their limits to get the limit of their sum and subtract their limits to get the limit of their difference. These laws simplify complex problems by allowing individual limit calculations.

✖️ Multiplying by Constants and Other Basic Operations

This paragraph covers the law that allows multiplying a function by a constant. The limit of the product is simply the product of the limit and the constant. This law makes it easier to handle functions multiplied by constants in limit problems.

⚙️ Product, Quotient, Power, and Root Laws

This section details the laws for products, quotients, powers, and roots of functions. The product law involves multiplying individual limits, the quotient law involves dividing limits (with the condition that the denominator limit is not zero), and the power and root laws involve applying the power or root to the limit of the function.

🔢 Applying Basic Limits

This paragraph introduces basic limits of identity and constant functions. The limit of the identity function (x) as x approaches a value is that value itself. The limit of a constant function is the constant. These basic limits are essential for solving more complex limit problems.

🔍 Breaking Down a Complex Limit Problem

This section applies the previously discussed laws to a specific problem: finding the limit as x approaches 4 of (x^2 - 1) / (4x). By breaking the problem into smaller parts and applying the limit laws, the solution becomes manageable. The quotient, difference, and constant multiplication laws are used to simplify the problem step-by-step.

✅ Final Computation of Limits

This paragraph shows the final steps of solving the example problem by plugging in values and simplifying the results. By applying the laws of limits and basic limits, the limit of the given function is computed as 15/4. The importance of breaking down complex problems into simpler parts is reinforced.

Mindmap

Keywords

💡Limits

Limits are fundamental concepts in calculus that describe the value that a function approaches as the input approaches some value. In the video, limits are used to compute the value of functions as variables approach certain points.

💡Limit Laws

Limit laws are rules that allow the manipulation and combination of limits to solve more complex problems. These laws simplify the process of finding limits by breaking down complicated expressions into manageable parts.

💡Functions

Functions are mathematical entities that assign a unique output to each input. In the video, functions such as f and g are used to demonstrate how limit laws apply to various mathematical operations like addition, subtraction, multiplication, and division.

💡Sum Rule

The sum rule states that the limit of the sum of two functions is the sum of their limits. This rule simplifies finding the limit of combined functions, as shown when adding the limits of f and g.

💡Difference Rule

The difference rule states that the limit of the difference of two functions is the difference of their limits. This helps in calculating limits when functions are subtracted from one another.

💡Constant Multiple Rule

The constant multiple rule states that the limit of a constant times a function is the constant times the limit of the function. This rule is used when a function is scaled by a constant factor.

💡Product Rule

The product rule states that the limit of the product of two functions is the product of their limits. This helps in calculating the limit when two functions are multiplied together.

💡Quotient Rule

The quotient rule states that the limit of the quotient of two functions is the quotient of their limits, provided the limit of the denominator is not zero. This rule is essential for dividing functions and finding their limits.

💡Power Rule

The power rule states that the limit of a function raised to a power is the limit of the function raised to that power. This rule is useful for functions involving exponents.

💡Root Rule

The root rule states that the limit of the root of a function is the root of the limit of the function. This is particularly useful for functions involving square roots or other fractional powers.

💡Identity Function

An identity function is a function that always returns the same value that was used as its input. In the video, it is shown that the limit of the identity function as x approaches a is simply a.

💡Constant Function

A constant function is a function that always returns the same value regardless of the input. The video demonstrates that the limit of a constant function as x approaches any value is the constant itself.

Highlights

Introduction to basic laws of limits in calculus.

Explanation of how limits allow us to handle complex situations by breaking them into simpler parts.

The first law of limits: adding functions and their limits.

The second law of limits: subtracting functions and their limits.

The third law of limits: multiplying a function by a constant and finding its limit.

Importance of understanding individual limits to solve more complex problems.

Introduction to the product, quotient, power, and root laws for limits.

Product rule for limits: multiplying individual limits of two functions.

Quotient rule for limits: dividing individual limits, with the restriction of non-zero denominator limit.

Power rule for limits: raising the limit of a function to a power.

Root rule for limits: taking the limit of a function under a root.

Limit of an identity function as x approaches a value.

Limit of a constant function as x approaches any value.

Using basic limits and limit laws to compute more complicated limits.

Example problem: computing the limit of (x^2 - 1) / (4x) as x approaches 4.

Application of the quotient law to simplify the example problem.

Use of difference law to further break down the numerator of the example problem.

Application of root law to the denominator of the example problem.

Final evaluation of the example problem using basic limits and limit laws.

Conclusion summarizing the process of using limit laws to solve complex problems.

Transcripts

Browse More Related Video

Limit Laws | Breaking Up Complicated Limits Into Simpler Ones

Properties of Limits

CALCULUS 1: DERIVATIVES

Calculus 1 Lecture 2.3: The Product and Quotient Rules for Derivatives of Functions

AP Calculus AB - 1.5 Determining Limits Using Algebraic Properties of Limits

Limits and Limit Laws in Calculus