epsilon-delta definition ultimate introduction
TLDRThis video script offers a comprehensive guide to understanding the epsilon-delta definition for proving limits in calculus. The presenter demystifies the concept by explaining the definition, illustrating it with an example involving the square root function, and demonstrating how to write a proof using the 'four keyword' method. The script also includes a practical approach to finding an appropriate delta for a given epsilon, emphasizing the importance of logical reasoning and algebraic manipulation in constructing a proof.
Takeaways
- 📚 The script discusses the epsilon-delta definition for proving limits in calculus, which is considered a challenging topic.
- 😌 The speaker reassures that the epsilon-delta definition is not as daunting as it seems and will be explained with examples and visuals.
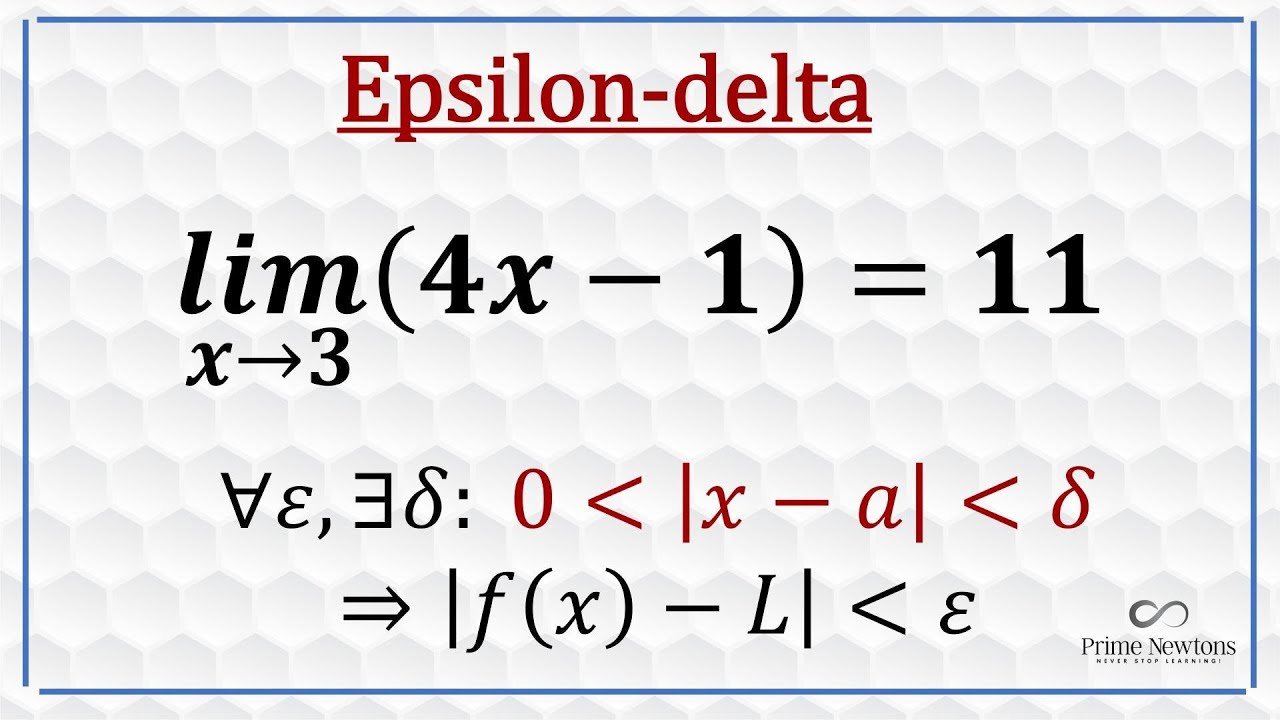
- 📝 The epsilon-delta definition is presented: for every ε > 0, there exists a δ > 0 such that if |x - a| < δ, then |f(x) - L| < ε.
- 🔍 An example is given using the limit of the function √(2x + 1) as x approaches 4, which simplifies to 3 since the function is continuous at that point.
- 🤔 The need for proof arises even when the limit seems obvious, as mathematicians require formal validation through the epsilon-delta definition.
- 📈 The concept of 'neighborhoods' is introduced, where x is within a δ-neighborhood of 'a', and f(x) is within an ε-neighborhood of 'L'.
- 📐 A graphical representation is used to visualize the limit, showing how the function values approach the limit as x gets closer to 'a'.
- 🔢 The process of selecting a specific ε value (e.g., 0.2) and determining the corresponding δ value is demonstrated through calculations.
- 📝 The 'four keyword method' for writing an epsilon-delta proof is introduced: Given, Choose, Suppose, Check.
- 🔄 The importance of using the assumptions made in the proof (e.g., |x - a| < δ) to establish the inequality |f(x) - L| < ε is emphasized.
- 📊 The script concludes with a proof example, showing how to algebraically manipulate the function to relate it to ε and ultimately choose an appropriate δ (δ = ε/2 in this case).
Q & A
What is the epsilon-delta definition used for in calculus?
-The epsilon-delta definition is used for proving limits in calculus. It establishes that for every small positive number epsilon (ε), there exists a corresponding small positive number delta (δ) such that if the input x is within a distance delta from a certain point a, then the function value f(x) is within a distance epsilon from the limit L.
Why is the epsilon-delta definition considered difficult in calculus?
-The epsilon-delta definition is considered difficult because it involves a precise mathematical language and requires a deep understanding of the concept of limits. It also demands the ability to manipulate inequalities and perform logical reasoning to construct a proof.
What is the first step in using the epsilon-delta definition to prove a limit?
-The first step is to state the limit you want to prove, which is usually in the form 'lim (as x approaches a) of f(x) equals L', and then to express this in terms of the epsilon-delta definition, which involves stating that for every epsilon > 0, there exists a delta > 0 with certain properties.
Can you provide an example of a limit that the script discusses?
-Yes, the script discusses the limit as x approaches 4 of the function f(x) = √(2x + 1), which is proven to be equal to 3.
What does the 'four keyword method' refer to in the context of the epsilon-delta definition?
-The 'four keyword method' refers to the steps involved in writing an epsilon-delta proof: 'Given', 'Choose', 'Suppose', and 'Check'. These keywords guide the structure of the proof, ensuring that all necessary components are addressed.
How does the script use an actual number and a picture to explain the epsilon-delta definition?
-The script uses the example of the limit as x approaches 4 of the function f(x) = √(2x + 1) and provides a visual representation of the function and the limit on a graph. It also uses a specific epsilon value (0.2) to demonstrate how the proof works and how to find an appropriate delta.
What is the purpose of the 'Given', 'Choose', 'Suppose', and 'Check' structure in an epsilon-delta proof?
-This structure ensures a logical flow in the proof. 'Given' states the assumptions, 'Choose' selects the delta based on epsilon, 'Suppose' assumes the condition for x is met, and 'Check' verifies that the conclusion (the function value being within epsilon of the limit) holds true under the given conditions.
How does the script demonstrate the process of finding delta in an epsilon-delta proof?
-The script provides a step-by-step process where it first assigns a specific value to epsilon, then uses the function to find the corresponding x values that would give function values within the epsilon neighborhood of the limit. It then calculates the distance between these x values and the point 'a' to determine an appropriate delta.
What is the significance of the conjugate in the 'Check' step of the epsilon-delta proof in the script?
-The conjugate is used to simplify the expression involving the square root in the function. By multiplying and dividing by the conjugate, the expression is simplified, allowing for the application of the epsilon-delta definition and the use of the given assumptions to show that the function value is within the epsilon neighborhood of the limit.
Why is it important to choose the smaller delta when there are multiple possibilities?
-Choosing the smaller delta ensures that the condition 'if the absolute value of x - a is less than delta, then the absolute value of f(x) - L is less than epsilon' is satisfied for a stricter range of x values, thus making the proof more robust.
How does the script conclude the epsilon-delta proof for the given limit?
-The script concludes by showing that after choosing delta to be epsilon over two, all conditions of the epsilon-delta definition are met. It demonstrates that if x is within the delta neighborhood of 'a', then f(x) will be within the epsilon neighborhood of the limit L, thus completing the proof.
Outlines
📚 Introduction to Epsilon-Delta Definition for Limits
The script begins with an introduction to the epsilon-delta definition used in calculus to prove limits. The speaker reassures the audience that despite its reputation for being difficult, it's manageable with the right approach. The definition is presented, which states that for any given function f(x) approaching a limit L as x approaches a, there exists a corresponding delta for any given epsilon, such that if the absolute difference between x and a is less than delta, then the absolute difference between f(x) and L is less than epsilon. The script emphasizes the importance of understanding this definition through examples, numbers, and visual representations, setting the stage for an in-depth explanation to follow.
📈 Demonstrating the Epsilon-Delta Definition with an Example
The speaker provides a practical example to illustrate the epsilon-delta definition using the function √(2x + 1) as x approaches 4. It is shown that the function's limit at this point is 3, which is derived by direct substitution. The script then transitions into a discussion about proving this limit using the epsilon-delta definition. The speaker explains the concept of 'delta neighborhood' and how it relates to the closeness of x to the point a, and the corresponding closeness of f(x) to the limit L. The explanation includes a visual representation of the function and its limit, with a focus on how the choice of epsilon affects the size of the 'epsilon neighborhood' around the limit.
🔍 Calculating Delta from Epsilon for the Epsilon-Delta Proof
This paragraph delves into the process of calculating an appropriate delta value given an epsilon for the epsilon-delta proof. The speaker uses the function √(2x + 1) and an arbitrary epsilon value of 0.2 to demonstrate the calculation. By solving the inequality that arises from setting the function's value within the epsilon neighborhood, the speaker finds the corresponding x values that satisfy the condition. The focus is on determining the distance that x can be from the point a (in this case, 4) while ensuring that f(x) remains within the epsilon neighborhood of the limit L (3). The speaker concludes by choosing the smaller of the two calculated distances as the delta value, emphasizing the importance of selecting a delta that ensures the proof's validity.
📝 Writing the Epsilon-Delta Proof with the Four Key Words
The final paragraph outlines the process of writing a formal proof using the epsilon-delta definition, focusing on four key words: 'given', 'choose', 'suppose', and 'check'. The speaker explains that the proof begins with an arbitrary epsilon and proceeds to choose an appropriate delta, which is then used to establish the conditions under which the proof holds. The script details the algebraic manipulations and logical reasoning required to show that the absolute difference between f(x) and the limit L is less than epsilon, given that the absolute difference between x and a is less than delta. The proof concludes with the selection of delta as epsilon over two, demonstrating that the chosen delta satisfies the epsilon-delta definition for any given epsilon. The speaker also addresses the relationship between the specific delta calculated in the example and the general formula derived from the proof, highlighting the flexibility and generality of the approach.
Mindmap
Keywords
💡Epsilon-Delta Definition
💡Limit
💡Continuity
💡Absolute Value
💡Inequality
💡Conjugate
💡Proof
💡Function
💡Arbitrary
💡Convergence
Highlights
Introduction to the epsilon-delta definition for proving limits in calculus.
Explanation of the epsilon-delta definition using a concrete example and visual representation.
Clarification that the limit of a function as x approaches a certain value can be proven using the epsilon-delta method.
Demonstration of the continuity of the square root function and its application in proving limits.
The necessity of proving that the limit of a function equals a specific value, exemplified with the square root function.
Description of the epsilon-delta condition for proving limits, emphasizing the relationship between x and the limit.
Illustration of how close a function's value must be to the limit, using epsilon as a measure of closeness.
Introduction of the concept of delta as a small number determining the proximity of x to the value a.
Use of a graphical representation to visually explain the epsilon-delta definition.
Discussion on the flexibility of choosing epsilon values and the resulting regions around the limit.
Explanation of how the value of the function approaches the limit as x gets closer to a, without needing to be exactly at a.
Process of finding a suitable delta value given an epsilon value in the context of the epsilon-delta definition.
Detailed walkthrough of solving for x values that correspond to a given epsilon, using the square root function as an example.
Strategy for selecting the appropriate delta value based on the proximity of x to a, ensuring the function's value is within the epsilon neighborhood.
Introduction of the four keyword method for writing epsilon-delta proofs: Given, Choose, Suppose, Check.
Step-by-step guide on writing a proof using the epsilon-delta definition, starting with the 'Given' keyword.
Explanation of how to 'Choose' a delta value that satisfies the epsilon-delta condition for a proof.
Use of the 'Suppose' keyword to establish the condition that x is within the delta neighborhood of a.
Final step of the proof process using the 'Check' keyword to verify that the function's value is within the epsilon neighborhood of the limit.
Application of algebraic manipulation and logical reasoning to complete the epsilon-delta proof.
Conclusion of the proof by showing that the absolute value of the function's value minus the limit is less than epsilon.
Emphasis on the importance of using assumptions in proofs and the correct formulation of delta in terms of epsilon.
Discussion on the practical application of the epsilon-delta definition in proving specific limit values.
Advice on choosing a delta value that is less than a computed value to ensure the proof's validity.
Encouragement to watch additional videos for further assistance with writing epsilon-delta proofs.
Transcripts
Browse More Related Video

Epsilon-delta limit definition 2 | Limits | Differential Calculus | Khan Academy
Epsilon - Delta Proof (precise definition of the limit)
Calculus 1: The Epsilon-Delta Definition of the Limit

Using the epsilon-delta defintion of a limit for a quadratic function

Epsilon delta limit (Example 2)
The precise definition of the limit EXPLAINED! (KristaKingMath)
5.0 / 5 (0 votes)
Thanks for rating: