Calculus 1: The Epsilon-Delta Definition of the Limit
TLDRThis video script delves into the epsilon-delta definition of limits, a rigorous mathematical concept not typically covered in AP Calculus but sometimes introduced by educators for a deeper understanding. The script explains the process of proving limits by finding a suitable delta for any given epsilon, ensuring that the function's Y value differs from a specified limit by epsilon when the X value differs by delta. The explanation includes step-by-step examples with linear functions and more complex scenarios, such as dealing with squared terms. It emphasizes the challenge of determining delta as a function of epsilon and provides strategies to overcome this, including making assumptions about delta's size. The script concludes by highlighting the importance of finding a delta for any arbitrary small epsilon to complete the proof, and invites viewers to explore more problems on the instructor's website.
Takeaways
- 📚 The video discusses the epsilon-delta definition of a limit, which is a rigorous mathematical concept used in calculus.
- 🔍 The main goal in epsilon-delta proofs is to find a corresponding delta for any given epsilon, ensuring that when the function's y-value differs by epsilon, the x-value differs by delta.
- 📉 For linear functions, the epsilon-delta definition is straightforward to apply, as shown with the example of the limit as x approaches 4 of 2x + 5.
- 🔢 The process involves setting up an inequality where the difference between the function's value and the limit is less than epsilon, and solving for delta.
- 🎯 In the example of 2x + 5, the delta is found to be 1/2 epsilon, which means for any epsilon, there exists a delta that ensures the x-value's difference is proportional to epsilon.
- 🤔 For non-linear functions, finding delta can be more challenging and may require assumptions or additional steps, as illustrated with the function x^2 + 1.
- 📐 The video uses the example of x^2 + 1 to demonstrate a more complex case, where an assumption is made about delta to simplify the process of finding a relationship between epsilon and delta.
- 📉 The speaker suggests that delta can be assumed to be small, which is a common approach since both epsilon and delta approach zero as the function gets closer to the limit.
- 🔎 By setting delta to be less than 1, the video derives that the absolute value of x must be less than 2, which leads to the conclusion that delta equals 1/3 epsilon.
- 📝 The video emphasizes that the choice of delta is not unique; any valid delta that corresponds to epsilon will complete the proof.
- 👍 The video concludes by encouraging viewers to explore more problems of this nature on the speaker's website, where longer videos provide further examples and explanations.
Q & A
What is the epsilon-delta definition of a limit?
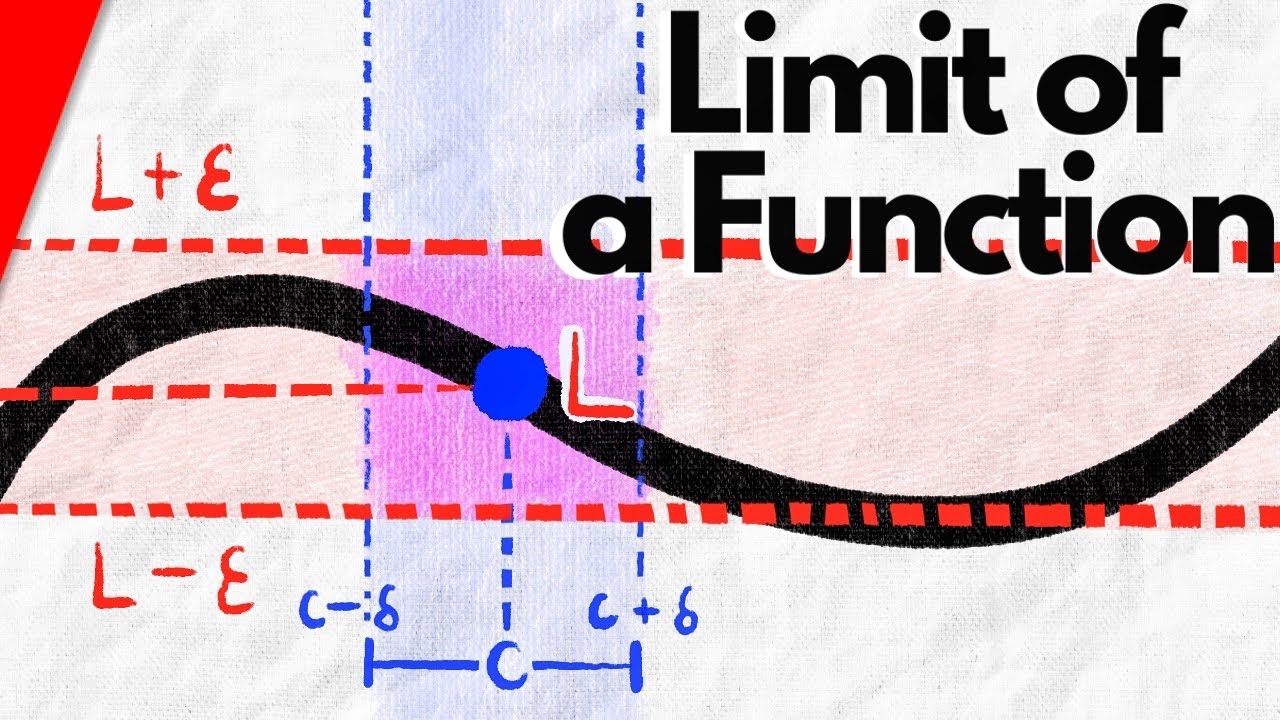
-The epsilon-delta definition of a limit is a rigorous mathematical concept used to define the limit of a function as the input approaches a certain value. It involves finding a corresponding delta for any given epsilon such that when the function's output is within epsilon of the limit, the input is within delta of the point it approaches.
Why is the epsilon-delta definition considered rigorous?
-The epsilon-delta definition is considered rigorous because it provides a precise way of defining limits by quantifying the relationship between the input and output values of a function. It ensures that for any degree of closeness (epsilon) to the limit, there is a corresponding closeness (delta) in the input values.
What is the purpose of finding both epsilon and delta in the epsilon-delta definition?
-The purpose of finding both epsilon and delta is to establish a proof that for any given epsilon (a measure of how close the function's output is to the limit), there exists a delta (a measure of how close the input needs to be to the point of approach) that ensures the function behaves as expected near the limit.
How does the process of proving a limit using the epsilon-delta definition work?
-The process involves setting up an inequality that relates the difference between the function's output and the limit (less than epsilon) and manipulating this inequality to express the input's deviation from the point of approach (less than delta). The goal is to find a functional relationship between delta and epsilon that satisfies the inequality for all sufficiently small epsilon.
Can you provide an example of a linear function and its limit from the script?
-An example from the script is the limit as x approaches 4 of the function 2x + 5. When x equals 4, the function's value is 13. The epsilon-delta definition is used to prove that for any epsilon, there exists a delta such that when the function's output is within epsilon of 13, the input x is within delta of 4.
What is the epsilon-delta definition for the function 2x + 5 as x approaches 4?
-For the function 2x + 5 as x approaches 4, the epsilon-delta definition would state that for any epsilon > 0, there exists a delta > 0 such that if 0 < |x - 4| < delta, then |2x + 5 - 13| < epsilon.
What is the significance of the absolute value in the epsilon-delta definition?
-The absolute value in the epsilon-delta definition is used to measure the magnitude of the difference between the function's output and the limit without considering the direction (positive or negative). It ensures that the definition accounts for both overshoots and undershoots of the limit.
Why might it be difficult to determine delta from epsilon in some cases?
-It might be difficult to determine delta from epsilon because the relationship between the function's output and input values can be complex and not easily invertible. In such cases, additional assumptions or algebraic manipulations are required to express delta in terms of epsilon.
How does the script handle the case where it's hard to find a direct relationship between delta and epsilon?
-The script suggests making assumptions about delta, such as setting delta to be less than 1, and then using algebraic manipulations to relate the function's output difference to delta. This allows for the derivation of a relationship between delta and epsilon that satisfies the epsilon-delta definition.
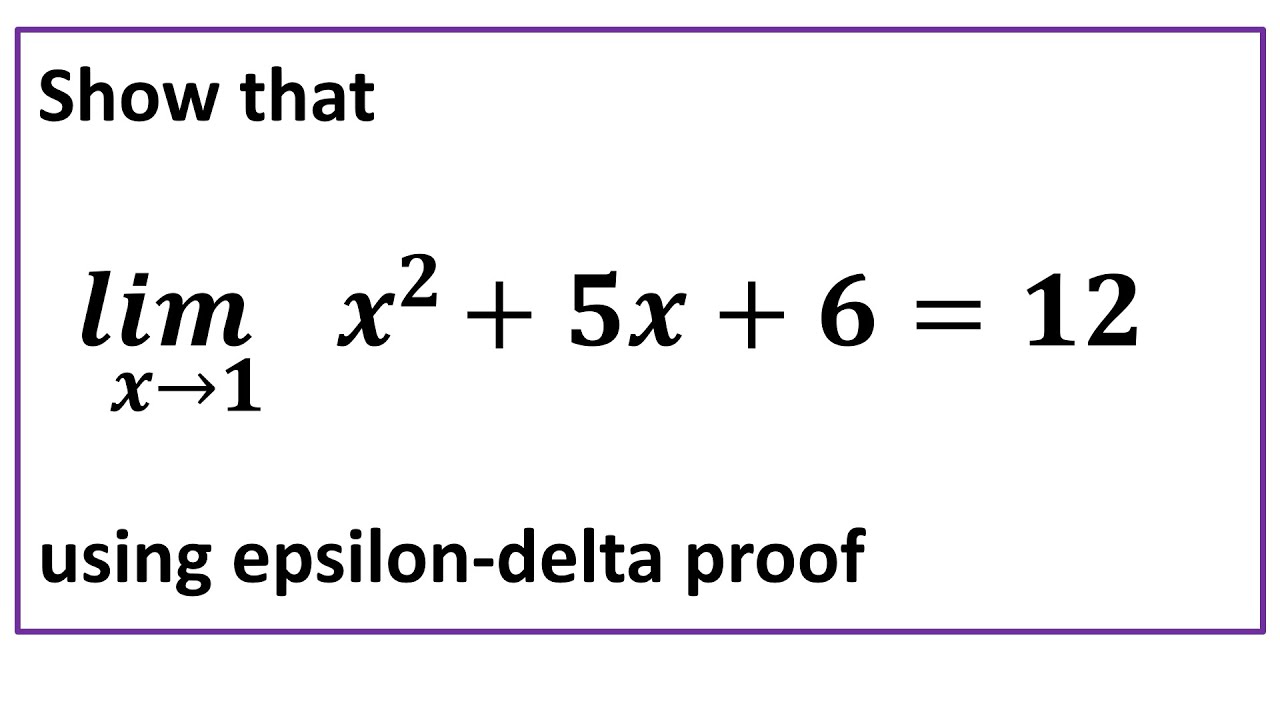
Can you give an example of a non-linear function discussed in the script and its limit?
-An example from the script is the limit as x approaches 1 of the function x^2 + 1. The script discusses the process of finding a delta for a given epsilon such that when the function's output is within epsilon of 2 (the limit), the input x is within delta of 1.
What is the final step in proving a limit using the epsilon-delta definition as illustrated in the script?
-The final step in proving a limit using the epsilon-delta definition, as illustrated in the script, is to show that for any arbitrarily small epsilon, there exists a corresponding delta such that the inequality relating the function's output and input values holds true, thus completing the proof.
Outlines
📚 Epsilon-Delta Definition of Limits
The first paragraph introduces the epsilon-delta definition of limits, which is a rigorous mathematical approach to understanding limits. The speaker explains that in epsilon-delta proofs, the goal is to find a corresponding delta for any given epsilon, ensuring that if the function's value differs from the limit by epsilon, the input x will differ by delta. The explanation uses linear functions as examples, demonstrating how to set up and solve for delta in relation to epsilon. The process involves algebraic manipulation to express the difference between the function's value and the limit in terms of epsilon and delta, ultimately finding a suitable delta that satisfies the condition for any given epsilon.
🔍 Analyzing Delta for Epsilon in Non-Linear Functions
The second paragraph continues the discussion on the epsilon-delta definition, but this time it addresses the challenge of finding delta when dealing with non-linear functions. The speaker illustrates this with the function x squared plus one, showing that it can be analytically difficult to directly determine delta from epsilon. Instead, an assumption is made that x minus one should be less than delta, and the goal is to find a suitable delta that works with this assumption. The explanation involves breaking down the absolute value expression and considering the behavior of the function as x approaches the point of interest. By setting an upper limit for delta and relating it to epsilon, the speaker demonstrates how to find a delta that ensures the function's value is within epsilon of the limit, thus completing the proof for an arbitrary small epsilon.
Mindmap
Keywords
💡Epsilon-Delta Definition
💡Limit
💡Arbitrary Epsilon
💡Delta
💡Linear Function
💡Proof
💡Absolute Value
💡Factoring
💡Analytically Hard
💡Assumption
Highlights
Introduction to the epsilon-delta definition of a limit, noting it's not typically covered in AP Calculus but some teachers may cover it for a rigorous understanding.
Explanation that the goal is to find delta for every given epsilon to show how the Y value of the function differs by epsilon when the X value differs by delta.
Example given for the limit as X approaches 4 of 2x + 5, showing the process of finding delta in relation to epsilon.
Description of simplifying the absolute value equation to find delta in terms of epsilon, showing a step-by-step algebraic process.
Completion of the proof by showing that for an arbitrary epsilon, there is a corresponding delta such that the absolute value conditions are satisfied.
Introduction to another linear function example and demonstrating the same process to find delta.
Discussion on the difficulty of finding delta for non-linear functions and introducing a quadratic example.
Explanation of the process for non-linear functions, showing how to handle absolute values and factorize to find delta.
Example of setting delta to be smaller than a particular value to simplify the problem and find a maximum bound for X.
Illustration of how to handle absolute value inequalities and setting bounds for delta in relation to epsilon.
Completion of the quadratic example by setting delta as a function of epsilon and showing how the bounds satisfy the initial conditions.
Reiteration that the specific delta value found is not unique as long as it corresponds to a given epsilon, completing the proof.
Invitation to watch more detailed problem-solving videos on the speaker's website, emphasizing the availability of free resources.
Encouragement to check the link in the description for more in-depth videos on similar mathematical problems and proofs.
Closing remarks thanking viewers and inviting them to engage with more content provided by the speaker.
Transcripts
Browse More Related Video

Precise Definition of a Limit - Example 1 Linear Function
Epsilon-Delta Definition of Functional Limits | Real Analysis

Epsilon delta limit (Example 2)

Epsilon-delta definition of limits

Formal definition of limits Part 4: using the definition | AP Calculus AB | Khan Academy
Epsilon-Delta Proof (Quadratic)
5.0 / 5 (0 votes)
Thanks for rating: