Lec 29 | MIT 18.01 Single Variable Calculus, Fall 2007

The professor begins by discussing the continuation of integration methods, specifically focusing on partial fractions. This technique is used for integrating rational functions, which are ratios of two polynomials. The class is introduced to the concept of breaking down complex rational functions into simpler components, making integration more manageable. An example is given where the function 1/(x - 1) + 3/(x + 2) is integrated, and the process is simplified by combining terms over a common denominator, leading to a disguised form that needs to be unraveled using algebraic methods.

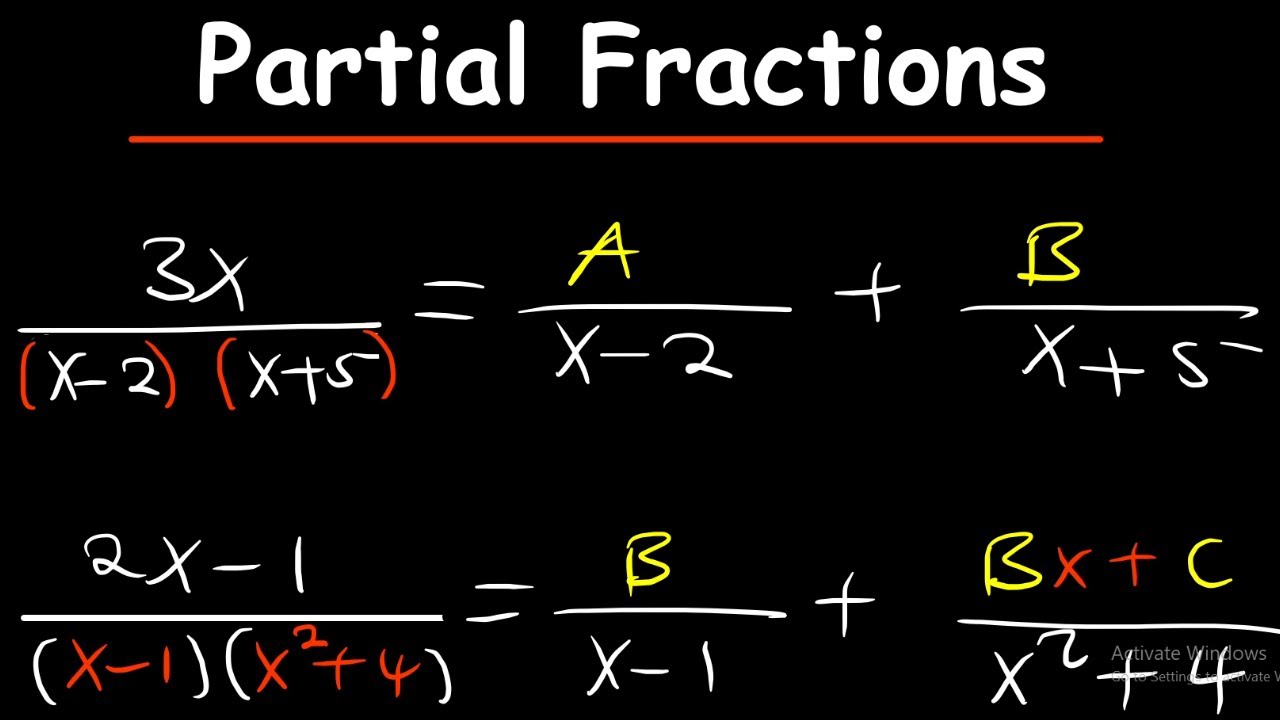
The professor introduces the cover-up method, an algebraic shortcut for dealing with partial fractions. This method involves factoring the denominator and setting up an equation with unknowns to represent the broken-down components of the original function. The goal is to solve for these unknowns efficiently. The cover-up method is demonstrated through an example where the function's denominator is factored, and unknowns A and B are introduced to represent parts of the function. The method simplifies the algebra involved in finding these coefficients by strategically multiplying and substituting values to isolate and solve for each unknown.

The professor continues to demonstrate the application of the cover-up method with a detailed example. The function's denominator is factored, and the setup involves expressing the function in terms of unknowns A and B, corresponding to the factors of the denominator. The method is used to solve for A by multiplying the equation by one of its factors and then substituting a value for x that simplifies the equation, allowing A to be easily determined. The same approach is used to find the value of B, with a different substitution. The efficiency of the method is highlighted, and the algebraic steps are explained to show how the method reduces the complexity of the problem.

The professor discusses a more complex scenario where the denominator has repeated linear factors. The setup for the partial fractions involves introducing additional terms to account for the powers of the repeated factors. An example is given where the denominator has a repeated (x - 1) factor and an (x + 2) factor. The setup includes terms for each power of the repeated factor, as well as a separate term for the non-repeated factor. The cover-up method is still applicable for finding the coefficients of the non-repeated factors, but the method for the repeated factors is slightly different and requires a bit more algebraic manipulation.

The professor provides a general guideline for setting up partial fraction decomposition when the denominator has repeated factors. An example is used to illustrate the pattern, where the denominator is a product of linear factors with repetitions. The setup involves creating terms for each power of the repeated factors, ensuring that the numerators of these terms are one degree less than the corresponding denominators. The analogy of decimal or binary expansion is used to explain the necessity of including terms for each power of the repeated factors.

The professor explains the process of solving for the coefficients in partial fractions when there are repeated factors. The cover-up method is used for some coefficients, while a more straightforward approach is taken for others. The example demonstrates how to isolate and solve for each coefficient, with a focus on simplifying the algebra as much as possible. The process involves multiplying through by the appropriate factors, setting x to values that eliminate certain terms, and solving the resulting simplified equations.

The professor moves on to a more advanced example involving a quadratic factor in the denominator. The setup for the partial fractions includes terms for linear factors as well as a first-degree polynomial term for the quadratic factor. The cover-up method is used to quickly find the coefficient associated with the linear factor. However, for the coefficients involving the quadratic factor, a more detailed algebraic approach is necessary, involving clearing the denominators and comparing coefficients on both sides of the equation.

The professor concludes the lecture by emphasizing the importance of completing the integration process after setting up and solving for the coefficients in the partial fractions. The example provided demonstrates how to integrate each term of the decomposed function, including terms with linear and quadratic factors in the denominator. The integration process involves applying the appropriate antiderivatives to each term, with a focus on accuracy and attention to detail.

In the final part of the lecture, the professor addresses the scenario where the degree of the numerator is equal to or greater than the degree of the denominator, creating an improper fraction. The solution involves reversing the factorization process and using long division to convert the improper fraction into a proper one, which can then be integrated using the previously discussed methods. The professor provides a step-by-step example of this process, highlighting the importance of careful arithmetic and the application of long division in polynomials.