Solving Systems of Equations With 3 Variables & Word Problems
TLDRThis video tutorial walks through the process of solving a system of three linear equations using elimination and substitution methods. The instructor demonstrates how to strategically combine equations to eliminate variables and simplify the system. The script also includes a step-by-step solution to a word problem involving investments with different interest rates, and another involving the cost of fruits. Each problem is methodically solved, showcasing the application of algebraic techniques in real-world scenarios.
Takeaways
- 🧩 The script explains how to solve a system of three linear equations by strategically choosing and combining equations to eliminate variables.
- 🔍 The first step involves adding the first two equations to eliminate the variable 'y', resulting in a new equation with 'x' and 'z'.
- 📚 To further eliminate 'y', the script suggests multiplying the first equation by two and adding it to the third equation, creating another equation with 'x' and 'z'.
- ➗ The next step is to negate one of the equations involving 'x' and 'z' and add it to the other to cancel out 'z' and solve for 'x'.
- 🔢 Once 'x' is found, the value is substituted back into one of the equations to solve for 'z'.
- 📉 After 'z' is determined, the original first equation is used to solve for 'y'.
- 💡 The script then transitions to a word problem involving two investments with different interest rates, aiming to find out how much was invested at a 15% interest rate.
- 📈 The investments problem is set up with two equations based on the total amount invested and the total interest earned.
- 🧮 The elimination method is again used, this time to eliminate 'y' and solve for 'x', which represents the amount invested at 15%.
- 🍎 Lastly, the script presents a problem involving the cost of apples and bananas, using 'a' for the cost of an apple and 'b' for the cost of a banana.
- 🛒 The final problem is solved by creating and manipulating two equations to find the individual costs of an apple and a banana, and then using these to calculate the total cost for seven apples and ten bananas.
Q & A
What is the first step to solve the given system of three equations involving x, y, and z?
-The first step is to choose two out of the three equations. The first two equations are chosen because adding them allows for the cancellation of the variable y.
How does adding the first two equations help in solving the system?
-Adding the first two equations results in a new equation where the variable y is eliminated, simplifying the system and allowing us to solve for x and z.
What is the resulting equation after adding the first two equations?
-The resulting equation after adding the first two equations is 4x + 3z = 13.
Why is the third equation included in the next step of the solution process?
-The third equation is included to cancel the variable y using a combination of the first and third equations, as it was not used in the initial step.
How is the first equation modified to cancel y when combined with the third equation?
-The first equation is multiplied by two to align the coefficients of y with those in the third equation, resulting in 4x + 2y + 2z = 14.
What is the second equation in terms of x and z after combining the modified first equation with the third equation?
-The second equation in terms of x and z is 5x + 3z = 14.
Why is one of the equations multiplied by negative one in the next step?
-Multiplying one of the equations by negative one is done to facilitate the elimination of the variable z when the two equations are added together.
What is the simplified equation for x after adding the modified equations?
-The simplified equation for x is x = 1.
How is the value of z determined after finding the value of x?
-The value of z is determined by substituting x = 1 into the second equation in terms of x and z (5x + 3z = 14), which simplifies to 3z = 9, and then solving for z to get z = 3.
What is the final step to find the value of y in the system of equations?
-The final step is to use the original first equation (2x + y + z = 7) with the found values of x and z to solve for y. Substituting x = 1 and z = 3 gives y + 5 = 7, leading to y = 2.
How does the script transition from solving algebraic equations to word problems involving investments?
-After demonstrating the method to solve a system of equations, the script applies a similar approach to word problems involving investments, using algebra to represent and solve real-world scenarios.
What is the strategy used to solve the word problem involving two investments with different interest rates?
-The strategy involves setting up a system of two equations based on the total investment and total interest received, then using algebraic manipulation and elimination to solve for the amount invested at each interest rate.
How is the interest earned in each account calculated after determining the amount invested?
-The interest earned in each account is calculated by multiplying the principal amount invested in that account by its respective interest rate.
What is the approach to solving the word problem about the cost of apples and bananas?
-The approach involves setting up a system of two equations with variables representing the cost of one apple (a) and one banana (b). By solving this system, the individual costs are determined, which are then used to calculate the total cost for seven apples and ten bananas.
How are the equations manipulated to eliminate one of the variables (b) in the apples and bananas problem?
-The equations are manipulated by multiplying the first equation by -7 and the second by 8, which aligns the coefficients of b, allowing for its elimination when the equations are added together.
What is the cost of one apple and one banana as determined by the script?
-The cost of one apple is determined to be 75 cents, and the cost of one banana is 35 cents.
How is the total cost of seven apples and ten bananas calculated?
-The total cost is calculated by multiplying the number of apples by the cost of one apple, the number of bananas by the cost of one banana, and then summing these products to get a total of eight dollars and seventy-five cents.
Outlines
📚 Solving a System of Three Equations
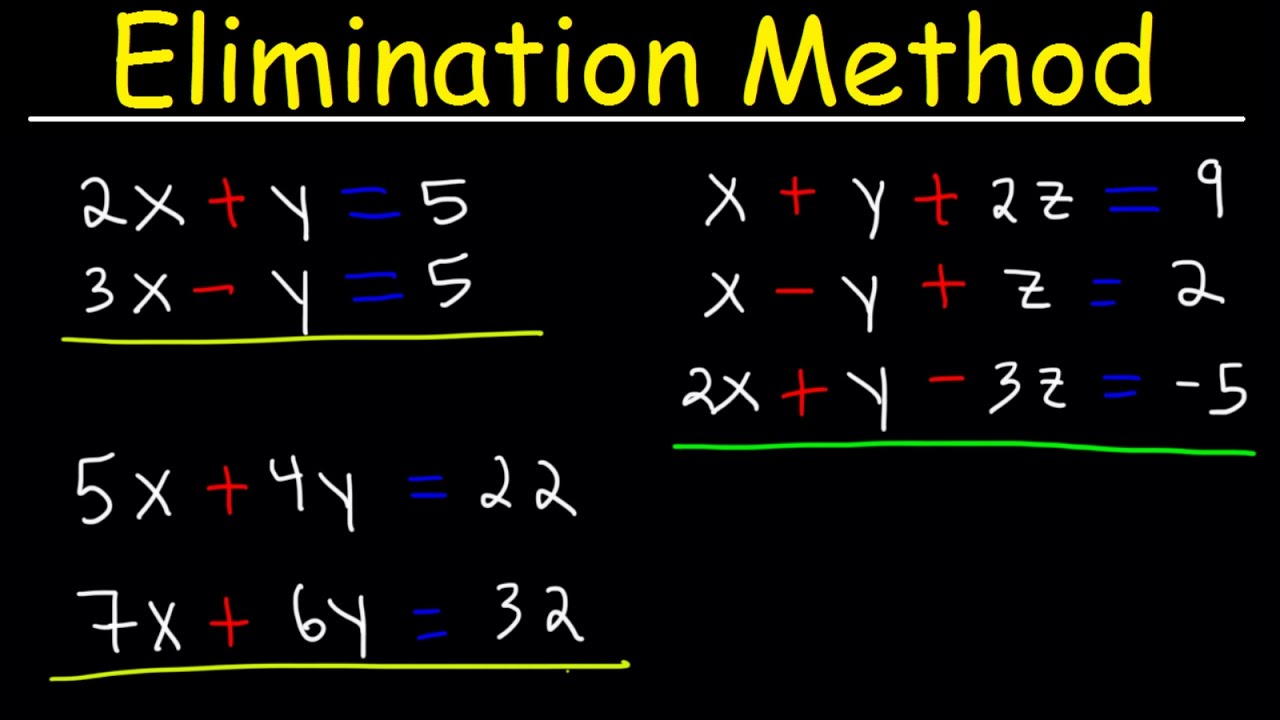
This paragraph demonstrates a step-by-step method for solving a system of three linear equations. The equations given are 2x + y + z = 7, 2x - y + 2z = 6, and x - 2y + z = 0. The process involves strategically choosing and combining equations to eliminate variables. By adding the first two equations, y is canceled out, resulting in 4x + 3z = 13. Then, by using the first and third equations and manipulating them to cancel y, a new equation 5x + 3z = 14 is formed. Afterward, by negating and adding the simplified equations, x and z are solved, finding x = 1 and z = 3. Substituting these values back into the original equations, y is found to be 2. The solution to the system is (x, y, z) = (1, 2, 3).
🏦 Two Investments Word Problem
The second paragraph presents a word problem involving two investments with different interest rates. The total investment is $13,000, and the accounts earn 15% and 14% annually. The total interest earned in the first year is $1,900. To solve, the paragraph outlines setting up a system of two equations based on the given information: x + y = 13000 and 0.15x + 0.14y = 1900. The equations are then multiplied by 100 to eliminate decimals and solved using the elimination method. By multiplying the first equation by -14 and adding it to the second, x is isolated, revealing that $8,000 was invested at 15% interest. Consequently, $5,000 was invested at 14% interest. The interest calculations confirm the total interest earned: $1,200 from the first account and $700 from the second, summing to $1,900.
🍌 Apples and Bananas Cost Problem
In the final paragraph, a cost problem involving apples and bananas is tackled. The cost of five apples and eight bananas is given as $6.55, and the cost of nine apples and seven bananas is $9.20. Variables a and b are assigned to the cost of one apple and one banana, respectively. Two equations are formed: 5a + 8b = 6.55 and 9a + 7b = 9.20. To eliminate b, the first equation is multiplied by -7 and the second by 8, resulting in a new system of equations. Solving this system yields the cost of one apple as $0.75 and one banana as $0.35. Using these individual costs, the total cost for seven apples and ten bananas is calculated to be $8.75.
Mindmap
Keywords
💡System of equations
💡Elimination method
💡Substitution method
💡Interest calculation
💡Percentages
💡Variables
💡Combining equations
💡Word problems
💡Coefficient
💡Solution set
Highlights
Introduction to solving a system of three equations using elimination method.
Choosing the first two equations to cancel variable y by addition.
Rewriting the equations to simplify the process.
Combining the first and third equations to eliminate y again.
Multiplying the first equation by two to align coefficients for elimination.
Adding the modified first equation with the third equation to find a new equation in terms of x and z.
Multiplying one of the equations by negative one to facilitate further elimination.
Eliminating z to find the value of x.
Solving for x and finding it to be equal to 1.
Substituting x's value into the equation to solve for z.
Finding the value of z to be 3.
Using the original first equation to solve for y.
Determining the value of y to be 2.
Presenting the solution as x = 1, y = 2, z = 3.
Transitioning to word problems involving investments and interest rates.
Setting up equations for two investments with given total amounts and interest rates.
Converting percentages to decimals to simplify calculations.
Using the total interest received to form a second equation.
Eliminating y by multiplying the first equation by negative 14.
Solving for x, the amount invested at 15% interest.
Calculating the amount invested at 14% interest by subtracting from the total.
Verifying the solution by calculating the interest earned from each account.
Introduction to a word problem involving the cost of apples and bananas.
Formulating equations based on the cost of apples and bananas.
Using elimination method to solve for the cost of a single apple (a).
Calculating the cost of each apple to be 75 cents.
Solving for the cost of a single banana (b) using the first equation.
Determining the cost of each banana to be 35 cents.
Calculating the total cost for seven apples and ten bananas.
Final calculation showing the cost of seven apples and ten bananas as $8.75.
Transcripts
Browse More Related Video
Elimination Method For Solving Systems of Linear Equations Using Addition and Multiplication, Algebr

Simultaneous Equations - Tons of Examples!

SOLVING SYSYEM OF NONLINEAR EQUATIONS || PRECALCULUS

How To Solve Word Problems of Any Kind . Nutshell maths-2

Gauss Jordan Elimination & Reduced Row Echelon Form

Ch. 10.2 Systems of Linear Equations in Several Variables
5.0 / 5 (0 votes)
Thanks for rating: