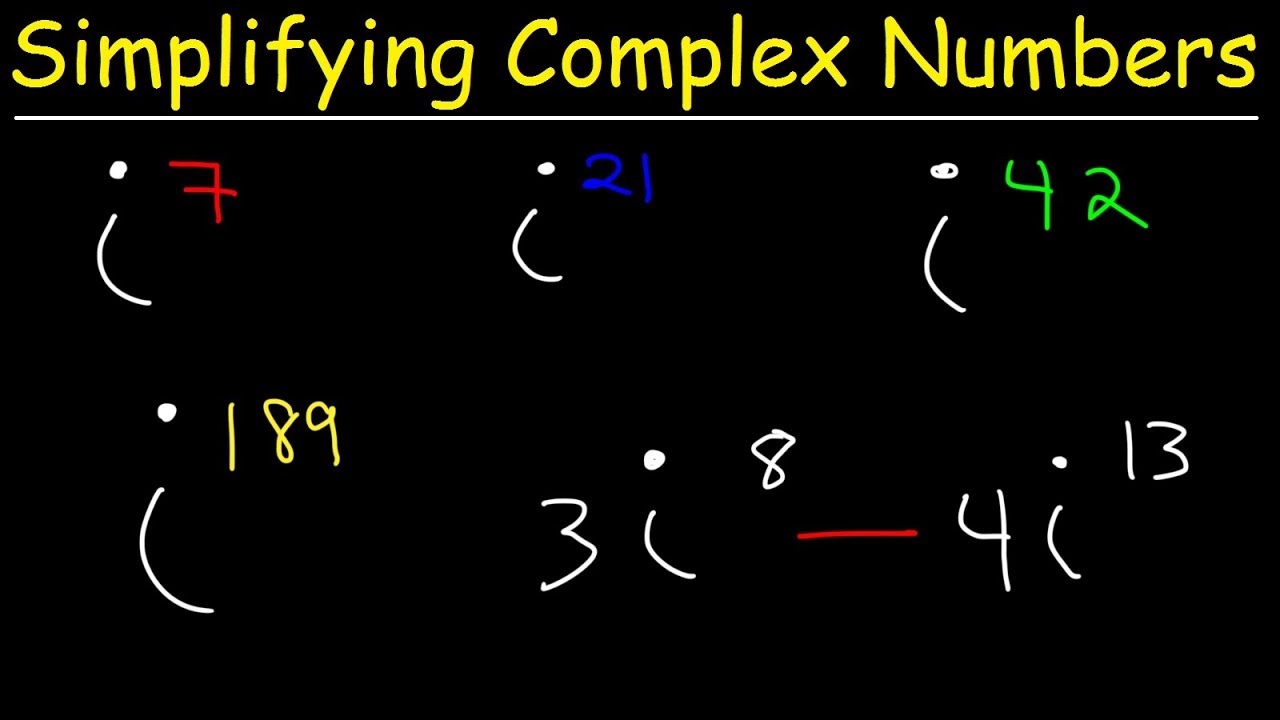
Multiplying Complex Numbers
TLDRThis educational video script delves into the intricacies of complex number multiplication, focusing on the imaginary unit 'i', where i^2 equals -1. It walks through several examples, starting with basic multiplication of complex numbers like 7i * 8i, resulting in -56. It progresses to more complex scenarios, such as multiplying negative 4i by negative 3i, yielding -12. The script also tackles operations involving the square root of negative numbers, like √(-6) * √(-30), resulting in -6√5i. The explanation includes simplifying expressions like 5i * (3 + 4i) and demonstrates how to multiply conjugate complex numbers, such as (6 + 4i) * (6 - 4i), which results in a real number, 52. The script concludes with an example of squaring a complex number, (2 + 3i)^2, resulting in -5 + 12i, highlighting the importance of combining like terms and correctly applying the properties of 'i'.
Takeaways
- 📚 When multiplying \(7i\) by \(8i\), remember that \(i\) is the square root of \(-1\), so \(i^2 = -1\). Thus, \(56i^2 = 56(-1) = -56\).
- 🧮 For \( -4i \times -3i \), multiply \(-4\) by \(-3\) to get \(12\), and \(i \times i = i^2 = -1\). Thus, \(12i^2 = 12(-1) = -12\).
- 🔢 To simplify \( 5i \times (3 + 4i) \), multiply \(5i\) by \(3\) and by \(4i\), resulting in \(15i\) and \(20i^2 = 20(-1) = -20\). Combine to get \(-20 + 15i\).
- 📝 When multiplying the square roots of negative numbers, such as \(\sqrt{-6} \times \sqrt{-30}\), express each as \(\sqrt{6}\sqrt{-1}\) and \(\sqrt{6}\sqrt{5}\sqrt{-1}\). Simplify to get \(-6\sqrt{5}\).
- ⚖️ The square root of \(-25\) times \(\sqrt{-9}\) involves expressing each as \(\sqrt{25}\sqrt{-1}\) and \(\sqrt{9}\sqrt{-1}\). This simplifies to \(15i^2 = 15(-1) = -15\).
- 🔄 For \( (3 + 4i) \times (5 - 2i) \), use the FOIL method: \(3 \times 5 = 15\), \(3 \times -2i = -6i\), \(4i \times 5 = 20i\), and \(4i \times -2i = -8i^2 = 8\). Combine to get \(23 + 14i\).
- 🧩 Multiplying conjugates \( (6 + 4i) \times (6 - 4i) \) results in \(36 - 24i + 24i - 16i^2\). Simplify to get \(36 + 16 = 52\).
- 🧠 Squaring \( (2 + 3i) \) involves using the FOIL method: \(2 \times 2 = 4\), \(2 \times 3i = 6i\), \(3i \times 2 = 6i\), and \(3i \times 3i = 9i^2 = -9\). Combine to get \(-5 + 12i\).
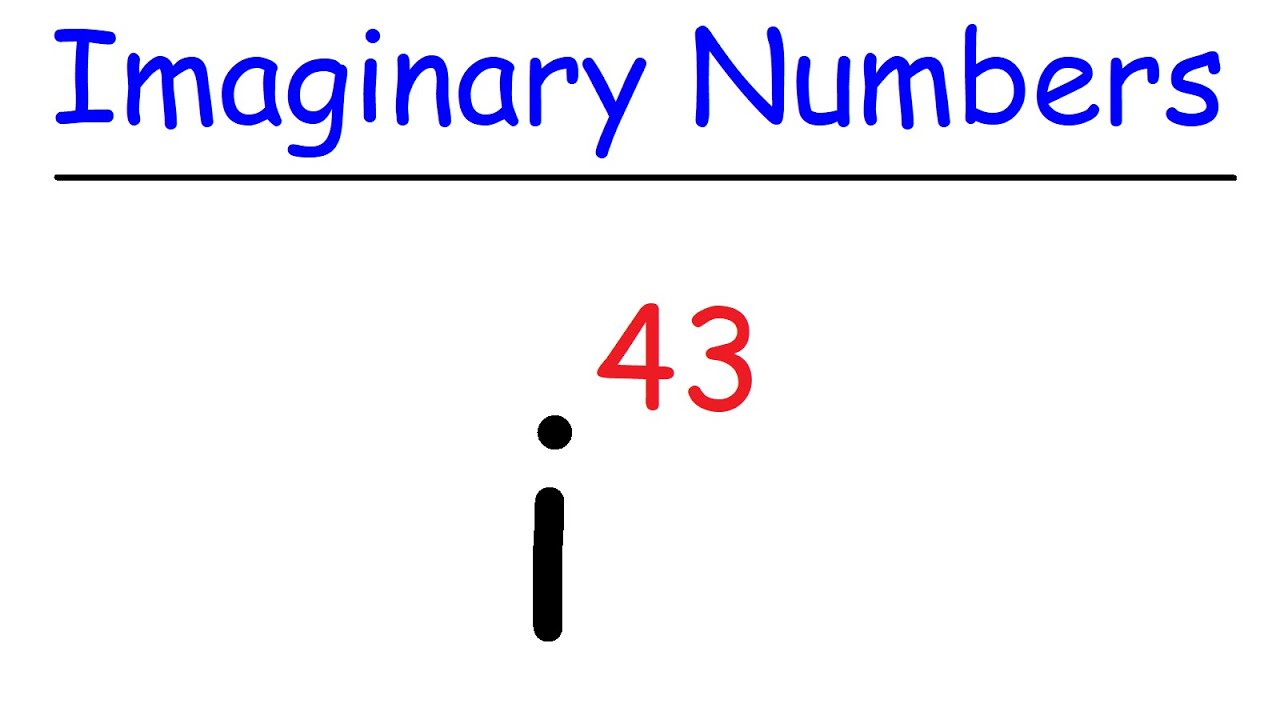
- 📏 Remember that \(i\) represents the square root of \(-1\), and \(i^2 = -1\). This is crucial for simplifying expressions involving imaginary numbers.
- 🧮 When multiplying complex numbers, combine like terms and simplify using \(i^2 = -1\) to find the final answer in the form \(a + bi\).
Q & A
What is the result of multiplying 7i by 8i?
-The result is -56. This is calculated by multiplying the real parts (7 * 8 = 56) and the imaginary parts (i * i = -1), so 56 * -1 equals -56.
What is the product of -4i and -3i?
-The product is 12. Multiplying the real parts (-4 * -3 = 12) and knowing that i^2 = -1, the result is a positive real number, 12.
How do you simplify the expression 5i multiplied by 3 + 4i?
-You distribute 5i to both terms: 5i * 3 = 15i and 5i * 4i = 20i^2. Since i^2 = -1, this becomes 20 * -1 = -20. The simplified expression is 15i - 20.
What is the result of multiplying the square root of negative six by the square root of negative thirty?
-The result is -6√5i. This is derived from (√6 * √(-1)) * (√6 * √(-1)) = 6√5 * i^2, and since i^2 = -1, it becomes -6√5.
What is the final result when you multiply the square root of negative 25 by the square root of negative nine?
-The final result is -15. This is because √(-25) * √(-9) = 5 * 3i * i, and since i^2 = -1, it simplifies to 15i * -1 = -15.
How do you multiply (3 + 4i) by (5 - 2i) using the FOIL method?
-You multiply each term in the first binomial by each term in the second: (3*5) + (3*-2i) + (4i*5) + (4i*-2i) = 15 - 6i + 20i - 8i^2. Since i^2 = -1, it simplifies to 15 + 14i - 8 = 23 + 14i.
What happens when you multiply a complex number by its conjugate, like (6 + 4i) * (6 - 4i)?
-The middle terms (the real and imaginary parts) cancel out, and you are left with a real number. In this case, 6*6 + (-4i)(4i) = 36 + 16 = 52.
How do you expand and simplify (2 + 3i) squared?
-You treat it as (2 + 3i)(2 + 3i) and apply the FOIL method: 2*2 + 2*3i + 3i*2 + 3i*3i = 4 + 6i + 6i + 9i^2. Combining like terms and knowing i^2 = -1, it simplifies to 4 - 9 + 12i = -5 + 12i.
What is the significance of i^2 in complex number calculations?
-i^2 is equal to -1. This is a fundamental property of imaginary units and is used to simplify expressions involving complex numbers.
Why do the imaginary parts disappear when you multiply a complex number by its conjugate?
-When multiplying a complex number by its conjugate, the cross terms (involving i) add up to zero because they are additive inverses of each other, leaving only the real parts, resulting in a real number.
Outlines
🧮 Operations with Complex Numbers and i
This paragraph explains the basic operations with complex numbers involving 'i', where 'i' is the imaginary unit equal to the square root of -1. It demonstrates multiplication of complex numbers, such as 7i multiplied by 8i resulting in -56, and negative 4i by negative 3i resulting in -12. The process of simplifying expressions like 5i times (3 + 4i) to -20 + 15i is also covered. Additionally, it shows how to handle square roots of negative numbers, like √(-6) * √(-30) which equals -6√5i, and √(-25) * √(-9) which equals -15. The paragraph concludes with multiplying (3 + 4i) by (5 - 2i) and simplifying it to 23 + 14i.
🔍 Multiplication of Complex Numbers and Their Conjugates
This paragraph delves into the multiplication of complex numbers and their conjugates. It begins with the multiplication of 6 + 4i by 6 - 4i, resulting in a real number 52, as the imaginary parts cancel each other out. The explanation continues with expanding (2 + 3i) squared, which involves the distributive property (FOIL method) and results in -5 + 12i after combining like terms and simplifying. The summary underscores the concept that multiplying a complex number by its conjugate yields a real number, eliminating the imaginary component.
Mindmap
Keywords
💡i (imaginary unit)
💡Complex numbers
💡Multiplication
💡Standard form
💡Conjugate
💡Square root
💡Exponentiation
💡Foil method
💡Combining like terms
💡Simplification
Highlights
7i multiplied by 8i equals -56, demonstrating the multiplication of complex numbers involving 'i'.
i is defined as the square root of negative one, and i squared equals negative one.
Negative 4i multiplied by negative 3i results in positive 12, showcasing the product of negative imaginary numbers.
5i multiplied by 3 plus 4i simplifies to -20 + 15i, illustrating the multiplication of a complex number by a real number.
The square root of negative numbers is expressed as the product of the square root of the absolute value and 'i'.
Square root of negative six times the square root of negative thirty equals -6√5i, combining square roots of negative numbers.
Square root of negative 25 times the square root of negative nine results in -15, multiplying square roots of negative numbers.
Three plus four i times five minus 2i simplifies to 23 + 14i, using the FOIL method for complex numbers.
Multiplying a complex number by its conjugate results in a real number, as demonstrated with 6 + 4i times 6 - 4i.
(2 + 3i) squared equals -5 + 12i, expanding a complex number squared.
The middle terms of the product of a complex number and its conjugate cancel out, leaving a real number.
Combining like terms in complex number multiplication is crucial for accurate results.
Double-checking work in complex number multiplication ensures accuracy.
The importance of understanding 'i' and its properties in complex number operations is emphasized.
Complex number multiplication can be simplified using standard algebraic methods.
The transcript provides a step-by-step guide to multiplying complex numbers, including those involving 'i'.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: