Calculus 1: Motion Problem Examples

Allen Tsao The STEM Coach
5 Aug 201903:23
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script discusses solving motion problems by integrating the concepts of derivatives and integrals. The instructor begins by explaining how to find the displacement function X(t) from a given velocity function V(t), using integration and applying initial conditions to solve for the constant of integration. The script continues with deriving the acceleration function from the velocity function, showcasing the process of differentiation. Examples include solving for displacement with a velocity function of -32t + 20 and acceleration with a cosine function, where the instructor also corrects a minor mistake regarding the sign of the derivative. The video concludes with a prompt for viewers to visit the instructor's website for more calculus problem-solving examples.

Takeaways
  • 🔢 Motion problems involve both derivatives and integrals.
  • 📈 To find displacement X(t), take the antiderivative of the velocity function V(t).
  • 🔄 Displacement formula: X(t) = ∫ V(t) dt.
  • 🧮 Example: Given V(t) = -32t + 20, X(t) is found by integrating V(t).
  • 📝 Initial values help determine the constant of integration.
  • ⚙️ In the example, X(0) = 0 helps find C, resulting in X(t) = -16t^2 + 20t.
  • 🚀 Acceleration a(t) is the derivative of the velocity function.
  • 📉 Example: For V(t) = 10, X(t) is found to be 10t + 10 using the initial value X(0) = 10.
  • 📊 Another example: V(t) = cos(t) leads to X(t) = sin(t) - 5 given X(0) = -5.
  • 🧑‍🏫 Acceleration for V(t) = cos(t) is found to be -sin(t).
Q & A

  • What is the relationship between derivatives and integrals in motion problems?

    -In motion problems, derivatives and integrals are related as follows: derivatives are used to find the velocity and acceleration of an object, while integrals are used to find the displacement and the distance traveled by the object.

  • What is the formula to find displacement in terms of velocity?

    -The formula to find displacement (X of T) is the integral of the velocity function (V of T) with respect to time (DT), which means X of T is the antiderivative of V of T.

  • How do you find the initial value of the displacement function given an initial condition?

    -To find the initial value of the displacement function, you plug in the initial condition (e.g., X of 0) into the displacement function and solve for the constant of integration (C).

  • What is the velocity function given in the script?

    -The velocity function given in the script is V of T, which is -32 times one-half T squared plus 20 T.

  • What is the displacement function X of T derived from the velocity function?

    -The displacement function X of T is derived from the velocity function by taking the antiderivative, resulting in -16 T squared plus 20 T plus C.

  • What is the acceleration function a of T?

    -The acceleration function a of T is the derivative of the velocity function V of T. In the script, it is found to be -32.

  • How do you find the constant of integration C in the displacement function?

    -To find the constant of integration C, you use the initial condition where X of 0 equals zero. By plugging in 0 into the displacement function, you can solve for C.

  • What is the process to find the displacement function X of T when given a velocity function V of T?

    -The process involves taking the antiderivative of the velocity function V of T to get the displacement function X of T, and then using the initial condition to solve for the constant of integration.

  • What is the correct antiderivative of the velocity function 10 T?

    -The correct antiderivative of the velocity function 10 T is 5 T squared plus C, where C is the constant of integration.

  • How do you find the acceleration function a of T when given a velocity function V of T?

    -To find the acceleration function a of T, you take the derivative of the velocity function V of T. In the script, the derivative of cosine of T is found to be negative sine of T.

  • What is the mistake made in the script regarding the derivative of the velocity function?

    -The mistake in the script is the incorrect sign for the derivative of the velocity function. The correct derivative of cosine of T is negative sine of T, but the script incorrectly states it as positive sine of T.

  • What is the correct displacement function if the initial condition is X of 0 equals negative five?

    -The correct displacement function, given the initial condition X of 0 equals negative five, would be negative cosine T plus C, where C is found to be negative four.

  • What is the final form of the acceleration function a of T in the script?

    -The final form of the acceleration function a of T in the script is sine of T minus five.

  • Where can one find more examples and step-by-step solutions for calculus problems?

    -More examples and step-by-step solutions for calculus problems can be found on the speaker's website, which offers free access to over 400 calculus questions.

Outlines
00:00
📚 Introduction to Motion Problems in Calculus

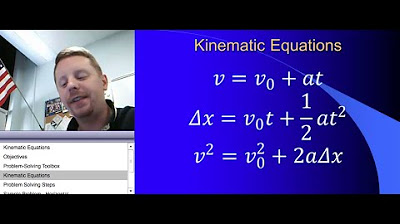
This paragraph introduces the topic of motion problems, which are common in calculus and involve both derivatives and integrals. The speaker explains the importance of understanding the relationship between these two concepts and how to solve problems in both directions. The main focus is on finding the displacement and acceleration of an object moving in a straight line, given its velocity function and an initial value.

Mindmap
Keywords
💡Motion Problems
Motion problems are a fundamental concept in physics and calculus, involving the study of an object's movement over time. In the context of this video, motion problems are used to illustrate the application of calculus in determining an object's displacement and acceleration when given its velocity function. The script discusses how to solve these problems by integrating and differentiating the velocity function.
💡Derivatives
Derivatives in calculus represent the rate of change of a function with respect to its variable. They are integral to motion problems as they allow us to find the acceleration of an object from its velocity function. In the video, the derivative of velocity is taken to find the acceleration, as seen when the script states 'the derivative of velocity derivative this is negative 32'.
💡Integrals
Integrals are the reverse process of derivatives and are used to calculate the accumulated change in a function over an interval. In the context of motion problems, integrals help us find the displacement of an object when given its velocity function. The script demonstrates this by integrating the velocity function to find 'X of T', the displacement function.
💡Velocity Function
A velocity function, denoted as V(t) in the script, is a mathematical representation of an object's speed at any given time 't'. It is a key component in motion problems as it provides the necessary information to calculate displacement and acceleration. The script uses the velocity function to derive both displacement and acceleration.
💡Displacement
Displacement refers to the change in position of an object from its initial point to its final point. In the script, displacement is calculated by integrating the velocity function over time, resulting in the function 'X of T', which represents the object's position at any time 't'.
💡Antiderivatives
Antiderivatives, also known as indefinite integrals, are functions that 'undo' the process of differentiation. They are used to find the original function when given its derivative. In the video, the script calculates the antiderivative of the velocity function to find the displacement function 'X of T'.
💡Initial Value
An initial value is a specific value assigned to a variable at the start of a problem or experiment. In the context of the video, the initial value is used to determine the constant of integration in the displacement function. The script uses the fact that 'X of 0 is equal to zero' to solve for the constant 'C'.
💡Acceleration
Acceleration is the rate at which an object's velocity changes over time. It is found by taking the derivative of the velocity function. The script demonstrates this by taking the derivative of the velocity function to find 'a of T', the acceleration function.
💡Constant of Integration
The constant of integration arises when finding antiderivatives and represents an arbitrary constant added to the indefinite integral. It accounts for the fact that when integrating, we are finding a family of functions that differ by a constant. In the script, the constant 'C' is determined using the initial condition 'X of 0 is equal to zero'.
💡Trigonometric Functions
Trigonometric functions, such as sine and cosine, are mathematical functions that relate angles to the ratios of two sides of a right triangle. In the video, the script encounters a velocity function involving cosine, and the antiderivative of cosine is discussed, which is negative sine plus a constant.
Highlights

Introduction to motion problems involving derivatives and integrals.

Understanding the relationship between derivatives and integrals in motion problems.

Finding displacement X of T using the integral of velocity function V of T.

Calculating X of T as the antiderivative of V of T.

Initial value problem setup with X of zero equals zero.

Determining the constant of integration C to be zero.

Deriving acceleration a of T from the velocity function.

Solving for X of T with a given initial condition X of 0 equals 10.

Finding the constant of integration C to be 10.

Using the antiderivative to find X of T when V of T is cosine of T.

Determining the constant of integration when X is zero.

Correcting the sign error in the derivative of velocity.

Final expression for acceleration a of T as sine of T minus five.

Derivative of cosine resulting in negative sine of T.

Resource recommendation for more calculus examples and solutions.

Transcripts

Browse More Related Video

2023 AP Calculus AB FRQ #2

Equations of Motion by Graphical Method

2018 AP Calculus AB Free Response #2

Integral of a Product

High School Physics: Kinematic Equations

Calculus - Working with position, velocity, and acceleration

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TutorialMotion ProblemsDerivativesIntegralsDisplacementVelocityAccelerationAntiderivativeTrigonometric FunctionsEducational ResourceFree Access