Visualizing a binomial distribution | Probability and Statistics | Khan Academy

Khan Academy
26 Nov 201409:26
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the concept of a random variable 'x', representing the number of heads obtained from flipping a fair coin five times. It delves into calculating the probabilities of x taking on values from zero to five and visually plotting these probabilities to illustrate the binomial distribution. The script explains the discrete nature of the distribution and its significance in statistics. Furthermore, it discusses how increasing the number of trials would lead to a distribution that approximates a normal distribution, commonly known as the bell curve. The binomial distribution is highlighted as a foundational concept in understanding statistical distributions and their applications.

Takeaways
  • 🎲 The video discusses a random variable 'x' representing the number of heads obtained from flipping a fair coin five times.
  • 📊 The script explains the process of plotting the probability distribution of the random variable x, which can take values from zero to five.
  • 📉 The probabilities for each value of x are calculated and visualized using a histogram, with values ranging from 1/32 to 10/32.
  • 📈 The video illustrates the concept of a discrete probability distribution, highlighting that x can only take on specific, finite values.
  • 🔢 The distribution is characterized by its symmetry and is identified as a binomial distribution due to the use of binomial coefficients in calculating probabilities.
  • 📚 The importance of the binomial distribution in statistics is emphasized, as it is often assumed for many discrete processes.
  • 🌐 The script suggests that with a large number of trials, such as flipping a coin millions of times, the binomial distribution approaches a bell curve.
  • 📉 The bell curve is associated with a normal distribution, which is a continuous probability distribution and is significant in science and statistics.
  • 🔑 The video explains the relationship between the binomial and normal distributions, and how the former can approximate the latter with a large number of trials.
  • 💡 It is pointed out that assumptions about underlying distributions, such as binomial or normal, are common in various fields but should be considered carefully to avoid misinterpretations.
  • 🎨 The presenter prefers hand-drawing the histogram over using a computer graph to maintain a personal touch and find the process therapeutic.
Q & A

  • What is the random variable x defined as in the script?

    -The random variable x is defined as the number of heads obtained from flipping a fair coin five times.

  • What is the purpose of plotting the probabilities of the random variable x?

    -Plotting the probabilities helps to visualize the probability distribution of the random variable x, giving a sense of how likely each outcome is.

  • What is the highest probability value for the random variable x in the script?

    -The highest probability value for the random variable x is 10/32, which corresponds to getting exactly two or three heads in five coin flips.

  • How is the probability distribution of a discrete random variable represented in the script?

    -The probability distribution of a discrete random variable is represented by a histogram, where each bar corresponds to a possible value of x and its height represents the probability of that value occurring.

  • What is the significance of the binomial distribution in statistics?

    -The binomial distribution is significant in statistics because it is often assumed as the underlying distribution for many discrete processes, making it important for modeling and analyzing such processes.

  • How does the binomial distribution relate to the normal distribution?

    -As the number of trials in a binomial distribution increases, the distribution starts to resemble a bell curve, which is characteristic of the normal distribution. This is known as the Central Limit Theorem.

  • What is the difference between a discrete and a continuous probability distribution?

    -A discrete probability distribution is defined for a finite or countably infinite number of distinct values, while a continuous probability distribution can take on any value within an interval and is represented by a probability density function.

  • Why might a normal distribution be assumed in economic models?

    -A normal distribution might be assumed in economic models because it represents the aggregate effect of many random processes, such as interactions among molecules or humans, which can lead to a bell-shaped distribution of outcomes.

  • What is the role of binomial coefficients in determining the probabilities of a binomial distribution?

    -Binomial coefficients, derived from combinatorics, are used to calculate the probabilities of each outcome in a binomial distribution, reflecting the number of ways a particular result can occur.

  • What could be the implications of assuming a normal distribution when it is not appropriate?

    -Assuming a normal distribution when it is not appropriate can lead to incorrect predictions and risk assessments, potentially causing issues such as economic crises if the tails of the actual distribution are fatter than those of a normal distribution.

  • How does the script illustrate the transition from a binomial to a normal distribution?

    -The script illustrates this transition by suggesting that if the random variable x represented the number of heads from flipping a coin five million times instead of five, the resulting distribution would start to look more like a bell curve, approaching a normal distribution.

Outlines
00:00
📊 Plotting the Probability Distribution of a Discrete Random Variable

This paragraph discusses the process of visualizing the probability distribution of a discrete random variable, specifically the number of heads (x) obtained from flipping a fair coin five times. The speaker explains how to plot the outcomes on a graph with two axes: one for the different outcomes (0 to 5 heads) and the other for the corresponding probabilities (from 1/32 to 10/32). A histogram is created to represent these probabilities, with bars indicating the likelihood of getting zero to five heads. The speaker emphasizes the discrete nature of the distribution, highlighting that it can only take on a finite number of values and cannot take on any value in between the specified outcomes.

05:00
📚 Understanding the Binomial Distribution and Its Relation to the Normal Distribution

The second paragraph delves into the concept of a finite discrete random variable and introduces the binomial distribution. It explains that this distribution arises from scenarios where there are a finite number of trials, each with two possible outcomes, and is characterized by its symmetrical shape and discrete probabilities. The speaker also discusses how probabilities in a binomial distribution can be calculated using binomial coefficients, which are related to combinatorics. Furthermore, the paragraph explores the idea that as the number of trials increases, the binomial distribution begins to resemble a bell curve, which is indicative of a normal distribution. This connection is significant in statistics, as it shows how discrete processes can approximate continuous ones under certain conditions. The speaker also touches on the importance of understanding the underlying assumptions of distributions in various fields, such as economics, where incorrect assumptions can lead to significant errors.

Mindmap
Keywords
💡Random Variable
A random variable is a variable whose value is determined by outcomes of a random phenomenon. In the context of the video, the random variable 'x' represents the number of heads obtained from flipping a fair coin five times. This concept is central to the video's theme, as it helps to quantify and analyze the outcomes of a probabilistic event.
💡Probability
Probability is a measure of the likelihood that a particular event will occur. The video discusses the probabilities of the random variable 'x' taking on values from zero to five, which are calculated based on the outcomes of flipping a coin. The script uses these probabilities to illustrate the distribution of the random variable.
💡Fair Coin
A fair coin is a coin that has an equal chance of landing on either heads or tails when flipped. In the video, a fair coin is used to demonstrate the concept of a random variable and its probability distribution, with the assumption that each flip is an independent event with a 50/50 chance.
💡Probability Distribution
A probability distribution is a statistical description of a random variable that specifies the probability of each possible outcome. The video script describes plotting the probability distribution of 'x', the number of heads, to visualize the likelihood of each outcome from zero to five heads.
💡Histogram
A histogram is a graphical representation of the distribution of a set of data. In the video, the instructor creates a histogram to represent the probability distribution of the random variable 'x', showing the probabilities of getting zero to five heads when flipping a coin five times.
💡Binomial Distribution
A binomial distribution is a discrete probability distribution of the number of successes in a fixed number of independent experiments, each asking a yes/no question, and each with its own boolean outcome. The video explains that the distribution of the random variable 'x' is a binomial distribution, as it represents the number of successes (heads) in five independent coin flips.
💡Binomial Coefficients
Binomial coefficients are used in combinatorics to count the number of ways a certain number of items can be chosen from a larger set. The video mentions that the probabilities in a binomial distribution can be calculated using binomial coefficients, which relate to the number of combinations of outcomes.
💡Normal Distribution
A normal distribution, also known as a Gaussian distribution, is a continuous probability distribution that is characterized by its symmetric bell-shaped curve. The video script discusses how as the number of trials (coin flips) increases, the binomial distribution approaches a normal distribution, which is a fundamental concept in statistics.
💡Continuous Probability Distribution
A continuous probability distribution is one where any value within an interval has a non-zero probability of occurring. The video explains that with a large number of trials, the discrete binomial distribution begins to resemble a continuous distribution, specifically a normal distribution.
💡Combinatorics
Combinatorics is a branch of mathematics concerned with counting, combination, and permutation of sets. The video script implies that combinatorics is used to calculate the probabilities in a binomial distribution through the use of binomial coefficients.
💡Economic Crises
An economic crisis is a period of financial instability that results in a significant downturn in an economy. The video script briefly touches on how assumptions about probability distributions, such as the normal distribution, can have real-world implications, including the potential for economic crises if the underlying assumptions are incorrect.
Highlights

Introduction of a random variable x representing the number of heads from flipping a fair coin five times.

Calculation of probabilities for x taking values from zero to five.

Visualization of the random variable's probability distribution through plotting.

Explanation of discrete probability distribution characteristics.

Illustration of the binomial distribution with a histogram.

Description of the probabilities in terms of 32nds, with the highest being 10/32.

Hand-drawn histogram to represent the probabilities of x taking different values.

The concept of a finite discrete random variable explained.

Introduction to the binomial distribution and its significance in statistics.

Explanation of how probabilities are derived using binomial coefficients and combinatorics.

Discussion on the transition from a binomial distribution to a normal distribution as the number of trials increases.

Visual representation of how a binomial distribution approaches a bell curve with a large number of trials.

Differentiation between discrete and continuous probability distributions.

Importance of understanding the binomial and normal distributions in various scientific and statistical contexts.

Potential implications of incorrectly assuming a normal distribution in fields like economics.

Final reflection on the process of visualizing a binomial distribution and its relation to a normal distribution.

Transcripts

Browse More Related Video

Binomial distribution | Probability and Statistics | Khan Academy

Constructing a probability distribution for random variable | Khan Academy

Math 119 Chapter 5 part 2

Binomial Distribution EXPLAINED in UNDER 15 MINUTES!

Free throw binomial probability distribution | Probability and Statistics | Khan Academy

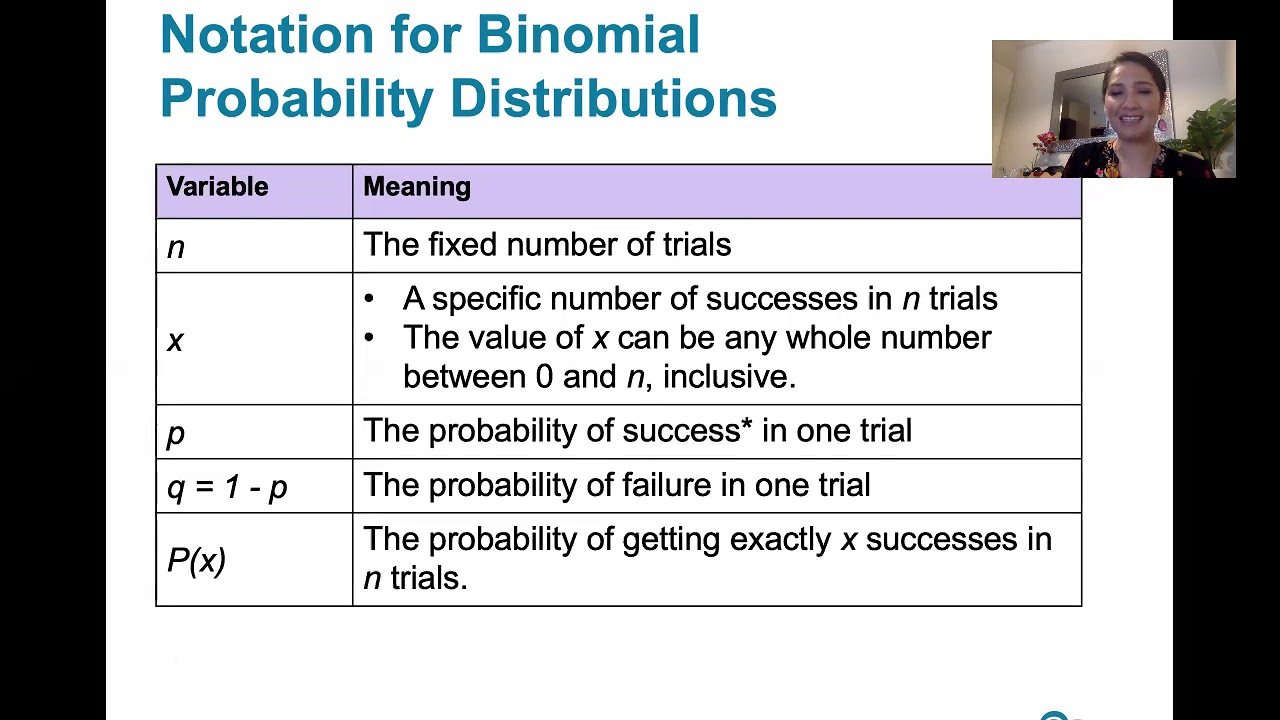
5.2.1 Binomial Probability Distributions - Is this procedure described by a binomial distribution?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Binomial DistributionProbability TheoryStatistical AnalysisDiscrete Random VariableFlipping CoinsHistogram PlotCombinatoricsNormal DistributionStatistical SimulationEducational Content