Lesson 5 - L'hopitals Rule (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis advanced calculus tutorial introduces L'Hôpital's Rule, a powerful and easy-to-understand method for evaluating limits that are not straightforward to solve by direct substitution. The instructor provides motivation by discussing the complexity of limits involving infinity in the early stages of calculus and demonstrates how L'Hôpital's Rule simplifies the process. The video aims to equip viewers with the tools to tackle challenging limits, such as 0/0 and infinity/infinity forms, with practical examples and a clear explanation of the rule's application.

Takeaways
  • 📚 The class is focused on a section of advanced calculus known as L'Hôpital's Rule.
  • 🗣️ The correct pronunciation of L'Hôpital's Rule is emphasized, highlighting its French origin.
  • 👨‍🏫 L'Hôpital's Rule is named after the person who discovered it and is presented as a simple concept once explained.
  • 🔍 The rule is particularly useful for evaluating limits that are not easily determined by direct substitution.
  • 📘 The script begins with a background on limits, a fundamental topic in calculus that is often studied in detail.
  • 🤔 The challenge of evaluating limits where both the numerator and denominator approach infinity is discussed.
  • 🍋 The script uses L'Hôpital's Rule to simplify the process of finding limits that would otherwise require extensive proofs and diagrams.
  • 📉 An example is given where the limit of 1/(2+x) as x approaches 0 is easily calculated to be 1/2.
  • 🚫 The script points out the difficulty of evaluating limits in the form of 0/0 without the proper mathematical tools.
  • ♾️ The concept of limits where x approaches infinity and the form of infinity/infinity is introduced as a common scenario tackled by L'Hôpital's Rule.
  • 💡 The overall message is that L'Hôpital's Rule provides a powerful and easy method to evaluate complex limits.
Q & A

  • What is the correct pronunciation of 'L'Hopital's Rule'?

    -The correct pronunciation is 'L'hopital's rule', which is named after the French mathematician who discovered it.

  • What is L'Hopital's Rule used for?

    -L'Hopital's Rule is used to evaluate limits that are not easily determined by simply plugging in the number, especially when dealing with indeterminate forms such as 0/0 or ∞/∞.

  • Why is L'Hopital's Rule considered powerful?

    -L'Hopital's Rule is considered powerful because it can quickly evaluate certain limits that would otherwise require extensive proofs and drawings, as often seen in the early sections of calculus textbooks.

  • What is the limit of 1/(2+x) as x approaches 0?

    -The limit of 1/(2+x) as x approaches 0 is 1/2, because as x approaches 0, the expression simplifies to 1/2.

  • What happens when you try to evaluate the limit of x/x^2 as x approaches 0?

    -When x approaches 0, both the numerator and the denominator become 0, resulting in an indeterminate form of 0/0, which cannot be directly evaluated without further tools like L'Hopital's Rule.

  • What is the indeterminate form 0/0?

    -The indeterminate form 0/0 occurs when both the numerator and the denominator of a fraction approach zero as the variable approaches a certain value, making it impossible to determine the limit without additional methods.

  • What is the indeterminate form ∞/∞?

    -The indeterminate form ∞/∞ occurs when both the numerator and the denominator of a fraction approach infinity as the variable approaches a certain value, similarly requiring further analysis to find the limit.

  • What is the limit of 2^x/x^2 as x approaches infinity?

    -The limit of 2^x/x^2 as x approaches infinity results in an indeterminate form of ∞/∞, which suggests that the limit grows without bound, but L'Hopital's Rule can be applied to find the exact behavior.

  • Why might a limit problem be considered difficult at the beginning of a calculus course?

    -A limit problem might be considered difficult at the beginning of a calculus course because students have not yet been introduced to advanced tools like L'Hopital's Rule and may struggle with indeterminate forms.

  • How does L'Hopital's Rule simplify the process of evaluating certain limits?

    -L'Hopital's Rule simplifies the process by allowing the direct application of differentiation to both the numerator and the denominator of an indeterminate form, which can then be used to find the limit.

Outlines
00:00
📚 Introduction to L'Hôpital's Rule

This paragraph introduces the topic of L'Hôpital's Rule, a concept in advanced calculus that many students find challenging. The instructor clarifies the correct pronunciation and emphasizes its importance, as it simplifies the evaluation of limits that are not easily determined by direct substitution. The paragraph provides a brief background on limits, which are typically the first topic studied in calculus, and highlights the common issue of indeterminate forms like 0/0 or ∞/∞. The instructor promises that L'Hôpital's Rule will make solving such problems much easier and sets the stage for a deeper dive into the concept.

Mindmap
Keywords
💡L'Hopital's Rule
L'Hopital's Rule is a fundamental concept in calculus used to evaluate limits that involve indeterminate forms, such as 0/0 or infinity/infinity. It is named after the French mathematician Guillaume de l'Hôpital. In the video, the tutor introduces L'Hopital's Rule as a powerful and easy method to find limits that are not easily solvable by direct substitution. The rule states that if the limit of the ratio of two functions results in an indeterminate form, then this limit is equal to the limit of the ratio of their derivatives.
💡Indeterminate Forms
Indeterminate forms are expressions that do not have a determinate value, such as 0/0 or infinity/infinity. They often arise when evaluating limits in calculus. In the script, the tutor discusses indeterminate forms as a common challenge in evaluating limits, especially at the beginning of calculus studies, and positions L'Hopital's Rule as a solution to handle these forms effectively.
💡Limits
Limits are a core concept in calculus that describe the behavior of a function as the input approaches a certain value. The video script begins with a discussion on limits, emphasizing their importance in the early stages of learning calculus. Limits are used to understand the value a function approaches but does not necessarily reach, as in the examples provided in the script, such as 1/(2+x) as x approaches 0.
💡Derivative
The derivative of a function measures how the function changes as its input changes. It is a fundamental concept in calculus that is closely related to the concept of limits. In the context of L'Hopital's Rule, derivatives play a crucial role, as the rule involves taking the derivative of the numerator and denominator functions to resolve indeterminate forms.
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. It is divided into differential calculus, which studies the concept of the derivative, and integral calculus, which deals with the concept of the integral. The video script focuses on a specific aspect of differential calculus, namely L'Hopital's Rule, which is used to evaluate limits within the broader study of calculus.
💡Convergence
In the context of limits, convergence refers to the property of a sequence or series approaching a certain value or behavior. The script mentions that textbooks devote pages to proving why certain limits converge. L'Hopital's Rule simplifies this process by providing a direct method to evaluate such limits.
💡Infinity
Infinity is a concept that represents an unbounded quantity that is larger than any number. In calculus, especially when dealing with limits, infinity is used to describe a situation where a variable grows without bound. The script provides examples of limits where the variable x approaches infinity, resulting in expressions like 2^x/x^2, which involve the concept of infinity.
💡Direct Substitution
Direct substitution is the process of plugging the value of the variable directly into the function to evaluate its value. The script mentions that direct substitution is not always possible or helpful, especially when dealing with indeterminate forms. L'Hopital's Rule offers an alternative method for evaluating limits when direct substitution leads to an indeterminate form.
💡Exponential Function
An exponential function is a mathematical function of the form f(x) = a^x, where 'a' is a constant. In the script, the tutor uses the example of 2^x to illustrate a limit involving an exponential function, which is a common scenario where L'Hopital's Rule can be applied.
💡Power Function
A power function is a function of the form f(x) = x^n, where 'n' is a real number and 'x' is the variable. The script includes x^2 as an example of a power function, which is used in the context of a limit as x approaches infinity, demonstrating the application of L'Hopital's Rule to evaluate such limits.
Highlights

Introduction to L'Hôpital's Rule in advanced calculus.

Common pronunciation mistake of L'Hôpital's Rule and its correct French pronunciation.

L'Hôpital's Rule named after its discoverer and its simplicity once explained.

The rule's utility in evaluating limits that are not easily solvable by direct substitution.

Background on the study of limits in calculus, often the first topic covered.

The typical approach to teaching limits before derivatives in most textbooks.

The challenge of evaluating limits that result in infinity over infinity.

L'Hôpital's Rule as a powerful tool to quickly solve complex limit problems.

An example of a simple limit calculation as x approaches 0 for 1/(2+x).

Explanation of the 0/0 indeterminate form and its common occurrence in limits.

The difficulty in evaluating limits of the form 0/0 without advanced tools.

An example of a limit as x approaches infinity for 2^x/x^2.

The indeterminate form of infinity over infinity and its complexity.

The upcoming section's focus on teaching methods to evaluate difficult limits.

Assurance that L'Hôpital's Rule will be easy to understand once explained.

Motivation for students by tackling limits that are initially challenging.

Transcripts

Browse More Related Video

Example of Using L'Hopital's Rule Twice to Evaluate a Limit

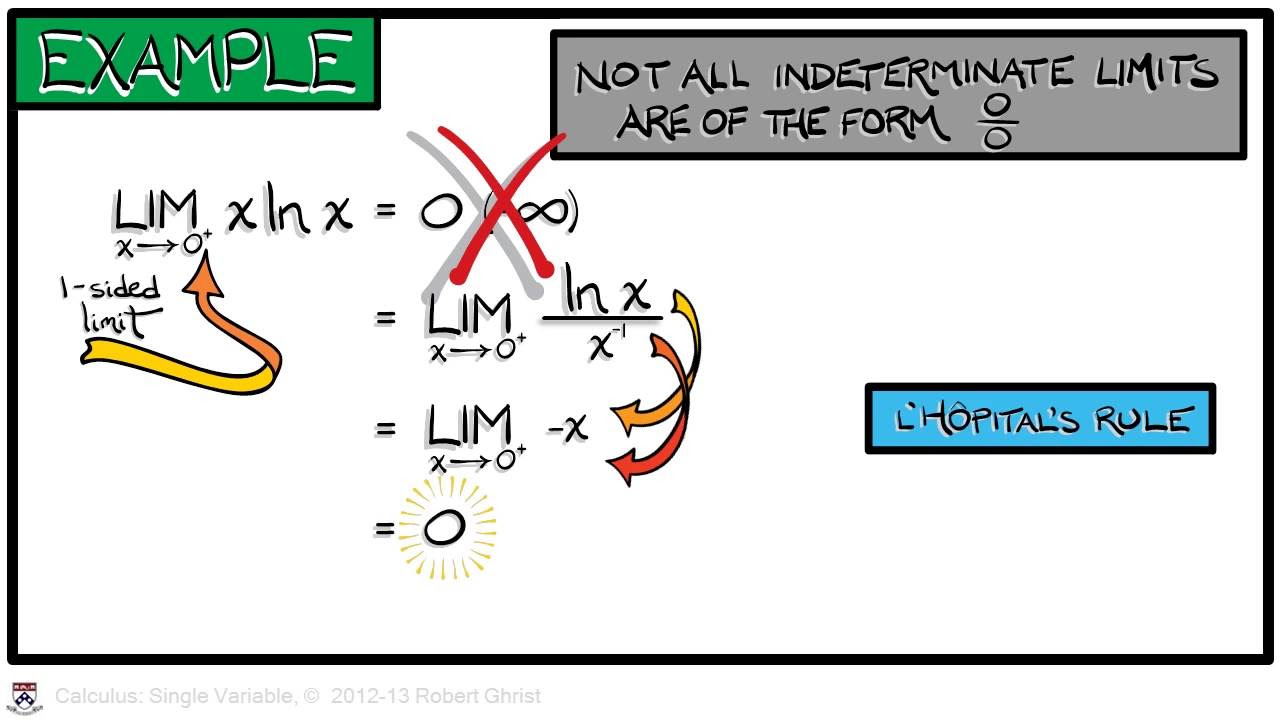
Calculus Chapter 1 Lecture 8 l'Hopital's Rule

Using L'Hopital's Rule to show that exponentials dominate polynomials

Other Indeterminate Forms

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy

Limits and Continuous Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusL'Hôpital's RuleLimitsDerivativeEducationInfinite LimitsMathematicsTutoringAdvanced MathLimit Evaluation