College Physics 1: Lecture 21 - Solving Circular Dynamics Problems
TLDRIn this lecture on circular dynamics, the instructor explains how to solve problems involving objects moving in circular paths. Despite constant speed, objects in circular motion experience acceleration due to continuous direction change, resulting in a force toward the circle's center. The lecture covers drawing free body diagrams, applying Newton's laws, and using equations to find forces and speeds. Two detailed examples and several questions illustrate these concepts, preparing students for more complex topics in the next lecture.
Takeaways
- 📚 The lecture focuses on solving circular dynamics problems, which involve objects moving in a circular path with constant speed but changing direction, resulting in acceleration.
- 🔄 In circular motion, an object is always accelerating due to the continuous change in direction, even though the speed remains constant, hence there's always a centripetal force acting on the object.
- 📐 The strategy for solving circular dynamics problems involves drawing a free body diagram with the x-axis pointing towards the center of the circle, writing Newton's laws for forces in the x and y directions, and solving the equations.
- 🧭 A unique aspect of circular motion problems is that the x-axis is always drawn pointing towards the center of the circle, regardless of the orientation of the motion.
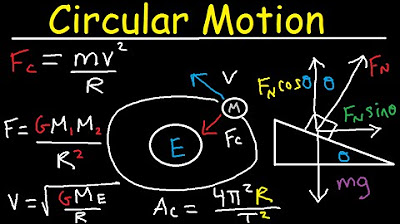
- ⚖️ In circular motion, the sum of forces in the x-direction results in an equation of the form F = mv^2/r, where F is the force acting towards the center of the circle, m is the mass, v is the velocity, and r is the radius.
- 🚫 In the y-direction of circular motion, the sum of forces equals zero, meaning any forces present balance each other out, simplifying the problem-solving process.
- 🔢 To solve for unknowns in circular motion, one must first determine the speed, which can be found using the net force equation or circular kinematics, and then use this speed to find other related quantities like period or frequency.
- 👨👦 The first example problem involves a child on a cart spinning in a circle with a given tension, mass, and radius, and the goal is to find the period of rotation, which is achieved by first calculating the speed and then the period.
- 🛣️ The second example problem discusses the maximum safe speed for a car to navigate a circular curve on a rainy day, taking into account the static friction coefficient and the radius of the curve.
- 🔄 The importance of correctly identifying the forces acting on an object in circular motion is emphasized, especially distinguishing between tension, weight, and normal force, and understanding their directions.
- 🎢 Special cases, such as a roller coaster car at the top of a loop-de-loop, are introduced, hinting at the discussion of apparent forces in the next lecture, which will further elaborate on situations where the normal force acts in unexpected directions.
Q & A
What is the primary force acting on an object in circular motion, even when moving at a constant speed?
-The primary force acting on an object in circular motion is the centripetal force, which is always directed towards the center of the circular path. This force is responsible for the continuous change in direction of the object, even if its speed remains constant.
Why is it necessary to draw a free body diagram when solving circular dynamics problems?
-A free body diagram is essential for visualizing all the forces acting on an object in a particular situation. In the context of circular dynamics, it helps to identify the centripetal force and other relevant forces, such as weight and normal force, which are crucial for applying Newton's laws to solve the problem.
How does the axis orientation change when drawing a free body diagram for circular motion problems?
-Unlike previous problems where the x and y axes are typically horizontal and vertical, for circular motion problems, the x-axis is always drawn pointing towards the center of the circle. This change in axis orientation simplifies the mathematics involved in solving the problems.
What is the relationship between the centripetal force and the velocity of an object in circular motion?
-The centripetal force (F) acting on an object in circular motion is directly proportional to the mass (m) of the object, the square of its velocity (v^2), and inversely proportional to the radius (r) of the circular path. The relationship is given by the equation F = m * v^2 / r.
Why is it important to consider the direction of the net force in circular motion?
-The direction of the net force is important because it always points towards the center of the circular path, which is where the centripetal force is applied. Understanding this direction helps in determining the forces acting on the object and in setting up the correct equations to solve for unknowns such as speed or period of rotation.
What is the formula for calculating the period of rotation in circular motion?
-The period (T) of rotation in circular motion, which is the time taken to complete one full circle, can be calculated using the formula T = 2 * π * r / v, where r is the radius of the circular path and v is the speed of the object.
How does the mass of an object affect the calculation of the speed in circular motion?
-The mass of the object (m) appears in the formula for calculating the speed (v) in circular motion as v = √(F * r / m), where F is the centripetal force and r is the radius of the circular path. The mass of the object inversely affects the speed, meaning that for a given force and radius, a larger mass will result in a lower speed.
What is the significance of the coefficient of static friction (μs) in the context of a car traversing a curve on a rainy day?
-The coefficient of static friction (μs) represents the maximum frictional force that can be exerted between the tires and the road before the car starts to skid. On a rainy day, with a reduced μs, the maximum safe speed at which a car can traverse a curve without skidding is lower to ensure safety.
Why is it incorrect to include a forward force in the free body diagram of a car coasting over the top of a hill?
-A forward force is not included in the free body diagram of a coasting car because, in the absence of friction and air resistance, there is no physical force propelling the car forward. The car's motion is due to its inertia, and the only forces acting on it are gravity (weight) and the normal force from the surface of the hill.
What is the role of the normal force in the free body diagram of a roller coaster car at the top of a loop-de-loop?
-At the top of a loop-de-loop, the normal force acts downward, opposing the weight of the roller coaster car. This is because the surface of the track is above the car, and the normal force always pushes back against the object, even when the surface is not horizontal but part of the circular loop.
How does the problem-solving strategy for circular dynamics simplify the process of solving for forces and motion?
-The problem-solving strategy for circular dynamics simplifies the process by guiding the solver through a systematic approach: drawing a free body diagram with the x-axis always pointing towards the center of the circle, writing out Newton's laws for the x and y directions, solving for the speed using the net force equation, and then using circular kinematics to find other quantities like period or frequency. This structured approach helps in organizing the problem and identifying the necessary steps to find the solution.
Outlines
📚 Introduction to Solving Circular Dynamics Problems
In Lecture 21 of College Physics 1, the focus is on solving circular dynamics problems. The lecture begins by revisiting the concept of circular motion, where an object moves in a circular path at a constant speed but with a continuously changing direction, resulting in acceleration. The necessity of a centripetal force to keep the object in its circular path is emphasized. The lecture's goal is to teach problem-solving strategies for forces in circular motion, including drawing free body diagrams and applying Newton's laws. A unique aspect of these problems is the orientation of the x-axis, which always points toward the center of the circle, differing from the standard Cartesian coordinate system.
📐 Problem-Solving Strategy for Circular Dynamics
The lecture outlines a problem-solving strategy for circular dynamics problems, which involves drawing a free body diagram with a twist: the x-axis is always drawn pointing toward the center of the circle, regardless of the orientation of the motion. This approach simplifies the math involved in solving the problems. The strategy also includes writing Newton's laws for the forces in the x and y directions. It is highlighted that in circular motion problems, the x equation often simplifies to mv^2/r, derived from F=ma, where 'a' is the centripetal acceleration v^2/r. The y direction typically results in a net force of zero, as the forces balance out. The importance of determining the speed first, either through force equations or circular kinematics, is stressed as it is essential for solving other aspects of the problem.
👨👦 Example 1: Child on a Cart in Circular Motion
The first example problem involves a father spinning his 20 kg child on a 5 kg cart using a 2-meter rope with a tension of 100 N. The goal is to find the time taken for one complete rotation, which translates to finding the period of the motion. The problem-solving strategy is applied by drawing a free body diagram, identifying the forces acting on the child (weight, normal force, and tension), and setting up Newton's laws accordingly. The tension force in the x direction is equated to mv^2/r, and the normal force equals the weight in the y direction. The speed of the child is calculated using the given tension, radius, and total mass. With the speed determined, the period is then found using the relationship between speed, distance (2πr), and time (period).
🚗 Example 2: Maximum Speed for a Level Curve on a Rainy Day
The second example addresses a level curve with a 150-meter radius and seeks to determine the maximum speed at which a car can safely traverse the curve on a rainy day with a static friction coefficient (μs) of 0.4. The problem introduces the concept of static friction as the force that prevents the car from sliding off the road. Newton's second law is applied in both the x and y directions. The x direction involves static friction as the centripetal force, while the y direction confirms that the normal force equals the weight. The challenge is to find the maximum speed without causing the car to skid. The solution involves using the friction equation (μs * N) and the normal force (N = mg) to solve for the speed, which turns out to be approximately 24 m/s.
🔍 Reviewing Free Body Diagrams for Circular Motion Scenarios
The lecture concludes with a review of free body diagrams for various circular motion scenarios, emphasizing the correct identification of forces acting on objects in motion. Three questions are presented, each with a different scenario: a pendulum swinging back and forth, a car coasting over a hill, and a roller coaster car at the top of a loop-de-loop. For each scenario, the correct free body diagram is chosen based on the forces present, such as weight, tension, and normal force. The importance of recognizing that there is no additional force propelling the object in the direction of motion is highlighted, and the correct diagrams are identified for each scenario.
🎢 Special Case: Roller Coaster Loop-de-Loop Free Body Diagram
A special case is discussed involving a roller coaster car at the top of a loop-de-loop. The normal force is highlighted as being downward, contrary to the common misconception that it acts upward. This is due to the surface of the track being above the car, which means the normal force acts in the opposite direction to the weight, both pointing downward. This scenario is set up to lead into the next lecture, which will delve deeper into apparent forces in circular motion.
Mindmap
Keywords
💡Circular Motion
💡Acceleration
💡Velocity
💡Force
💡Free Body Diagram
💡Newton's Laws
💡Tension
💡Period
💡Static Friction
💡Coefficient of Friction
Highlights
Introduction to solving circular dynamics problems in physics, emphasizing the importance of understanding forces in circular motion.
Explanation of why an object in circular motion at constant speed is still accelerating due to the continuous change in direction.
The necessity of a centripetal force to keep an object in a circular path, preventing it from moving in a straight line.
Introduction of a problem-solving strategy for circular dynamics, starting with a free body diagram and applying Newton's laws.
The unique approach to drawing axes for circular motion problems, with the x-axis always pointing toward the center of the circle.
Different scenarios for drawing free body diagrams based on whether the motion is in a horizontal or vertical plane.
Newton's laws applied to circular motion, specifically the equation mv^2/r for the x-axis force.
Clarification that the sum of forces in the y-direction in circular motion is always zero, simplifying calculations.
The importance of determining the speed in circular motion problems as it is essential for further calculations.
A step-by-step example problem involving a child on a cart spinning in a circle, demonstrating the application of the problem-solving strategy.
Calculation of the period of rotation for the child on the cart, using the derived equations and given values.
Another example problem calculating the maximum safe speed for a car on a circular road during rainy conditions.
Use of friction and normal force equations to determine the maximum speed before skidding occurs.
The cancellation of mass (m) in the equations, indicating it is not required for solving the problem.
Practice questions on drawing free body diagrams for different circular motion scenarios, reinforcing the concepts learned.
Discussion on the forces acting on a pendulum, a coasting car, and a roller coaster car at the top of a loop, emphasizing the correct free body diagrams.
Conclusion of the lecture with a preview of the next topic: apparent forces in circular motion.
Transcripts
Browse More Related Video

College Physics 1: Lecture 15 - Equilibrium and Newton's Second Law

1.6 - Tangential Acceleration
Centripetal Acceleration & Force - Circular Motion, Banked Curves, Static Friction, Physics Problems

Uniform Circular Motion Free Body Diagrams

High School Physics - Vertical Circular Motion

Physics 1 Final Exam Review
5.0 / 5 (0 votes)
Thanks for rating: