Einstein's General Theory of Relativity | Lecture 2

The program is introduced by Stanford University, and the speaker sets the stage for a discussion on dark energy. Dark energy, also referred to as the cosmological constant or vacuum energy, is explained as a force causing the accelerated expansion of the universe. The speaker likens its effect to a repulsive component of gravity, which increases with distance. The analogy of particles connected by a spring is used to describe how dark energy affects atoms and other systems by adding a minute repulsive force that grows with distance.

The speaker continues to explain that the effect of dark energy on atoms is negligible, only slightly altering their equilibrium positions. The discussion then shifts to the 'Big Rip' theory, which posits that dark energy could grow over time, eventually overcoming the forces that hold galaxies, solar systems, and even atoms together, leading to their disintegration. The speaker criticizes this theory, suggesting that it violates fundamental principles of physics and is largely dismissed by serious theorists.

The speaker quantifies the dark energy density as extremely small, comparable to the energy contained in a thousand protons per cubic meter. This small amount has negligible gravitational effects on a local scale but becomes significant over cosmological distances. The idea of harnessing vacuum energy for practical use is dismissed due to its impracticality. The speaker emphasizes that dark energy only affects the universe on a large scale and has no significant impact on laboratory or smaller astronomical systems.

The discussion transitions to Newtonian gravity and the use of mathematical formalism to describe physical phenomena. The speaker introduces the del operator as a vector of partial derivatives and explains its application in creating vectors from scalars and calculating divergences. This formalism is crucial for understanding concepts like gravitational fields and the equations governing them.

The speaker elaborates on the concepts of scalar and vector fields, defining them as functions of position. Scalar fields, like potential fields, depend on position, while vector fields, such as velocity fields, have directional components. The importance of these fields in physics, particularly in understanding gravitational fields, is emphasized.

The gravitational field is introduced as a vector field representing the acceleration experienced by a test mass due to surrounding masses. The speaker explains the derivation of the gravitational field using the concept of mass density and Gauss's law, which relates the divergence of the gravitational field to the mass density. This law provides a foundational understanding of gravitational interactions.

The relationship between mass density and the gravitational field is further explored. The speaker introduces the concept of mass density as mass per unit volume and explains how it varies from point to point. Gauss's law is used to relate the gravitational field to the mass density, highlighting the role of Newton's constant in this relationship.

Gauss's theorem is introduced, stating that the integral of the divergence of a vector field over a volume is equal to the integral of the field's perpendicular component over the surface bounding the volume. This theorem is applied to gravitational fields, demonstrating its utility in simplifying complex gravitational calculations.

The speaker discusses the gravitational field of spherically symmetric mass distributions, using the example of a planet. By applying Gauss's law and considering a spherical shell around the mass, the speaker derives the well-known inverse-square law of gravitation. This example illustrates how Gauss's law simplifies the understanding of gravitational fields around spherical objects.

The gravitational field inside a spherical mass distribution, such as the Earth, is analyzed. Assuming uniform mass density, the speaker derives the linear relationship between the gravitational field and the distance from the center of the Earth. This leads to the conclusion that within the Earth, the gravitational field behaves like a harmonic oscillator, causing objects to oscillate if dropped through a tunnel drilled through the Earth.

The discussion revisits the concept that only the mass inside a spherical shell contributes to the gravitational field within the shell. Newton's proof and Gauss's law are used to explain why the gravitational field inside a shell is zero and how the field outside the shell is equivalent to that of a point mass at the center. This reinforces the utility of Gauss's law in gravitational calculations.

The equivalence principle, which states that acceleration and gravity are indistinguishable, is introduced using the analogy of an accelerating elevator. The speaker explains that the effects of gravity and acceleration are equivalent for an observer in an accelerating frame. This principle forms the basis for understanding gravitational fields in the context of general relativity.

The implications of the equivalence principle are explored through various scenarios. The principle is used to explain why gravity and acceleration produce identical physical effects, such as the bending of light in a gravitational field. The discussion emphasizes the importance of this principle in Einstein's development of general relativity.

The speaker introduces coordinate transformations for accelerated frames of reference, explaining how these transformations describe the relationship between stationary and moving frames. The transformation equations for uniform velocity and uniform acceleration are derived, highlighting their role in understanding the motion of objects in different reference frames.

The relationship between uniform acceleration and curved coordinates is discussed. The speaker explains how an accelerated reference frame can be described using curved coordinates, illustrating this with the example of a uniformly accelerating elevator. The connection between acceleration, curved coordinates, and gravity is emphasized.

The speaker discusses the bending of light in gravitational fields, a key prediction of general relativity. By considering the effect of an accelerating elevator on a light beam, the speaker demonstrates that light bends in a gravitational field. This concept is extended to the deflection of light by the sun, which was confirmed by observations during a solar eclipse.

An estimation of the deflection of light by the sun is presented. Using the acceleration due to the sun's gravity and the time it takes for light to cross the sun's radius, the speaker calculates the angle of deflection. This estimation, while crude, aligns with the predictions of general relativity and serves as a precursor to more precise calculations.

The limitations of the equivalence principle are discussed, particularly its applicability only to uniform gravitational fields and over small distances and times. The speaker explains that tidal forces, caused by the variation of the gravitational field with distance, prevent the complete elimination of gravity through accelerated reference frames.

Tidal forces, resulting from the non-uniformity of the gravitational field, are described as the primary obstruction to replacing gravity with acceleration. The speaker explains how these forces cause stretching and squeezing effects, which can be felt by objects in free fall. This concept is crucial for understanding the limitations of the equivalence principle.

The speaker plans to review the special theory of relativity but instead focuses on the geometry of space and time. The importance of understanding curvature and flatness in geometry is emphasized, with examples of flat and curved surfaces such as planes, cylinders, and spheres. The concept of the metric, which describes distances in different coordinate systems, is introduced.

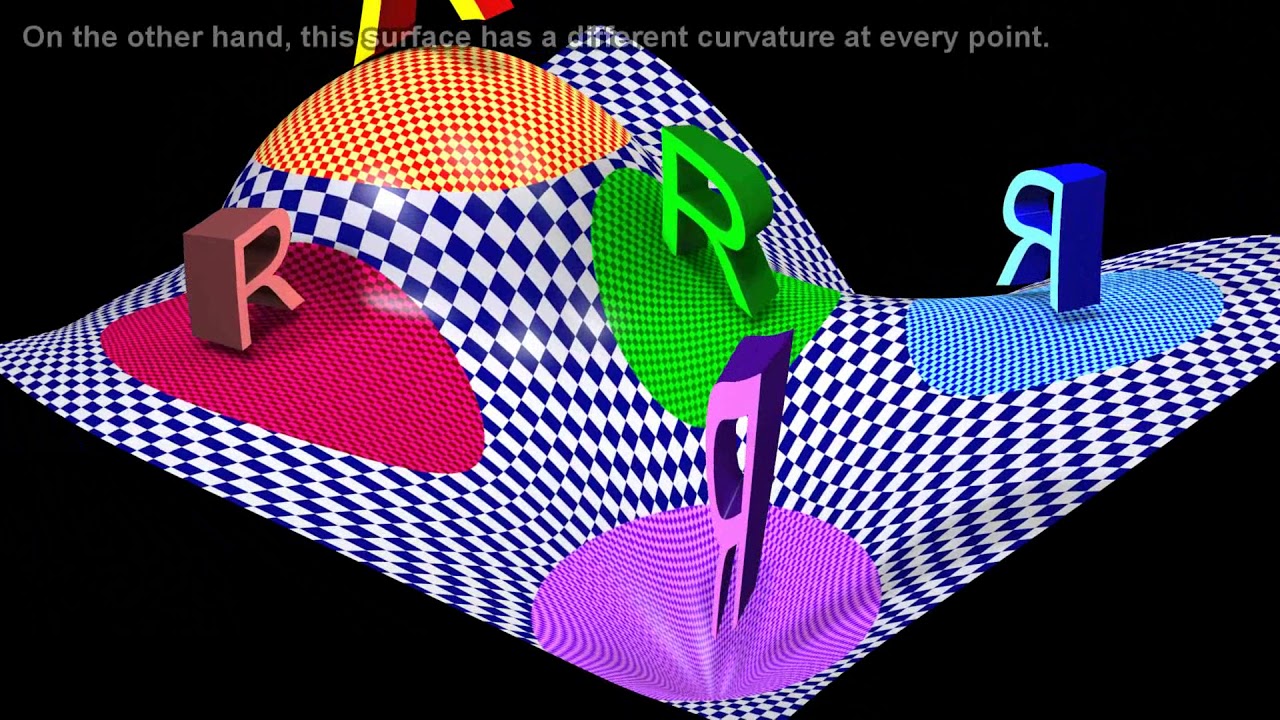
The discussion continues with examples of flat and curved geometries. The speaker explains how different surfaces, like spheres and saddles, cannot be flattened out without distortion. The concept of curvature as an obstruction to flatness is introduced, setting the stage for understanding the relationship between curvature and gravitational fields in general relativity.