Torque and a Balance Beam | Physics with Professor Matt Anderson | M12-14

Physics with Professor Matt Anderson
1 Nov 202105:16
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational transcript, Professor Anderson introduces a problem involving a beam suspended by two wires, with a mass at one end. The beam is in static equilibrium, and the forces and torques acting on it are analyzed. The forces in the y-direction are considered, including the tensions in the wires (T1 and T2) and the gravitational force (mg) on the mass. It is assumed that the beam is massless, but the center of mass of the beam would be considered if it had mass. The torques are analyzed around the left end of the beam, with T1 having a zero lever arm, T2 having a positive value with a lever arm of x2, and mg having a negative value with a lever arm of x_sub_m. The problem is solved using two equations for the two unknown tensions, leading to expressions for T2 and T1 in terms of x_sub_m and x2. The limits of these expressions are also discussed, such as when the mass is directly under the T2 wire, resulting in T1 being zero. The summary concludes by inviting students to ask questions and seek clarification, emphasizing the importance of understanding the concepts.

Takeaways
  • ๐Ÿ” The problem involves a beam suspended by two wires with a mass at the end, creating tensions in the wires.
  • ๐Ÿ“ The beam is in static equilibrium, meaning the sum of forces and torques around a chosen axis must be zero.
  • ๐Ÿ“ Tensions in the wires are labeled as tension one (T1) and tension two (T2), with positions x2 and x=0, respectively.
  • ๐Ÿ“ The mass's position on the beam is denoted as x_sub_m.
  • โš–๏ธ There are no forces in the x-direction, but there are forces in the y-direction, including T2, T1, and the weight of the mass mg.
  • โš–๏ธ Assuming the beam is massless simplifies the problem, but if the beam has mass, the force at the center of mass must be considered.
  • ๐Ÿ”— The torques are analyzed around the left end of the beam, with T1 having a lever arm of zero, T2 having a positive torque with a lever arm of x2, and mg having a negative torque with a lever arm of x_sub_m.
  • ๐Ÿงฎ Two equations are formed from the force and torque balances, allowing for the solution of the unknown tensions T1 and T2.
  • ๐Ÿ“‰ Solving for T2 gives the formula T2 = (mg * x_sub_m) / x_sub_2.
  • ๐Ÿ” Solving for T1 using the first equation results in T1 = mg * (x_sub_m / x_sub_2) - 1.
  • ๐Ÿ A special case occurs when x_sub_m equals x2, which would result in T1 being zero, indicating the mass is directly below the attachment point of T2.
  • โ“ Professor Anderson encourages students to approach him with any questions, emphasizing the importance of understanding the concepts.
Q & A

  • What is the primary focus of the problem discussed by Professor Anderson?

    -The primary focus of the problem is to analyze the equilibrium of forces and torques acting on a beam suspended by two wires with a mass at the end.

  • What are the two tensions acting on the beam?

    -The two tensions acting on the beam are labeled as tension one (T1) and tension two (T2).

  • What is the role of tension one (T1) in the system?

    -Tension one (T1) acts downward, trying to hold the beam in position.

  • What is the role of tension two (T2) in the system?

    -Tension two (T2) acts upward, carrying the weight of the mass at the end of the beam.

  • What is the force acting downward with a force mg?

    -The force acting downward with a force mg is the weight of the mass (m) at the end of the beam due to gravity (g).

  • What is the assumption made about the beam in the problem?

    -The assumption made is that the beam is massless, which simplifies the analysis by not considering the weight of the beam itself.

  • How does the presence of mass on the beam affect the analysis?

    -If there is mass on the beam, it would need to be accounted for by adding the force acting at the center of mass of the beam to the analysis.

  • What is the condition for static equilibrium in the y-direction?

    -For static equilibrium in the y-direction, the sum of all forces (T2 upward, T1 downward, and mg downward) must equal zero.

  • What is the axis of rotation chosen for analyzing torques?

    -The left end of the beam is chosen as the axis of rotation for analyzing torques.

  • How does the position of the mass (x_m) affect the torque?

    -The position of the mass (x_m) affects the torque as it determines the lever arm for the force of gravity (mg) acting on the mass, which is crucial for calculating the torque due to mg.

  • What are the two equations derived from the analysis of forces and torques?

    -The two equations derived are: 1) The sum of forces in the y-direction equals zero, and 2) The sum of torques around the chosen axis equals zero.

  • What happens to the tension in cable one (T1) if the mass is moved to where cable two (T2) is attached?

    -If the mass is moved to where cable two (T2) is attached (x_m = x2), then T1 would be zero because the entire weight of the mass and the beam is supported by T2.

Outlines
00:00
๐Ÿ” Analyzing Equilibrium with Beam and Tensions

Professor Anderson introduces an equilibrium problem involving a beam suspended by two wires with a mass at one end. The beam is in static equilibrium, and the forces and torques acting on it are analyzed. Tensions in the wires are labeled as T1 and T2, and positions are defined with x2 for the right end of the beam and x0 at the left end. The mass's position is denoted as x_sub_m. Forces in the y-direction are considered, including T2 upwards, T1 downwards, and the weight of the mass mg also downwards. The torques are analyzed around the left end of the beam, with T1 having a lever arm of zero, T2 having a positive torque with a lever arm of x2, and mg having a negative torque with a lever arm of x_sub_m. The problem is solved by setting up two equations based on the forces and torques and solving for the unknown tensions T1 and T2. The limits of the problem are also discussed, such as when the mass is positioned directly under the T2 wire, which would result in T1 being zero and T2 supporting the entire system.

05:03
๐Ÿ“š Applying the Problem to Different Parameters

The script encourages students to apply the problem to various parameters and to ensure they understand the concepts. Professor Anderson invites students to ask questions and offers his office hours for further clarification. The goal is to ensure a clear understanding of the equilibrium problem and the application of forces and torques in solving for tensions in a physical system.

Mindmap
Keywords
๐Ÿ’กEquilibrium
Equilibrium in physics refers to a state where all forces acting on a system are balanced, resulting in no net force. In the video, the theme revolves around finding the equilibrium state of a beam suspended by wires with a mass at the end, ensuring that the forces and torques sum to zero.
๐Ÿ’กBeam
A beam is a horizontal or sloping load-bearing element that is capable of withstanding load primarily by resisting bending. In the context of the video, the beam is the structure being suspended and analyzed for its equilibrium under the influence of forces and torques.
๐Ÿ’กTension
Tension is the force carried by a member subjected to compression or tension. In the video, tension is exerted by the wires suspending the beam and is a key factor in maintaining the beam's equilibrium. The script discusses 'tension one' and 'tension two' as forces acting on the beam.
๐Ÿ’กTorques
Torques are rotational forces that can cause an object to rotate about an axis. The video script involves analyzing torques to ensure the beam is in rotational equilibrium, with the forces of tension and the weight of the mass creating torques about the chosen axis.
๐Ÿ’กStatic Equilibrium
Static equilibrium is the condition of a body at rest, where the net force and net torque acting on it are zero. The video script focuses on calculating the conditions for static equilibrium of the beam under the influence of various forces and torques.
๐Ÿ’กLever Arm
A lever arm is the distance from the axis of rotation to the point where a force is applied. In the context of the video, the lever arms of the tensions and the mass's weight are critical in calculating the torques acting on the beam.
๐Ÿ’กT1 and T2
T1 and T2 are labels for the two tensions in the wires suspending the beam. The script uses these labels to represent the unknown tensions that need to be solved for in order to understand the beam's equilibrium state.
๐Ÿ’กMass (mg)
Mass (represented as 'mg' in the script, where 'm' is the mass and 'g' is the acceleration due to gravity) is the force due to gravity acting on the mass at the end of the beam. It is a downward force that contributes to the torque and overall equilibrium of the system.
๐Ÿ’กPositions (x2, x=0, xm)
Positions x2, x=0, and xm are used in the script to denote specific points along the beam for the application of forces and torques. These positions help in calculating the lever arms and are crucial for the mathematical analysis of the beam's equilibrium.
๐Ÿ’กAxis of Rotation
The axis of rotation is the point or line around which an object rotates. In the video, the left end of the beam is chosen as the axis of rotation for analyzing the torques acting on the beam.
๐Ÿ’กLimits
Limits in this context refer to the boundary conditions or extreme cases that can be considered to understand the behavior of the system under study. The script discusses a limit where the mass is moved to the position where the second cable is attached, which simplifies the system for analysis.
Highlights

Professor Anderson introduces the concept of equilibrium in a problem involving forces and torques.

A beam is suspended from a wire with a mass at the end, creating a system with tensions in the wires.

The beam is supported by two wires, one at each end, with the left end anchored to the floor.

Tensions are labeled as T1 and T2, with positions x2 and x=0, and the mass position as x_sub_m.

Analysis of forces in the y-direction shows T2 acting upwards, T1 downwards, and mg (mass times gravity) also downwards.

The beam is assumed to be massless, which simplifies the equilibrium analysis.

Static equilibrium requires that the sum of forces equals zero.

Torque analysis is performed around the left end of the beam as the axis of rotation.

T1 has a lever arm of zero, T2 has a positive torque with a lever arm of x2, and mg has a negative torque with a lever arm of x_sub_m.

Two equations are derived from force and torque balance, with two unknowns to solve for T1 and T2.

T2 is calculated as mg * x_sub_m / x_sub_2, providing the tension in cable two.

T1 is determined by the equation T1 = T2 - mg, which includes the mass and positions of the system.

The limits of the system are explored, such as when the mass position equals the position of T2, resulting in T1 being zero.

The condition where T1 is zero indicates a perfectly balanced system with all the weight supported by T2.

The problem encourages students to try different parameters and verify the logic behind the equilibrium calculations.

Professor Anderson invites students to discuss the problem further or ask questions in their office.

The transcript concludes with a cheerful sign-off, encouraging engagement and clarification.

Transcripts

Browse More Related Video

Torque and Tipping Point - Man on Beam | Physics with Professor Matt Anderson | M12-21

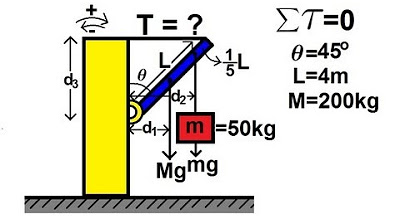
Physics 15 Torque Example 2 (2 of 7) Mass on Rod and Cable

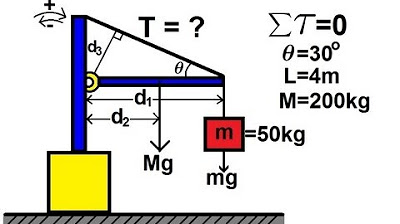
Physics 15 Torque Example 1 (1 of 7) Mass on Rod and Cable

Static equilibrium (Examples)

How to solve Rotational Equilibrium Problems

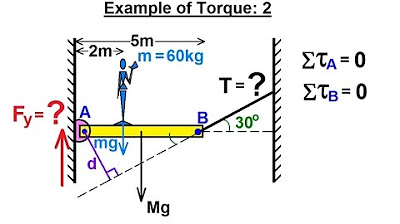
Physics 15 Torque (12 of 27) Example 2: Forces=? Tension=?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Static EquilibriumForces AnalysisTorquesBeam MechanicsEngineeringPhysicsTensionMass BalanceEducationalProblem SolvingMechanical Engineering