Physics 15 Torque (13 of 27) Body Mechanics: Ex. 1, F=? To Lift Up Arm

Michel van Biezen
9 Jan 201605:27
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into the fascinating world of biomechanics, specifically the strength required by the human triceps to lift the arm horizontally. It explains that the triceps, connected to the humerus, must exert a significant force to overcome the weight of the arm and the leverage involved. Using physics, the script calculates the necessary force by considering the arm's weight (50 Newtons), the distance from the shoulder joint to the muscle's attachment (8 cm), and the angle of the muscle (12°). The calculation reveals that the triceps must apply approximately 200 pounds of force to lift and hold the arm in place, highlighting the extraordinary strength of human muscles for everyday movements.

Takeaways
  • 💪 The triceps muscle is responsible for lifting the arm into a horizontal position.
  • 🏋️‍♂️ The weight of the arm is considered to be 50 Newtons for the purpose of this example.
  • ⚖️ The center of mass for the arm is approximately 30 cm away from the shoulder joint.
  • 🔗 The triceps is connected to the bone about 8 cm away from the shoulder pivot point.
  • 📐 The angle made by the triceps muscle relative to the horizontal is about 12° when the arm is outstretched.
  • 🔄 The sum of all torques about the pivot point must equal zero according to physics principles.
  • 📐 The force exerted by the triceps is calculated using the torque equation, considering the perpendicular distance from the force's line of action to the pivot point.
  • 🧮 The calculated force required for the triceps to lift and hold the arm in a horizontal position is approximately 92.99 Newtons.
  • 🔀 Converting Newtons to pounds, the triceps must apply a force of about 200 to 203 pounds.
  • 🤔 The human body's muscles are incredibly strong, capable of exerting large forces even for lifting relatively small weights like the arm's own weight.
  • 📚 Understanding human body mechanics is crucial for appreciating the complex interplay between muscle strength, skeletal structure, and the physics of movement.
Q & A

  • What is the primary focus of the discussion in the transcript?

    -The primary focus of the discussion is the study of human body mechanics, specifically how much tension the triceps muscle needs to apply to lift the arm into a horizontal position.

  • What is the weight of the arm considered in the example?

    -The weight of the arm is considered to be 50 Newtons in the example provided.

  • What is the distance from the center of mass of the arm to the shoulder joint in the example?

    -The distance from the center of mass of the arm to the shoulder joint is about 30 cm.

  • How far from the shoulder joint is the triceps muscle connected to the bone?

    -The triceps muscle is connected to the bone about 8 cm away from where the arm pivots at the shoulder joint.

  • What is the angle made by the triceps muscle relative to the horizontal when the arm is outstretched?

    -The angle made by the triceps muscle relative to the horizontal when the arm is outstretched is about 12°.

  • What is the formula used to calculate the force required by the triceps muscle?

    -The formula used is based on the sum of torques about the pivot point being equal to zero, which includes the negative torque from the arm's weight and the positive torque from the triceps muscle's force multiplied by the perpendicular distance to the pivot point.

  • What is the force that the triceps muscle needs to apply to hold the arm in a horizontal position?

    -The triceps muscle needs to apply a force of approximately 92.99 Newtons to hold the arm in a horizontal position.

  • How is the force in Newtons converted to pounds?

    -The force in Newtons is converted to pounds by dividing by 4448, with the result being approximately 202 to 203 pounds.

  • Why are the muscles in the human body considered extremely strong?

    -The muscles in the human body are considered extremely strong because they can generate a large amount of force over short distances, which is necessary to move the body's skeletal structure effectively.

  • What is the significance of the pivot point in the context of the triceps muscle and arm movement?

    -The pivot point is significant because it is the point around which the torques created by the muscle forces and the weight of the arm are calculated. It is the reference point for the leverage that the triceps muscle uses to lift the arm.

  • What does the human body's ability to lift its own weight, such as the weight of an arm, reveal about its muscular strength?

    -The ability to lift the weight of an arm, which may not seem heavy, actually requires a significant amount of force, indicating that the human body's muscles are much stronger than one might initially perceive.

  • Why is it important to understand the physics behind muscle strength and body mechanics?

    -Understanding the physics behind muscle strength and body mechanics is important because it provides insights into how the human body functions, how much force muscles can generate, and the efficiency of the skeletal and muscular systems in movement.

Outlines
00:00
💪 Mechanics of Human Body and Tricep Strength

This paragraph delves into the physics of human body mechanics, specifically focusing on the strength required by the tricep muscle to lift an arm horizontally. It begins by posing a question about the force needed for this action and then explains the role of the tricep as the muscle attached to the humerus bone. The paragraph uses a hypothetical scenario where the arm weighs 50 Newtons and calculates the force exerted by the tricep using the torque equation. It factors in the arm's weight, the distance from the shoulder joint to the muscle's attachment point, and the angle of the muscle relative to the horizontal. The calculation results in the tricep needing to exert a force of approximately 200 pounds to lift and hold the arm in the horizontal position. This illustrates the remarkable strength of human muscles and the intricate design of the skeletal structure.

05:01
🏋️‍♂️ Human Muscles' Surprising Strength

The second paragraph emphasizes the surprising strength of human muscles, even when lifting seemingly light weights like the weight of one's own arm. It reiterates the significant force—200 pounds—that the tricep muscle must exert, as calculated in the previous paragraph. The paragraph highlights the body's ability to generate such force through its muscular structure and the efficient design of muscle attachment points relative to the center of mass of the weights being lifted. It concludes by marveling at the strength required for these actions and the overall amazing design of the human body.

Mindmap
Keywords
💡Mechanics
Mechanics is a branch of physics that deals with the behavior of physical bodies when subjected to forces or displacements. In the context of the video, it is used to describe the forces and movements involved in human body mechanics, particularly focusing on the muscular and skeletal system.
💡Torque
Torque is the measure of the force that can cause an object to rotate about an axis. It is a vector quantity that represents the rotational equivalent of linear force. In the video, torque is central to understanding how the triceps muscle generates enough force to lift the arm against gravity.
💡Triceps
The triceps, or more specifically the triceps brachii, is a large muscle at the back of the upper arm in humans and other animals. It is responsible for extending the forearm and is a key muscle in the discussion of the video, as it is the muscle that lifts the arm into a horizontal position.
💡Body Mechanics
Body mechanics refers to the study of the body's movement and the forces acting upon it, especially in relation to physical work and posture. The video uses this concept to explain the physics behind the human arm's movement, emphasizing the strength required by the triceps to perform a simple action like lifting the arm.
💡Skeletal System
The skeletal system is the internal framework of bones in the body, providing structure, support, and protection for organs. In the video, the skeletal system is mentioned in relation to how the triceps muscle is attached to the humerus bone, which is critical for the arm's movement.
💡Muscle Strength
Muscle strength is the amount of force a muscle can produce. The video calculates the force the triceps must exert to lift the arm, highlighting the surprising strength of human muscles for everyday movements.
💡Newtons
Newtons are the unit of force in the International System of Units (SI). In the video, the weight of the arm is given in Newtons to quantify the force required for the triceps to lift and hold the arm in a horizontal position.
💡Center of Mass
The center of mass is the point at which the mass of an object is concentrated for the purpose of calculating gravitational force. In the context of the video, the center of mass of the arm is used to calculate the torque needed for the arm to be lifted.
💡Pivot Point
A pivot point is a fixed point around which a body rotates or oscillates. In the video, the pivot point is the shoulder joint, which is the point around which the arm rotates when lifted by the triceps.
💡Physics
Physics is the natural science that involves the study of matter and its motion through space and time, along with related concepts like energy and force. The video uses physics to explain the biomechanics of lifting the human arm, demonstrating the principles of force and torque.
💡Human Body
The human body is the entire structure of a human organism, which in the video is the subject of study for understanding how muscles work in conjunction with the skeletal system to perform physical actions. The video emphasizes the complexity and strength of the human body's muscular system.
Highlights

Study of human body mechanics involves understanding how muscles like the tricep apply tension to perform actions, such as lifting an arm horizontally.

The tricep is the only muscle capable of lifting the arm due to its attachment to the humerus in the upper arm.

The physics of lifting an arm involves calculating the force required by the tricep, considering the arm's weight and the distance from the shoulder joint.

The weight of the arm is assumed to be 50 Newtons for the purpose of this calculation.

The center of mass of the arm is about 30 cm away from the shoulder joint, which affects the torque calculation.

The tricep is connected to the bone about 8 cm away from the arm's pivot point, influencing the force it needs to exert.

The angle made by the tricep relative to the horizontal is approximately 12° when the arm is outstretched.

The sum of all torques about the pivot point must equal zero according to the principle of moments.

The negative torque is calculated as the weight times the perpendicular distance from the force's line of action to the pivot point.

The positive torque is the force exerted by the tricep, multiplied by the distance from its line of action to the pivot point.

To find the force exerted by the tricep, the perpendicular distance (D) from the force's line of action to the pivot point is calculated using trigonometry.

The force required by the tricep is calculated to be 92.99 Newtons, which is a significant amount of force for a single muscle.

Converting the force to pounds, the tricep must apply approximately 200 to 203 pounds of force to lift and hold the arm in a horizontal position.

The human body's skeletal structure and muscle movement are intricate and require a precise balance of force and leverage.

Despite lifting relatively small weights, such as the weight of an arm, the body's muscles exert an enormous amount of force.

The strength of human muscles is often surprising, as even small movements require significant force.

The tricep's ability to exert such force for a small muscle group illustrates the remarkable strength of the human body.

The study of human mechanics is not only about understanding the body's capabilities but also appreciating the complexity of its design.

Transcripts

Browse More Related Video

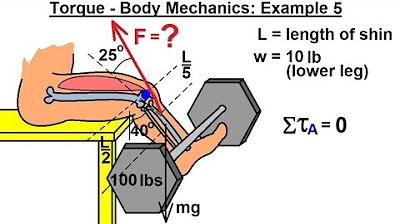
Physics 15 Torque (17 of 25) Body Mechanics: Ex. 5, F=? Leg Lifting Weights

Physics 15 Torque (14 of 27) Body Mechanics: Ex. 2, F=? To Lift Dumbbell

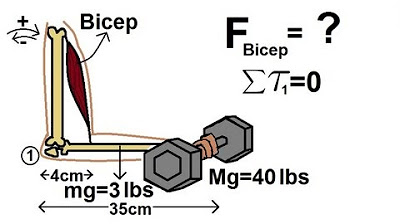
Physics 15 Torque Example 5 (5 of 7) The Bicep

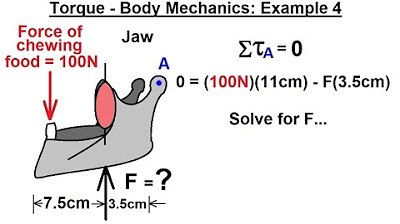
Physics 15 Torque (16 of 25) Body Mechanics: Ex. 4, F=? Jaw Muscle

Physics 15 Torque (20 of 25) More Examples: 2 F=? of Screw on Bracket

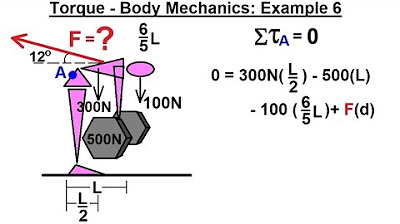
Physics 15 Torque (18 of 25) Body Mechanics: Ex. 6, F=? on Back Muscle

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Human MechanicsMuscle StrengthPhysicsTricep ForceBiomechanicsBody MechanicsMuscular PowerHealth ScienceHuman BodyStrength Analysis