2 Masses on a Pulley - Torque Demonstration
TLDRIn the video script, a group of students and their teacher, Mr. P, engage in a detailed physics problem-solving session. They tackle a problem involving a pulley system with two masses and explore the concept of angular acceleration. The discussion covers the rotational inertia of the pulley, the forces of tension in the strings, and the free body diagrams for both masses and the pulley itself. The students clarify misconceptions about the direction of forces and the impact of the pulley's mass on the tension forces. They use torque and force equations to solve for the pulley's angular acceleration and the tension forces, finding that the forces are not equal due to the pulley's mass and rotational inertia. The teacher, Mr. P, emphasizes the importance of considering the pulley's friction and the correct approach to summing torques and forces. The session concludes with a comparison of their calculated angular acceleration with a measured value, resulting in a small percentage difference, and a caution against common incorrect methods of solving such problems.
Takeaways
- 📐 The problem involves a pulley system with two masses and the pulley has a specific rotational inertia and radius.
- 🔵 Mass 1 is 0.100 kg and Mass 2 is 0.200 kg, hanging from a pulley with a rotational inertia of 0.0137 kg·m² and a radius of 0.0385 m.
- ⚙️ The pulley's rotational inertia includes the spokes, which were considered in a previous discussion.
- 🔄 The angular acceleration of the pulley and the tension in each string are unknowns to be solved for.
- 🧷 Tension forces on either side of the pulley are not the same due to the pulley's mass and rotational inertia.
- 📈 The net torque on the pulley is calculated by the difference in torques caused by the two tension forces.
- 🌀 The direction of torque is defined as clockwise or into the board, which is considered positive.
- 📉 The forces of gravity and normal do not contribute to the torque on the pulley as they act on the axis of rotation.
- ↗️ The linear acceleration of the hanging masses is equivalent to the tangential acceleration of the pulley's rim.
- 🔢 The angular acceleration of the pulley is calculated using the formula involving mass, gravitational acceleration, and pulley dimensions.
- 🔴 The measured angular acceleration is compared with the predicted value, showing a 9.03% difference.
- ⚖️ The predicted forces of tension in the strings are calculated, showing that they are not equal due to the pulley's rotational inertia.
Q & A
What is the mass of the two objects hanging from the pulley?
-The mass of the first object (Mass 1) is 0.100 kilograms, and the mass of the second object (Mass 2) is 0.200 kilograms.
What are the physical characteristics of the pulley mentioned in the script?
-The pulley has a rotational inertia of 0.0137 kilograms times meters squared and a radius of 0.0385 meters.
Why are the two forces of tension on either side of the pulley not the same?
-The two forces of tension are not the same because the pulley has mass and rotational inertia, which requires a net torque to angularly accelerate the pulley.
What is the definition of positive direction as defined by Billy?
-Positive direction is defined as clockwise or into the board. To the left of the pulley, up is positive, and to the right of the pulley, down is positive.
How is the linear acceleration of the hanging mass related to the angular acceleration of the pulley?
-The linear acceleration of the hanging mass is the same as the tangential acceleration of the rim of the pulley, which can be expressed as acceleration = radius times angular acceleration.
What is the calculated angular acceleration of the pulley?
-The calculated angular acceleration of the pulley is 2.67016 or 2.67 radians per second squared.
What is the percentage difference between the measured and predicted angular acceleration?
-The percentage difference is negative 9.03 percent, indicating that the measured angular acceleration was about 9 percent less than the predicted angular acceleration.
What are the predicted forces of tension in each string?
-The predicted force of tension in the first string is 0.991 N, and in the second string, it is 1.94 N.
Why do students sometimes incorrectly sum the torques on the whole system with the axis of rotation at the axle of the pulley?
-This approach is incorrect because the rotational inertia and angular acceleration on the right-hand side of the equation would then refer to the entire system, including the two hanging masses, which do not have rotational inertia nor angular acceleration.
Why is the assumption of the pulley having negligible friction important?
-The assumption of negligible friction refers to the axle of the pulley, allowing for the interaction between the pulley surface and the string without hindering the rotation of the pulley due to the mass's weight.
What is the common mistake students make when summing forces on the entire system?
-A common mistake is summing forces in the positive direction for the entire system, which does not work because the mass times acceleration on the right-hand side of the equation would be for the entire system, not just the pulley.
Why can't the pulley have a single tangential acceleration?
-The pulley cannot have a single tangential acceleration because the tangential acceleration depends on the radius; the larger the radius, the larger the tangential acceleration at that specific point on the pulley.
Outlines
🔍 Pulley System Analysis and Angular Acceleration
The first paragraph introduces a physics problem involving a frictionless pulley with two masses hanging from either side. The problem requires calculating the angular acceleration of the pulley and the tension in each string. The participants, Bobby, Bo, and Billy, discuss the pulley's rotational inertia and how it affects the tension forces. They also clarify that the tension forces on either side of the pulley are not the same due to the pulley's mass and rotational inertia. The discussion leads to drawing free body diagrams and summing torques around the pulley's axle to find the angular acceleration. The process involves understanding the direction of positive torque and the forces acting on the masses and the pulley.
📐 Correcting Directional Errors and Calculating Tensions
In the second paragraph, the focus shifts to correcting a mistake regarding the direction of forces and torques. The group realizes that the direction of positive torque was defined as clockwise, which affects how forces are summed. They then proceed to calculate the forces of tension in the strings using the equations developed from summing forces on the masses and the torque equation. The paragraph emphasizes the importance of direction in physics problems and provides a detailed method for combining equations to solve for the unknowns. The group also calculates the angular acceleration using the accepted values and compares it with a measured value to find the percentage difference, concluding that their prediction was reasonably close.
🧮 Solving for Tensions and Common Pitfalls in Pulley Problems
The third paragraph deals with solving for the predicted forces of tension in the strings. Billy uses the previously derived equations, substituting the pulley radius times angular acceleration for linear acceleration. The paragraph highlights the fact that the two forces of tension are not equal due to the pulley's mass and rotational inertia. Mr. P clarifies misconceptions about the pulley's friction and explains the difference between the axle's negligible friction and the pulley's surface friction. The paragraph also points out common incorrect approaches students take when solving pulley problems, such as summing torques or forces on the entire system. The correct method involves focusing on the pulley's rotation and the individual forces acting on it. The group concludes by appreciating the learning experience.
Mindmap
Keywords
💡Frictionless Pulley
💡Rotational Inertia
💡Radius
💡Angular Acceleration
💡Tension
💡Free Body Diagram
💡Torque
💡Linear Acceleration
💡Relative Error
💡Significant Digits
💡Negligible Friction
Highlights
The problem involves a pulley system with two masses of 0.100 kg and 0.200 kg, and a pulley with specific rotational inertia and radius.
The pulley is frictionless with a given rotational inertia of 0.0137 kg*m² and a radius of 0.0385 meters.
The challenge is to find the angular acceleration of the pulley and the force of tension in each string.
Billy initiates the problem-solving by drawing free body diagrams for the pulley and the masses.
Bo questions the inclusion of the rotational inertia of the pulley's spokes, which were previously accounted for.
Mr. P clarifies that the two tension forces on either side of the pulley cannot be the same due to the pulley's mass and rotational inertia.
Billy sums the torques on the pulley, considering the direction of positive torque as clockwise.
The net torque on the pulley is equated to the product of the pulley's rotational inertia and its angular acceleration.
Bobby sums the forces on mass 1 and mass 2, creating equations for the force of tension 1 and 2.
Billy corrects the direction of forces, emphasizing positive torque on the side where mass 2 is located.
Mr. P emphasizes the importance of defining a positive direction for force summation.
Bo combines the equations for force of tension 1 and 2 with the torque equation to solve for the unknowns.
Linear accelerations are related to angular acceleration through the radius of the pulley.
Bo calculates the angular acceleration of the pulley to be 2.67 radians per second squared.
The measured angular acceleration is found to be approximately 9% less than the predicted value.
Billy solves for the predicted forces of tension, yielding non-equal magnitudes due to the pulley's rotational inertia.
Mr. P discusses the misconceptions regarding the pulley's friction and the incorrect methods students may use to solve the problem.
The session concludes with a summary of the correct approach and a reminder of the importance of considering the pulley's mass and friction.
Transcripts
Browse More Related Video
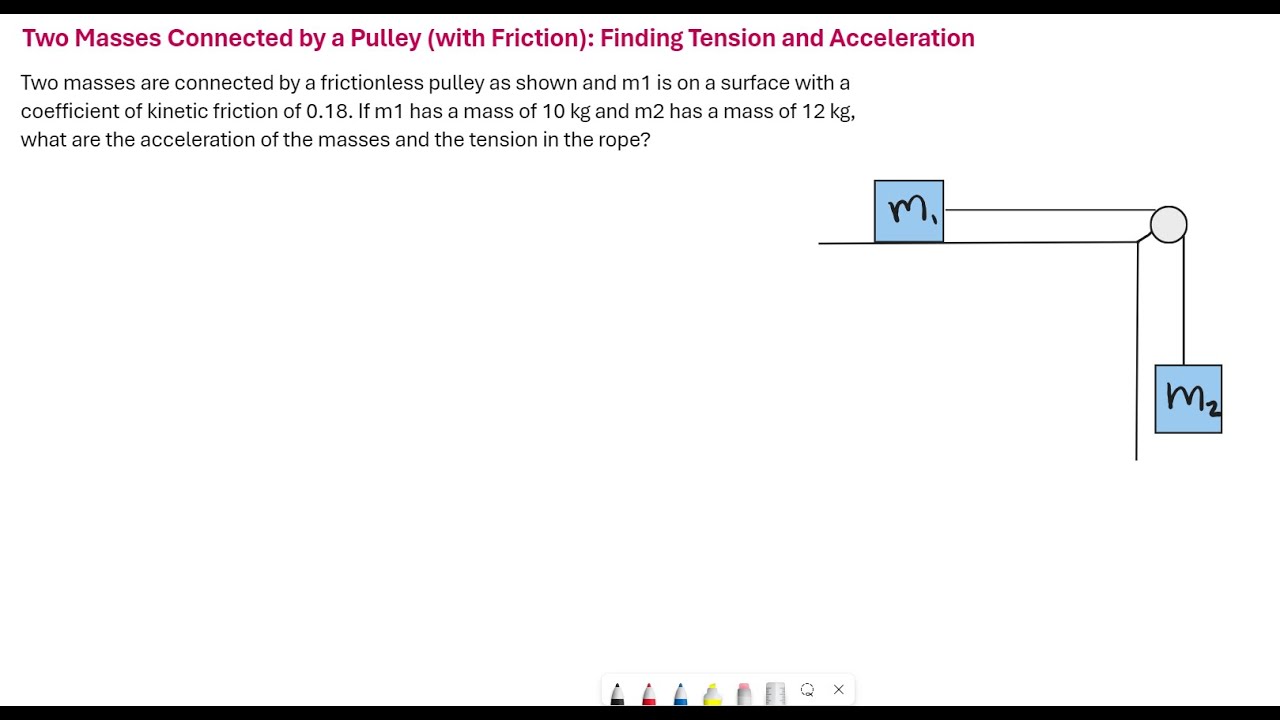
Finding the Tension and Acceleration for a Two Mass Pulley (with Friction)
AP Physics Workbook 7.M Massive Pulley
Demonstrating Rotational Inertia (or Moment of Inertia)

College Physics 1: Lecture 25 - Moment of Inertia and Rotational Dynamics
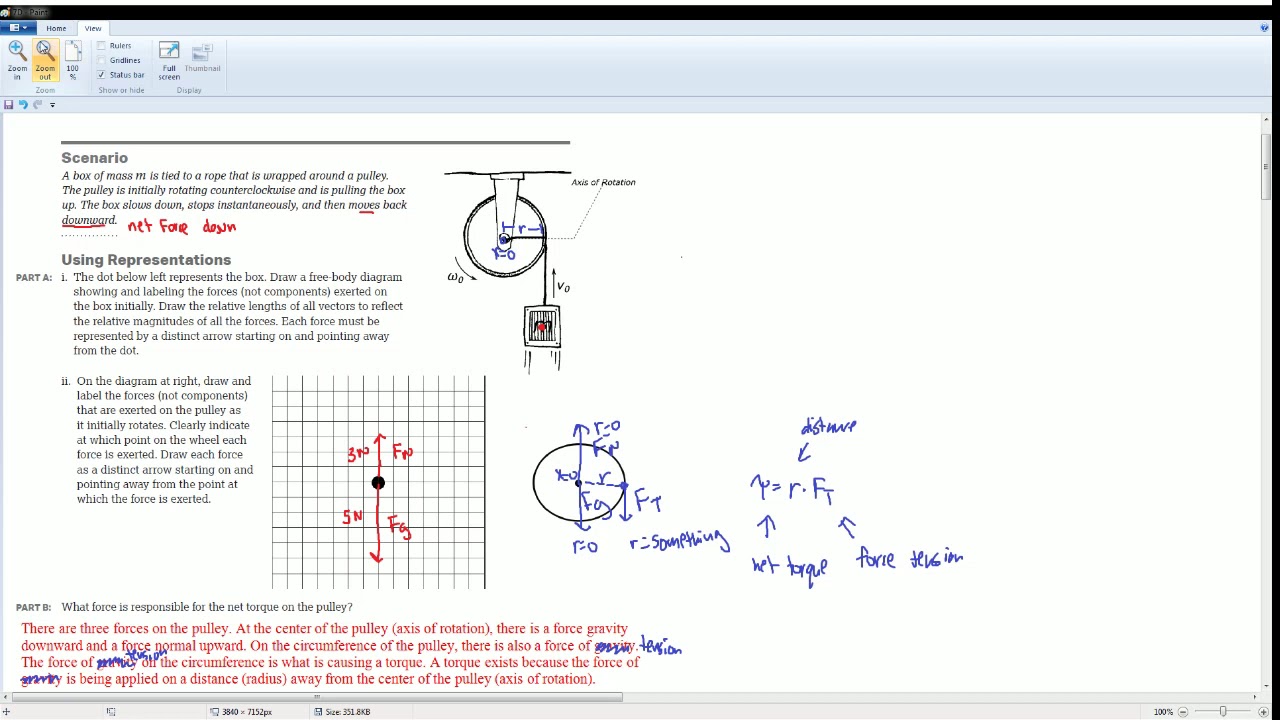
AP Physics Workbook 7.D Forces vs Torques

2018 #1 Free Response Question - AP Physics 1 - Exam Solution
5.0 / 5 (0 votes)
Thanks for rating: