2 | FRQ (Calculator Active) | Practice Sessions | AP Calculus AB
TLDRThe video script is a detailed AP Calculus tutorial where Mark Kiraly and Virge Cornelius discuss a free response question involving calculus concepts and the use of a calculator. They begin by setting up the problem, which involves an online bookseller's order and shipping rates over time, and an initial backlog of orders. The session covers finding the derivative of the order rate function, calculating the average shipping rate, and determining the total number of orders waiting to be shipped at a specific time without evaluating the integral. The instructors also explore whether the number of orders waiting to ship is increasing or decreasing at a particular time by analyzing the rates. Throughout the video, they emphasize the importance of using a calculator effectively and encourage students to keep working through problems by applying what they know about differentiation and integration.
Takeaways
- 📚 **Use Calculators Effectively**: Remember to utilize your calculator for derivatives and integrals when allowed, as it can save time and effort.
- ⏱️ **Practice with the Calculator**: Familiarize yourself with your calculator and its functions to ensure you can quickly and accurately perform necessary calculations during an exam.
- 💡 **Store Functions**: In multi-part free response questions, storing functions in your calculator can be helpful for quick reference and to avoid retyping.
- 🔢 **Accuracy and Precision**: When providing answers, ensure they are accurate to three decimal places as required by the AP exam, but also be prepared to provide more if necessary.
- 📈 **Interpret Units Correctly**: Understand the units of the functions and their derivatives to interpret the results correctly in the context of the problem.
- 📉 **Increasing and Decreasing Functions**: Determine if a quantity is increasing or decreasing by analyzing the sign of the derivative or the difference between input and output rates.
- 📝 **Write Clearly**: When interpreting mathematical results in a word problem, write clearly and include the units to convey the correct meaning.
- 🔑 **Start with What You Know**: If unsure where to begin, start by differentiating or integrating what you know from the problem statement.
- 📌 **Use Function Notation**: Utilize function notation on your calculator to easily manipulate and reference stored functions.
- 📐 **Understand the Problem**: Before jumping into calculations, make sure you understand what the problem is asking for, whether it's a rate, an integral, or a derivative.
- 📈 **Average Rate**: Recognize that integrating a rate function over an interval gives you the total quantity over that interval, which can be used to find averages.
- 📘 **Yellow Sheet Method**: Keep in mind the yellow sheet method for organizing and solving multi-part problems, which can help in keeping track of your work.
Q & A
What is the rate at which the online bookseller receives orders, represented by B(t), in terms of t?
-The rate at which the online bookseller receives orders, B(t), is given by the square root of 480t minus 40t squared, in orders per hour since t equals 0 after opening.
What is the rate at which the bookseller begins shipping orders, represented by S(t), in terms of t?
-The bookseller begins shipping orders at a rate of S(t) equals 9 plus 3 to the power of 0.035t squared orders per hour, starting at t equals 1 hour after opening.
How many orders are in the system waiting to be shipped when the bookseller opens?
-When the bookseller opens, there are 57 orders in the system waiting to be shipped.
What does B'(2) represent in the context of the problem?
-B'(2) represents the rate at which orders are received, which is increasing at a rate of 5.656 orders per hour per hour at 2 hours after opening.
What is the integral of 1/4 of the integral from 1 to 5 of S(t) dt, and what does it represent in the context of the problem?
-The integral represents the average rate of orders shipped from time t equals 1 to t equals 5 hours after opening, which is 10.545 orders per hour.
How do you write an integral expression for the total number of orders waiting to be shipped at time t equals 3 hours?
-The integral expression is the initial 57 orders plus the integral from 0 to 3 of B(t) dt minus the integral from 1 to 3 of S(t) dt.
At t equals 10 hours after the bookseller opens, are the number of orders waiting to be shipped increasing or decreasing?
-The number of orders waiting to be shipped is decreasing at t equals 10 hours after opening, as indicated by B(10) - S(10) being less than 0.
What is the significance of using the calculator in solving the given problems?
-The calculator is significant as it allows for the efficient computation of derivatives, integrals, and solving equations, which can be complex to solve manually, especially within the constraints of an exam setting.
What is the advice given for students who are unsure of where to start on a problem, particularly with word problems?
-The advice is to 'just do what you know', meaning to start taking derivatives or integrating, which keeps the problem-solving process moving forward even when the initial steps are not clear.
What is the importance of storing functions in the calculator for free response questions?
-Storing functions in the calculator is important for free response questions because it allows for quick reference and reuse of these functions throughout the problem-solving process, making it easier to manage multiple parts of a complex problem.
Why is it recommended to write down more than three decimal places when providing answers on the AP exam?
-Writing more than three decimal places is recommended because it ensures accuracy and allows for rounding or truncating to the required precision without losing relevant information.
What is the interpretation of the units for B'(t) in the context of the problem?
-The units for B'(t) are orders per hour squared, which specifically at t equals 2 hours, means the rate at which orders are received is increasing at a rate of 5.656 orders per hour per hour.
How does the concept of the average value of a rate function apply in the context of the problem?
-The concept is applied when finding the average rate of orders shipped from time t equals 1 to t equals 5 hours after opening. It represents the mean rate over the given time interval, which is useful for understanding the overall trend without focusing on specific values.
Outlines
📚 AP Calculus Practice with Mark and Virge
Mark Kiraly and Virge Cornelius introduce an AP Calculus free-response question focused on the use of a calculator. They discuss the scenario of an online bookseller's order and shipping rates, emphasizing the importance of downloading the question and preparing the calculator in advance. Mark demonstrates how to find the derivative of the order rate function B(t) at t=2 and interpret its meaning in the context of the problem.
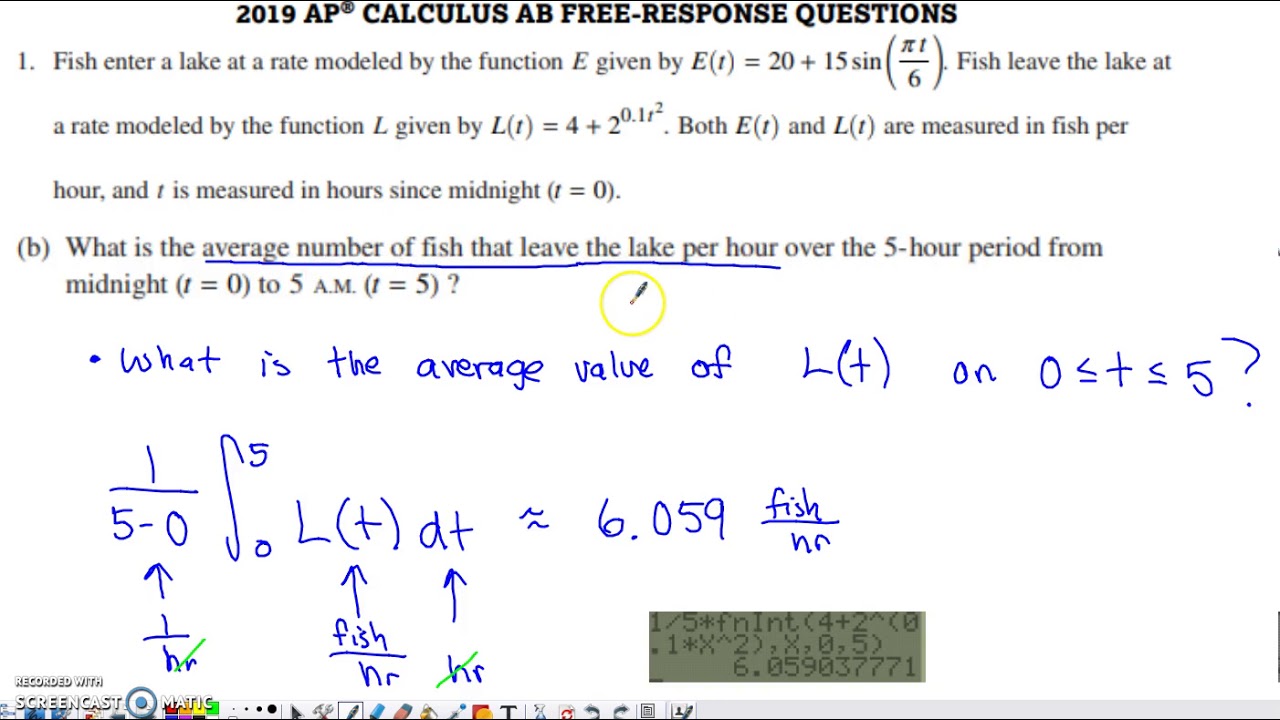
🧮 Calculating the Average Shipping Rate
The instructors proceed to part B of the problem, which involves calculating the integral of the shipping rate function S(t) from t=1 to t=5, divided by 4. Mark shows how to use the calculator to find the integral and emphasizes the importance of understanding the units and context of the calculation. They interpret the result as the average rate of orders shipped within the given time frame.
📈 Deriving the Total Orders Waiting to Ship
In paragraph three, the focus shifts to part C, where Mark and Virge discuss creating an integral expression for the total number of orders waiting to be shipped at t=3 hours without evaluating it. They explain the process of subtracting the outgoing shipped orders rate from the incoming orders rate to find the net change in the number of orders waiting to be shipped.
↗️ or ↘️ Analyzing Order Waiting Trend at t=10 hours
The final paragraph, four, deals with part D, where the presenters determine whether the number of orders waiting to be shipped is increasing or decreasing at t=10 hours. They calculate the difference between the incoming and outgoing rates at that specific time and interpret the sign of the result to conclude the trend. The paragraph concludes with general advice on using a calculator effectively and the importance of taking action, even when unsure of the initial steps.
Mindmap
Keywords
💡AP Calculus
💡Free Response Question
💡Calculator
💡Derivative
💡Integral
💡Rate
💡Units
💡Average Rate
💡Increasing/Decreasing
💡Function
💡Interpretation
Highlights
Mark Kiraly and Virge Cornelius discuss a free response question from AP Calculus that involves heavy use of a calculator.
The problem involves an online bookseller's order and shipping rates, represented by the functions B(t) and S(t), respectively.
The initial number of orders in the system is 57, and the question asks to find B'(2) and interpret its meaning.
Mark demonstrates storing functions in the calculator for easy reference throughout the problem-solving process.
The derivative B'(2) is calculated to be 5.6568, representing the rate at which orders are received per hour per hour at 2 hours after opening.
The integral of S(t) from 1 to 5, divided by 4, is calculated to find the average rate of orders shipped.
Mark emphasizes the importance of knowing your calculator well and using it to its full potential during exams.
The interpretation of the integral result indicates an average rate of 10.545 orders per hour shipped between 1 and 5 hours after opening.
An integral expression is written to represent the total number of orders waiting to be shipped at t = 3 hours without evaluating it.
The expression accounts for the initial 57 orders and the difference between incoming and outgoing orders over time.
The question of whether the number of orders waiting to be shipped is increasing or decreasing at t = 10 hours is addressed.
Mark and Virge discuss the approach of taking the difference between the rate of incoming and outgoing orders to determine the trend.
The rate of change at t = 10 hours is found to be negative, indicating a decrease in the number of orders waiting to be shipped.
The importance of using correct units when interpreting mathematical results within the context of the problem is highlighted.
Mark and Virge encourage students to utilize their calculators and to keep working through problems, even if they are unsure of the initial steps.
The session concludes with a reminder that for complex word problems, starting with known actions like differentiation or integration can guide students towards the solution.
The next video will cover a free response question without the use of a calculator, indicating a shift in focus to more conceptual problem-solving.
Transcripts
Browse More Related Video

5 | MCQ | Practice Sessions | AP Calculus AB
2022 AP Calculus AB BC Free Response #1

6 | FRQ (Calculator Active) | Practice Sessions | AP Calculus AB

3 | FRQ (No Calculator) | Practice Sessions | AP Calculus AB

All about Rate In-Rate Out AP Calculus FRQs
2019 AP Calculus AB & BC Free Response Question #1
5.0 / 5 (0 votes)
Thanks for rating: