Intro to Lipschitz Continuity + Examples
TLDRIn this informative video, the presenter delves into the concept of Lipschitz continuity, a mathematical property that sits between normal continuity and C1 continuity. The video uses the example of the function x squared to illustrate the concept, explaining that a function is Lipschitz continuous if there exists a constant 'l' such that the absolute difference in function values at any two points 'x' and 'y' is no more than 'l' times the absolute difference between 'x' and 'y'. The presenter further clarifies that while C1 functions (those with continuous derivatives) are automatically Lipschitz continuous, the converse is not always true. The video provides a clear explanation of how to determine the Lipschitz constant for given functions and how this concept can simplify the analysis of certain functions, such as arctangent and the square root function, without needing to resort to complex formulas. The content is engaging and provides a solid understanding of Lipschitz continuity, making it accessible to viewers with a basic understanding of calculus.
Takeaways
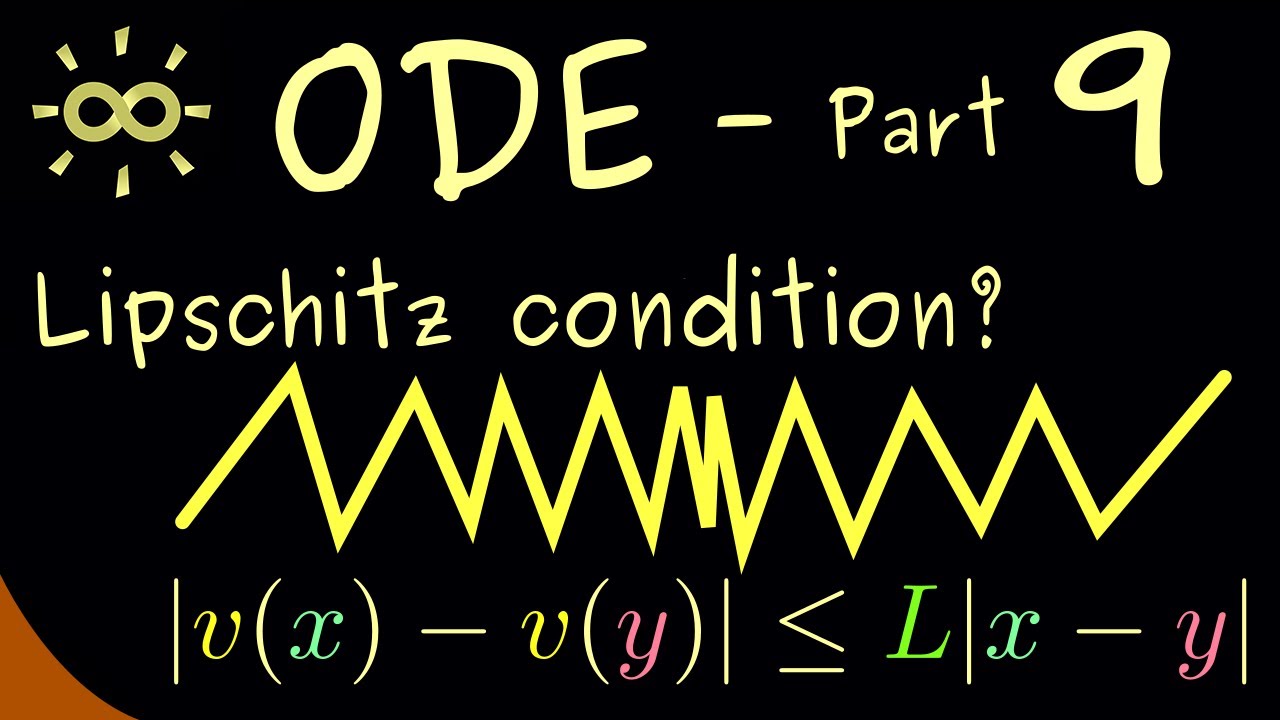
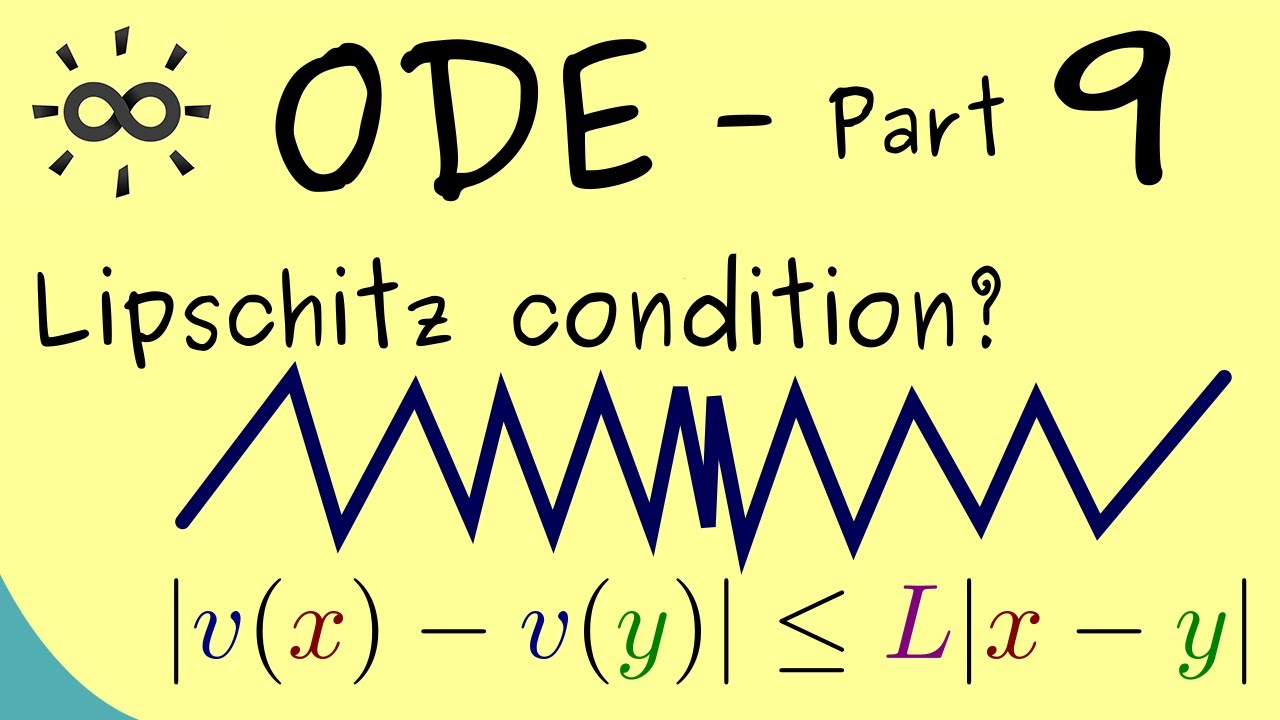
- 📐 **Lipschitz Continuity**: A function is Lipschitz continuous if there exists a constant 'l' such that the absolute difference of the function values at any two points 'x' and 'y' is at most 'l' times the absolute difference of 'x' and 'y'.
- 📈 **Cone Visualization**: Lipschitz continuity can be visualized by ensuring the graph of the function lies within a cone of slope 'l'.
- 🌐 **Global vs. Local**: A function can be globally Lipschitz continuous on its entire domain or locally Lipschitz continuous in a neighborhood around a point.
- 🔢 **Lipschitz Constant**: The Lipschitz constant 'l' is a measure that bounds how much the function can change and is always greater than zero.
- ⛰ **C1 Functions**: If a function is in the C1 class (its derivative is continuous), it is also Lipschitz continuous because the maximum derivative can serve as the Lipschitz constant.
- 🚫 **Non-Example**: A function like 1/√x is not Lipschitz continuous, as its derivative does not bound the rate of change sufficiently.
- 🧮 **Fundamental Theorem of Calculus**: For C1 functions, the difference between function values can be expressed as the integral of the derivative over the interval between 'x' and 'y'.
- 📉 **Bounded at Infinity**: For a function to be Lipschitz continuous, it should be bounded at infinity, meaning its behavior does not 'explode' towards infinity.
- 🔄 **Arctangent Example**: The function arctan(x) is Lipschitz continuous, and its Lipschitz constant can be determined using the integral of its derivative.
- 📋 **Compact Subsets**: On compact subsets of the real line, even functions that are not globally Lipschitz can exhibit Lipschitz continuity.
- 🔍 **Domain Consideration**: The domain of definition for a Lipschitz continuous function must be considered, as behavior at the boundaries or at infinity can affect its continuity properties.
Q & A
What is the difference between Lipschitz continuity and normal continuity?
-Lipschitz continuity is a stronger condition than normal continuity. It requires that there exists a constant 'l' such that the absolute difference between the function values at any two points 'x' and 'y' is less than or equal to 'l' times the absolute difference of the points themselves. Normal continuity only requires that the function values approach the same limit as 'x' approaches 'y'.
What is a Lipschitz constant?
-A Lipschitz constant, denoted as 'l', is a non-negative real number associated with a Lipschitz continuous function. It bounds the rate at which the function value can change with respect to changes in the input.
How does the concept of a cone relate to Lipschitz continuity?
-In the context of Lipschitz continuity, a cone is used to visualize the condition that the function values must satisfy. The function must lie within a cone of slope 'l', which means that for any two points 'x' and 'y', the vertical distance between the function values at these points is less than or equal to 'l' times the horizontal distance between the points.
What is the relationship between C1 functions and Lipschitz continuity?
-Every C1 function (a function with a continuous derivative) is also Lipschitz continuous. This is because the derivative of a C1 function provides an upper bound on the rate of change of the function, which can be used as the Lipschitz constant.
Why is the function f(x) = x^2 Lipschitz continuous on the interval [1, 2]?
-The function f(x) = x^2 is Lipschitz continuous on the interval [1, 2] because its derivative f'(x) = 2x is bounded. The maximum value of the derivative on this interval is 4 (when x = 2), which can serve as the Lipschitz constant for the function on this interval.
What does it mean for a function to be globally Lipschitz continuous?
-A function is globally Lipschitz continuous if it is Lipschitz continuous on its entire domain. This means there exists a single Lipschitz constant 'l' that bounds the rate of change of the function for all points in its domain.
Is Lipschitz continuity a local or global property?
-Lipschitz continuity can be both a local and a global property. A function can be locally Lipschitz continuous, meaning it satisfies the Lipschitz condition on every interval around each point in its domain, or it can be globally Lipschitz continuous, satisfying the condition over its entire domain.
What is the derivative of the function f(x) = arctan(x), and how does it relate to Lipschitz continuity?
-The derivative of f(x) = arctan(x) is f'(x) = 1/(1+x^2). This derivative is always positive and does not exceed 1 for all real x, which means that the function is Lipschitz continuous with a Lipschitz constant of at most 1.
How does the function f(x) = 1/sqrt(x) demonstrate that Lipschitz continuity does not imply C1?
-The function f(x) = 1/sqrt(x) is Lipschitz continuous but not C1 because its derivative f'(x) = -1/(2*sqrt(x)^3) becomes unbounded as x approaches 0. This shows that a function can be Lipschitz continuous without having a continuous derivative, thus not satisfying the C1 condition.
What is the maximum value of the derivative of a function that is C1 and bounded at infinity?
-For a function that is C1 (has a continuous derivative) and bounded at infinity, the maximum value of its derivative can serve as the Lipschitz constant. This is because the derivative provides an upper bound on the rate of change of the function, and if the function is bounded at infinity, the derivative does not blow up.
What is the interval of definition for the function f(x) = sqrt(x) that makes it locally Lipschitz continuous?
-The function f(x) = sqrt(x) is locally Lipschitz continuous for any interval 'j' that does not include the point x = 0, since the function is not defined for negative values and the derivative at x = 0 is not finite. For any interval 'j' > 0, the function is Lipschitz continuous with a constant that can be determined by the maximum of the derivative over that interval.
Outlines
📚 Introduction to Lipschitz Continuity
This paragraph introduces the concept of Lipschitz continuity, which is a type of continuity that imposes stricter conditions on a function than regular continuity but is less stringent than C1 continuity. The speaker uses the example of the function x squared over the interval from 1 to 2 to illustrate the concept. Lipschitz continuity is defined by the existence of a Lipschitz constant 'l' such that the absolute difference of the function values at any two points 'x' and 'y' is less than or equal to 'l' times the absolute difference of 'x' and 'y'. The example demonstrates how the entire function can be contained within a cone of slope 'l', and the Lipschitz constant for the example is determined to be four.
🔍 Differentiating Lipschitz from C1 Continuity
The second paragraph delves into the differences between Lipschitz continuity and C1 continuity. C1 continuity implies that the derivative of the function is continuous, which is a stronger condition than Lipschitz continuity. The speaker explains that if a function is C1, its derivative can be integrated over an interval to find the difference in function values, which can then be bounded by the maximum value of the derivative (referred to as the infinity norm) times the difference in 'x' and 'y'. This paragraph also clarifies that while every C1 function that is bounded at infinity is also Lipschitz continuous, the converse is not necessarily true. Examples are provided to illustrate these concepts, including the function x squared and the arctangent function.
🌐 Local vs. Global Lipschitz Continuity
The final paragraph discusses the distinction between local and global Lipschitz continuity. It provides an example of a function, f(x) = 1/√x, which is not C1 but is locally Lipschitz continuous. The speaker shows that while the derivative of this function does not exist at x=0, the function itself satisfies the Lipschitz condition locally on intervals not including zero. The Lipschitz constant for this function varies depending on the interval considered. The paragraph concludes with a reminder that care must be taken when approaching points where the function may not be well-behaved, such as x approaching zero in this case.
Mindmap
Keywords
💡Elliptical Continuity
💡Lipschitz Constant
💡C1 Continuity
💡Fundamental Theorem of Calculus
💡Arctan Function
💡Local vs Global Lipschitz Continuity
💡Compact Subset
💡Infinity Norm
💡Derivative
💡Square Root Function
💡Difference of Squares
Highlights
The video discusses Lipschitz continuity, a type of continuity that is between normal continuity and C1.
Lipschitz continuity enforces additional conditions on a function beyond just requiring it to be continuous.
A function f is Lipschitz continuous if for all x, y in an interval I, there exists an L such that |f(x) - f(y)| ≤ L|x - y|.
L is called the Lipschitz constant and can be greater than zero.
The whole function can be contained within a cone of slope L as an example to visualize Lipschitz continuity.
If the Lipschitz constant exists for the whole interval, the function is said to be globally Lipschitz continuous.
If the constant only exists locally around any domain, the function is locally Lipschitz continuous.
Being C1 is a stronger requirement than just being Lipschitz continuous.
If a function is C1, its derivative is continuous, and it satisfies a certain integral inequality involving the derivative.
A C1 function that is bounded at infinity is also Lipschitz continuous, with the maximum derivative as the Lipschitz constant.
Examples are given to illustrate when a function is Lipschitz continuous, like x^2, and when it is not, like 1/sqrt(x).
The function 1/sqrt(x) is not C1 since its derivative is not continuous, but it is locally Lipschitz continuous.
The video provides a formula to calculate the Lipschitz constant for the square root function on an interval (a, b).
The arctangent function is shown to be globally Lipschitz continuous with a constant of 1, using an integral inequality.
The video emphasizes the importance of restricting the domain to avoid issues at infinity for certain functions.
Lipschitz continuity provides a way to bound the rate of change of a function, which is useful for analysis and applications.
The video concludes by encouraging viewers to suggest topics for future videos on mathematical concepts.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: