The magic of Fibonacci numbers | Arthur Benjamin | TED

TED
8 Nov 201306:25
EducationalLearning
32 Likes 10 Comments

TLDRThe speaker emphasizes the importance of learning mathematics for three main reasons: calculation, application, and inspiration. They argue that while calculation and application are often the focus, inspiration is sadly overlooked. To illustrate the beauty of mathematics, the speaker uses the Fibonacci sequence as an example, showing how it can be appreciated through simple calculations, its prevalence in nature, and the intriguing patterns it forms. They demonstrate a surprising pattern involving the squares of Fibonacci numbers and explain its connection to the Golden Ratio. The speaker concludes by advocating for a greater appreciation of the beauty and thinking behind mathematics in education, stating that it's not just about solving equations but understanding the 'why' behind them.

Takeaways
  • ๐Ÿงฎ Mathematics is studied for three main reasons: calculation, application, and inspiration.
  • ๐Ÿ” Mathematics is the science of patterns and helps us learn logical, critical, and creative thinking.
  • ๐Ÿ“š The motivation behind learning math in school is often not effectively communicated, leading to questions about its relevance.
  • ๐Ÿ˜Š Mathematics can be appreciated for its fun, beauty, and mind-excitement, not just for its utility.
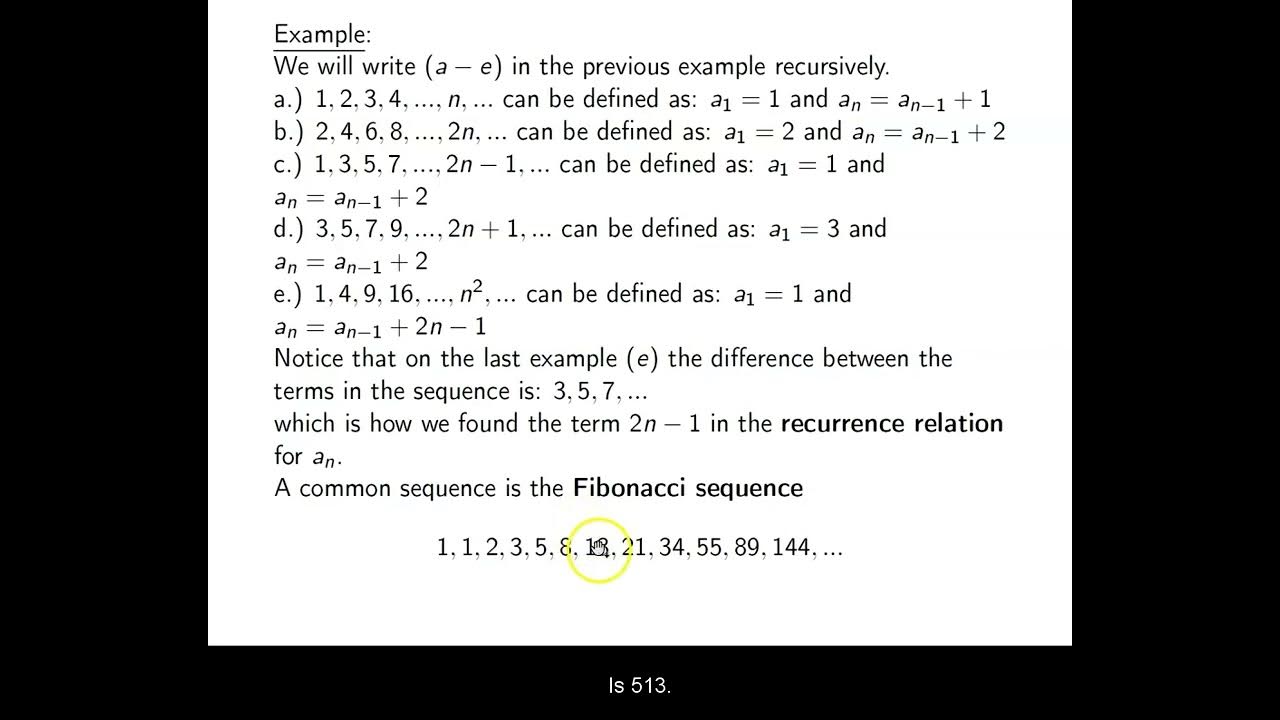
  • ๐ŸŒฟ Fibonacci numbers are a favorite example that can be appreciated in various ways, including their simplicity and natural occurrences.
  • ๐Ÿ“– Fibonacci numbers were introduced to the Western world by Leonardo of Pisa in his book 'Liber Abaci'.
  • ๐ŸŒผ Fibonacci numbers are found in nature, such as the arrangement of petals on a flower or spirals on a sunflower.
  • ๐Ÿ”ข The squares of Fibonacci numbers exhibit intriguing patterns, such as the sum of the squares of consecutive Fibonacci numbers resulting in the next number in the sequence.
  • ๐Ÿ“ By visualizing the pattern through geometric shapes, the mathematical reasoning behind these patterns becomes clear.
  • ๐Ÿ“ˆ The ratio of consecutive Fibonacci numbers approximates the Golden Ratio (1.618), a concept that has captivated various fields.
  • ๐Ÿ’ก Mathematics is not just about solving equations but also about understanding the 'why' behind them, which is crucial for learning how to think.
Q & A

  • Why do we learn mathematics according to the speaker?

    -We learn mathematics for three main reasons: calculation, application, and inspiration.

  • What is the science of patterns?

    -Mathematics is the science of patterns, which helps us learn how to think logically, critically, and creatively.

  • Why do students often hear that they need to learn mathematics for upcoming math classes or future tests?

    -This is because the motivation behind learning mathematics is not effectively communicated, and students are often given practical reasons related to academic requirements.

  • What is the speaker's suggestion for an alternative reason to learn mathematics?

    -The speaker suggests that we should sometimes do mathematics because it is fun, beautiful, or because it excites the mind.

  • Who was Fibonacci and why is he significant?

    -Fibonacci, actually named Leonardo of Pisa, introduced the Fibonacci numbers in his book 'Liber Abaci', which taught the Western world the arithmetic methods we use today.

  • How do Fibonacci numbers appear in nature?

    -Fibonacci numbers appear in nature in various forms, such as the number of petals on a flower or the number of spirals on a sunflower or a pineapple, which are often Fibonacci numbers.

  • What is a surprising pattern that occurs when you add the squares of consecutive Fibonacci numbers?

    -When you add the squares of consecutive Fibonacci numbers, the result is another number that is a product of two consecutive Fibonacci numbers.

  • How does the speaker demonstrate the relationship between the squares of Fibonacci numbers and the number 13?

    -The speaker uses a visual representation of squares to show that the sum of the squares of the first few Fibonacci numbers equals eight times 13, which is the area of a rectangle formed by these squares.

  • What is the Golden Ratio and how is it related to the Fibonacci sequence?

    -The Golden Ratio, approximately 1.618, is a number that has fascinated mathematicians, scientists, and artists. It is related to the Fibonacci sequence as the ratio of consecutive Fibonacci numbers tends to approach the Golden Ratio.

  • What does the speaker believe is the most important application of mathematics?

    -The speaker believes that the most important application of mathematics is learning how to think, which includes not just solving for x, but also figuring out why.

  • What is the main message the speaker wants to convey about mathematics?

    -The main message is that mathematics is not just about solving equations but also about appreciating its beauty, understanding its applications, and learning to think logically, critically, and creatively.

  • Why does the speaker believe that the beauty of mathematics does not get enough attention in schools?

    -The speaker believes that the focus on calculation and practical applications in schools often overshadows the inspirational and beautiful aspects of mathematics, which can excite the mind and foster a deeper appreciation for the subject.

Outlines
00:00
๐Ÿ“š The Purpose and Beauty of Mathematics

This paragraph discusses the three main reasons why we learn mathematics: calculation, application, and inspiration. It emphasizes the importance of understanding the logic, critical thinking, and creativity inherent in mathematics. The speaker highlights the lack of motivation in traditional math education and proposes that math should sometimes be appreciated for its beauty and fun. The Fibonacci sequence is used as an example to demonstrate how numbers can be both simple and profoundly inspiring, with applications in nature and intriguing patterns that can be discovered through mathematical exploration.

05:01
๐ŸŒผ Fibonacci Numbers and the Golden Ratio

The second paragraph delves into the Fibonacci numbers, illustrating their simplicity and their surprising prevalence in nature, such as the arrangement of petals on flowers and spirals on plants. The speaker introduces an intriguing pattern related to the squares of Fibonacci numbers, showing that the sum of the squares of consecutive Fibonacci numbers results in another number that is a multiple of a subsequent Fibonacci number. This leads to an exploration of the Golden Ratio, a mathematical constant approximately equal to 1.618, which is found by dividing a pair of consecutive Fibonacci numbers. The paragraph concludes with the assertion that mathematics is about more than just solving equations; it's also about understanding the 'why' behind mathematical truths.

Mindmap
Keywords
๐Ÿ’กMathematics
Mathematics is the abstract science of number, quantity, and space, often involving patterns, structures, and relationships. In the video, it is presented as a discipline that goes beyond calculation and application, encompassing inspiration and the beauty of patterns. It is the central theme, with the speaker advocating for a more holistic approach to learning math that includes enjoyment and aesthetic appreciation.
๐Ÿ’กCalculation
Calculation refers to the process of performing arithmetic operations to obtain a numerical result. The video emphasizes that while calculation is a fundamental aspect of mathematics, it is not the only reason we study math. Calculations are exemplified through the simple addition involved in generating Fibonacci numbers.
๐Ÿ’กApplication
Application in the context of the video means the practical use of mathematical concepts in real-world scenarios. Fibonacci numbers, for instance, are highlighted for their frequent appearance in nature, such as the arrangement of petals on a flower or the spirals on a pineapple, demonstrating the relevance of math in understanding natural phenomena.
๐Ÿ’กInspiration
Inspiration is the process of being motivated or stimulated intellectually or emotionally. The video argues that inspiration is an undervalued aspect of learning mathematics in schools. The beauty and patterns found in math, such as those in Fibonacci numbers, are presented as sources of inspiration that can excite the mind and foster a deeper appreciation for the subject.
๐Ÿ’กFibonacci Numbers
Fibonacci numbers are a series of numbers in which each number is the sum of the two preceding ones, usually starting with 0 and 1. Named after Leonardo of Pisa, also known as Fibonacci, these numbers are used in the video to illustrate the concepts of calculation and application, as well as to inspire with their inherent patterns and their occurrence in nature.
๐Ÿ’กPatterns
Patterns in mathematics refer to the regularity and repetition of numbers, shapes, or structures. The video discusses how mathematics is the science of patterns, using the Fibonacci sequence as an example of how mathematical patterns can be both simple and profound, leading to the discovery of deeper mathematical truths, such as the Golden Ratio.
๐Ÿ’กLogical Thinking
Logical thinking is the process of reasoning that uses a set of general principles which are applied to reach a conclusion. The video emphasizes the importance of learning to think logically, critically, and creatively through the study of mathematics, which is not just about performing calculations but also about understanding the 'why' behind mathematical concepts.
๐Ÿ’กGolden Ratio
The Golden Ratio, often denoted by the Greek letter ฯ† (phi), is a mathematical constant approximately equal to 1.618. It is found in various natural and artistic contexts and is known for its aesthetic properties. In the video, the Golden Ratio is derived from the proportions of rectangles formed by the squares of Fibonacci numbers, illustrating the harmony and beauty inherent in mathematical relationships.
๐Ÿ’กLiber Abaci
Liber Abaci, which translates to 'Book of Calculation,' is a 12th-century book by Leonardo of Pisa, also known as Fibonacci. It introduced the Hindu-Arabic numeral system to the Western world and is mentioned in the video as a historical reference that connects the Fibonacci numbers to the broader development of arithmetic and mathematics.
๐Ÿ’กCritical Thinking
Critical thinking involves analyzing and evaluating information to form a judgment. The video stresses the importance of teaching students not just to perform mathematical operations but to engage in critical thinking about why and how these operations work, fostering a deeper understanding of mathematical principles.
๐Ÿ’กCreativity
Creativity in the context of mathematics refers to the ability to come up with new ideas or concepts, often by combining existing ideas in novel ways. The video suggests that studying mathematics helps develop creativity, as it involves thinking beyond the immediate problem to explore and discover new patterns and relationships, such as the unexpected pattern found when squaring Fibonacci numbers.
Highlights

We learn mathematics for three main reasons: calculation, application, and inspiration.

Mathematics is the science of patterns and helps us to think logically, critically, and creatively.

Many students are not effectively motivated to learn math and often hear it's for future tests.

The speaker advocates for learning math for its own beauty and excitement.

Fibonacci numbers are introduced as an example of mathematical beauty and application.

Fibonacci numbers are easy to understand and have appeared since Leonardo of Pisa's 'Liber Abaci'.

Fibonacci numbers are found in nature, such as the number of petals on a flower or spirals on a sunflower.

The Fibonacci sequence shows beautiful patterns, such as the sum of squares of consecutive Fibonacci numbers.

The sum of the squares of the first few Fibonacci numbers results in numbers that contain buried Fibonacci numbers.

The area of a rectangle formed by Fibonacci squares can be calculated in two different ways, leading to the same result.

The ratio of consecutive Fibonacci numbers approaches the Golden Ratio (1.618).

The Golden Ratio has been a fascination for mathematicians, scientists, and artists for centuries.

The beauty of mathematics is often overlooked in schools, where focus is on calculation.

Mathematics is not just about solving for x, but also about understanding why.

The importance of learning how to think is highlighted as a key application of studying mathematics.

The speaker emphasizes the need to appreciate the beauty and inspiration in mathematics, not just its practical applications.

The talk concludes with a call to recognize the full spectrum of what mathematics has to offer, beyond just calculation.

Transcripts

Browse More Related Video

The Fibonacci Sequence: Nature's Code

The Beauty of Math and Music | Marcus Miller | TEDxOttawa

Mathematics is the sense you never knew you had | Eddie Woo | TEDxSydney

The fabulous Fibonacci flower formula

The Golden Ratio: Is It Myth or Math?

Ch. 12.1 Sequences and Summation Notation

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsFibonacciPatternsLogical ThinkingGolden RatioEducationInspirationCreativityNumeracyRatiosApplications