Using the Quadratic Formula (Precalculus - College Algebra 21)

Professor Leonard

4 Mar 202046:04

EducationalLearning

32 Likes 10 Comments

TLDRThe video script offers an in-depth exploration of the quadratic formula, detailing its application in solving quadratic equations and finding x-intercepts. It emphasizes the importance of setting up the equation correctly and identifying coefficients (a, b, and c) for the formula. The script also discusses the significance of the discriminant in determining the nature of the solutions—real, complex, or a repeated root. Additionally, it highlights the technique of simplifying expressions and avoiding fractions in the quadratic formula for ease of computation. The video aims to demystify the quadratic formula, making it accessible and understandable for viewers.

Takeaways

📐 The quadratic formula is a universal method to find the x-intercepts of a parabola, which is represented by a quadratic equation.
✅ Always set the quadratic equation equal to zero and ensure the first term is positive to avoid sign errors when applying the quadratic formula.
🔍 Before using the quadratic formula, attempt simpler methods like the square root method or factoring, if applicable.
📉 The discriminant (the part under the square root in the quadratic formula) determines the nature of the solutions: two real solutions, one real solution, or two complex solutions.
🤔 When the discriminant is positive, the parabola crosses the x-axis twice. If it's zero, the parabola touches the x-axis at one point (vertex). If it's negative, the parabola misses the x-axis completely.
🚫 Avoid plugging in fractions directly into the quadratic formula as it complicates the process; instead, eliminate fractions by multiplying through by the least common denominator.
📝 Memorize the quadratic formula and practice creating a consistent pattern for plugging in the coefficients (a, b, and c) to minimize errors.
🔢 The quadratic formula is derived from completing the square, and understanding this derivation can help in recognizing the formula's structure and application.
🏗 Use parentheses in the quadratic formula to maintain the signs and operations, especially when dealing with negative coefficients.
➗ When simplifying the results from the quadratic formula, look for perfect square factors to simplify the square root, if possible.
✉️ Remember that the solutions provided by the quadratic formula are exact, and any decimal approximations are rounded estimates of these exact values.

Q & A

What is the main topic of the video?
-The main topic of the video is the quadratic formula, discussing how to use it to find x-intercepts of quadratic equations.
Why is the quadratic formula considered a universal method for solving quadratic equations?
-The quadratic formula is considered universal because it works for all quadratic equations, unlike factoring or the square root method, which only work under certain conditions.
What does the video suggest doing before applying the quadratic formula?
-The video suggests setting the quadratic equation equal to zero, trying the square root method, and attempting to factor the equation before applying the quadratic formula.
How does the video recommend setting up the quadratic formula for ease of use?
-The video recommends setting up the quadratic formula with blank spaces for coefficients and creating a pattern for filling in the coefficients to avoid sign errors and maintain consistency.
What is the significance of the discriminant in the quadratic formula?
-The discriminant, which is the part inside the square root in the quadratic formula, determines the nature of the solutions: two real solutions, one real solution, or two complex solutions.
What does the video say about the relationship between the quadratic formula and completing the square?
-The video explains that the quadratic formula is derived from the process of completing the square and that they yield similar results.
Why should you avoid plugging in fractions when using the quadratic formula?
-Plugging in fractions makes the work ten times harder and can lead to more opportunities for sign errors. It's better to eliminate fractions by multiplying both sides of the equation by the least common denominator (LCD).
What is the purpose of the x-intercepts in the context of the video?
-The x-intercepts are the points where the parabola of the quadratic function crosses the x-axis, and the quadratic formula is used to find these points.
How does changing the sign of the first term in a quadratic equation affect the use of the quadratic formula?
-Changing the sign of the first term to positive can help avoid sign errors and simplifies the use of the quadratic formula, especially when dealing with negative coefficients.
What does the video suggest for dealing with complex solutions in the quadratic formula?
-The video suggests that when the discriminant is negative, resulting in complex solutions, you should simplify the expression to express the solutions in terms of 'i', the imaginary unit.
Why is it important to identify the A, B, and C coefficients in the quadratic formula?
-Identifying the A, B, and C coefficients is crucial for correctly applying the quadratic formula. They correspond to the terms in the quadratic equation (ax^2 + bx + c = 0) and their correct identification ensures accurate calculation of the solutions.

Outlines

00:00

📚 Introduction to Quadratic Formula

The video begins with an introduction to the quadratic formula, emphasizing its utility in finding x-intercepts of quadratic equations. The presenter discusses the formula's consistency and reliability compared to other methods like factoring, square root method, and completing the square. The focus is on setting up the formula correctly and avoiding common pitfalls, such as sign errors, especially when dealing with a negative leading coefficient.

05:01

🔍 Understanding and Applying the Quadratic Formula

The presenter explains the quadratic formula's structure, advocating for the use of parentheses to maintain the correct signs and operations. They demonstrate how to plug in coefficients (a, b, and c) into the formula systematically, creating a pattern to avoid errors. The video also covers the concept of the discriminant, which determines the nature of the solutions (real or complex) and the number of x-intercepts.

10:02

🔢 Simplifying Solutions and Recognizing Discriminant Cases

The video continues with an example of applying the quadratic formula, emphasizing the importance of simplifying the results and understanding the discriminant's role in determining the number of real solutions. The presenter also highlights the similarities between the quadratic formula and completing the square, noting that the formula can be derived from this method.

15:03

📉 Analyzing Parabolas and Their x-Intercepts

The presenter discusses how to identify the direction in which a parabola opens and whether it will cross the x-axis based on the coefficient of the quadratic term. They also explain how to handle quadratic equations with fractions by multiplying through by the least common denominator to simplify the equation before applying the quadratic formula.

20:04

❌ Avoiding Fractions in Quadratic Formula

The video stresses the importance of avoiding fractions when using the quadratic formula, as they can complicate the process. The presenter suggests multiplying by the LCD to eliminate fractions before applying the formula. They also provide a method to simplify the square root of the discriminant by finding the largest perfect square factor.

25:06

🤔 Dealing with Negative Coefficients and Complex Solutions

The presenter addresses how to handle quadratic equations with a negative leading coefficient, suggesting multiplying through by -1 to make the coefficient positive. They also explain how to interpret the discriminant when it's negative, indicating that the quadratic equation has no real solutions and instead has complex solutions.

30:10

📐 Reflecting on Quadratic Formula and Parabola Characteristics

The video concludes with a discussion on the implications of changing the sign of a quadratic equation on the parabola's graph. The presenter clarifies that while the graph's appearance may change, the x-intercepts (the points where the parabola crosses the x-axis) remain the same, which is the primary concern when using the quadratic formula.

35:10

🔗 Wrapping Up and Previewing Future Topics

In the final paragraph, the presenter summarizes the key points about the quadratic formula and its application. They also give a preview of what will be covered in the next video, which will focus on the intersections of functions, adding another layer to understanding quadratic equations and their graphs.

Mindmap

Keywords

💡Quadratic Formula

The quadratic formula is a standard algebraic formula for solving quadratic equations, which are equations of the second degree. It is given by x = (-b ± √(b² - 4ac)) / (2a), where a, b, and c are coefficients of the quadratic equation ax² + bx + c = 0. In the video, the quadratic formula is discussed as a universal method to find the x-intercepts of a parabola, which is the main theme of the video.

💡X-Intercepts

X-intercepts, also known as zeros or roots, are the points where a parabola or graph of a quadratic function intersects the x-axis. They are important in the context of the video because the primary purpose of using the quadratic formula is to find these points. The script mentions setting the quadratic function equal to zero to find x-intercepts.

💡Discriminant

The discriminant is the part of the quadratic formula under the square root, represented as b² - 4ac. It determines the nature of the roots of a quadratic equation. If the discriminant is positive, there are two distinct real roots; if it's zero, there's exactly one real root (or a repeated root); and if it's negative, the roots are complex and the parabola does not intersect the x-axis. The video explains the role of the discriminant in determining the number of solutions to a quadratic equation.

💡Completing the Square

Completing the square is a method for solving quadratic equations by transforming them into a perfect square trinomial. It involves rewriting the quadratic equation in the form of (x - h)² = k, where h and k are constants. The video briefly mentions this method but focuses more on the quadratic formula as a universal solver.

💡Factoring

Factoring is a mathematical technique used to break down a quadratic equation into two binomial factors. If the equation can be factored, it can be solved by setting each factor equal to zero. The video script discusses attempting to factor a quadratic equation before resorting to the quadratic formula if factoring is not possible.

💡Square Root Method

The square root method is a technique used to solve quadratic equations when one side can be isolated as a perfect square trinomial. It involves taking the square root of both sides to solve for x. The video mentions this method but notes that it is not applicable in all cases, unlike the quadratic formula.

💡Quadratic Equation

A quadratic equation is a polynomial equation of the second degree, typically in the form ax² + bx + c = 0, where a, b, and c are constants. The video's main focus is on solving these types of equations using the quadratic formula.

💡Parabola

A parabola is a U-shaped curve that is the graph of a quadratic equation y = ax² + bx + c. The video discusses the properties of parabolas, such as whether they open upward or downward and their width, in relation to the quadratic formula and x-intercepts.

💡Standard Form

Standard form of a quadratic equation is when it is written as ax² + bx + c = 0, with the terms ordered from highest to lowest degree and set equal to zero. The video emphasizes the importance of having the equation in standard form before applying the quadratic formula.

💡LCD (Least Common Denominator)

The Least Common Denominator (LCD) is the smallest multiple that each of the denominators of a set of fractions can divide into. In the context of the video, the LCD is used to eliminate fractions from a quadratic equation before applying the quadratic formula, simplifying the process.

💡Sign Errors

Sign errors are mistakes made in mathematical calculations due to incorrect handling of plus and minus signs. The video script warns about the potential for sign errors when dealing with negative coefficients in the quadratic formula and provides strategies to avoid them.

Highlights

The video introduces the quadratic formula and its application in finding x-intercepts of a parabola.

Explains that the quadratic formula is a universal method that works all the time, unlike some other methods like the square root method.

Provides a systematic way to set up the quadratic formula to avoid errors and maintain consistency.

Discusses the importance of setting the quadratic equation to zero and having the first term positive for the formula to work effectively.

Demonstrates how to identify coefficients A, B, and C from the quadratic equation before applying the formula.

Uses parentheses in the formula to maintain the signs and operations, which is crucial for accuracy.

Stresses the need to avoid sign errors when dealing with the negative B term in the quadratic formula.

Introduces the concept of the discriminant, explaining its role in determining the nature of the solutions (real or complex).

Shows how the quadratic formula can be applied to different types of quadratic equations, including those with fractions.

Advises against plugging in fractions directly into the quadratic formula, instead suggesting to eliminate fractions first.

Provides a method to simplify the square root in the formula by finding the largest perfect square factor.

Explains the process of changing the sign of the first term in a quadratic equation to positive to avoid complications with the formula.

Illustrates the calculation of complex solutions using the quadratic formula when the discriminant is negative.

Clarifies the difference between exact solutions and approximations when dealing with irrational numbers like the square root of a non-perfect square.

Details how to handle equations with a negative first term by multiplying through by -1 to make the first term positive.

Demonstrates the application of the quadratic formula to find x-intercepts, emphasizing that the formula only provides the x-values of the intercepts.

Concludes with a reminder that the quadratic formula is a fail-safe method for finding x-intercepts, regardless of the equation's complexity.

Transcripts

Browse More Related Video

Quadratic Formula – MOST COMMON MISTAKES!

Proving the Quadratic Formula - Twice (Precalculus - College Algebra 20)

Algebra Pop Quiz: what is the discriminant and why is it important?

Learn The Quadratic Formula in 10 min

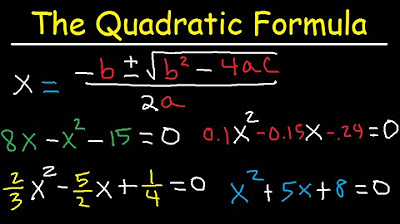
How To Use The Quadratic Formula To Solve Equations

Quadratic Equations Multiple Choice Practice Problems

Related Tags

Quadratic Formula Mathematics X-Intercepts Parabola Solving Equations Algebra Error Avoidance Educational Content Math Tips Complex Solutions