Electric Potential

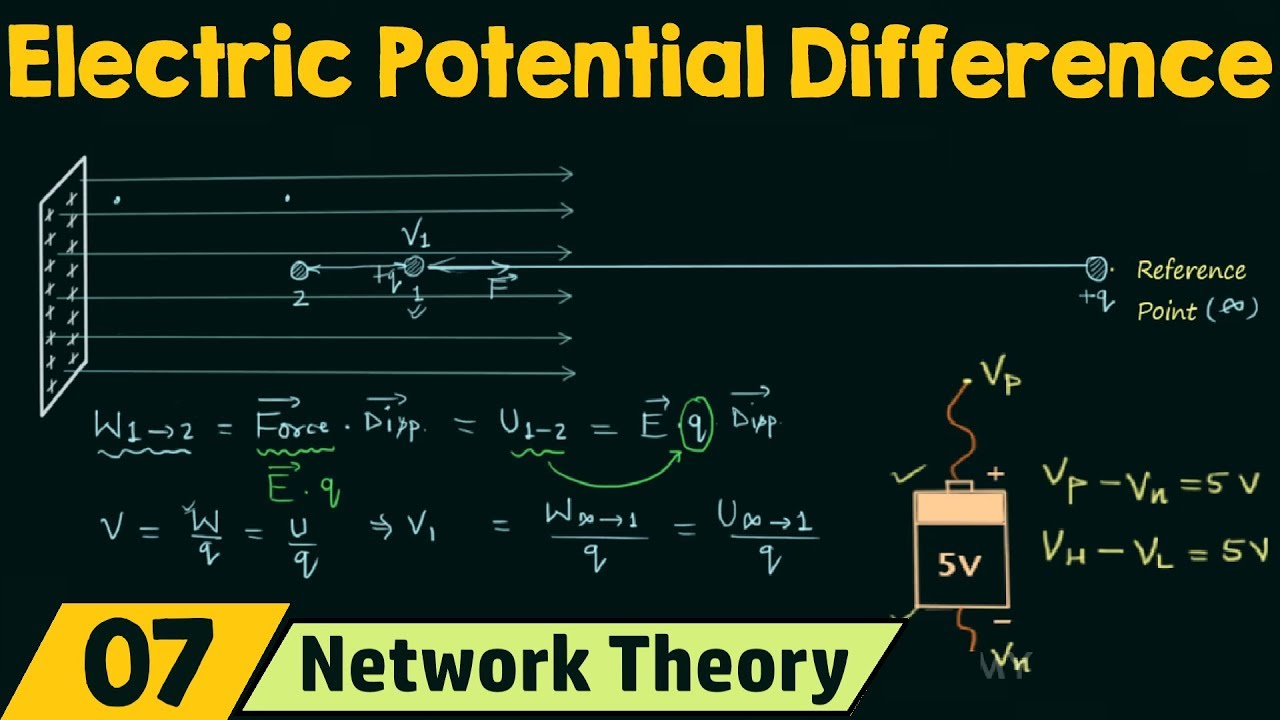
This paragraph introduces the concept of electric potential, defined as the potential energy divided by charge. It distinguishes between different types of potential energy, such as elastic, gravitational, and electric potential energy. The paragraph emphasizes the importance of understanding the difference between electric potential (measured in volts) and electric potential energy (measured in joules). It explains that a one-volt electric potential means a one-coulomb charge has one joule of potential energy, and further illustrates this with examples of different electric potentials and their corresponding potential energies.

This paragraph delves into the relationship between voltage and electric potential. It defines voltage as the electric potential difference, or the change in electric potential. The paragraph uses examples to illustrate how to calculate voltage (V) by finding the difference between the potential at two points (Vb - Va or Va - Vb). It also explains how voltage relates to the work done by an electric field and the potential energy change when a charge moves between two points. The paragraph reinforces the concept by contrasting the direction of potential change and its effect on the calculated voltage.

This paragraph focuses on the calculation of work done when a charge moves through an electric field. It introduces the formula for work in terms of charge (q) and change in potential (ΔV), and explains how the work is related to the change in kinetic and potential energy in a system. The paragraph emphasizes that work is positive when the force and displacement are in the same direction and negative when they are opposite. It also provides an example of calculating the work done to move a negative charge across a potential difference, demonstrating how to apply the formula and interpret the result.

This paragraph explores the relationship between work done on a charge, the electric field, and the motion of the charge. It explains how the direction of the electric field affects the motion of positive and negative charges, and how this relates to the work done by the electric field. The paragraph uses examples to illustrate how a positive charge moves from a high potential to a low potential when accelerated by an electric field, and vice versa for a negative charge. It also discusses the conservation of mechanical energy and how it applies to the work done by conservative forces like the electric field.

This paragraph examines the changes in kinetic and potential energy as a charge accelerates due to an electric field. It explains how the kinetic energy increases as the charge's speed increases, leading to a decrease in potential energy. The paragraph uses a specific example of a positive charge accelerating from rest towards a negatively charged plate, illustrating how the work done by the electric field is positive, resulting in an increase in kinetic energy. It also provides a calculation for the final speed of the charge based on the work done and the charge's mass.

This paragraph discusses the work done by an electric field on a negative charge moving from a lower to a higher electric potential. It explains the direction of the electric field force and how it accelerates the negative charge towards the positively charged plate. The paragraph calculates the work done using the formula involving charge and voltage, and confirms the result by analyzing the direction of the charge's motion and the force applied. It also discusses the implications of the work done on the charge's kinetic energy and the final speed of the charge.