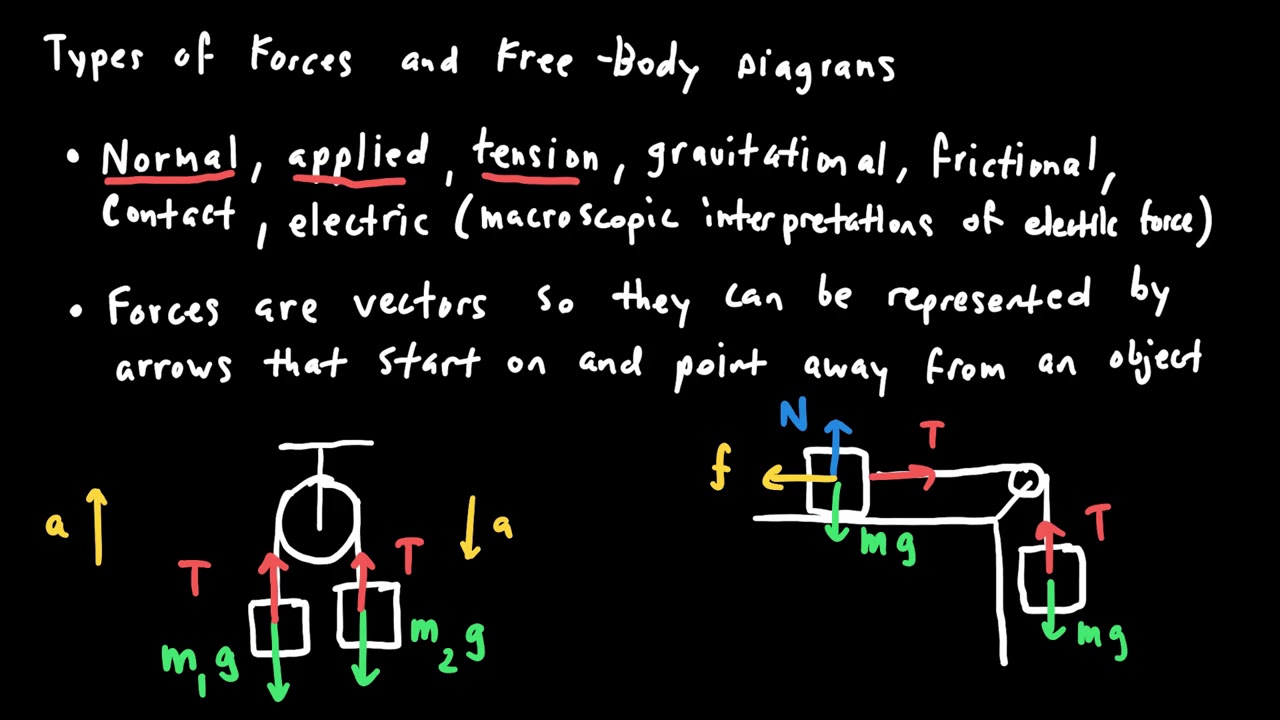
AP Physics Workbook 2.D Newton's Third Law and Eliminating Internal Forces
TLDRThis video script offers a comprehensive walkthrough of Newton's third law and its application to a train system with three cars of varying masses. It explains how to draw Freebody diagrams for each car, label the forces correctly, and set up equations based on Newton's second law. The script then demonstrates how to calculate the tension forces (F1, F2, and F3) for each connection between the cars, emphasizing the relationship between mass, acceleration, and tension. The video also illustrates the concept of action-reaction pairs, reinforcing the understanding of forces in mechanics.
Takeaways
- 🚂 The problem involves a train engine pulling three cars of equal mass, connected by metal bars with tension forces indicated as F1, F2, and F3.
- 📐 The task is to draw accurate Freebody diagrams for each car, labeling the forces correctly, including gravity, normal force, and tension forces.
- 🌐 Newton's second law (F=ma) is central to setting up equations for each car, considering the individual masses and the overall acceleration of 2 m/s².
- 🔄 When analyzing the system, the internal forces (tension forces between the cars) cancel each other out when considering the system as a whole.
- 🎯 The combined mass of the first two cars is 5000 kg, leading to F2 being equal to 5000 times the acceleration.
- 🔢 For the last car (1 kg), the Freebody diagram includes F1, the normal force, gravity, and friction, with F1 being longer due to the rightward acceleration.
- 🌟 When all three cars are considered together, the total mass is 6000 kg, and F1 is calculated to be 12000 N using the combined mass and acceleration.
- 📌 Newton's third law is discussed, stating that for every action, there is an equal and opposite reaction. This is represented by the tension forces between the cars.
- 📈 The script provides a method to calculate the tension in a system of connected objects, emphasizing that the total force is distributed among the individual parts.
- 📚 The video script serves as a comprehensive guide for solving dynamics problems involving multiple connected objects, including the calculation of individual and combined forces.
- 💡 Understanding the distribution of forces and accelerations in a system is crucial for grasping the dynamics of connected objects in physics.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the application of Newton's third law and the concept of tension forces in a physics problem involving a train engine pulling three cars of different masses.
How many cars does the train have and what are their masses?
-The train has three cars with masses of 1 kilogram, 2 kilograms, and 3 kilograms.
What are the forces acting on the cars as described in the script?
-The forces acting on the cars include the force of gravity (downward), the normal force (upward), and the tension forces (horizontal). There is also a mention of friction forces, but it is assumed to be negligible in the problem.
How does the script illustrate the concept of action and reaction forces in Newton's third law?
-The script illustrates the concept of action and reaction forces by explaining that for every force exerted by one object on another, there is an equal and opposite force exerted back. This is shown in the example of the tension forces between the connected cars and the forces exerted by and on the sleigh in the Newton's third law discussion.
What is the acceleration rate of the train cars?
-The train cars are accelerating at a rate of 2 meters per second.
How does the mass of each car affect the tension forces (F1, F2, F3)?
-The mass of each car affects the tension forces by determining the magnitude of the force required to accelerate the car. The larger the mass, the greater the force needed for the same acceleration. For example, F1, which accelerates the combined mass of all three cars, is the largest tension force at 12,000 Newtons.
What is the combined mass when considering the first two cars together?
-When considering the first two cars together, the combined mass is 5000 kilograms (3000 kg + 2000 kg).
How does the script demonstrate the calculation of the tension forces (F1, F2, F3)?
-The script demonstrates the calculation of the tension forces by applying Newton's second law (F = ma) to each car separately and then combining the forces for the combined masses. It shows how to set up equations for each car, solve for the forces, and then use those to determine the individual tension forces.
What is the final calculated value for F1, F2, and F3?
-The final calculated values for the tension forces are F1 = 12,000 Newtons, F2 = 10,000 Newtons, and F3 = 6,000 Newtons.
Why is F1 the largest tension force despite F3 being connected to the greatest mass?
-F1 is the largest tension force because it is responsible for accelerating the entire system, which includes all three cars with a combined mass. Even though F3 is connected to the greatest mass, it only needs to accelerate that one car, hence its value is less than F1.
How can the information in the script be used as a study guide for mechanics problems?
-The information in the script can be used as a study guide for mechanics problems by providing a step-by-step approach to solving for tension forces and understanding the dynamics of connected masses. It serves as a cheat sheet for solving similar problems involving multiple blocks on a horizontal surface and the calculations of acceleration and tension forces.
Outlines
🚂 Analyzing Newton's Third Law in a Train System
This paragraph introduces a physics problem involving a train with three cars of equal mass, connected by metal bars with indicated tension forces. The task is to draw a Freebody diagram for each car, correctly labeling forces such as gravity, normal force, and tension (F1, F2, F3). It emphasizes the importance of accurate force lengths corresponding to the car's mass and the acceleration of 2 meters per second in the absence of friction. The paragraph also explains how to set up equations based on Newton's second law, where force equals mass times acceleration, for each car's mass and the combined masses, leading to the understanding of how the tension forces (F1, F2, F3) interact within the system.
📊 Combining Forces and Masses in a Mechanical System
The second paragraph delves into the algebraic combination of forces and masses to simplify the Freebody diagram. It explains how to combine the forces and masses of the 2,000 kg and 3,000 kg carts, resulting in a single force equation (F2 = 5000 * acceleration). The process is extended to include all three carts, combining their forces and masses to find the total acceleration (6,000 kg * acceleration). The paragraph highlights the calculation of individual tension forces (F1, F2, F3) using the given acceleration value, leading to the understanding that F1, which pulls all masses, has the greatest tension due to the combined mass of the system.
📐 Applying Newton's Third Law to Tension Forces
This paragraph discusses Newton's third law, explaining the concept of action and reaction forces. It uses the train system as an example to illustrate how the tension forces between the cars are equal and opposite. The explanation includes a breakdown of how to calculate the forces for each part of the system, emphasizing the relationship between the forces and the masses involved. The paragraph concludes with a comprehensive cheat sheet for solving mechanics problems on a horizontal surface, including single and multiple block systems with and without friction.
Mindmap
Keywords
💡Newton's Third Law
💡Freebody Diagram
💡Tension Force
💡Acceleration
💡Mass
💡Friction
💡Force
💡Newton's Second Law
💡Dynamics
💡Internal Forces
💡External Forces
Highlights
The scenario involves a train engine pulling a train with three cars, each with the same mass.
The cars are connected by a metal bar with tension forces indicated as F1, F2, and F3.
The train accelerates at a rate of 2 meters per second with no friction force acting on it.
The Freebody diagram for the 3 kg car includes gravity, normal force, and tension force F3.
The lengths of the force of gravity and normal are equal for the 3 kg car due to its mass.
For the 2 kg and 1 kg cars, the forces of gravity and normal are smaller, resulting in shorter Freebody diagram lengths.
The tension force F3 is the same length in the Freebody diagrams for all cars, representing the connection between them.
The equation for each car is set up using Newton's second law, with forces being the mass times the acceleration.
When combining the first two cars, the equation simplifies to F2 equal to the combined mass (5000 kg) times the acceleration.
For the last two cars, combining their equations results in F1 minus F3 equal to 3000 times the acceleration.
When all three cars are considered together, the equation simplifies to F1 equal to the total mass (6000 kg) times the acceleration.
The tension forces F1, F2, and F3 can be calculated using the given acceleration and the total mass of the system.
F1 is found to be 12,000 Newtons, F2 is 10,000 Newtons, and F3 is 6,000 Newtons.
Newton's third law is discussed, stating that forces between two objects are equal in magnitude and opposite in direction.
The tension forces between the cars represent an action-reaction pair according to Newton's third law.
The video provides a visual representation of the forces acting on the train cars and how they relate to Newton's laws.
A cheat sheet for mechanics is presented, covering simple blocks on a horizontal surface and their calculations for acceleration and tension.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: