Derivative of inverse cosine | Taking derivatives | Differential Calculus | Khan Academy

Khan Academy
30 Apr 201403:43
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a mathematical proof for the derivative of the inverse cosine function. It guides viewers to derive the expression -1/√(1-x^2) by setting up an equation where y equals the inverse cosine of x, differentiating both sides with respect to x, and then substituting x with cosine y. The script emphasizes the relationship between the derivatives of inverse sine and inverse cosine, highlighting that they are similar except for the sign difference.

Takeaways
  • 📚 The script demonstrates the process of finding the derivative of the inverse cosine of x, paralleling the approach used for the inverse sine of x in a previous video.
  • 📈 The initial step involves setting y equal to the inverse cosine of x, and then expressing x as the cosine of y.
  • 🔨 Deriving both sides with respect to x, the script shows that the derivative of cosine y with respect to x is -sin(y) dy/dx.
  • 🤷 To express the derivative in terms of x, the script utilizes the trigonometric Pythagorean identity, where cosine squared y plus sine squared y equals 1.
  • 📝 It is derived that sine of y equals the square root of 1 minus cosine squared of y, leading to the derivative expression in terms of x.
  • ✔️ The final expression for the derivative of the inverse cosine of x is negative 1 over the square root of 1 minus x squared.
  • 📗 This result is directly compared to the previously found derivative of the inverse sine of x, highlighting the only difference is the sign in front of the fraction.
  • 🚨 Emphasizes the importance of understanding and applying the Pythagorean trigonometric identity to transform the derivative from a function of y to a function of x.
  • 📓 The script provides a step-by-step methodical approach to solving derivatives of inverse trigonometric functions, enhancing comprehension through repetition of similar steps.
  • ℹ️ Highlights the neat and systematic nature of derivatives for inverse trigonometric functions, showcasing the subtle but important differences between them.
Q & A

  • What is the derivative of the inverse cosine of x?

    -The derivative of the inverse cosine of x is equal to -1 over the square root of 1 minus x squared.

  • How is the derivative of the inverse cosine of x derived?

    -The derivative is derived by setting y equal to the inverse cosine of x, expressing x as the cosine of y, differentiating both sides with respect to x, and using the Pythagorean trigonometric identity to express the derivative in terms of x.

  • What trigonometric identity is used in the derivation of the derivative of the inverse cosine of x?

    -The Pythagorean trigonometric identity, which states that cosine squared of y plus sine squared of y equals 1, is used in the derivation.

  • Why is the derivative expressed in terms of x rather than y?

    -The derivative is expressed in terms of x to make it more applicable and understandable since the original function is in terms of x.

  • What does setting y equal to the inverse cosine of x imply about the relationship between x and y?

    -Setting y equal to the inverse cosine of x implies that x is the cosine of y, establishing a functional relationship between x and y.

  • What is the significance of the negative sign in the derivative of the inverse cosine of x?

    -The negative sign indicates the direction of the slope of the tangent line to the curve of the inverse cosine function at any given point x.

  • How does the derivative of the inverse cosine of x compare to the derivative of the inverse sine of x?

    -The derivative of the inverse cosine of x is negative 1 over the square root of 1 minus x squared, while the derivative of the inverse sine of x is positive 1 over the same denominator, indicating that the only difference is the sign.

  • What mathematical manipulation is used to express the derivative in terms of x?

    -A manipulation of the Pythagorean trigonometric identity is used to express sine of y in terms of cosine of y, which is then expressed as x, allowing the derivative to be expressed in terms of x.

  • Why is it useful to compare the derivatives of the inverse sine and cosine functions?

    -Comparing these derivatives helps highlight the structural similarities and differences between these two inverse trigonometric functions, enhancing understanding of their behavior and relationship.

  • What is the mathematical representation of the derivative of the inverse cosine of x?

    -The mathematical representation is ( -/(dx) (\cos^-1(x)) = -/(1/\sqrt(1 - x^2)) ).

Outlines
00:00
📚 Derivative of Inverse Cosine Function

This paragraph introduces the task of deriving the derivative of the inverse cosine function with respect to x. It encourages viewers to pause the video and attempt the proof themselves before proceeding. The voiceover explains the goal of finding the derivative of the inverse cosine of x and guides through the process of setting up the equation with y as the inverse cosine of x. The paragraph details the steps of differentiation, including taking the derivative of both sides with respect to x, and simplifying the expression to obtain the derivative in terms of y. It then proceeds to convert the derivative from being in terms of y to x, using the Pythagorean trigonometric identity. The final result is the derivative of the inverse cosine function, which is negative one over the square root of one minus x squared, and this result is compared with the derivative of the inverse sine function, highlighting the difference in sign.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, the derivative of the inverse sine and inverse cosine functions are being explored. The video aims to prove these derivatives, which are essential for understanding the behavior of inverse trigonometric functions.
💡inverse sine
Inverse sine, also known as arcsin, is a trigonometric function that gives the angle whose sine is a given number. The video focuses on finding the derivative of the inverse sine function, which is a process of determining how this function changes as its input variable 'x' changes. This is crucial for analyzing the rate of change and the slope of the tangent line to the curve at any point.
💡inverse cosine
Inverse cosine, similar to inverse sine, is a trigonometric function that returns the angle whose cosine is a given number. In the video, the main goal is to derive the formula for the derivative of the inverse cosine function, which is analogous to the process followed for the inverse sine function but with a different sign in the resulting formula.
💡proof
A proof in mathematics is a logical argument that demonstrates the truth of a statement. In the video, the proof is the process of showing that the derivative of the inverse sine of x is 1 over the square root of 1 minus x squared, and similarly for the inverse cosine. The proof involves algebraic manipulation and the application of calculus rules.
💡square root
The square root of a number is a value that, when multiplied by itself, gives the original number. In the context of the video, the square root is used in the denominator of the derivatives of inverse sine and inverse cosine functions, indicating the importance of dealing with non-negative values and the geometric interpretation of these functions.
💡Pythagorean identity
The Pythagorean identity is a fundamental trigonometric identity stating that the square of the sine of an angle plus the square of the cosine of the same angle is equal to 1. This identity is crucial in the video as it is used to express the sine term in terms of the cosine, which is then used to express the derivative of the inverse cosine in terms of x.
💡algebraic manipulation
Algebraic manipulation refers to the process of transforming and rearranging mathematical expressions using algebraic rules and operations. In the video, algebraic manipulation is extensively used to derive the formulas for the derivatives of the inverse sine and inverse cosine functions by transforming the expressions involving 'y' into expressions involving 'x'.
💡trigonometric functions
Trigonometric functions are mathematical functions that relate angles to the ratios of the sides of a right triangle. In the video, sine and cosine functions are used to define the inverse trigonometric functions, which are the focus of the derivative calculations being discussed.
💡rate of change
The rate of change, or the derivative, of a function at a particular point describes how quickly the function's output changes as the input changes. In the video, the derivatives of the inverse sine and inverse cosine functions are calculated to understand the rate at which these inverse trigonometric functions change with respect to their input variable 'x'.
💡sign
In the context of the video, the term 'sign' refers to the positive or negative nature of a mathematical expression. The sign of the derivative of the inverse cosine function is negative, whereas the sign of the derivative of the inverse sine function is positive. This difference in sign is crucial for understanding the behavior of these functions and their graphical representations.
💡slope of the tangent line
The slope of the tangent line to a curve at a particular point is a measure of how steep the curve is at that point. In the video, the derivatives of the inverse sine and inverse cosine functions represent the slopes of their tangent lines, which provide insight into the rate at which these functions increase or decrease on their graphs.
Highlights

The video aims to prove the derivative of the inverse cosine of x.

The viewer is encouraged to attempt the proof themselves before watching the solution.

The derivative of the inverse sine of x is mentioned as a reference, being 1 over the square root of 1 minus x squared.

The process starts by setting y equal to the inverse cosine of x, which is equivalent to x being the cosine of y.

Differentiating both sides with respect to x yields a 1 on the left and the derivative of cosine y with respect to y on the right.

The derivative is expressed as negative 1 over sine of y, multiplied by the derivative of y with respect to x.

The goal is to express the derivative in terms of x, not y.

Using the Pythagorean identity, sine of y is expressed as the square root of 1 minus cosine squared of y.

The derivative with respect to x of the inverse cosine of x is found to be negative 1 over the square root of 1 minus x squared.

The result is compared with the derivative of the inverse sine function, highlighting the sign difference.

The process demonstrates the relationship between the derivatives of inverse sine and inverse cosine functions.

The video emphasizes the practicality and theoretical importance of understanding these derivatives.

The method used is a combination of algebraic manipulation and trigonometric identities.

The video serves as an educational resource for those learning about derivatives and inverse trigonometric functions.

The proof showcases the step-by-step process of deriving the formula, which is beneficial for a clear understanding.

The video's approach to teaching is interactive, encouraging viewer participation in the problem-solving process.

The content is relevant for individuals studying calculus or higher-level mathematics.

The video provides a clear and concise explanation, making complex mathematical concepts more accessible.

Transcripts

Browse More Related Video

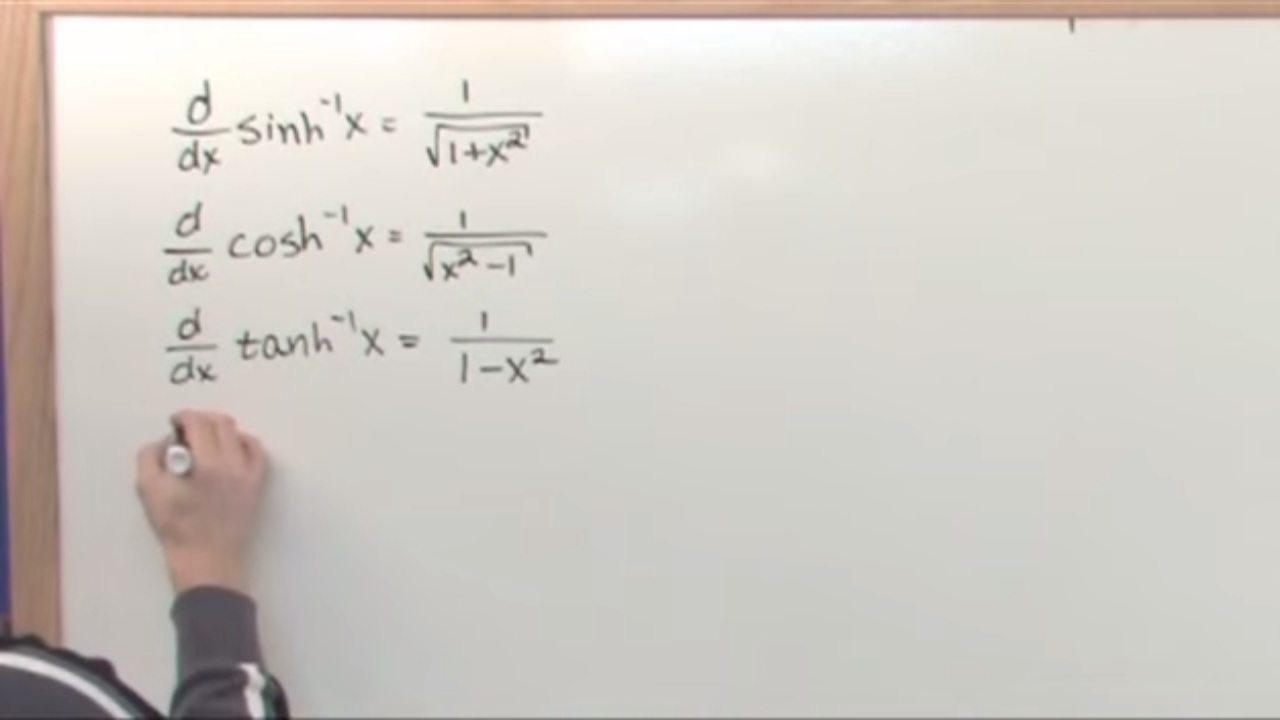
Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

Derivative of Inverse Trig Functions via Implicit Differentiation

Function inverses example 2 | Functions and their graphs | Algebra II | Khan Academy

Function inverse example 1 | Functions and their graphs | Algebra II | Khan Academy

Function inverses example 3 | Functions and their graphs | Algebra II | Khan Academy

Derivative of Inverse Functions | Calculus 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativeCalculusInverseTrigonometricMathematicalProofTrigonometryPythagoreanIdentityEducationalContentMathTutorialsOnlineLearningAcademicMathematics