Limit of sin(x)/x as x approaches 0 | Derivative rules | AP Calculus AB | Khan Academy

Khan Academy
24 Jul 201709:15
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a geometric proof demonstrating that the limit of the ratio of sine of theta to theta as theta approaches zero equals one. Utilizing a unit circle and trigonometric concepts, the instructor constructs a series of triangles to compare areas and establish inequalities. By applying the squeeze theorem, the proof shows that the limit converges to one, offering a clear understanding of this fundamental limit in calculus.

Takeaways
  • πŸ“ The video begins with an introduction to a proof involving a limit of a trigonometric function as theta approaches zero.
  • πŸ”΄ A unit circle is used as a reference for the trigonometric construction in the proof.
  • πŸ“ˆ The length of the salmon-colored line represents the sine of theta, with its absolute value considered for all quadrants.
  • πŸ”΅ The blue line represents the tangent of theta, also using its absolute value for consistency across quadrants.
  • β–³ The areas of the triangles formed within the unit circle are used to establish inequalities between trigonometric functions.
  • πŸ₯³ The area of the shaded triangle is expressed as half the product of its base (1) and its height (the absolute value of sine of theta).
  • πŸ’› The area of the yellow-highlighted wedge is a fraction (theta/2Ο€) of the entire circle's area, which is pi times the radius squared.
  • πŸ”Ž A comparison of the areas of the triangles helps to establish that the area of the salmon triangle is less than or equal to the area of the wedge, which is less than or equal to the area of the blue triangle.
  • πŸ“Œ Inequalities are set up to compare the absolute value of sine of theta to the absolute value of theta and then to the absolute value of tangent of theta over the absolute value of cosine of theta.
  • πŸ”„ By dividing through by the absolute value of sine of theta, the inequalities are transformed, leading to the reciprocals being compared.
  • πŸŽ‰ The squeeze theorem is applied to conclude that the limit of sine of theta over theta as theta approaches zero is equal to one.
Q & A

  • What is the main goal of the video?

    -The main goal of the video is to prove that the limit, as theta approaches zero, of sine of theta over theta is equal to one.

  • How does the instructor begin the proof?

    -The instructor begins the proof by using a geometric or trigonometric construction involving a unit circle and explaining the trigonometric functions related to the circle.

  • What does the salmon-colored line in the construction represent?

    -The salmon-colored line represents the length of the y-coordinate where the radius intersects the unit circle, which is equal to the sine of theta.

  • Why does the instructor use the absolute value for the sine and tangent functions?

    -The instructor uses the absolute value to ensure that the functions work for thetas in all quadrants, particularly the first and fourth quadrants, which are relevant for the proof.

  • How does the instructor express the area of the shaded triangle?

    -The instructor expresses the area of the shaded triangle as 1/2 times the base (which is one) times the height (the absolute value of the sine of theta), resulting in the absolute value of the sine of theta over two.

  • What is the relationship between the areas of the pink or salmon-colored triangle, the wedge, and the blue triangle?

    -The area of the salmon-colored triangle is less than or equal to the area of the wedge, and the area of the wedge is less than or equal to the area of the blue triangle.

  • How does the instructor use inequalities to compare the areas?

    -The instructor sets up inequalities comparing the absolute value of sine of theta to the absolute value of theta and the absolute value of tangent of theta, which are then manipulated algebraically to prove the desired limit.

  • What is the significance of the reciprocal of the functions in the proof?

    -The reciprocal of the functions is used to switch the inequalities, which is crucial for applying the squeeze theorem to find the limit as theta approaches zero.

  • How does the squeeze theorem help in this proof?

    -The squeeze theorem allows the instructor to conclude that since the value is bounded between two other values that approach the same limit, the limit of the function itself must also be equal to that limit.

  • What is the final result of the limit as theta approaches zero?

    -The final result of the limit as theta approaches zero is that the value is equal to one, which is what the proof aimed to demonstrate.

  • Why is the limit of the cosine function over theta as theta approaches zero equal to one?

    -The limit of the cosine function over theta as theta approaches zero is equal to one because the cosine of zero is one, and cosine is a continuous function.

Outlines
00:00
πŸ“ Geometric Proof of a Limit: Introduction

The instructor begins by outlining the goal of the video, which is to prove that the limit of the ratio of the sine of theta to theta, as theta approaches zero, equals one. To start, the instructor introduces a geometric or trigonometric construction involving a unit circle and explains the significance of the lengths of certain lines in relation to trigonometric functions. The explanation includes a discussion of how the absolute value of sine can be used to account for angles in the fourth quadrant and how to express the tangent of theta using the sides of a triangle inscribed in the unit circle.

05:01
πŸ“Š Area Comparison and Inequality Analysis

The instructor continues by constructing a series of triangles within the unit circle and using them to explore the relationship between the areas of these shapes. The focus is on expressing the areas in terms of trigonometric functions and setting up inequalities to compare the areas of different triangles. The assistant then manipulates these inequalities algebraically, dividing by the absolute value of sine theta and taking reciprocals to switch the direction of the inequalities. The process leads to a clearer understanding of the relationship between the sine, tangent, and cosine of theta, ultimately setting the stage for the application of the squeeze theorem to find the limit as theta approaches zero.

Mindmap
Keywords
πŸ’‘limit
In the context of the video, 'limit' refers to a mathematical concept that describes the behavior of a function when the input (theta in this case) approaches a certain value, which is zero in this case. The video aims to prove that the limit of the ratio of the sine of theta to theta as theta approaches zero is equal to one. This is a fundamental concept in calculus and is used to understand the behavior of functions and their derivatives at certain points.
πŸ’‘theta
Theta is used in the video to represent an angle in trigonometric functions. It is the variable that is approaching zero, and the behavior of functions involving theta is the focus of the video. Theta is a common symbol used in mathematics and physics to denote angles, and in this particular context, it is central to the demonstration of the limit of a trigonometric function.
πŸ’‘sine
The sine function is one of the fundamental trigonometric functions. In the video, the sine of theta is used to represent the y-coordinate of a point on the unit circle corresponding to an angle theta. The length of a line segment in the geometric construction is given by the sine of theta, which is crucial in the proof of the limit as theta approaches zero.
πŸ’‘tangent
The tangent function is another key trigonometric function, defined as the ratio of the opposite side to the adjacent side in a right-angled triangle. In the video, the tangent of theta is used to represent the ratio of the height to the base of a triangle in the unit circle, and it is crucial in the comparison of areas and the proof involving limits.
πŸ’‘unit circle
A unit circle is a circle with a radius of one. In the video, the unit circle is used as a reference for defining trigonometric functions and constructing geometric representations. The properties of the unit circle are essential for understanding the relationships between angles and their corresponding trigonometric function values.
πŸ’‘absolute value
The absolute value of a number is its non-negative value, regardless of its sign. In the video, absolute value is used to ensure that the trigonometric functions sine and tangent yield positive values, which is necessary for the geometric construction and the proof involving limits.
πŸ’‘area
In the context of the video, area refers to the amount of space inside a geometric shape, such as a triangle. The calculation of area is crucial in the proof, as it helps to establish relationships between the sizes of different triangles and the wedge formed within the unit circle.
πŸ’‘quadrant
In mathematics, a quadrant is one of the four regions formed by the intersection of the positive and negative axes on a coordinate system. In the video, the first and fourth quadrants are specifically mentioned as the regions where the proof is valid, as they are relevant to the behavior of the sine and tangent functions.
πŸ’‘inequalities
Inequalities are mathematical statements that compare the size of two expressions or values. In the video, inequalities are used to establish the relationships between the areas of different geometric shapes and to prove the limit of a function as theta approaches zero.
πŸ’‘reciprocal
The reciprocal of a number is the value that, when multiplied by the original number, results in a product of one. In the video, taking the reciprocal of certain expressions is part of the process to switch the inequalities and ultimately prove the limit of the sine function.
πŸ’‘squeeze theorem
The squeeze theorem, also known as the sandwich theorem, is a method in calculus that allows one to determine the limit of a function by 'squeezing' it between two other functions with known limits. In the video, this theorem is used to conclude that the limit of sine of theta over theta as theta approaches zero is equal to one, based on the established inequalities.
Highlights

The video aims to prove that the limit of sine of theta over theta as theta approaches zero is equal to one.

A geometric or trigonometric construction is used, involving a unit circle and trigonometric functions.

The length of the salmon-colored line represents the sine of theta, which is the y-coordinate of the radius intersecting the unit circle.

The blue line's length is expressed in terms of the tangent of theta, which is the opposite over adjacent side of the triangle formed.

The area of the shaded triangle is represented as half the product of its base (one) and its height (the absolute value of the sine of theta).

The area of the yellow-highlighted wedge is a fraction of the entire circle, with the fraction being theta over two pi radians.

The area of the larger blue triangle is calculated as half the product of its base (one) and its height (the absolute value of the tangent of theta).

The area of the salmon triangle is less than or equal to the area of the wedge, which is in turn less than or equal to the area of the blue triangle.

An inequality is established relating the absolute value of sine of theta, theta, and the absolute value of tangent of theta.

By dividing everything by the absolute value of sine of theta, the direction of the inequalities remains unchanged.

Taking the reciprocal of the established inequality switches the inequalities, leading to a new set of inequalities.

In the first and fourth quadrants, sine of theta and theta have the same sign, making the absolute value signs unnecessary.

The cosine of theta does not require absolute value signs in the first or fourth quadrants, as the x-coordinate is not negative and cosine of theta is positive.

Three functions are set up for the interval of interest, using the squeeze theorem to find the limit.

The limit of sine of theta over theta as theta approaches zero is established to be equal to one.

The proof involves comparing areas of different shapes and using algebraic manipulation to derive the result.

The use of the unit circle and trigonometric functions demonstrates a practical application of these mathematical concepts.

The video provides a clear and detailed explanation of the mathematical process, making it accessible for learners.

Transcripts

Browse More Related Video

Proof: Limit of sinx/x as x approaches 0 with Squeeze Theorem | Calculus 1

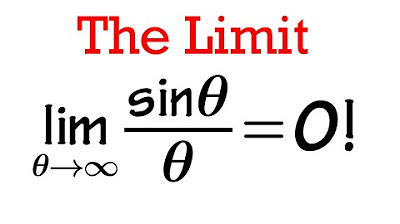
The Limit (do not use L'Hospital rule)

Proof: lim (sin x)/x | Limits | Differential Calculus | Khan Academy

Calculus 1: Finding Limits of Trigonometric Functions | Math with Professor V

Trig limit using pythagorean identity | Limits and continuity | AP Calculus AB | Khan Academy

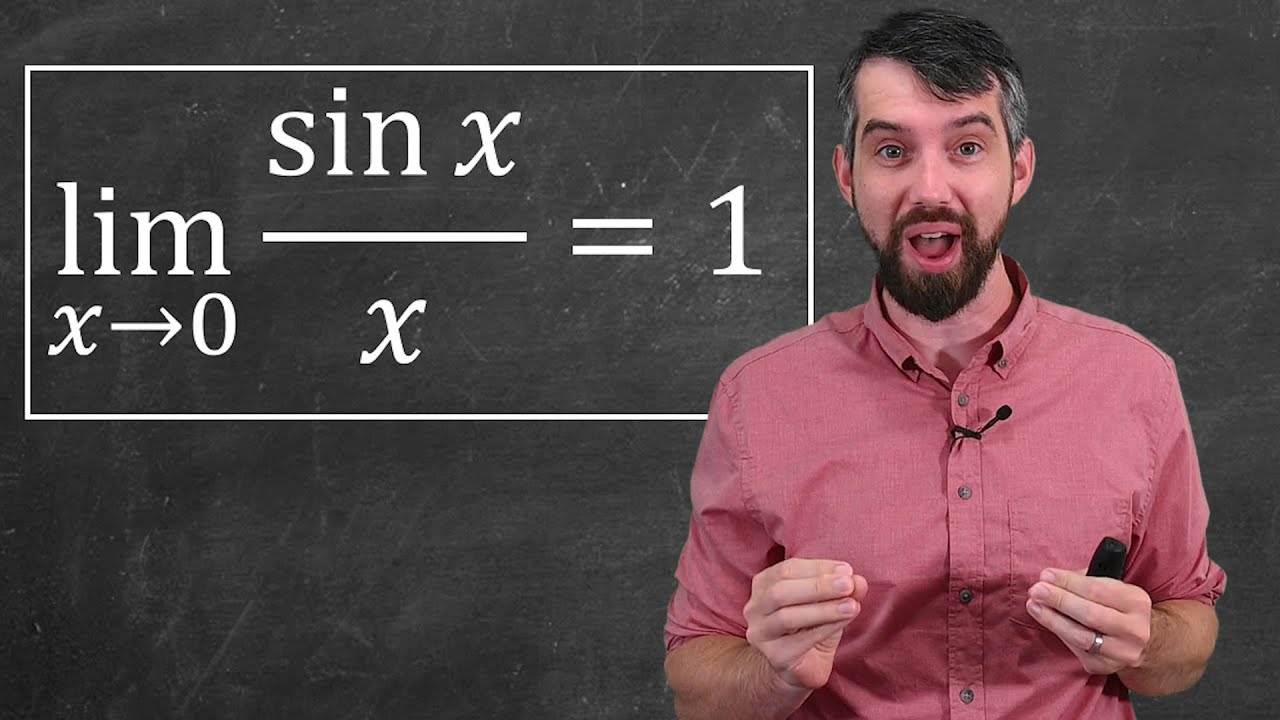
The most important limit in Calculus // Geometric Proof & Applications

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometryLimitProofSineThetaThetaApproachesZeroUnitCircleTrigonometricFunctionsMathematicsGeometryEducationalContentMathTutorial