Calculus 1: Finding Limits of Trigonometric Functions | Math with Professor V
TLDRIn this Math TV video, Professor V dives into the intricacies of finding limits of trigonometric functions. He begins by introducing two fundamental limit formulas: the limit of sine theta over theta as theta approaches zero, which equals one, and the limit of cosine theta minus one over theta, which also equals zero. Throughout the video, Professor V works through various examples, emphasizing the importance of manipulating expressions to match these formulas without resorting to L'Hôpital's rule, which is typically introduced later in a calculus course. He demonstrates how to rewrite tangent, cotangent, and other trigonometric functions in terms of sines and cosines, and how to handle indeterminate forms by using Pythagorean identities and direct substitution. The video is a comprehensive guide for students looking to master the art of evaluating limits in trigonometry.
Takeaways
- 📚 The first limit formula discussed is (lim_theta_to_0 (sin(theta)/theta) = 1), which requires the argument of the sine function to match the denominator and approach zero.
- 📚 The second limit formula is (lim_theta_to_0 (theta/sin(theta)) = 1), which is derived from taking the reciprocal of the first formula.
- 📚 A variation of the second formula is (lim_theta_to_0 (1 - cos(theta)/theta) = 0), which is useful when dealing with cosine in the numerator.
- 📚 L'Hôpital's rule is not used in these examples, and it's typically introduced later in calculus courses.
- 📚 When given a trigonometric function like tangent, secant, or cosecant, it's helpful to rewrite it in terms of sines and cosines.
- 📚 Factoring out common terms from the numerator and denominator can simplify limits and make them easier to evaluate.
- 📚 Direct substitution can sometimes be used to find limits if the function simplifies to a form where the limit can be directly calculated.
- 📚 Pythagorean identities can be useful when dealing with trigonometric functions, especially when stuck at an indeterminate form.
- 📚 When rewriting trigonometric functions, it's important to be careful with the signs, especially when dealing with negative terms.
- 📚 The process of evaluating limits often involves manipulating the expression into a form that matches known limit formulas.
- 📚 Clear and step-by-step work is essential for full credit on exams, avoiding shortcuts that skip necessary steps.
Q & A
What are the two main limit formulas for trigonometric functions discussed in the video?
-The two main limit formulas are: 1) as theta approaches zero, the limit of sine theta over theta is equal to one, and 2) as theta approaches zero, the limit of cosine theta minus one over theta is equal to zero.
Why is it important to match the argument of the sine function with the expression on the opposite side of the fraction in the first limit formula?
-It is important because the limit only holds true when both the argument and the expression on the opposite side approach zero simultaneously.
What is the reciprocal of the first limit formula when theta approaches zero?
-The reciprocal of the first limit formula is the limit as theta approaches zero of theta over sine theta, which is also equal to one.
How does the video suggest rewriting tangent and other trigonometric functions in terms of sines and cosines?
-The video suggests that for tangent, secant, cosecant, and cotangent, it is helpful to rewrite them in terms of sines and cosines to make it easier to apply the limit formulas.
What is the result of the first example where the limit as x approaches zero of sine of five x over three x is evaluated?
-The result of the first example is five thirds, which is obtained by manipulating the expression to match the limit formula and then applying it.
In the video, how is the limit of the reciprocal of zero approached?
-The video clarifies that the reciprocal of zero is not defined, hence the limit of the reciprocal as theta approaches zero does not have the same value as the original function.
What is the strategy used in the video to evaluate the limit as t approaches zero of tangent of six t over sine of two t?
-The strategy is to rewrite tangent in terms of sine and cosine, then manipulate the expression by multiplying by appropriate factors to match the limit formulas, and finally apply the limit formulas to find the result.
What is the final result of the limit as x approaches zero of sine of three x over five x cubed minus four x?
-The final result is negative three-fourths, obtained by factoring out x from the denominator, manipulating the expression, and applying the limit formulas.
How does the video approach the limit as theta approaches zero of sine theta over theta plus tan theta?
-The video approaches this by dividing everything by theta, which allows the use of the first limit formula, and then simplifying the expression to find the limit.
What is the result of the limit as theta approaches zero of sine theta over theta plus tan theta?
-The result is one half, obtained by applying the limit formulas and simplifying the expression after dividing by theta.
Why does the video advise against using the second limit formula in the example where theta approaches zero of cosine theta minus one over two theta squared?
-The video advises against it because the expression results in an indeterminate form of 0/0 as theta approaches zero, which means the second limit formula does not apply in this scenario.
What is the final result of the limit as x approaches pi over four of one minus tangent of x over sine of x minus cosine of x?
-The final result is negative root two (or negative 1 over root 2 if not rationalized), which is obtained by simplifying the expression and then applying direct substitution.
Outlines
📚 Introduction to Trigonometric Limits
This paragraph introduces the topic of finding limits of trigonometric functions. Two key limit formulas are presented: the first being the limit as theta approaches zero of sine theta over theta equals one, with the emphasis on matching the argument of the sine function with the expression in the denominator. The second formula is the limit as theta approaches zero of cosine theta minus one over theta equals zero, with a variation provided. The video clarifies that L'Hôpital's rule will not be used for these initial exercises and focuses on foundational limit concepts.
🔍 Manipulating Trigonometric Expressions
The paragraph demonstrates how to manipulate trigonometric expressions to apply the limit formulas. It walks through an example involving the limit as x approaches zero of sine of five x over three x, showing how to adjust the expression to match the formula by multiplying both the numerator and denominator by the necessary factors. The result is obtained by applying the limit formula, yielding five thirds as the final answer.
🤔 Advanced Trigonometric Limit Examples
This section tackles more complex examples, starting with rewriting tangent functions in terms of sine and cosine. It then proceeds to factor out common terms and simplify expressions to apply the limit formulas. The paragraph emphasizes the importance of showing all steps for full credit and provides a step-by-step guide through the thought process of evaluating limits, including canceling out variables and focusing on constants.
📉 Limits with Cotangent and Cosecant Functions
The paragraph discusses how to approach limits involving cotangent and cosecant functions by rewriting them in terms of sine and cosine. It provides a detailed example involving the limit as x approaches zero of six x squared times cotangent of three x times cosecant of two. The solution involves factoring and breaking down the expression to match the known limit formulas, resulting in a final answer of one.
🚫 Avoiding Misuse of Limit Formulas
This paragraph cautions against the misuse of the second limit formula when dealing with expressions like cosine theta minus one over two theta squared. It explains why the formula does not apply due to the indeterminate form that arises and suggests using the Pythagorean identity to simplify the expression instead. The paragraph guides through the correct approach to obtain the limit, emphasizing the importance of sign management.
🧹 Simplifying Expressions for Direct Substitution
The final paragraph discusses a method for simplifying expressions to use direct substitution when finding limits. It provides an example involving the limit as x approaches pi over four, showing how to clear out the extra denominator and cancel terms to simplify the expression. The result is obtained by substituting the value of the trigonometric function at the limit point, yielding negative root two as the final answer.
Mindmap
Keywords
💡Limit
💡Trigonometric functions
💡Sine function
💡Cosine function
💡Tangent function
💡Direct substitution
💡Indeterminate form
💡Pythagorean identities
💡Factoring
💡L'Hôpital's Rule
💡Reciprocal
Highlights
Two key limit formulas are introduced for finding limits of trigonometric functions: lim(theta->0) [sin(theta)/theta] = 1 and lim(theta->0) [(cos(theta)-1)/theta] = 0.
The argument of the trig function (e.g. sin(theta)) must match the expression in the denominator for the limit formulas to apply.
Rewriting trig functions in terms of sines and cosines is often helpful for finding limits, especially for tangent, secant, cosecant, and cotangent.
Factoring out common terms from the numerator and denominator can simplify the expression and make it easier to apply limit formulas.
When dealing with limits involving x->0, factoring out an x from terms in the denominator can separate the x dependencies and simplify the limit.
Regrouping terms and canceling common factors can often simplify the expression and make the limit easier to evaluate.
Direct substitution can sometimes be used to find a limit if the function is well-behaved at the point of interest.
The Pythagorean identity sin^2(theta) + cos^2(theta) = 1 can be useful for rewriting expressions and simplifying limits.
When stuck, multiplying by the conjugate of an expression can help to eliminate cross terms and simplify the limit.
Care must be taken with signs, especially when applying identities or distributing negative signs.
Clearing out denominators by multiplying through by a common factor can sometimes simplify the expression and make the limit easier to evaluate.
Rewriting secant and cotangent in terms of sines and cosines can make limits easier to evaluate, as demonstrated in the example.
When faced with an indeterminate form, consider using algebraic manipulation or factoring to simplify the expression and avoid the indeterminate form.
Recognizing and canceling common factors in the numerator and denominator can greatly simplify the expression and make the limit easier to find.
In some cases, a limit can be evaluated by direct substitution once the expression has been simplified and the point of interest is well-behaved.
The video provides many step-by-step examples demonstrating how to apply these strategies to find limits of trigonometric functions.
The presenter emphasizes the importance of careful algebraic manipulation and simplification in finding limits, rather than relying solely on memorized formulas.
The video concludes with a reminder that sometimes direct substitution can be used to find a limit if the function is well-behaved at the point of interest.
Transcripts
Browse More Related Video

Trig limit using pythagorean identity | Limits and continuity | AP Calculus AB | Khan Academy
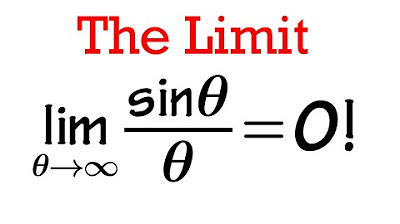
The Limit (do not use L'Hospital rule)
Chain Rule | MIT 18.01SC Single Variable Calculus, Fall 2010

Proof: Limit of sinx/x as x approaches 0 with Squeeze Theorem | Calculus 1
Limit of sin(x)/x as x approaches 0 | Derivative rules | AP Calculus AB | Khan Academy

How to Find Any Limit: Part 2 (NancyPi)
5.0 / 5 (0 votes)
Thanks for rating: