2011 Calculus AB free response #5b | AP Calculus AB | Khan Academy
TLDRThe video script discusses the process of finding the second derivative of a function W with respect to time t, and using it to determine the accuracy of an earlier estimate regarding the amount of solid waste in a landfill at a quarter of a year. The host calculates the second derivative and explains how its value indicates whether the initial slope-based estimate was an underestimate or overestimate. The conclusion is that the second derivative being positive implies an increasing slope and thus, an underestimate of the waste amount at t equals 1/4.
Takeaways
- 📈 The task involves finding the second derivative of a function W with respect to time (t).
- 🧠 The second derivative is used to analyze the rate of change in the slope of the function W.
- 🔄 The process starts by differentiating the given differential equation with respect to t.
- 📐 The first derivative of W was previously calculated and is used as a reference in this problem.
- 🔢 The second derivative in terms of W is derived using the chain rule and the given differential equation.
- 🌟 The second derivative is calculated as 1/625 times W(t) minus 300.
- 🕒 At time t equals 1/4, the goal is to determine if the initial estimate of solid waste is accurate.
- 📈 The slope of the function W is compared to the slope of the tangent line at t equals 0.
- 🚶♂️ If the function W's slope increases over time, the initial estimate is an underestimate.
- 🚶♀️ If the function W's slope decreases over time, the initial estimate is an overestimate.
- 📌 At t equals 0, the second derivative is found to be positive, indicating an increasing slope.
- 🏆 The conclusion is that the initial estimate in part a is an underestimate for the landfill's solid waste content at t equals 1/4.
Q & A
What is the main objective of the video?
-The main objective of the video is to find the second derivative of W with respect to time (t) and use it to determine whether the estimate of solid waste in a landfill at time t equals 1/4, made in part a, is an underestimate or overestimate.
How does the speaker begin the process?
-The speaker begins by stating readiness for the task, having prepared by wiping off salt and drinking water, symbolizing a transition from casual to focused work.
What is the first step in finding the second derivative?
-The first step is to take the derivative of both sides of the given differential equation with respect to time (t).
What does the speaker do after finding the second derivative in terms of the first derivative?
-The speaker then expresses the second derivative in terms of W, using the information provided in the problem.
How does the second derivative help in determining the accuracy of the estimate made in part a?
-The second derivative indicates whether the slope of the function W is increasing or decreasing over time. If it is positive, the slope is increasing, leading to an underestimate in part a. If it is negative or zero, the slope is decreasing or constant, which would indicate an overestimate or accurate estimate, respectively.
What does the speaker conclude about the second derivative at time t equals 1/4?
-The speaker concludes that the second derivative is positive at time t equals 1/4, indicating that the slope of the function W is increasing, and therefore, the estimate made in part a is an underestimate.
What is the significance of the second derivative being positive?
-A positive second derivative signifies that the rate of change of the function (in this case, the amount of solid waste in the landfill) is itself increasing. This means the initial estimate did not account for the increasing rate and thus was too low.
How does the speaker illustrate the concept of increasing and decreasing slopes?
-The speaker uses a graphical approach, drawing a random function to represent W and its slope at different points, to visually demonstrate how the slope of W could either increase or decrease over time.
What is the mathematical expression for the second derivative of W?
-The second derivative of W with respect to t is expressed as (1/625) * W(t) - 300, where W(t) represents the amount of waste at time t.
What is the value of the second derivative at time t equals 0?
-At time t equals 0, the second derivative is calculated as (1/625) * 1,400 - 300, which simplifies to 1.2, a positive number.
What does the positive second derivative at time 0 indicate about the landfill's waste amount at t equals 1/4?
-A positive second derivative at time 0 indicates that the amount of waste in the landfill is increasing at a rate that is itself increasing. This suggests that the landfill will contain more waste at time t equals 1/4 than what was estimated in part a.
Outlines
📚 Calculating the Second Derivative and its Implications
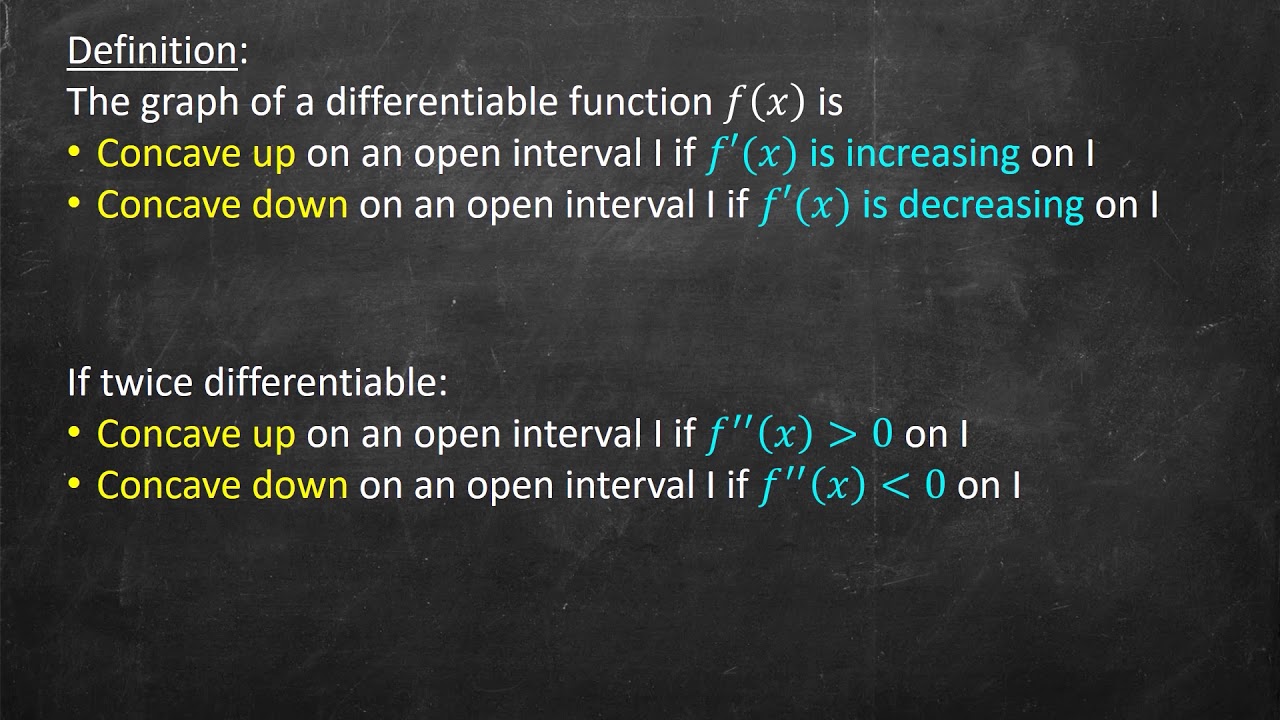
This paragraph delves into the mathematical process of finding the second derivative of a function W with respect to time t. The speaker begins by setting up the problem, ensuring that all previous calculations are in place. The first step is to differentiate both sides of the given differential equation with respect to t, leading to the second derivative of W in terms of t. The speaker then translates this second derivative into a function of W, using information provided in the problem statement. The goal is to use this second derivative to evaluate whether the initial answer to part a (the amount of solid waste in a landfill at time t equals 1/4) is an underestimate or overestimate. The speaker explains that by examining the sign of the second derivative, one can determine if the slope of the function W is increasing or decreasing over time, which in turn affects the accuracy of the initial estimate.
📈 Analyzing the Slope and Extrapolating the Waste Amount
In this paragraph, the speaker continues the mathematical analysis by focusing on the implications of the second derivative on the initial estimate of solid waste in a landfill at a quarter of a year. The speaker clarifies that if the second derivative is positive, it indicates that the slope of the function W is increasing, suggesting that the initial estimate from part a is an underestimate. The speaker uses visual examples to illustrate how different scenarios of slope change (increasing, constant, or decreasing) would affect the extrapolation of the waste amount. The speaker then calculates the actual second derivative at time t equals 0 and confirms that it is a positive number, leading to the conclusion that the initial estimate is indeed an underestimate of the actual waste amount in the landfill at t equals 1/4.
Mindmap
Keywords
💡second derivative
💡slope of the tangent line
💡solid waste
💡extrapolate
💡underestimate
💡overestimate
💡first derivative
💡differential equation
💡function W
💡time t equals 1/4
💡waste management
Highlights
The task involves finding the second derivative of W with respect to time, t.
The second derivative is used to assess the accuracy of an earlier estimate regarding solid waste in a landfill at a specific time.
The first step is to differentiate both sides of the given differential equation with respect to t to find the second derivative.
The second derivative expression is initially derived in terms of the first derivative, and then expressed in terms of W.
The second derivative calculation results in an expression involving W as a function of t, which is a function of time.
The second derivative is calculated to be 1/625 times W of t minus 300.
The slope of the function W is used to extrapolate the amount of solid waste in the landfill over time.
The accuracy of the estimate in part a depends on whether the slope of W is increasing or decreasing over time.
A positive second derivative indicates that the slope of W is increasing, leading to an underestimate in the extrapolation.
The second derivative at time 0 is calculated using the given initial condition and the derived formula.
The second derivative at time 0 is found to be a positive number, indicating an increasing slope for W.
The positive second derivative confirms that the estimate in part a is an underestimate of the actual amount of solid waste in the landfill at time t equals 1/4.
The process demonstrates the application of calculus in real-world scenarios, such as waste management and environmental studies.
The method illustrates the importance of higher-order derivatives in understanding the behavior of a function over time.
The analysis provides a clear explanation of how the rate of change of a function can affect predictions and estimates.
The transcript serves as an educational resource for understanding the practical applications of differential equations and their derivatives.
The problem-solving approach showcased can be applied to various scientific and engineering disciplines where dynamic systems are analyzed.
The discussion emphasizes the significance of initial conditions and constants in the context of differential equations.
The transcript highlights the role of mathematical modeling in making informed decisions and assessments in environmental management.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: