Second example of line integral of conservative vector field | Multivariable Calculus | Khan Academy
TLDRThe video script discusses a line integral calculation over a non-closed curve defined by the parameterization x=cos(t), y=sin(t) along the path from t=0 to t=π. It introduces the concept of a conservative vector field and its potential function, demonstrating how to find the potential function by equating the partial derivatives of the vector field. The integral is then evaluated by computing the potential function at the end points, yielding a result of -2/3, highlighting the path independence of conservative fields.
Takeaways
- 📌 The problem discussed involves a line integral over a curve C, which is not closed, unlike the previous example.
- 🔄 The curve C is parameterized by x = cos(t) and y = sin(t), with t ranging from 0 to π, representing a quarter of the unit circle.
- 🌀 The vector field in question is f(x, y) = (x^2 + y^2)i + (2xy)j, which is similar to the one in the previous problem but with a different integral setup.
- 🤔 The main goal is to determine if the field is conservative and if there exists a potential function F such that f = ∇F.
- 🧠 To verify if f is conservative, the partial derivatives with respect to x and y must be equal, which in this case, they are, confirming that the field is indeed conservative.
- 📈 The potential function F is found by integrating the components of f, resulting in F(x, y) = (1/3)x^3 + xy^2.
- 🏁 The line integral along the path is evaluated by computing F at the end points (x(π), y(π)) and (x(0), y(0)), and taking the difference.
- 📍 The specific values for x and y at t = 0 and t = π are (1, 0) and (-1, 0), respectively.
- 🧮 By evaluating F at these points, the result of the line integral is found to be -2/3.
- 🌐 The problem emphasizes the importance of understanding the conditions for a conservative vector field and how to find the potential function and line integral.
Q & A
What is the main difference between this problem and the one discussed in the previous video?
-The main difference is that this problem involves a line integral over a non-closed curve, whereas the previous one was about a closed line integral.
How is the curve C parameterized in this problem?
-The curve C is parameterized by x = cos(t) and y = sin(t), with t going from 0 to π, which represents a quarter of the unit circle in the x-y plane.
What is the vector field f in this problem?
-The vector field f is given by f(x, y) = (x^2 + y^2)i + 2xyj.
What does it mean for a vector field to be conservative?
-A vector field is conservative if it is the gradient of a scalar function, which implies that the line integral of the field is path-independent, depending only on the endpoints of the path.
How can we determine if the vector field f is conservative?
-To determine if f is conservative, we need to check if there exists a scalar function F such that f is the gradient of F. This involves comparing the partial derivatives of F with respect to x and y to the components of f.
What is the potential function F if the vector field f is conservative?
-The potential function F, if it exists, is given by F(x, y) = (1/3)x^3 + xy^2. This function is derived by integrating the components of the vector field f with respect to x and y.
How is the line integral of a conservative vector field calculated?
-For a conservative vector field, the line integral is calculated by evaluating the potential function F at the endpoints of the path and subtracting the values, i.e., F(x_b, y_b) - F(x_a, y_a).
What are the values of x and y at the endpoints for this problem?
-At t = 0, x = cos(0) = 1 and y = sin(0) = 0. At t = π, x = cos(π) = -1 and y = sin(π) = 0.
What is the result of the line integral in this problem?
-The result of the line integral is -2/3, which is obtained by evaluating the potential function F at the endpoints and subtracting the values.
Why is it not necessary to deal with the parameterization functions (cos(t) and sin(t)) when calculating the line integral of a conservative field?
-For a conservative field, the line integral is path-independent, so we don't need to deal with the parameterization functions. Instead, we only need to find the potential function and evaluate it at the endpoints to get the result.
How does the result of this problem illustrate the concept of path independence?
-The result of -2/3 for the line integral demonstrates path independence because the value of the integral does not depend on the specific curve taken between the endpoints, but only on the values of the potential function at those endpoints.
Outlines
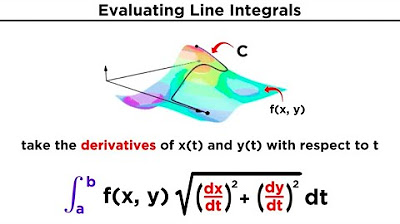
📚 Introduction to the Line Integral Problem
The paragraph introduces a new problem related to line integrals, with a focus on the integral over a curve c of the function x squared plus y squared dx plus 2xy dy. It contrasts this with a previous problem that involved a closed line integral, but emphasizes that the current curve c, parameterized by x equals cosine of t and y equals sine of t, is not closed. The discussion sets the stage for exploring whether the vector field is conservative and if there exists a potential function that could simplify the integral.
🔍 Analysis of the Vector Field and Path Independence
This paragraph delves into the properties of the vector field f, exploring whether it is conservative and if the line integral is path independent. It explains that although the loop is not closed, the integral can still be evaluated by examining if f is the gradient of a potential function F. The process of determining this involves comparing the partial derivatives of f with respect to x and y, leading to the identification of F as a function of x and y. The conclusion is that f is conservative, and the integral depends only on the initial and final points of the path.
📈 Calculation of the Line Integral
The final paragraph focuses on the calculation of the line integral by evaluating the potential function F at the endpoints of the curve c. It details the process of substituting the values of x and y at t equals 0 and pi into the function F, which is derived from the vector field f. The calculation yields the result of the line integral as minus 2/3, demonstrating the simplicity of evaluating the integral when the vector field is conservative and path independent.
Mindmap
Keywords
💡Line Integral
💡Curve
💡Parameterization
💡Vector Field
💡Conservative Vector Field
💡Gradient
💡Potential Function
💡Partial Derivative
💡Dot Product
💡Path Independence
💡Antiderivivative
Highlights
The problem involves a line integral over a curve defined by x = cos(t) and y = sin(t), with t ranging from 0 to pi.
The curve c is not a closed line integral, unlike the example in the previous video.
The vector field f is defined as f = (x^2 + y^2)i + 2xyj.
The integral can be rewritten as an integral of f dot dr along the curve c.
The question posed is whether the field f is conservative and has a potential function.
If f is conservative, the integral is path independent and equals F(pi) - F(0), where F is the potential function.
The potential function F can be found by integrating the components of f with respect to x and y.
The partial derivatives of f with respect to x and y must match the components of f for it to be conservative.
The potential function F is found to be F(x, y) = (1/3)x^3 + xy^2.
The line integral along the path is evaluated by finding f at the endpoints (x(pi), y(pi)) and (x(0), y(0)).
The value of x(pi) is -1, and x(0) is 1, while y(pi) and y(0) are both 0.
The line integral is calculated to be -2/3 by evaluating F at the end points and subtracting.
The process demonstrates the application of conservative vector fields and path independence in line integrals.
The problem showcases the importance of understanding the relationship between vector fields and their potential functions.
The example serves as a step-by-step guide on how to evaluate line integrals for non-closed paths.
The solution emphasizes the simplification of line integrals through the identification of conservative fields.
The method used can be applied to various problems involving line integrals and vector calculus.
The problem highlights the practical applications of theoretical concepts in calculus, particularly in understanding the behavior of vector fields.
Transcripts
Browse More Related Video

Closed curve line integrals of conservative vector fields | Multivariable Calculus | Khan Academy

Conservative Fields & Path Independence (Vector Fields)
Evaluating Line Integrals
Line integral example 1 | Line integrals and Green's theorem | Multivariable Calculus | Khan Academy

Example of closed line integral of conservative field | Multivariable Calculus | Khan Academy

Multivariable chain rule
5.0 / 5 (0 votes)
Thanks for rating: