Gradient of a scalar field | Multivariable Calculus | Khan Academy
TLDRThe video script discusses the concept of a gradient for a function of three variables, using the analogy of temperature distribution in a three-dimensional room to illustrate the idea. It explains how the temperature function衰减 as one moves away from the heat source at the center and describes the temperature function as 10 times e to the minus sum of squares of x, y, and z coordinates. The script then delves into calculating the gradient, which is represented as a vector field indicating the direction and magnitude of the largest increase in temperature. The gradient is computed by taking partial derivatives with respect to x, y, and z, resulting in a vector field that visually demonstrates how temperature changes in the space around the heat source.
Takeaways
- 📈 The concept of a scalar field is introduced, using temperature in a 3D room as an example where temperature is a function of x, y, and z coordinates.
- 🌡️ The temperature function is hypothetically defined as 10 times e to the minus r squared, with r being the radial distance from the heat source at the center of the room.
- 📊 The radial distance (r) is calculated using the Pythagorean theorem in three dimensions, represented as r squared equals x squared plus y squared plus z squared.
- ✏️ The gradient of a function of three variables is explained, which generates a vector field indicating the direction of the largest increase in temperature and the magnitude of temperature change.
- 🔄 The computation of the gradient involves taking partial derivatives with respect to x, y, and z, treating the other variables as constants during the process.
- 🎯 The gradient is represented as a vector field, transitioning from a scalar field, with each coordinate (i, j, k) corresponding to the partial derivatives and direction.
- 🌟 The gradient's magnitude is shown through the length of the vectors in the vector field, which corresponds to the rate of change (increase) in temperature.
- 🔍 Intuition is emphasized as crucial for understanding gradients, with the visualization of the vector field at different points in space providing insights.
- 🚀 As one gets closer to the heat source, the gradient vectors become larger, indicating a higher rate of temperature increase.
- 💡 The video script aims to clarify the conceptual understanding of gradients, emphasizing the transition from scalar fields to vector fields and the importance of the direction and magnitude of the gradient.
- 📚 The script concludes by highlighting the broad applicability of understanding gradients to various scalar fields, not limited to the temperature model discussed.
Q & A
What is the main concept discussed in the video?
-The main concept discussed in the video is the gradient of a function of three variables, using a scalar field as an example to explain the concept.
How does the video introduce the idea of a scalar field?
-The video introduces the idea of a scalar field by using the example of temperature distribution in a three-dimensional room, where temperature is a function of x, y, and z coordinates.
What is the temperature function proposed in the video?
-The temperature function proposed in the video is 10 times e to the minus the sum of squares of x, y, and z (r squared), which represents an exponential decay of temperature as one moves away from the heat source at the center of the room.
What is the significance of the radial distance in the temperature function?
-The radial distance in the temperature function represents how far one is from the heat source in the room. It is calculated using the Pythagorean theorem in three dimensions, which is the square root of the sum of the squares of x, y, and z.
How is the gradient of the temperature function represented?
-The gradient of the temperature function is represented as a vector field, where each vector at a point in space indicates the direction and magnitude of the largest increase in temperature at that point.
What is the formula for the gradient of T?
-The formula for the gradient of T is given by the sum of the partial derivatives of T with respect to x, y, and z, each multiplied by their respective unit vectors in the i, j, and k directions.
How are partial derivatives with respect to x, y, and z calculated in the context of the temperature function?
-Partial derivatives with respect to x, y, and z are calculated by treating the other two variables as constants and then differentiating the function with respect to the variable of interest.
What does the vector field of the gradient function look like?
-The vector field of the gradient function shows vectors at different points in space, where the length of the vectors represents the magnitude of the temperature increase, and the direction is given by the i, j, and k components.
How does the rate of temperature increase change as one gets closer to the heat source?
-As one gets closer to the heat source, the rate of temperature increase becomes larger, which is reflected in the vector field by vectors becoming bigger as they approach the center of the heat source.
What is the key takeaway from the video regarding gradients?
-The key takeaway from the video is that while the computation of gradients involves partial derivatives, the intuition behind them is crucial for understanding how they represent the direction and magnitude of the largest increase in a scalar field.
Outlines
📚 Introduction to Scalar Fields and Gradients
This paragraph introduces the concept of a three-dimensional scalar field, using the analogy of temperature distribution in a room. The speaker explains how the temperature can be described as a function of x, y, and z coordinates, and how it decays exponentially from a central heat source. The concept of the gradient is then introduced, explaining how it can be used to determine the direction and magnitude of the largest increase in temperature, effectively providing a three-dimensional slope. The speaker also discusses the mathematical process of computing the gradient, emphasizing the importance of understanding the underlying concepts rather than just the computations.
🔢 Calculating Partial Derivatives for Scalar Fields
In this paragraph, the speaker delves into the specifics of calculating the gradient of a scalar field by taking partial derivatives with respect to each variable. Using the temperature model from the previous paragraph, the speaker demonstrates how to compute the partial derivatives with respect to x, y, and z, treating the other variables as constants during each calculation. The process is explained step by step, highlighting the application of the chain rule and the properties of the exponential function. The speaker also emphasizes the importance of understanding the intuition behind the gradient, which is to identify the direction and rate of the fastest increase in the scalar field.
🌡️ Visualizing the Gradient of the Temperature Function
The final paragraph focuses on visualizing the gradient of the temperature function in a three-dimensional space. The speaker explains how the gradient at any point in space can be represented as a vector, with its components corresponding to the partial derivatives of the temperature function. The speaker then describes a vector field that represents the gradient at various points in space, illustrating how the magnitude and direction of the vectors change as one moves closer to the heat source. The visualization helps to provide an intuitive understanding of how the temperature increases most rapidly in the vicinity of the heat source, reinforcing the concept of the gradient as a vector field that indicates the direction and rate of change in a scalar field.
Mindmap
Keywords
💡three-dimensional surface
💡gradient
💡scalar field
💡vector field
💡partial derivative
💡temperature function
💡Pythagorean theorem
💡heat source
💡exponential decay
💡unit vector
💡intuition
Highlights
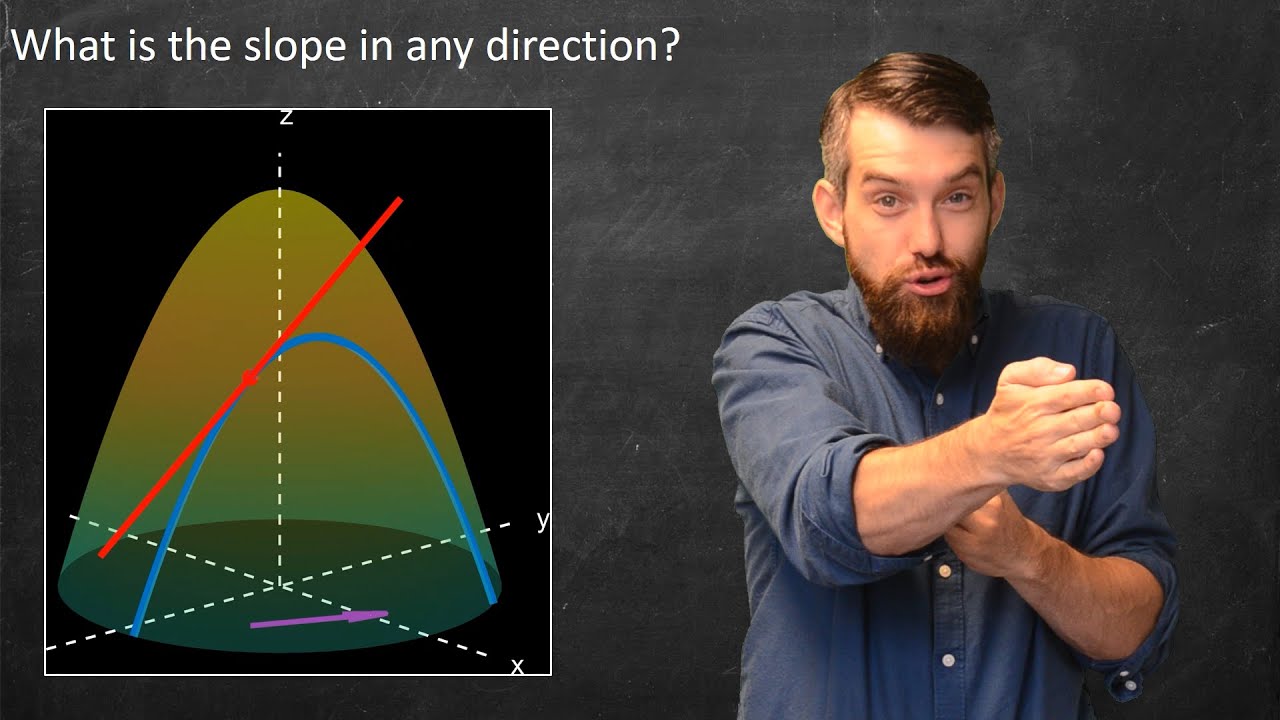
Introduction to the concept of a three-dimensional surface with height z as a function of x and y.
Exploration of the gradient of a function of three variables, using a scalar field as an example.
Intuitive explanation of a scalar field using the analogy of temperature distribution in a three-dimensional room.
The establishment of a hypothetical temperature function that decreases exponentially with distance from a heat source at the center of the room.
Use of the Pythagorean theorem to calculate the radial distance in three dimensions.
Definition and explanation of the gradient as a vector field that indicates the direction of the largest increase in temperature.
Description of how to compute the gradient by taking partial derivatives with respect to x, y, and z.
Explanation of the process for taking partial derivatives by treating other variables as constants.
Derivation of the gradient of the temperature function in terms of i, j, and k unit vectors.
Transition from scalar field to vector field by associating a vector with each point in space.
Visualization of the gradient vector field, showing how the magnitude and direction of vectors change in space.
Observation that closer proximity to the heat source results in a greater rate of temperature increase, reflected by larger vector magnitudes.
Emphasis on the importance of understanding the intuition behind gradients, beyond just the computation.
The temperature model as a practical application to help conceptualize gradients in scalar fields.
The video content serves as an educational tool for those learning about gradients and their applications in various fields.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: