Proof: d/dx(sqrt(x)) | Taking derivatives | Differential Calculus | Khan Academy

Khan Academy
4 May 200805:07
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a detailed proof of the derivative of the square root of x (x^(1/2)). It begins with the definition of a derivative and proceeds to calculate the limit as delta x approaches 0. The proof involves multiplying the numerator and denominator by the conjugate of the numerator and simplifying the expression. Ultimately, it is shown that the derivative of x^(1/2) is (1/2)x^(-1/2), which aligns with the general rule for derivatives of the form x^n, where the derivative is nx^(n-1). The explanation is clear, methodical, and provides a solid understanding of the concept.

Takeaways
  • 📌 The video is a walkthrough of the proof for the derivative of the square root of x function.
  • 🔍 The derivative is defined as the limit of the difference quotient as delta x approaches 0.
  • 🎨 The video uses different notations for the change in x, such as delta x and h, but focuses on using delta x.
  • 📈 To simplify the expression, the numerator and denominator are multiplied by the conjugate of the numerator.
  • 🌟 The conjugate multiplication helps to isolate the term with delta x, which is crucial for taking the limit as delta x approaches 0.
  • 📊 The cancellation of x terms in the numerator and denominator simplifies the expression further.
  • 🔢 By dividing both the numerator and the denominator by delta x, the expression is simplified to a form that allows taking the limit.
  • 🎓 The limit of the simplified expression as delta x approaches 0 results in 1 divided by the square root of x.
  • 🌐 The result aligns with the general property of derivatives for power functions, where the derivative of x to the power n is nx to the power n-1.
  • 📝 The proof is specific to the square root function (x to the 1/2 power) and demonstrates the derivative to be x to the -1/2 power.
  • 🚀 The video aims to clarify the concept and provide a foundation for understanding derivatives of similar functions.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the proof of the derivative of the square root of x.

  • How is the derivative defined in the context of this video?

    -The derivative is defined as the limit as delta x approaches 0 of the change in function (f(x + delta x) - f(x)) divided by the change in x (delta x).

  • What does the video mention about the different notations for 'delta x'?

    -The video mentions that some people use 'h' or 'd' to represent the change in x, but the speaker prefers using 'delta x'.

  • What is the purpose of multiplying the numerator and denominator by the conjugate of the numerator in the proof?

    -Multiplying by the conjugate simplifies the expression and helps to isolate the term that will allow the limit to be taken as delta x approaches 0.

  • What is the result of the simplification process in the numerator after canceling out the x terms?

    -After canceling out the x terms, the numerator is left with delta x terms only.

  • How does the video handle the limit as delta x approaches 0 in the final expression?

    -The video sets delta x equal to 0 since that is what it is approaching, and this allows the simplification to the final result.

  • What is the final result of the derivative of the square root of x?

    -The derivative of the square root of x is 1/2x to the negative 1/2, or (1/√x).

  • How does the result relate to the general property of derivatives of power functions?

    -The result is consistent with the general property that the derivative of x to the power n is nx to the power (n-1), even in this case where n is 1/2.

  • What is the significance of the proof presented in the video?

    -The proof is significant as it demonstrates the derivative of a square root function, which is a common type of function encountered in calculus.

  • How does the video conclude?

    -The video concludes by summarizing the proof and stating that the result is consistent with the general rule for derivatives of power functions.

  • What is the speaker's attitude towards the complexity of the proof?

    -The speaker hopes that the proof is not too complicated and that it is a satisfying explanation for the derivative of the square root of x.

Outlines
00:00
📚 Proof of the Derivative of the Square Root of x

This paragraph presents a step-by-step explanation of the proof for the derivative of the square root of x. It begins with an introduction to the concept of a derivative and its definition, emphasizing the limit as delta x approaches 0. The explanation then moves on to the manipulation of the function, including the use of the conjugate of the numerator to simplify the expression. The process involves expanding and simplifying the equation, leading to the cancellation of terms and the isolation of delta x in both the numerator and the denominator. The final step is to take the limit as delta x approaches 0, resulting in the derivative of x to the 1/2 power being 1/2x to the negative 1/2. The summary concludes by highlighting the consistency of this result with the general property of derivatives for power functions.

05:01
👋 Closing Remarks and Future Videos

The second paragraph is a brief conclusion to the video, where the speaker expresses their intention to continue exploring mathematical concepts in future videos. It serves as a closing remark, inviting viewers to look forward to additional educational content and discussions on similar topics.

Mindmap
Keywords
💡Derivative
The derivative is a fundamental concept in calculus that represents the rate of change or the slope of a function at a particular point. In the video, the derivative of the square root of x is being calculated, which is a specific application of finding how a function changes as its input varies. The process involves using the definition of a derivative, which is the limit of the ratio of the change in the function's value to the change in its input as the input approaches zero.
💡Square Root
A square root is a mathematical operation that finds a number which, when multiplied by itself, gives the original number, the radicand. In the context of the video, the square root of x (√x) is the function whose derivative is being determined. The square root function is an important concept in various mathematical and real-world applications, such as calculating areas and solving quadratic equations.
💡Limit
In mathematics, a limit is a value that a function or sequence approaches as the input (or index) approaches some value. The concept of a limit is crucial in the definition of a derivative, as it describes the behavior of a function near a specific point. In the video, the limit is used to define the derivative of the square root of x, illustrating the process of taking the limit as delta x approaches zero.
💡Conjugate
In the context of the video, the conjugate refers to a mathematical technique used to simplify expressions involving square roots and other radicals. The conjugate of an expression is obtained by changing the sign of the radicand in the square root. In the process of finding the derivative of the square root of x, the conjugate is used to rationalize the numerator, which simplifies the expression and allows for the calculation of the limit.
💡Delta x
Delta x, often denoted as 'h' or 'dx' in calculus, represents an infinitesimally small change in the independent variable 'x' of a function. It is used in the definition of a derivative and the concept of limits to analyze the behavior of functions as inputs change by very small amounts. In the video, delta x is used to define the change in x and is crucial in the process of finding the derivative of the square root of x.
💡Change in x
The change in x, often symbolized by delta x, refers to the difference in the value of the independent variable before and after a small, infinitesimally small alteration. In calculus, this concept is used to explore how a function's value changes in response to these tiny changes in its input. The video's main theme involves calculating the derivative of the square root of x, which directly depends on understanding how the function's value changes with respect to changes in x.
💡Numerator
The numerator is the top part of a fraction, which is the expression that is divided by the denominator to yield the value of the fraction. In the context of the video, the numerator is a critical component of the limit definition of the derivative, where the difference in the function's values (f(x + delta x) - f(x)) is divided by the change in x (delta x).
💡Denominator
The denominator is the bottom part of a fraction that, together with the numerator, determines the value of the fraction. In calculus, particularly when dealing with limits and derivatives, the denominator often represents the change in the independent variable or a similar quantity that influences the function's behavior. The denominator is essential in the definition of a derivative, as it is part of the ratio that describes the rate of change of the function.
💡Simplification
Simplification in mathematics refers to the process of making a complex expression or equation more straightforward or easier to understand. It often involves reducing the number of terms, combining like terms, or applying algebraic techniques to eliminate complexity. In the video, simplification is key to finding the derivative of the square root of x, as it allows the calculation of the limit that defines the derivative.
💡Rationalize
Rationalizing the denominator is a process in algebra where the denominator of a fraction is made 'rationally' equivalent to 1, or the denominator is simplified so that it contains no radicals or other complex numbers. In the context of the video, rationalization is used to simplify the expression involving square roots before taking the limit to find the derivative.
💡Limit Approaching Zero
The phrase 'limit approaching zero' refers to a situation in calculus where the input to a function or the change in the input (denoted as delta x) is considered to be getting infinitely close to zero. This concept is fundamental in the definition of a derivative, as it describes the behavior of a function as the change in its input becomes arbitrarily small. In the video, the limit as delta x approaches zero is used to find the derivative of the square root of x.
Highlights

The video presents a proof of the derivative of the square root of x function.

Derivative is defined as the limit of the change in y divided by the change in x as x approaches 0.

The function under consideration is the square root of x, denoted as f(x) = √x.

The change in x is represented by delta x, which is equivalent to h or d in other notations.

The expression for the derivative involves the difference quotient of f(x + delta x) and f(x).

To simplify the expression, the numerator and denominator are multiplied by the conjugate of the numerator.

The conjugate multiplication results in a difference of squares, which simplifies the expression.

After simplification, the expression contains terms with delta x, which will approach 0.

The limit is taken as delta x approaches 0, which allows for the simplification of the expression.

The final simplified form of the derivative is 1/(2*sqrt(x)), derived from the limit process.

The proof demonstrates the consistency of the result with the general property of derivatives of power functions.

The derivative of x to the power of 1/2 is 1/(2*sqrt(x)), which aligns with the derivative rule for x^n being nx^(n-1).

The video provides a clear and methodical approach to deriving the derivative of a square root function.

The proof is a good starting point for understanding the differentiation of more complex functions.

The process of simplifying the expression by multiplying conjugates is a key technique in proving derivatives.

The video is a valuable resource for those learning about calculus and the properties of derivatives.

Transcripts

Browse More Related Video

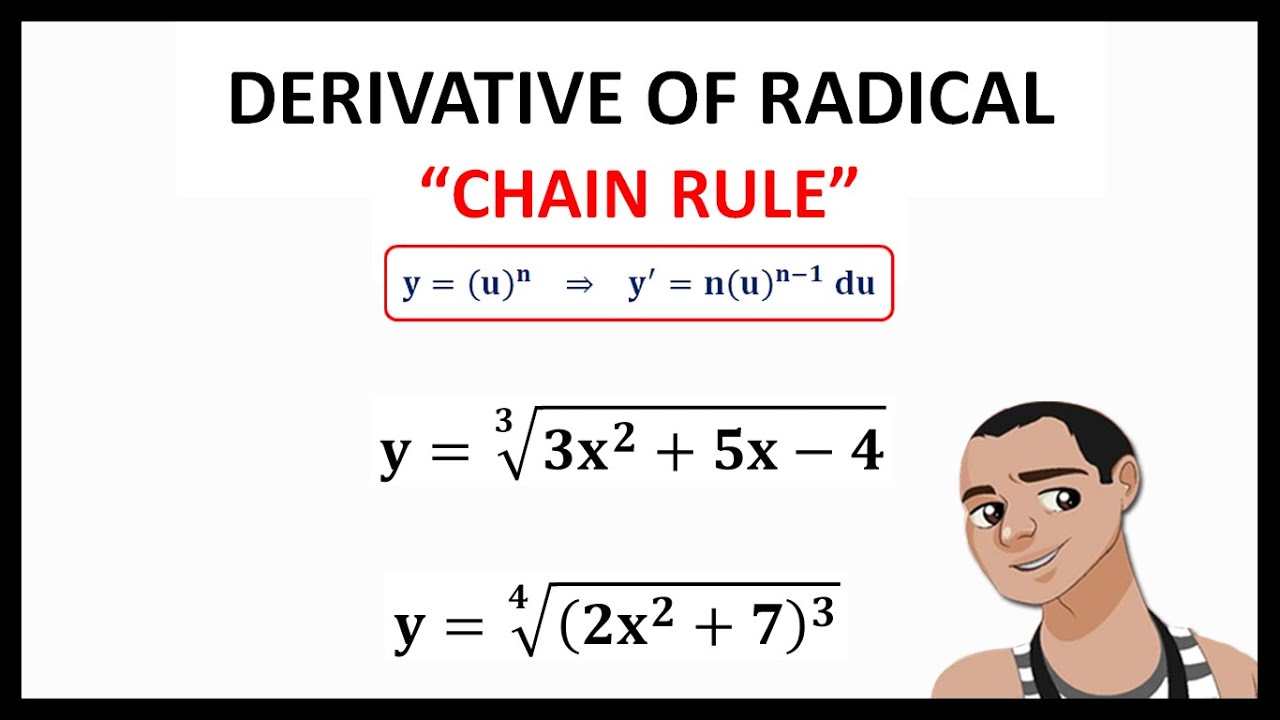
DERIVATIVE OF RADICAL: THE CHAIN RULE

Proof: d/dx(x^n) | Taking derivatives | Differential Calculus | Khan Academy

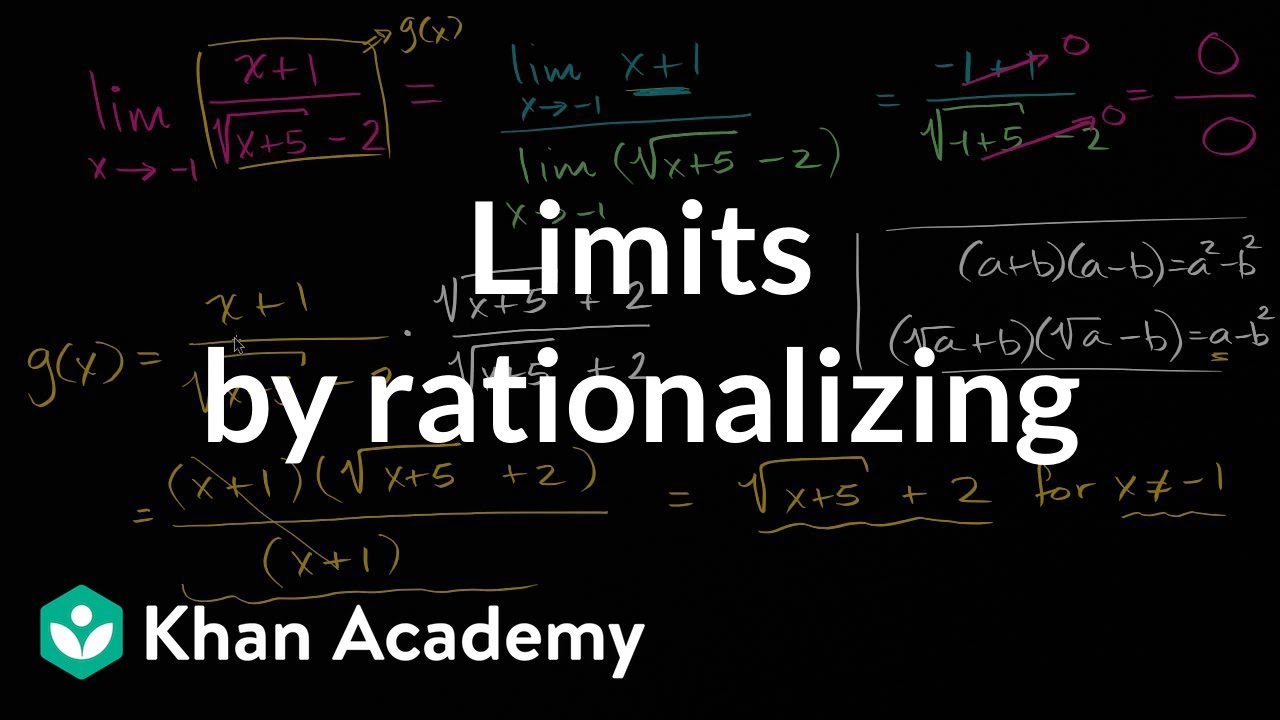
Limits by rationalizing | Limits and continuity | AP Calculus AB | Khan Academy

Definition of the Derivative | MIT 18.01SC Single Variable Calculus, Fall 2010

Applying the Definition of the Derivative to 1/x

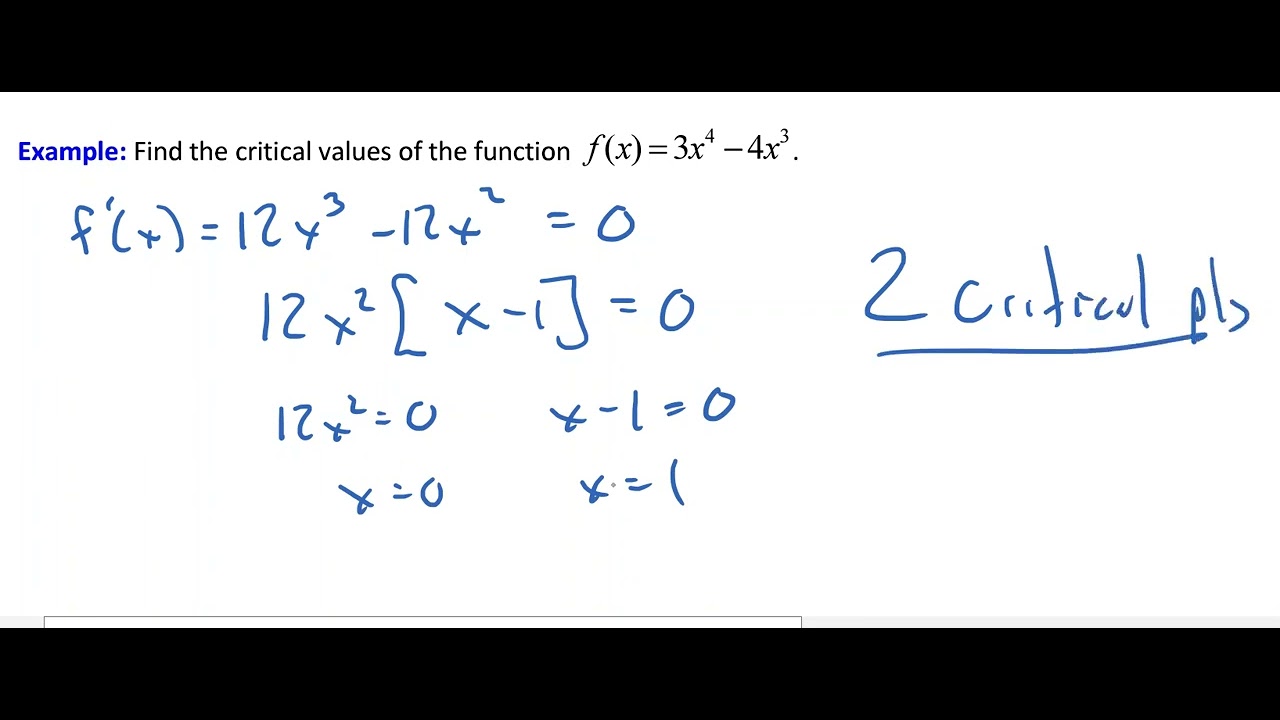
Finding Critical Values

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusDerivativeProofEducationalMath TutorialSquare RootLimitx to the power of 1/2