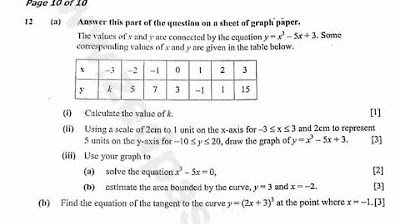
Integration (Calculus)
TLDRIn this educational video, the host, C Chamber Jacob, guides viewers through the process of solving two exam questions involving calculus, specifically integration. He emphasizes the importance of following simple rules, such as adjusting powers of variables and handling fractions, to arrive at the correct integrated forms. The host simplifies the expressions and reminds viewers to include the constant 'c' in their final answers unless specific limits are given. The video concludes with an invitation for viewers to subscribe and contact the host for online tuitions.
Takeaways
- 📝 Start by copying the given expression for integration.
- 🔍 Check the powers of the variable present in the expression.
- ✍️ Add 1 to the power of the variable when integrating.
- 📊 Simplify the expression by performing necessary arithmetic operations.
- 🔧 Integrate each term separately, maintaining the structure of the original expression.
- 📈 When integrating a fraction, use the laws of indices to rearrange if necessary.
- 🔄 Change the negative exponent to its reciprocal and adjust the expression accordingly.
- 🧩 Combine like terms and simplify the resulting integrated expression.
- 🔎 Include a constant 'C' in the final answer to represent the integral constant.
- 📌 Omit the constant 'C' only if there are specified limits of integration.
- 📝 The final integrated form should be a simplified expression with the variable's powers adjusted.
Q & A
What is the first step in solving the given integral problem?
-The first step is to copy the given expression without any changes.
What is the rule for dealing with powers in integration?
-When integrating a power, you should add 1 to the power of the variable you are integrating with.
How do you handle a term without a variable when integrating?
-If there's a term without a variable, you simply replace it with the variable you're expecting (in this case, x).
What is the purpose of adding 'plus C' in the result of an indefinite integral?
-The 'plus C' represents the constant of integration, which accounts for all possible values the function could have due to the indefinite nature of the integral.
When is it necessary to include the constant 'plus C' in your answer?
-You need to include 'plus C' in your answer for an indefinite integral. If there are limits (definite integral), you can avoid adding 'plus C'.
How does the speaker approach the second integral problem (part B)?
-The speaker first copies the expression, then uses the laws of indices to rearrange the terms to make the integration possible.
What is the significance of changing the form of the expression in part B of the integral?
-Changing the form is necessary to isolate the variable with its power on the top of the fraction, which is a requirement for integration.
How do you simplify the expression after integrating the second part of the problem?
-After integrating, you simplify the expression by combining like terms and performing the necessary arithmetic operations, such as division and addition.
What is the final result of the integral problem presented in the script?
-The final result is 3x^4/8 - 5x^2/2 + x^(-1) + C.
What advice does the speaker give at the end of the video?
-The speaker encourages viewers to subscribe to their YouTube channel and offers personal online tuitions for those interested.
How can viewers get in touch with the speaker for further assistance?
-Viewers can contact the speaker via WhatsApp using the number provided at the end of the video.
Outlines
📝 Calculating Exam Question A - Integration
This paragraph focuses on solving an integration problem referred to as 'question A'. The speaker, C Chamber Jacob, explains the process of integrating a given mathematical expression. He emphasizes the importance of checking the powers of the variable (in this case, 'x') and adjusting them according to the rules of integration. The speaker demonstrates how to handle different terms in the expression, including adding 1 to the power, simplifying the expression, and finally, incorporating the constant of integration (C). The explanation is detailed, walking through each step of the integration process.
🧠 Tackling Exam Question B - Fractions and Indices
In this paragraph, the speaker continues with the second part of the exam question, referred to as 'question B'. The focus here is on handling fractions and applying the laws of indices during the integration process. The speaker explains how to transform the given expression to make it integrable, particularly focusing on the term with 'x squared'. The explanation includes the steps to simplify the transformed expression, including dealing with fractions and negative powers. The speaker also reminds the audience to include the constant of integration (C) in the final answer. The summary ends with a call to action for viewers to subscribe to the YouTube channel and offer contact for online tuitions.
Mindmap
Keywords
💡Integration
💡Powers
💡Simplification
💡Constants
💡Fractions
💡Laws of Indices
💡Variables
💡Antiderivative
💡Limits
💡Online Tuitions
💡YouTube Channel
Highlights
Introduction to the video and the exam questions
Starting with exam question B and explaining the process of integration
Rule number one: Checking the powers of the letter in the expression
Adding 1 to the power of x in the expression
Integrating a number without a letter and placing the expected letter (x)
Simplifying the expression after integration
Integrating question A by following the same rules
Transforming the expression using the laws of indices
Placing x squared on top of the fraction
Integrating the transformed expression by adding 1 to the power
Dividing by the power and handling the fraction with the letter
Calculating the division and simplifying the fraction
Finalizing the integration and including the constant 'C'
Explanation of when to avoid putting 'C' in the integration
Completing the integration of question B and simplifying the result
Invitation to subscribe to the YouTube channel for more content
Offer for online tuitions and contact information
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: