Sensitivity and Specificity Explained Clearly (Biostatistics)

MedCram - Medical Lectures Explained CLEARLY
16 Mar 201412:15
EducationalLearning
32 Likes 10 Comments

TLDRThis MedCram lecture delves into the concepts of sensitivity and specificity in the context of medical testing and statistical analysis. Using the analogy of a car alarm system, it explains how adjusting the sensitivity can lead to more true positives (catching thieves) but also to false positives (reacting to motorcycles). Specificity is introduced as the true negative rate, emphasizing the importance of balancing sensitivity and specificity to minimize errors in medical diagnosis. The lecture aims to enhance understanding of these statistical measures and their practical implications in everyday medical decision-making.

Takeaways
  • πŸ” Sensitivity and specificity are crucial concepts in understanding and interpreting medical test results and statistics.
  • πŸš— The analogy of a car alarm system explains the trade-off between sensitivity (detecting true threats) and specificity (avoiding false alarms).
  • πŸ”Š High sensitivity in a test means a low chance of false negatives, but it may increase the likelihood of false positives.
  • 🚫 Low sensitivity may result in false negatives, which is like a car alarm failing to alert when a theft actually occurs.
  • βœ… High specificity means a low chance of false positives, ensuring that when a test is positive, it is very likely to be accurate.
  • πŸ” Setting the sensitivity of a test is context-dependent and should consider the environment and the consequences of false positives and negatives.
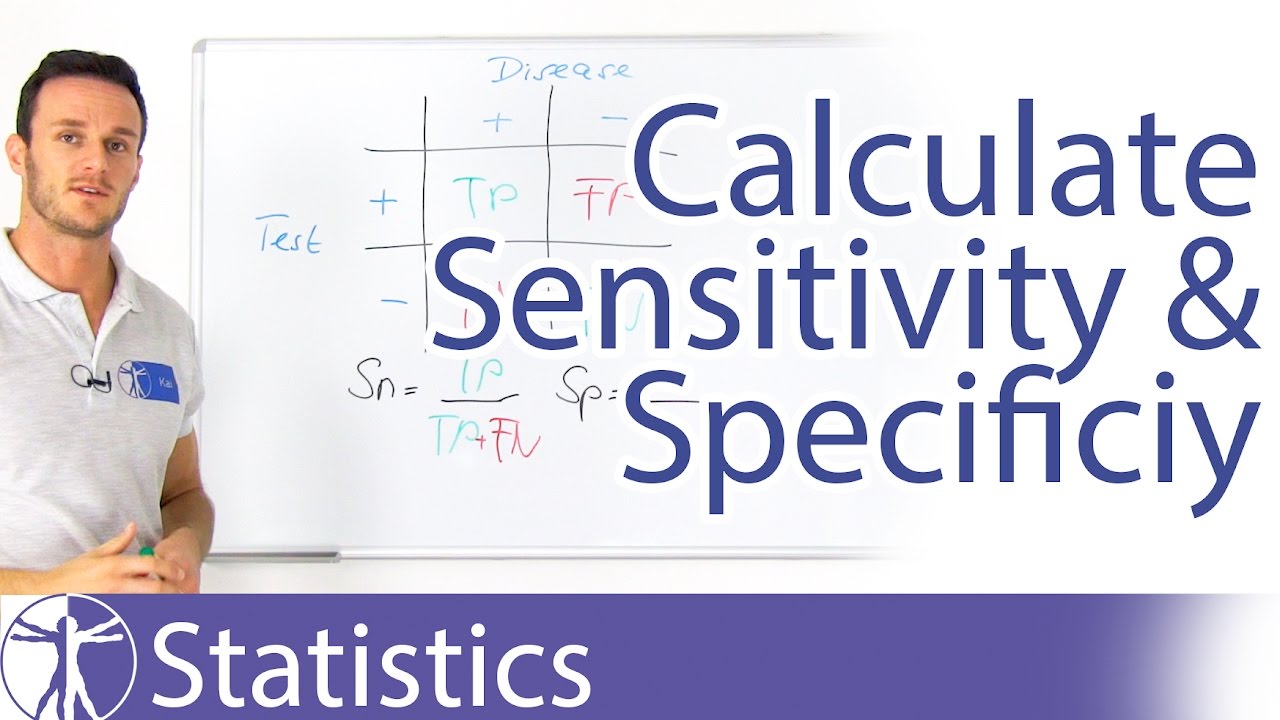
  • πŸ“ˆ Sensitivity is mathematically defined as the ratio of true positives to the sum of true positives and false negatives.
  • 🎯 Specificity is mathematically defined as the ratio of true negatives to the sum of true negatives and false positives.
  • πŸ€” The ideal test setting balances sensitivity and specificity based on the specific situation and the costs associated with false results.
  • πŸ“Š Understanding sensitivity and specificity is essential for healthcare professionals when ordering and interpreting diagnostic tests for patients.
  • πŸ“– Further lectures will delve into the mathematical calculations and practical applications of sensitivity and specificity in medical testing.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is sensitivity and specificity in relation to statistics and their importance in medical testing and interpretation of data.

  • How does the analogy of a car alarm relate to the concepts of sensitivity and specificity?

    -The car alarm analogy compares the sensitivity of a test to the car alarm's sensitivity. A highly sensitive alarm (or test) is likely to go off with both true threats (true positives) and benign events (false positives), while a specific setting avoids false positives but may miss true threats (false negatives).

  • What are the four possible outcomes when testing for a condition?

    -The four possible outcomes are true positive (correctly identified as having the condition), false positive (incorrectly identified as having the condition), false negative (incorrectly identified as not having the condition), and true negative (correctly identified as not having the condition).

  • How is sensitivity mathematically defined?

    -Sensitivity is mathematically defined as the number of true positives divided by the sum of true positives and false negatives.

  • How is specificity mathematically defined?

    -Specificity is mathematically defined as the number of true negatives divided by the sum of true negatives and false positives.

  • What is the trade-off between sensitivity and specificity?

    -The trade-off is that increasing sensitivity reduces the chance of false negatives but increases the chance of false positives, while increasing specificity reduces the chance of false positives but increases the chance of false negatives.

  • Why is it important to consider the setting of sensitivity and specificity based on the situation?

    -It is important because different situations may prioritize catching true threats (high sensitivity) over avoiding false alarms (high specificity), depending on the consequences and the context, such as the prevalence of the condition and the cost of false results.

  • What does a true positive result in the car alarm analogy represent?

    -A true positive in the car alarm analogy represents a situation where the alarm goes off because a thief is actually trying to break into the car.

  • What does a false negative result in the car alarm analogy represent?

    -A false negative in the car alarm analogy represents a situation where the alarm does not go off, indicating no threat, even though a thief is actually breaking into the car.

  • How can the concepts of sensitivity and specificity be applied in medicine?

    -In medicine, sensitivity and specificity are used to evaluate the performance of diagnostic tests. High sensitivity is desired to catch diseases that might be missed, while high specificity is needed to avoid misdiagnoses due to false positives.

  • What is the significance of understanding sensitivity and specificity in medical testing?

    -Understanding sensitivity and specificity is crucial for healthcare professionals to make informed decisions about diagnostic tests, treatment plans, and patient care, as it helps to interpret test results accurately and manage the balance between detecting true cases and avoiding unnecessary interventions.

Outlines
00:00
πŸ” Introduction to Sensitivity and Specificity

This paragraph introduces the concepts of sensitivity and specificity in the context of medical testing and statistics. It uses the analogy of a car alarm system to explain how these terms relate to true positive, false positive, false negative, and true negative results. The speaker emphasizes the importance of understanding these concepts for interpreting medical data and making informed decisions about patient care.

05:02
πŸ“ˆ Mathematical Explanation of Sensitivity and Specificity

The speaker delves into the mathematical definitions of sensitivity and specificity. Sensitivity is defined as the ratio of true positives to the sum of true positives and false negatives, while specificity is the ratio of true negatives to the sum of true negatives and false positives. The paragraph highlights the inverse relationship between sensitivity and specificity, and how adjusting the sensitivity of a test can lead to a trade-off between false positives and false negatives.

10:09
πŸš— Car Alarm Analogy for Sensitivity and Specificity

This paragraph continues the car alarm analogy to illustrate the practical implications of setting sensitivity and specificity thresholds. It explains how different settings can lead to various outcomes, such as catching all thieves (true positives) at the cost of increased false positives (e.g., alarms triggered by motorcycles). Conversely, setting a very specific test can eliminate false positives but might miss some true cases (false negatives). The speaker emphasizes the importance of balancing sensitivity and specificity based on the context and the consequences of each type of error.

Mindmap
Keywords
πŸ’‘Sensitivity
Sensitivity refers to the ability of a test to correctly identify those with a condition, also known as the true positive rate. In the context of the video, it is likened to a car alarm's capability to accurately detect a thief attempting to break into the car. A highly sensitive test will have a low false negative rate, meaning it will rarely miss detecting the actual presence of a condition or event, such as a thief trying to steal the car.
πŸ’‘Specificity
Specificity is the ability of a test to correctly identify those without a condition, known as the true negative rate. It is the measure of how often a test correctly does not indicate the presence of a condition when it is not there. In the video, it is compared to a car alarm that only goes off when there is no theft occurring, thus avoiding false alarms. A test with high specificity is less likely to give a positive result when there is no actual condition present, similar to a car alarm that does not go off due to non-threatening noises like a motorcycle.
πŸ’‘True Positive
A true positive occurs when a test correctly identifies the presence of a condition or event. In the video's analogy, it is represented by the car alarm going off when there actually is a thief attempting to break into the car. This is a correct and desirable outcome of a test, indicating that the test is working as intended to detect the condition.
πŸ’‘False Positive
A false positive is when a test incorrectly indicates the presence of a condition or event when there is none. In the context of the video, this would be the car alarm going off due to a passing motorcycle or loud noise, rather than an actual theft attempt. False positives can lead to unnecessary stress and interventions, as resources are expended to investigate a non-existent issue.
πŸ’‘True Negative
A true negative is when a test correctly identifies the absence of a condition or event. In the video, a true negative would be the car alarm not going off when there is no theft attempt, indicating that the test or system is correctly identifying the lack of a problem. True negatives are important as they confirm the absence of a condition, avoiding unnecessary actions or concerns.
πŸ’‘False Negative
A false negative occurs when a test fails to detect the presence of a condition or event when it actually exists. In the car alarm analogy, this would be the car alarm not going off when a theft is in progress. False negatives can lead to serious consequences, as they may result in the condition going untreated or unnoticed.
πŸ’‘Car Alarm Analogy
The car alarm analogy is a conceptual tool used in the video to explain the concepts of sensitivity and specificity in a testing context. It compares the functioning of a car alarm to a medical test, where the alarm's sensitivity is akin to a test's ability to detect true positives and the alarm's specificity is like the test's ability to avoid false positives. The analogy helps to visualize the trade-offs between sensitivity and specificity and how adjusting one can impact the other.
πŸ’‘Trade-off
In the context of the video, a trade-off refers to the balance between sensitivity and specificity in testing. As the sensitivity of a test increases (the ability to detect true positives), the likelihood of false positives also rises. Conversely, increasing specificity (correctly identifying true negatives) reduces the chance of false positives but may also lead to more false negatives. This trade-off is crucial in deciding the appropriate sensitivity and specificity levels for different testing scenarios.
πŸ’‘Medical Testing
Medical testing is the application of various diagnostic procedures to identify, treat, or prevent diseases or conditions. In the video, the concepts of sensitivity and specificity are applied to medical tests, emphasizing the importance of understanding these parameters for accurate diagnosis and patient care. The video uses the car alarm analogy to explain how medical tests need to balance between detecting all cases of a disease (sensitivity) and avoiding misdiagnoses (specificity).
πŸ’‘Test Interpretation
Test interpretation involves understanding and analyzing the results of a test to make informed decisions. In the video, interpreting medical tests requires knowledge of sensitivity and specificity to determine the reliability of the test results. Proper interpretation can lead to accurate diagnoses and appropriate treatment plans, while incorrect interpretation may result in unnecessary interventions or missed conditions.
Highlights

The lecture introduces the concepts of sensitivity and specificity in the context of medical testing and statistics.

Sensitivity and specificity are crucial for interpreting medical journal data and understanding tests ordered for patients.

A car alarm analogy is used to explain the trade-off between sensitivity (true positive rate) and specificity (true negative rate).

A high sensitivity car alarm is likely to detect all thieves (true positives) but also produce false alarms (false positives).

A low sensitivity setting may miss thieves (false negatives) but will rarely produce false alarms.

Specificity is about correctly identifying when a car is not being stolen (true negatives) to avoid unnecessary false alarms.

High specificity reduces the chance of a false positive, ensuring that a positive test result is very likely to be accurate.

The mathematical formula for sensitivity is presented as true positives divided by the sum of true positives and false negatives.

The mathematical formula for specificity is true negatives divided by the sum of true negatives and false positives.

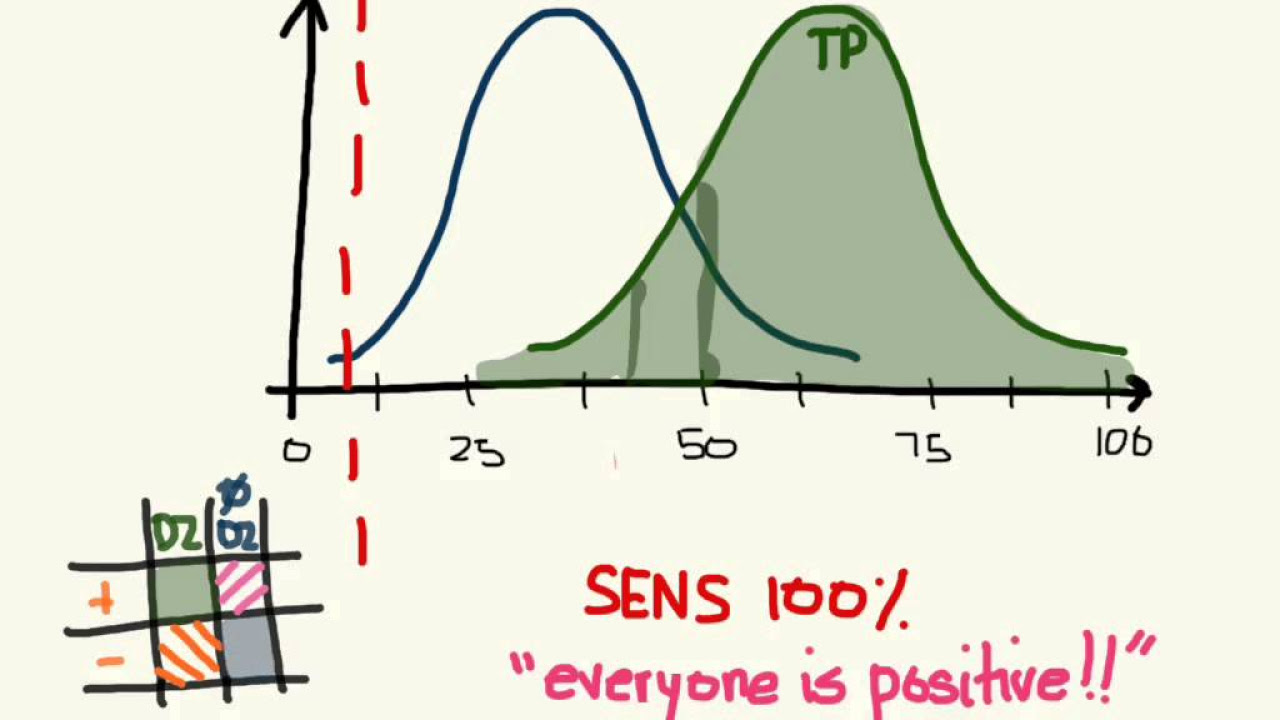
The concept of a threshold is introduced, showing how different settings can lead to varying rates of true and false positives and negatives.

The impact of setting the sensitivity too high or too low is discussed, relating it to the balance between catching thieves and avoiding false alarms.

The lecture emphasizes the importance of setting the right sensitivity and specificity based on the context, such as the prevalence of crime or the environment.

A visual representation of sensitivity and specificity is used to illustrate the concept of a test's threshold and its consequences.

The direction of sensitivity and specificity on the graph is clarified, with sensitivity on the left and specificity on the right.

The lecture concludes with a reminder of the importance of understanding sensitivity and specificity for medical professionals.

Transcripts

Browse More Related Video

Sensitivity vs Specificity Explained (Medical Biostatistics)

Sensitivity and Specificity simplified

Sensitivity, Specificity, Screening Tests & Confirmatory Tests

How to calculate Sensitivity and Specificity

The tradeoff between sensitivity and specificity

How To Catch A Cheater With Math

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MedicalTestingSensitivitySpecificityDiagnosticPrinciplesHealthcareEducationMedicalStatisticsCarAlarmAnalogyDiseaseDetectionTestInterpretationMedicalJournalsHealthcareIndustry