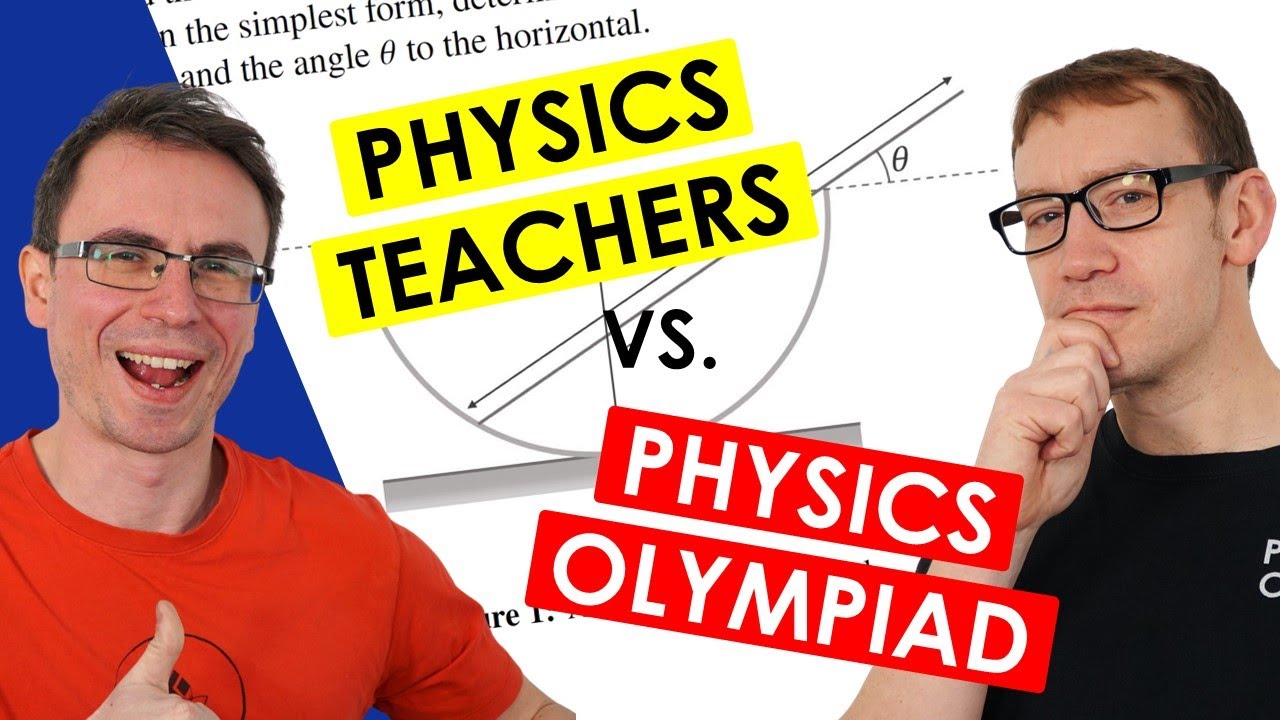
Here is a HARD Physics Olympiad Question - BPhO Round 1, Section 2 2020
TLDRIn this educational video, the host delves into solving complex physics problems from the British Physics Olympiad 2020. Starting with circular motion and gravity, the video explores the maximum speed a car can maintain on a circular bridge without losing contact. It then shifts to astronomical concepts, deriving the orbital period of the sun around the Milky Way and estimating the mass and number of stars in the galaxy. The host also calculates the speed and period of a satellite orbiting Earth, and discusses the fractional change in orbital period with altitude. The video is a rich resource for students preparing for physics competitions, offering clear explanations and step-by-step problem-solving.
Takeaways
- 📚 The video discusses solutions to the British Physics Olympiad Round One Section Two from 2020, with an emphasis on circular motion and gravity.
- 🔍 The presenter highlights the importance of using hints provided in the Olympiad questions and demonstrates how to apply them to solve problems.
- 🎯 The first problem involves calculating the maximum speed a car can travel on a circular bridge while maintaining contact, using geometry and physics principles.
- 📐 A diagram is used to visualize the problem, identifying key distances and applying the Pythagorean theorem to find the bridge's radius.
- 🔄 The formula for centripetal force, mv^2/r, is used to find the maximum speed, equating it to the gravitational force to ensure the car remains in contact with the bridge.
- 🌌 The second part of the video explores the sun's orbit around the Milky Way galaxy, applying Kepler's third law to derive an expression for the orbital period.
- ⚖️ An expression for the mass of the galaxy is derived using the orbital period and radius, providing an estimate of the galaxy's mass.
- ✨ The number of stars in the Milky Way is estimated by dividing the mass of the galaxy by the mass of the sun, assuming the sun represents an average star.
- 🛰️ The video also covers the physics of satellites orbiting Earth, using the gravitational force as the centripetal force to find the satellite's speed.
- 🌍 For the lowest possible theoretical orbit, the speed of a satellite is calculated by equating gravitational acceleration to centripetal acceleration.
- ⏱️ The period of a satellite's orbit is determined using the relationship between speed, radius, and time, and an expression for the fractional change in the orbital period is derived for small heights above Earth.
- 📈 The final part of the video involves sketching a graph to represent the relationship between the orbital period and the height of the satellite above Earth, demonstrating a linear relationship.
Q & A
What is the main topic discussed in the video?
-The main topic discussed in the video is solving problems from the British Physics Olympiad, Round One Section Two from 2020, with a focus on circular motion and gravity.
Why is it recommended to use the hint provided in the circular motion and gravity problem?
-Using the hint is recommended because it can simplify the problem-solving process and is often a key to unlocking the solution, as it relates to the geometry of the bridge's cross-section.
What is the formula used to calculate the maximum speed a car can drive over the bridge while remaining in contact with it?
-The formula used is \( v = \sqrt{r \times g} \), where \( v \) is the speed, \( r \) is the radius of the bridge, and \( g \) is the acceleration due to gravity.
What is the radius of the bridge calculated to be in the video?
-The radius of the bridge is calculated to be approximately 12.125 meters.
What is the maximum speed calculated for the car to remain in contact with the bridge?
-The maximum speed calculated for the car is approximately 10.9 meters per second.
What is Kepler's Third Law as derived in the video for the orbit of the Sun around the Milky Way galaxy?
-Kepler's Third Law, as derived in the video, states that \( T^2 = \frac{4\pi^2}{GM} R^3 \), where \( T \) is the orbital period, \( G \) is the gravitational constant, \( M \) is the mass of the galaxy, and \( R \) is the radius of the orbit.
How is the mass of the galaxy estimated in the video?
-The mass of the galaxy is estimated by rearranging the derived formula to \( M = \frac{4\pi^2 R^3}{GT^2} \) and plugging in the values for the radius of the Sun's orbit and the orbital period.
What is the estimated mass of the galaxy in kilograms according to the video?
-The estimated mass of the galaxy is approximately \( 3.41 \times 10^{41} \) kilograms.
How many stars are estimated to be in the Milky Way based on the mass of the galaxy and the mass of the Sun?
-Based on the given mass estimates, there are approximately \( 1.7 \times 10^{11} \) stars in the Milky Way, assuming the Sun represents the mass of an average star.
What is the expression for the speed \( v \) of a satellite orbiting the Earth in terms of the Earth's radius \( R_e \), the satellite's orbital radius \( r \), and the Earth's mass \( M_e \)?
-The expression for the speed \( v \) is \( v = \sqrt{\frac{GM_e}{r}} \), where \( G \) is the gravitational constant.
What is the significance of the height \( h \) being significantly less than the Earth's radius \( R_e \) in the context of the satellite's orbital period?
-When \( h \) is significantly less than \( R_e \), it allows for the use of an approximation in the expansion of Kepler's Third Law, simplifying the calculation of the satellite's orbital period.
How is the fractional change in the orbital period \( \Delta t / t \) expressed in terms of the satellite's height \( h \) and the Earth's radius \( R_e \)?
-The fractional change in the orbital period is expressed as \( \Delta t / t = \frac{3h}{2R_e} \).
What type of graph represents the relationship between the orbital period \( t' \) and the satellite's height \( h \)?
-The relationship between the orbital period \( t' \) and the satellite's height \( h \) is represented by a straight line graph that passes through the origin.
Outlines
🔍 Solving Circular Motion Problems
This paragraph introduces a problem from the 2020 British Physics Olympiad, focusing on circular motion and gravity. The host emphasizes the importance of using the provided hint and begins by drawing a diagram to visualize the problem. The scenario involves a car driving over a circular bridge with a specific width and height, and the challenge is to determine the maximum speed at which the car can safely drive without losing contact with the bridge. The host plans to use geometric rules to find the radius of the bridge and then apply the physics of circular motion to solve for the speed.
🌌 The Sun's Orbit Around the Milky Way
The second paragraph delves into a problem involving the Sun's orbit around the center of the Milky Way galaxy. The host discusses using Kepler's third law to derive an expression for the orbital period of the Sun in terms of the radius of its orbit, the gravitational constant, and the mass of the galaxy. The host also calculates an estimate for the mass of the galaxy using the given astronomical values and explains the steps involved in this calculation, including the conversion of light years to meters and the use of the gravitational constant.
🌟 Estimating the Number of Stars in the Milky Way
In this paragraph, the host estimates the number of stars in the Milky Way by using the mass of the galaxy and the mass of the Sun. The host divides the estimated mass of the galaxy by the mass of the Sun to arrive at an approximate number of stars, assuming that the Sun represents an average star. The result is a staggering number of stars, highlighting the vastness of the galaxy.
🛰️ Satellite Orbital Mechanics
The host explains the physics behind calculating the speed of a satellite orbiting the Earth. The gravitational force is equated to the centripetal force required for circular motion, leading to a formula for the satellite's speed in terms of the Earth's mass, the satellite's orbital radius, and the gravitational constant. The host also discusses the concept of the lowest possible theoretical orbit, where the satellite would be just grazing the Earth's surface, and calculates the corresponding speed.
⏱️ Calculating Satellite Orbital Periods
This paragraph focuses on calculating the orbital period of a satellite. The host uses the relationship between speed, distance, and time to find the period of the satellite's orbit. The host then introduces a scenario where the satellite orbits at a height significantly less than the Earth's radius and derives an expression for the fractional change in the orbital period due to this height. The host applies mathematical expansions to simplify the expression and explains the steps involved in this process.
📈 Graphing Orbital Periods vs. Height
The final paragraph discusses graphing the relationship between a satellite's orbital period and its height above the Earth's surface. The host identifies the graph as a straight line with a y-intercept, representing the time period at the Earth's surface, and a slope representing the rate of change of the period with respect to height. The host explains the mathematical relationship as 'y = mx + c' and provides a brief analysis of how the graph would appear, indicating the simplicity of the expression derived for the fractional change in the time period.
Mindmap
Keywords
💡Circular Motion
💡British Physics Olympiad
💡Centripetal Force
💡Gravitational Force
💡Orbital Period
💡Kepler's Third Law
💡Mass of the Galaxy
💡Satellite Orbit
💡Expansion
💡Fractional Change
💡Graph
Highlights
Introduction to solving British Physics Olympiad Round One Section Two (2020), focusing on circular motion and gravity.
Explanation of problem-solving strategy using geometry to find the radius of a circular bridge.
Deriving the radius of the bridge using chord geometry.
Calculation of the maximum speed at which a car can drive over the bridge while maintaining contact.
Introduction to Kepler's Third Law and its application to the Sun's orbit in the Milky Way galaxy.
Derivation of the orbital period of the Sun using gravitational force and centripetal force.
Calculation of the mass of the Milky Way galaxy using derived orbital period and Kepler's Third Law.
Estimation of the number of stars in the Milky Way by comparing the galaxy's mass to the Sun's mass.
Derivation of the speed of a satellite orbiting the Earth in terms of the Earth's mass and radius.
Calculation of the speed of a satellite in the lowest possible theoretical orbit, just grazing the Earth.
Calculation of the period of a satellite orbiting at Earth's surface using circular motion principles.
Application of mathematical expansion techniques to determine the fractional change in orbital period at a small height above Earth's surface.
Graphical representation of the orbital period as a function of height, with an analysis of the gradient and intercept.
Conclusion summarizing key lessons on expansions and recognizing clues in physics problem-solving.
Encouragement for students preparing for the Physics Olympiad, emphasizing the importance of practice and problem-solving strategies.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: