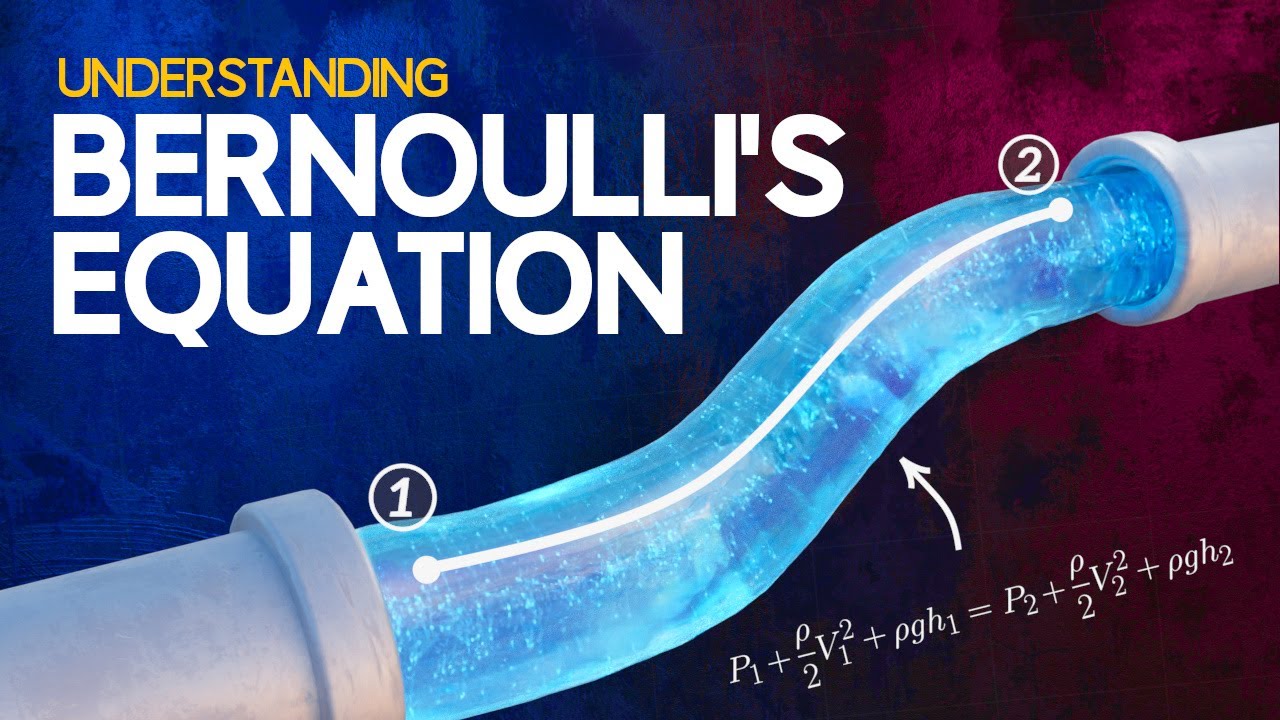
IMPORTANT Derivations in Physics: Bernoulli's Equation

ZPhysics
14 Jan 202403:57
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script delves into the derivation of Bernoulli's equation, a fundamental principle in fluid dynamics. It explains how the pressure in a pipe decreases as fluid velocity increases, using the concept of energy conservation. The script guides through the derivation process, starting from the work done on a particle to the change in kinetic and potential energy, leading to the equation's famous form. It highlights the equation's significance in understanding fluid behavior and its practical applications, such as lift force generation.

Takeaways
  • πŸ“š The script discusses the importance of derivations in physics, particularly in the context of fluid mechanics.
  • πŸŒ€ It introduces the concept of deriving the Bernoulli's equation, a fundamental principle in fluid dynamics.
  • πŸ› οΈ The derivation begins with the application of energy conservation to fluid particles moving through a pipe that narrows.
  • πŸ”§ Work done (DW) on a small particle is equated to the difference in forces due to pressure multiplied by the distance traveled.
  • βš–οΈ The forces are expressed in terms of pressure (P1 and P2) and the distances (DS1 and DS2).
  • πŸš€ The change in kinetic energy is represented as the difference between the square of velocities (v2^2 - v1^2) over 2.
  • πŸ“Š The change in potential energy is accounted for by the product of mass, gravity (g), and the difference in height (y2 - y1).
  • πŸ’§ The script assumes an incompressible fluid, meaning the same mass flows through both regions of the pipe.
  • πŸ”„ The mass flow rate is expressed in terms of density and volume, which simplifies the equation by canceling out terms.
  • πŸ“ The final form of the equation is presented, showing that the sum of kinetic, potential, and pressure energies is constant along a streamline.
  • πŸŽ“ The Bernoulli's equation has numerous applications in fluid mechanics, including the explanation of lift forces in aerodynamics.
Q & A

  • What is the main focus of the video script?

    -The main focus of the video script is to derive one of the most beautiful equations in fluid mechanics, which is related to the behavior of fluid particles in a pipe that narrows as they move.

  • What is the driving force for fluid particles moving in a pipe?

    -The driving force for fluid particles moving in a pipe is the difference in pressure.

  • What is the infinitesimal amount of work done on a small particle in the script called?

    -The infinitesimal amount of work done on a small particle is referred to as DW in the script.

  • How are the forces due to pressure expressed in the script?

    -The forces due to pressure are expressed as F1 multiplied by a small element DS1 and F2 multiplied by DS2, where P1 and P2 are the pressures at different points.

  • What is the relationship between work done and the change in energy as a particle moves?

    -The work done is equal to the change in energy as the particle moves, which includes changes in both kinetic and potential energies.

  • What assumption is made about the fluid in the script?

    -The script assumes that the fluid is incompressible and the same amount of mass per second flows through both regions of the pipe.

  • How is mass related to density and volume in the script?

    -In the script, mass is equal to the product of density and volume, expressed as ρ times the tiny volume DV.

  • What is the famous equation derived in the script?

    -The famous equation derived in the script is Bernoulli's equation, which has numerous applications in fluid mechanics.

  • What does Bernoulli's equation explain about the relationship between velocity and pressure?

    -Bernoulli's equation explains that if the velocity of the fluid is high, the pressure will drop, potentially creating a difference in pressure that can generate a lift force.

  • What is the next step suggested for students after understanding Bernoulli's equation?

    -The next step suggested is to apply Bernoulli's equation to problems, with an emphasis on finding problems with cool applications of the equation.

  • What is the significance of canceling terms in the derivation process mentioned in the script?

    -Canceling terms in the derivation process simplifies the equation and helps in reaching Bernoulli's equation in its most famous form, where all terms are equal to a constant.

Outlines
00:00
πŸ“š Derivation of Bernoulli's Equation in Fluid Mechanics

The paragraph introduces the derivation of one of the most significant equations in fluid mechanics, Bernoulli's equation. It begins by setting a scenario involving a narrowing pipe through which fluid particles move due to a pressure difference. The process of derivation involves applying the principle of energy conservation, considering the work done (DW) on an infinitesimal particle, the forces due to pressure (F1 and F2), and the distances traveled (DS1 and DS2). The equation is then developed by equating the work done to the change in energy, including changes in kinetic and potential energy. The derivation assumes an incompressible fluid, leading to the cancellation of terms and the final form of Bernoulli's equation, which relates pressure, velocity, and height in a fluid flow. The paragraph concludes by emphasizing the importance of applying this equation to practical problems, hinting at its wide-ranging applications in fluid mechanics.

Mindmap
Keywords
πŸ’‘Derivations
Derivations in the context of physics refer to the process of deriving or deducing mathematical formulas from fundamental principles. They are crucial for understanding the underlying principles of physical phenomena. In the video, derivations are highlighted as a vital skill, especially in the derivation of the Bernoulli's equation, which is a central theme of the video.
πŸ’‘Fluid Mechanics
Fluid Mechanics is a branch of physics that studies the behavior of fluids, both liquids and gases, at rest and in motion. It is essential for understanding how fluids interact with their environment and how they can be controlled. The video focuses on a derivation within fluid mechanics, specifically the Bernoulli's equation, which is a key concept in this field.
πŸ’‘Pipe
In the video, a 'pipe' is used as a practical example to illustrate the principles of fluid dynamics. It is a conduit through which fluid flows, and its narrowing shape is central to the demonstration of how pressure differences can cause fluid particles to move.
πŸ’‘Pressure
Pressure is defined as the force exerted per unit area and is a key factor in fluid dynamics. It is what drives the flow of fluid in the pipe example given in the video. The difference in pressure is what causes the fluid particles to accelerate as they move through the narrowing pipe.
πŸ’‘Energy Conservation
Energy Conservation is a fundamental principle stating that energy cannot be created or destroyed, only transformed. In the video, this principle is applied to derive the Bernoulli's equation, showing how the work done on a fluid particle is equal to the change in its energy as it moves through the pipe.
πŸ’‘Kinetic Energy
Kinetic Energy is the energy possessed by an object due to its motion, calculated as one-half the mass of the object times the square of its velocity. In the script, the change in kinetic energy is considered when calculating the work done on a fluid particle as it moves through the pipe.
πŸ’‘Potential Energy
Potential Energy is the stored energy of an object due to its position in a force field, such as gravity. In the video, the change in potential energy is also considered in the derivation, as the height of the fluid changes along the pipe, affecting the total energy of the system.
πŸ’‘Incompressible Fluid
An incompressible fluid is one whose density does not change when subjected to pressure. The video assumes the fluid to be incompressible, which simplifies the derivation by allowing the cancellation of terms in the equation, leading to the Bernoulli's equation.
πŸ’‘Bernoulli's Equation
Bernoulli's Equation is a fundamental principle in fluid dynamics that relates the pressure, kinetic, and potential energy of a fluid in motion. The video script derives this equation, demonstrating how these energies are conserved along a streamline in a fluid flow.
πŸ’‘Lift Force
Lift Force is the upward force that is exerted on an object in a fluid flow, often experienced in aerodynamics. The video script mentions that the Bernoulli's principle can explain how a high-velocity fluid flow can lead to a pressure drop, potentially creating a lift force.
Highlights

The importance of derivations in physics exams, particularly in fluid mechanics.

Derivation of one of the most beautiful equations in fluid mechanics.

The concept of a pipe narrowing and the effect of pressure difference on fluid particles.

Application of energy conservation in deriving the equation.

Calculation of work done (DW) for an infinitesimal amount on a small particle.

Expression of forces in terms of pressure (F1 and F2) and their respective distances (DS1 and DS2).

Equating work done to the change in energy, including kinetic and potential energy changes.

Assumption of incompressibility and constant mass flow rate through different regions.

Rewriting mass in terms of density and volume for the equation.

Simplification of the equation by canceling terms and rearranging components.

Introduction of the famous Bernoulli's equation in its most recognized form.

Explanation of how velocity and pressure are related in fluid dynamics.

Practical applications of Bernoulli's equation, including the creation of lift forces.

Encouragement to apply the derived equation to problems for a deeper understanding.

Mention of an amazing problem with a cool application of Bernoulli's equation.

The significance of understanding and applying derivations in physics for problem-solving.

Transcripts

Browse More Related Video

Bernoulli's Equation

20. Fluid Dynamics and Statics and Bernoulli's Equation

Understanding Bernoulli's Equation

Fluids in Motion: Crash Course Physics #15

Fluids (AP Physics SuperCram Review)

AP Physics 2 Fluids Review

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Fluid MechanicsBernoulli's EquationPhysics DerivationEnergy ConservationIncompressible FluidPressure DifferenceKinetic EnergyPotential EnergyLift ForceEducational ContentSTEM Learning