Bernoulli's Equation

Bozeman Science

12 Mar 201510:12

EducationalLearning

32 Likes 10 Comments

TLDRThis AP Physics essentials video by Mr. Andersen delves into Bernoulli's Equation, a fundamental principle in fluid dynamics. It explains how the equation, which conserves energy within a fluid, can be applied to various real-world scenarios, such as the design of docks to prevent damage from large boats. The script introduces the continuity equation as a precursor to understanding Bernoulli's, which relates pressure, potential, and kinetic energy within a fluid. Through examples like a pipe with varying cross-sectional areas and a thought experiment with a milk carton, the video illustrates how changes in fluid velocity and height affect pressure. The video also creatively connects Bernoulli's principles to phenomena like the curveball in baseball, demonstrating the equation's broad applicability in analyzing fluid behavior.

Takeaways

🚤 The reason docks are not solid is to allow water to flow underneath, preventing high pressure on the outside of a boat from damaging it.
🌊 Bernoulli's Equation is an application of the conservation of energy within a fluid, relating pressure, potential energy, and kinetic energy.
🔍 The Continuity Equation (A1V1 = A2V2) is crucial for understanding fluid dynamics, showing the relationship between cross-sectional area and velocity at different points in a pipe.
📐 As the cross-sectional area of a pipe decreases, the velocity of the fluid increases, and vice versa, according to the Continuity Equation.
💧 When the cross-sectional area of a hose is reduced (e.g., by a thumb), the velocity of the water increases due to the same principle of fluid dynamics.
📈 Bernoulli's Equation is composed of pressure energy (P), potential energy (ρgy), and kinetic energy (½ρv²), which are all forms of energy within a fluid.
🏞️ The potential energy in a fluid is influenced by its height (y) and is calculated as mgh, where m is mass, g is gravitational field strength, and h is height.
⚖️ The conservation of energy principle allows us to equate the total energy on one side of a pipe to the other, enabling us to solve for unknown variables like pressure.
🔑 Understanding the distribution of potential energy in a system helps predict the behavior of fluid flow and the effects of Bernoulli's Equation.
🧐 Bernoulli's Equation can be used to analyze and calculate complex fluid dynamics problems, including the pressure changes in various scenarios like docks and curveballs.
🏈 The curve of a curveball in baseball is explained by Bernoulli's Equation, where the spinning ball creates different airflow velocities above and below it, leading to pressure differences and a curved trajectory.

Q & A

What is Bernoulli's Equation and why is it important in fluid dynamics?
-Bernoulli's Equation is a principle that represents the conservation of energy within a fluid. It's important because it allows us to understand and calculate the behavior of fluids in various situations, such as the flow around objects or the pressure differences that occur when a fluid's velocity changes.
How does Bernoulli's Equation relate to the design of docks?
-Docks are often constructed with pylons rather than being solid to allow water to flow underneath. This design is supported by Bernoulli's Equation, which states that when a fluid flows faster (like under a dock), it exerts lower pressure. If the dock were solid, the water would be forced out of the way, creating a pressure difference that could damage the dock.
What is the Continuity Equation and how does it work?
-The Continuity Equation, represented as A1 * V1 = A2 * V2, is a principle that states the product of the cross-sectional area (A) and the velocity (V) of a fluid at any two points in a pipe remains constant if the fluid is incompressible and the flow is steady. It helps in determining the velocity at different points in a pipe when the cross-sectional area changes.
How does the cross-sectional area of a pipe affect the fluid velocity?
-According to the Continuity Equation, if the cross-sectional area of a pipe increases, the fluid velocity decreases, and vice versa. This is because the same amount of fluid must pass through the pipe every second, so a larger area allows for a slower flow rate.
What is the significance of the potential energy term in Bernoulli's Equation?
-The potential energy term in Bernoulli's Equation, represented by rho * g * y, accounts for the energy a fluid has due to its height (y) above a reference point. It's important because it shows how the height of a fluid column can affect the total energy and pressure within the fluid.
How does the density of a fluid (rho) influence the energy within a fluid?
-The density of a fluid (rho) is a factor in both the potential energy (rho * g * y) and kinetic energy (rho * V^2 / 2) components of Bernoulli's Equation. A denser fluid will have more potential and kinetic energy for a given height and velocity, respectively, which affects the overall energy balance within the fluid.
What is the application of Bernoulli's Equation in the case of a hose with a thumb over it?
-When you place your thumb over the end of a hose, you decrease the cross-sectional area through which water can flow. According to the Continuity Equation, this decrease in area results in an increase in velocity. Bernoulli's Equation then tells us that with an increase in velocity (kinetic energy), the pressure will decrease.
Why do streams of milk come out farther when a carton with holes is tapped from the bottom?
-When a carton with holes is tapped from the bottom, the streams of milk come out farther because the milk at the bottom has more potential energy due to its height above the ground. This potential energy is converted into kinetic energy when the tape is removed, resulting in a faster velocity and farther reach of the streams.
How can Bernoulli's Equation be used to explain the curve of a curveball in baseball?
-A curveball curves due to the differential air pressure created by the ball's spin. The spinning motion causes the air to move faster over one side of the ball, reducing the pressure on that side according to Bernoulli's Equation. The higher pressure on the other side creates a force that pushes the ball downward, causing it to curve.
What is the practical application of Bernoulli's Equation in solving complex fluid dynamics problems?
-Bernoulli's Equation can be used to solve complex problems involving fluid dynamics by providing a way to calculate and compare the pressure, potential energy, and kinetic energy at different points in a fluid system. This allows engineers and scientists to predict and analyze the behavior of fluids in various scenarios, such as the flow through pipes, the lift on an airfoil, or the forces acting on a moving object.

Outlines

00:00

🌊 Understanding Bernoulli's Equation and Fluid Dynamics

In this segment, Mr. Andersen introduces Bernoulli's Equation, a fundamental principle in fluid dynamics that explains the relationship between pressure, velocity, and energy within a fluid. He uses the example of a dock made of pylons to illustrate how a solid dock would force water out, creating a pressure difference that could damage the dock. The video then delves into the continuity equation, which is essential for understanding Bernoulli's Equation. The continuity equation, A1*V1 = A2*V2, relates the cross-sectional area and velocity of a fluid at different points in a pipe. Mr. Andersen explains how decreasing the area of a pipe increases the fluid's velocity, using the example of a hose with a thumb placed over it. The summary of Bernoulli's Equation, which includes pressure, potential energy, and kinetic energy components, is presented, highlighting its conservation of energy principle. Practical applications, such as the design of docks and the behavior of fluids in pipes, are discussed to cement the understanding of the concepts.

05:05

🚀 Applying Bernoulli's Equation to Real-World Scenarios

This paragraph continues the exploration of Bernoulli's Equation by applying it to real-world scenarios. Mr. Andersen discusses a thought experiment involving a half-gallon milk carton with holes, demonstrating how the streams of milk would behave when the potential energy is converted to kinetic energy. The explanation focuses on the differences in potential energy at the bottom and top of the carton, affecting the distance the milk streams travel. The video then moves on to solving a problem using Bernoulli's Equation, where the unknown pressure on the right side of a pipe is calculated based on known variables such as velocity, height, and pressure on the left side. The process involves using the conservation of energy principle to equate the total energy on both sides of the pipe. The video concludes with an explanation of how a curveball in baseball works, utilizing the principles of fluid dynamics to explain the ball's trajectory and the effect of air pressure on its movement. The summary emphasizes the practical applications of Bernoulli's Equation in analyzing and solving problems related to fluid dynamics.

Mindmap

Keywords

💡Bernoulli's Equation

Bernoulli's Equation is a fundamental principle in fluid dynamics that describes the conservation of energy in a fluid. It states that the sum of pressure energy, kinetic energy, and potential energy per unit volume remains constant along a streamline. In the video, Bernoulli's Equation is used to explain why docks are not solid and to analyze the behavior of fluids in various scenarios, such as the flow of water under a dock or the movement of air around a curveball.

💡Fluid Dynamics

Fluid dynamics is the study of the motion of liquids and gases. It is a subset of fluid mechanics and is crucial for understanding how fluids behave under various conditions. The video script delves into fluid dynamics to explain the principles behind Bernoulli's Equation and its applications, such as the design of docks and the aerodynamics of a curveball.

💡Conservation of Energy

The conservation of energy is a fundamental concept in physics, stating that energy cannot be created or destroyed, only transformed from one form to another. In the context of the video, this principle is applied to fluids, where the energy within a fluid is conserved and can be exchanged between kinetic, potential, and pressure energy forms as described by Bernoulli's Equation.

💡Continuity Equation

The continuity equation is a fundamental principle in fluid dynamics that relates the velocity and cross-sectional area of a fluid at different points in a flow. It states that the product of the cross-sectional area and the velocity at any point in a streamline is constant. The video uses the continuity equation to explain how changes in the area of a pipe affect the velocity of the fluid flowing through it.

💡Cross-Sectional Area

The cross-sectional area is the area of a plane cutting through an object perpendicular to the direction of flow. In the context of the video, changes in the cross-sectional area of a pipe are used to illustrate how the velocity of a fluid changes according to the continuity equation. A decrease in area results in an increase in velocity, and vice versa.

💡Velocity

Velocity is a vector quantity that refers to the speed of an object in a particular direction. In fluid dynamics, it is used to describe the speed and direction of a fluid flow. The video script explains how velocity is related to the cross-sectional area in the continuity equation and how it affects the pressure and kinetic energy in Bernoulli's Equation.

💡Pressure

Pressure is the force per unit area exerted on a surface. In the context of the video, pressure is one of the components in Bernoulli's Equation, representing the pressure energy within a fluid. The script uses the concept of pressure to explain how faster-moving fluids have lower pressure, which can affect the behavior of objects in a fluid flow, such as a boat in a dock.

💡Potential Energy

Potential energy is the stored energy of an object due to its position in a force field, such as gravitational potential energy. In the video, potential energy is incorporated into Bernoulli's Equation as the height of the fluid column (rho * g * y), where rho is the fluid density, g is the gravitational field strength, and y is the height. It is used to explain how the height of a fluid affects its energy and pressure.

💡Kinetic Energy

Kinetic energy is the energy possessed by an object due to its motion. In the video, kinetic energy is represented in Bernoulli's Equation as the energy associated with the motion of the fluid (1/2 * rho * v^2), where rho is the fluid density and v is the velocity. The script explains how changes in kinetic energy are related to changes in velocity and how this affects the overall energy balance in a fluid flow.

💡Curveball

A curveball is a type of pitch in baseball that curves or bends as it approaches the batter. In the video, the curveball is used as an example to illustrate the application of Bernoulli's Equation to real-world phenomena. The spin of the ball creates different air velocities above and below it, resulting in different pressures that cause the ball to curve.

Highlights

Bernoulli’s Equation is an application of conservation of energy inside a fluid.

Docks are not solid to allow water flow, preventing damage from pressure differences.

The Continuity Equation (A1V1 = A2V2) is essential for understanding fluid dynamics.

A larger cross-sectional area in a pipe results in a lower velocity and vice versa.

The velocity of fluid can be determined if the cross-sectional area is known.

Decreasing the cross-sectional area of a hose increases the velocity of the water.

Bernoulli’s Equation incorporates height (potential energy) and fluid density.

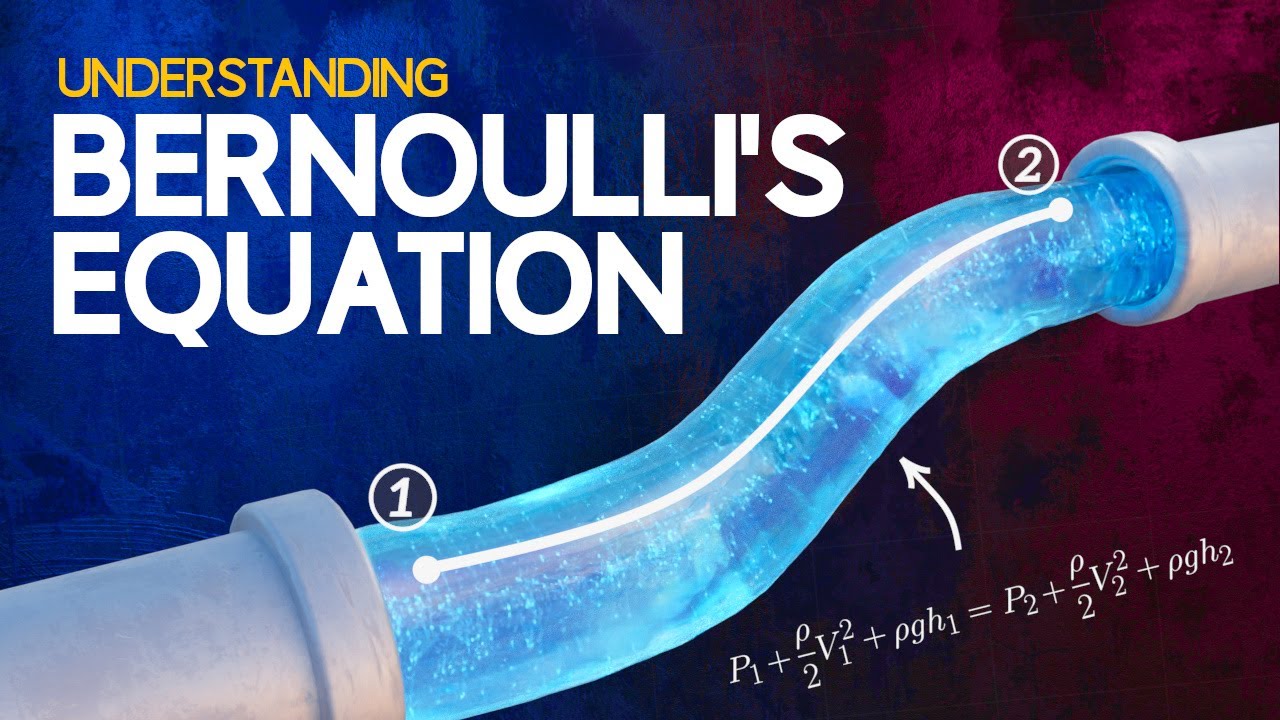
The equation P + (ρgy) + (1/2)ρv^2 represents the conservation of energy in a fluid.

Pressure, potential energy, and kinetic energy can be calculated using Bernoulli’s Equation.

Fluid moving faster will have a lower pressure, as demonstrated with a ship and a dock.

The PHEt simulation illustrates fluid dynamics and the application of the Continuity Equation.

Bernoulli’s Equation can be used to solve for unknown pressures in a fluid system.

The thought experiment with a milk carton demonstrates how potential energy converts to kinetic energy.

Understanding potential energy distribution helps in applying Bernoulli’s Equation.

Bernoulli’s Equation can be used to explain the curve of a curveball in baseball.

The pressure difference created by the spin of a curveball causes it to curve.

Bernoulli’s Equation is a powerful tool for analyzing fluid dynamics and pressure changes.

Transcripts

Browse More Related Video

Understanding Bernoulli's Equation

IMPORTANT Derivations in Physics: Bernoulli's Equation

Fluids in Motion: Crash Course Physics #15

20. Fluid Dynamics and Statics and Bernoulli's Equation

Bernoulli's principle

Bernoulli's Equation

Takeaways

Q & A

What is Bernoulli's Equation and why is it important in fluid dynamics?

How does Bernoulli's Equation relate to the design of docks?

What is the Continuity Equation and how does it work?

How does the cross-sectional area of a pipe affect the fluid velocity?

What is the significance of the potential energy term in Bernoulli's Equation?

How does the density of a fluid (rho) influence the energy within a fluid?

What is the application of Bernoulli's Equation in the case of a hose with a thumb over it?

Why do streams of milk come out farther when a carton with holes is tapped from the bottom?

How can Bernoulli's Equation be used to explain the curve of a curveball in baseball?

What is the practical application of Bernoulli's Equation in solving complex fluid dynamics problems?