Biot Savart law (vector form) | Moving charges & magnetism | Khan Academy
TLDRThis educational video script delves into the principles of magnetism, specifically focusing on the Biot-Savart Law. It explains how to calculate the magnetic field generated by a current-carrying wire. The script breaks down the formula, emphasizing the importance of considering infinitesimal wire elements and the angle between the current and the position vector. It highlights the inverse square law's role in magnetic field strength and the sine function's impact on field variation with angle. The constant 'mu naught' and its value for a vacuum are introduced, and two methods for determining the magnetic field's direction—the right-hand rule and cross product—are discussed, illustrating the field's maximum perpendicularity to the current element and minimum along the axis.
Takeaways
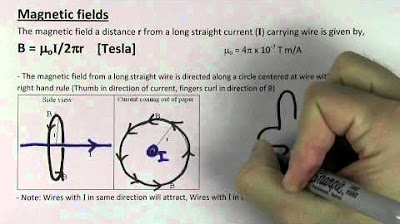
- 🧲 The magnetic field around a current-carrying wire can be calculated using Ampère's law, also known as the Biot-Savart law.
- 📏 To apply the Biot-Savart law, consider an infinitesimal element of wire with length \( \text{d}l \).
- 🔌 The strength of the magnetic field \( \text{d}B \) at a point due to this element is given by the equation involving the permeability constant, current, and the vector cross product of the position vector and the infinitesimal length vector.
- 🎛 The magnitude of \( \text{d}B \) depends on the current \( I \), the length of the wire element \( \text{d}l \), the sine of the angle between the wire element and the position vector, and is inversely proportional to the square of the distance \( r \).
- 🔍 The magnetic field is strongest at a right angle (90 degrees) to the current element and weakest on the axis of the current element.
- 🌐 The magnetic field decreases with distance from the wire, following an inverse square law similar to Newton's law of gravity and Coulomb's law for electric fields.
- 🔢 The constant \( \mu_0 \) in the equation, known as the permeability of free space, has a value of \( 4\pi \times 10^{-7} \) Tm/A.
- 🤔 The direction of the magnetic field can be determined using the right-hand rule, which involves the direction of the current and the position vector.
- 📐 The cross product in the Biot-Savart law also gives the direction of the magnetic field, aligning the thumb of the right hand to point in the direction of the field when the fingers point along the cross.
- 📚 For the total magnetic field due to the entire wire, sum up the contributions from each infinitesimal element or perform an integral over the wire's length.
- 🔬 The Biot-Savart law is analogous to Coulomb's law for electric fields but includes the sine of the angle between the current element and the position vector, reflecting the directional nature of magnetic fields.
Q & A
What is the Biot-Savart Law?
-The Biot-Savart Law is a fundamental principle in magnetism that describes the magnetic field produced by a steady electric current. It states that the magnetic field strength at a point due to an infinitesimal current element is directly proportional to the current, the length of the element, and the sine of the angle between the element and the line from the element to the point, and inversely proportional to the square of the distance from the element to the point.
How do you calculate the magnetic field due to a tiny element of wire using the Biot-Savart Law?
-To calculate the magnetic field at a point due to a tiny element of wire, you use the formula: \( \text{dB} = \frac{\mu_0}{4\pi} \cdot I \cdot \text{d}l \times \hat{r}}{r^2} \), where \( \mu_0 \) is the permeability of free space, \( I \) is the current, \( \text{d}l \) is the infinitesimal length of the wire, \( \hat{r} \) is the unit vector pointing from the wire element to the point, and \( r \) is the distance from the wire element to the point.
What is the significance of the cross product in the Biot-Savart Law?
-The cross product in the Biot-Savart Law, \( \text{d}l \times \hat{r} \), determines the direction of the magnetic field. It shows that the magnetic field is perpendicular to both the direction of the current and the line connecting the current element to the point where the field is being measured.
What is the unit vector in the direction of r in the Biot-Savart Law?
-The unit vector in the direction of r, denoted as \( \hat{r} \), is a vector with a magnitude of 1 that points from the current element to the point where the magnetic field is being calculated. It is used to account for the direction of the magnetic field in the Biot-Savart Law.
How does the strength of the current affect the magnetic field produced by a wire?
-The strength of the current (I) is directly proportional to the magnetic field produced by the wire. A higher current means more moving charges per second, resulting in a stronger magnetic field.
What is the role of the length of the current element (dl) in the magnetic field calculation?
-The length of the current element (dl) is also directly proportional to the magnetic field. A longer current element means more moving charges are contributing to the magnetic field, thus increasing its strength.
What is the significance of the angle theta (θ) in the Biot-Savart Law?
-The angle theta (θ) between the current element and the line to the point where the magnetic field is being measured determines the contribution of the current element to the magnetic field at that point. The magnetic field is maximum when the angle is 90 degrees (sine of 90 degrees is 1) and minimum (or zero) when the angle is 0 or 180 degrees (sine of 0 and 180 degrees is 0).
What is the value of the permeability constant (mu naught) in a vacuum?
-The permeability constant (mu naught) in a vacuum is \( 4\pi \times 10^{-7} \) Tm/A (Tesla meters per Ampere), which is a measure of the ability of a vacuum to support the formation of a magnetic field.
How does the distance from the current element affect the magnetic field strength?
-The magnetic field strength is inversely proportional to the square of the distance (r^2) from the current element. This means that the magnetic field decreases rapidly as the distance from the current element increases.
How can you determine the direction of the magnetic field produced by a current element?
-The direction of the magnetic field can be determined using the right-hand rule, where you point your thumb in the direction of the current and your fingers will curl around the conductor in the direction of the magnetic field. Alternatively, you can use the cross product of the current element vector and the unit vector pointing from the element to the point of interest.
What is the difference between the Biot-Savart Law and Coulomb's Law in terms of field direction?
-Unlike Coulomb's Law, which gives a radial electric field around a point charge, the Biot-Savart Law includes a sine term that accounts for the angle between the current element and the line to the point of interest, resulting in a magnetic field that is not the same everywhere around a current element and is maximum perpendicular to the current element.
Outlines
🧲 Understanding the Biot-Savart Law
The first paragraph introduces the concept of the magnetic field generated by current-carrying wires and presents the Biot-Savart Law, a fundamental principle in magnetism. It explains the necessity to consider an infinitesimal element of the wire, denoted as 'dl', with a current 'i' flowing through it. The law is used to calculate the magnetic field 'dB' at a point 'r' distance away from this wire element. The formula involves a constant 'mu naught' (μ₀), the permeability of free space, and emphasizes the vector nature of the magnetic field, highlighting the cross product between 'dl' and the unit vector 'r̂', and the sine of the angle θ between them. The explanation simplifies the vector equation to its magnitude, discussing the dependency of the magnetic field on the current, the length of the wire element, and the inverse square of the distance from the element, drawing parallels with Coulomb's Law and Newton's Law of Gravity.
🌐 The Directionality of Magnetic Fields
The second paragraph delves into the directional aspect of magnetic fields, contrasting it with the uniform electric field around a point charge. It uses the sine function from the Biot-Savart Law to illustrate how the magnetic field's strength varies with the angle θ between the current element and the position vector 'r'. The paragraph explains that the magnetic field is strongest at a 90-degree angle (perpendicular to the current) and weakest or zero when parallel or anti-parallel to the current (0 or 180 degrees). It emphasizes the importance of considering both distance and angle when evaluating magnetic field strength, and introduces 'mu naught' as a constant for vacuum with a value of 4π × 10^-7 Tm/A, also known as the permeability of vacuum.
🤔 Determining the Direction of the Magnetic Field
The third paragraph focuses on determining the direction of the magnetic field produced by a current element. It presents two methods: the right-hand clasp rule and the cross product of 'dl' and 'r'. The right-hand clasp rule involves pointing the thumb in the direction of the current, with the fingers encircling the conductor, indicating the direction of the magnetic field. The cross product method requires aligning the palm to face the direction of the cross between 'dl' and 'r', with the thumb pointing in the direction of the magnetic field. The paragraph confirms that both methods yield consistent results, providing a clear understanding of the magnetic field direction around current elements. It concludes with the idea that the total magnetic field due to an entire wire can be found by summing the contributions from each infinitesimal piece or by integrating over the wire.
Mindmap
Keywords
💡Magnetic Field
💡Biot-Savart Law
💡Infinitesimal Element
💡Current (I)
💡Magnetic Field Strength (dB)
💡Cross Product
💡Magnitude
💡Perpendicularity
💡Permeability (μ₀)
💡Right-Hand Rule
Highlights
Current-carrying wires produce a magnetic field around them, a concept explained through the Biot-Savart law.
The Biot-Savart law is used to calculate the strength of the magnetic field created by a tiny element of a wire.
The law involves an infinitesimal length of wire, denoted as 'dl', carrying a current 'i'.
The magnetic field strength at a point is given by the vector equation involving 'dl', current 'i', and the position vector 'r'.
The magnitude of the magnetic field 'db' is calculated using the cross product of 'dl' and 'r', with a constant 'mu naught'.
The magnetic field is inversely proportional to the square of the distance 'r' from the wire element.
The sine of the angle theta between 'dl' and 'r' determines the variation of the magnetic field strength with angle.
The magnetic field is maximum at 90 degrees to the current element and minimum on the axis of the current element.
The constant 'mu naught' is a property of vacuum and has a value of 4 pi x 10^-7 Tm/A.
The direction of the magnetic field can be determined using the right-hand rule or by evaluating the cross product.
The magnetic field direction is always perpendicular to the current element, differing from electric fields around point charges.
The Biot-Savart law is analogous to Coulomb's law in terms of constants and inverse square relationships but includes a sine term.
The magnetic field's dependence on the angle between the current element and the position vector is a key distinction from electric fields.
The permeability of vacuum, 'mu naught', is similar in concept to permittivity but pertains to magnetic fields instead of electric fields.
The Biot-Savart law is applicable to point current elements and requires integration to calculate the total magnetic field for an entire wire.
The video promises future demonstrations of applying the Biot-Savart law to calculate the total magnetic field for complex wire configurations.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: