Unit III: Lec 2 | MIT Calculus Revisited: Single Variable Calculus
TLDRThis video script from an MIT OpenCourseWare lecture explores the concept of inverse trigonometric functions, focusing on the sine function. The instructor discusses the limitations of defining inverse sine due to its non-1:1 nature but introduces a method to restrict the domain for a 1:1 relationship, creating 's sub 0' of x. The lecture delves into calculus applications, including deriving the inverse sine function and using trigonometric identities to simplify the process. It also touches on the historical context and practical applications of trigonometry beyond surveying.
Takeaways
- 📚 The video script is an educational lecture discussing the concept of inverse trigonometric functions, emphasizing the importance of understanding these functions in various mathematical applications.
- 🔍 The sine function 'y = sine x' is highlighted as not being one-to-one (1:1) over its entire domain, which complicates defining an inverse function due to the infinite number of 'x' values for each 'y' value between -1 and 1.
- 📉 To address the non-1:1 nature of the sine function, the lecturer suggests restricting the domain to specific intervals, such as from -π/2 to π/2, to create 1:1 functions that can be inverted.
- 🔑 The concept of 'principal values' is introduced as a way to define inverse trigonometric functions by restricting the range of the output to specific intervals, which is a common practice in textbooks.
- 🤔 The lecture clarifies the process of inverting a function by reflecting the graph over the 45-degree line or rotating and flipping the graph, which is essential for understanding inverse functions.
- 📈 The derivative of the inverse sine function is derived in the lecture, showing that it can be expressed as 1 over the square root of (1 - x squared), which is a direct application of the chain rule in calculus.
- 📚 The inverse trigonometric functions are shown to be useful in solving integrals, such as the integral of 1 over the square root of (1 - x squared), which is related to the inverse sine function.
- 📐 A geometric interpretation is provided using right triangles to solve integrals involving the sum or difference of squares, demonstrating the practical application of trigonometry in calculus.
- 🔄 The video emphasizes the importance of understanding the inverse trigonometric functions not only for their own sake but also for their role in solving broader mathematical problems.
- 📝 The script concludes with a reminder that the language and notation of inverse functions can be challenging, but with practice, it becomes a powerful tool in mathematical analysis.
- 💡 Lastly, the lecture encourages students to explore the material further on their own, highlighting the self-learning aspect of understanding inverse trigonometric functions.
Q & A
What is the main topic of the lecture?
-The main topic of the lecture is the inverse trigonometric functions.
Why can't the inverse sine function be defined for the entire domain of the sine function?
-The inverse sine function can't be defined for the entire domain of the sine function because the sine function is not one-to-one (1:1) over its entire domain, as it has multiple 'x' values for a single 'y' value between -1 and 1.
How does the lecturer suggest dealing with the non-1:1 nature of the sine function to define its inverse?
-The lecturer suggests dealing with the non-1:1 nature of the sine function by breaking it down into a union of 1:1 functions, each defined over a restricted domain where the sine function is 1:1.
What is the term used to describe the restricted range of 'y' values for which the inverse sine function is defined?
-The term used to describe the restricted range of 'y' values for which the inverse sine function is defined is 'principal values'.
What is the domain of the function 's sub 0' of x as defined by the lecturer?
-The domain of the function 's sub 0' of x is the closed interval from -π/2 to π/2.
How can the inverse sine function be graphically obtained from the sine function?
-The inverse sine function can be graphically obtained from the sine function by reflecting the graph of the sine function with respect to the line y=x, which involves rotating the graph by 90 degrees and then flipping it over the x-axis.
What is the derivative of the inverse sine function with respect to 'x'?
-The derivative of the inverse sine function with respect to 'x' is 1 over the square root of 1 minus 'x squared' (1/√(1 - x^2)).
How does the lecturer explain the relationship between the derivative of the inverse sine function and the cosine function?
-The lecturer explains that since the derivative of sine with respect to 'y' is cosine, and in the 1:1 case, 'dx/dy' and 'dy/dx' are reciprocals, the derivative of the inverse sine function with respect to 'x' is 1 over cosine 'y', which simplifies to 1/√(1 - x^2) given the range of 'y'.
What is the significance of the inverse trigonometric functions in calculus?
-The significance of the inverse trigonometric functions in calculus is that they allow for the simplification of calculus results about derivatives and integrals by restating them in terms of ordinary trigonometric functions.
How does the lecturer illustrate the connection between trigonometric functions and geometry?
-The lecturer illustrates the connection between trigonometric functions and geometry by using a right triangle to express the integral of 1/√(1 - x^2) in terms of trigonometric functions, showing that the integral evaluates to the inverse sine function.
What is the principal value range for the inverse cosine function as discussed in the lecture?
-The principal value range for the inverse cosine function is between 0 and π, as it is the range for 'y' values when 'x' equals cosine 'y'.
How does the lecturer use the concept of a right triangle to solve the integral of 1/√(1 - x^2)?
-The lecturer uses the concept of a right triangle by setting the hypotenuse to 1, one side to 'x', and the other side to √(1 - x^2), then expressing the integral in terms of the trigonometric function sine and cosine, which simplifies the integral to an expression involving the angle 'theta' whose sine is 'x'.
Outlines
📚 Introduction to Inverse Trigonometric Functions
The paragraph introduces the concept of inverse trigonometric functions, emphasizing the need for a function to be one-to-one (1:1) to have an inverse. The speaker explains that while the sine function is not naturally 1:1, it can be restricted to certain intervals where it is 1:1, allowing for the definition of inverse functions. The concept of 's sub 0 of x' is introduced as a restricted version of the sine function, and the importance of domain in defining functions is highlighted. The paragraph sets the stage for a deeper exploration of inverse trigonometric functions.
🔍 Exploring the Inverse Sine Function and Principal Values
This paragraph delves into the specifics of the inverse sine function, discussing how it can be derived from the sine function by restricting its domain to intervals where it is 1:1. The speaker introduces the idea of 'principal values' and explains how texts often define the inverse sine function with a restricted range for 'y', between -π/2 and π/2. The paragraph also touches on the historical approach to multi-valued functions in older mathematics and how modern definitions require a 1:1 correspondence for inverse functions to exist.
📈 Deriving the Derivative of the Inverse Sine Function
The speaker demonstrates how to find the derivative of the inverse sine function with respect to 'x'. By using the relationship between the sine function and its inverse, and understanding the reciprocal relationship between 'dx/dy' and 'dy/dx' on a 1:1 curve, the derivative is found to be '1 over the square root of 1 - x squared'. The paragraph also discusses the importance of the restricted range for 'y' in ensuring the cosine function's positivity, which simplifies the derivative to a single-valued expression.
🌐 The Role of Trigonometry in Solving Non-Trigonometric Problems
The paragraph highlights the utility of inverse trigonometric functions in solving calculus problems that may not initially appear to involve trigonometry. The speaker provides an example of an integral problem whose solution involves the inverse sine function, even though the problem statement does not explicitly mention trigonometric functions. This underscores the importance and broad applicability of inverse trigonometric functions in various mathematical contexts.
🎓 Trigonometric Substitution in Integration
This paragraph introduces the technique of trigonometric substitution as a method for solving integrals, particularly those involving the square root of a difference of squares. The speaker illustrates how a right triangle can be used to express the integral in terms of trigonometric functions, leading to a simplification that results in the inverse sine function. The technique is presented as a powerful tool for integrating certain types of functions and is connected to the broader theme of inverse trigonometric functions.
🔄 Understanding Inverse Trigonometric Identities and Derivatives
The speaker discusses the process of understanding and deriving identities and derivatives for inverse trigonometric functions. They provide an example of how to visualize and understand the identity involving the inverse cosine and inverse sine functions. The paragraph emphasizes the importance of being familiar with the language of inverse functions and how reducing complex identities to simpler terms can aid in understanding and calculation. The speaker also touches on the derivative of the inverse cosine function, showing how it can be derived from previously established results.
👋 Conclusion and Encouragement for Self-Study
In the concluding paragraph, the speaker summarizes the importance of inverse trigonometric functions and encourages students to explore the material further on their own. They remind students that the key to understanding these functions lies in recognizing the necessity of a 1:1 relationship for defining inverse functions and that the language of 'principal values' is simply a way to ensure this. The speaker also acknowledges the support provided by the Gabriella and Paul Rosenbaum Foundation for the publication of the video and invites viewers to support MIT OpenCourseWare.
Mindmap
Keywords
💡Creative Commons license
💡MIT OpenCourseWare
💡inverse function
💡trigonometric functions
💡1:1 function
💡domain
💡range
💡principal values
💡derivative
💡integral
💡trigonometric substitution
Highlights
Introduction to the concept of inverse trigonometric functions and their significance in mathematics.
Explanation of why the sine function cannot have a true inverse due to its non-1:1 nature.
Technique to redefine the sine function as 's sub 0 of x' to create a 1:1 function within a restricted domain.
Discussion on the importance of domain specification when defining functions to ensure they are 1:1.
Introduction of different domains for sine function to create multiple 1:1 functions, such as 's sub 1 of x' and 's sub -1 of x'.
The concept of principal values in inverse trigonometric functions to address the issue of multi-valuedness.
Derivation of the derivative of the inverse sine function using the relationship between the derivative of sine and cosine.
Explanation of how to express the derivative of inverse sine in terms of x using the Pythagorean identity.
The application of inverse trigonometric functions in solving integrals, specifically the integral of 1 over the square root of 1 minus x squared.
Introduction of trigonometric substitution as a method to solve integrals involving sums or differences of squares.
Demonstration of how to use a right triangle to express the integral of the derivative of inverse sine in terms of theta.
The significance of the range of principal values in ensuring the positivity of cosine within the inverse sine function.
The use of trigonometric identities to simplify the expression for the derivative of inverse cosine in terms of inverse sine.
The importance of understanding the language and notation of inverse functions for calculus applications.
The caution against being misled by the term 'principal values' and the necessity of viewing multi-valued functions as unions of 1:1 curves.
Encouragement for students to explore and understand the material on inverse trigonometric functions independently.
The conclusion emphasizing the comprehensive study of inverse trigonometric functions based on previous lectures.
Transcripts
Browse More Related Video

Derivative of Inverse Trig Functions via Implicit Differentiation
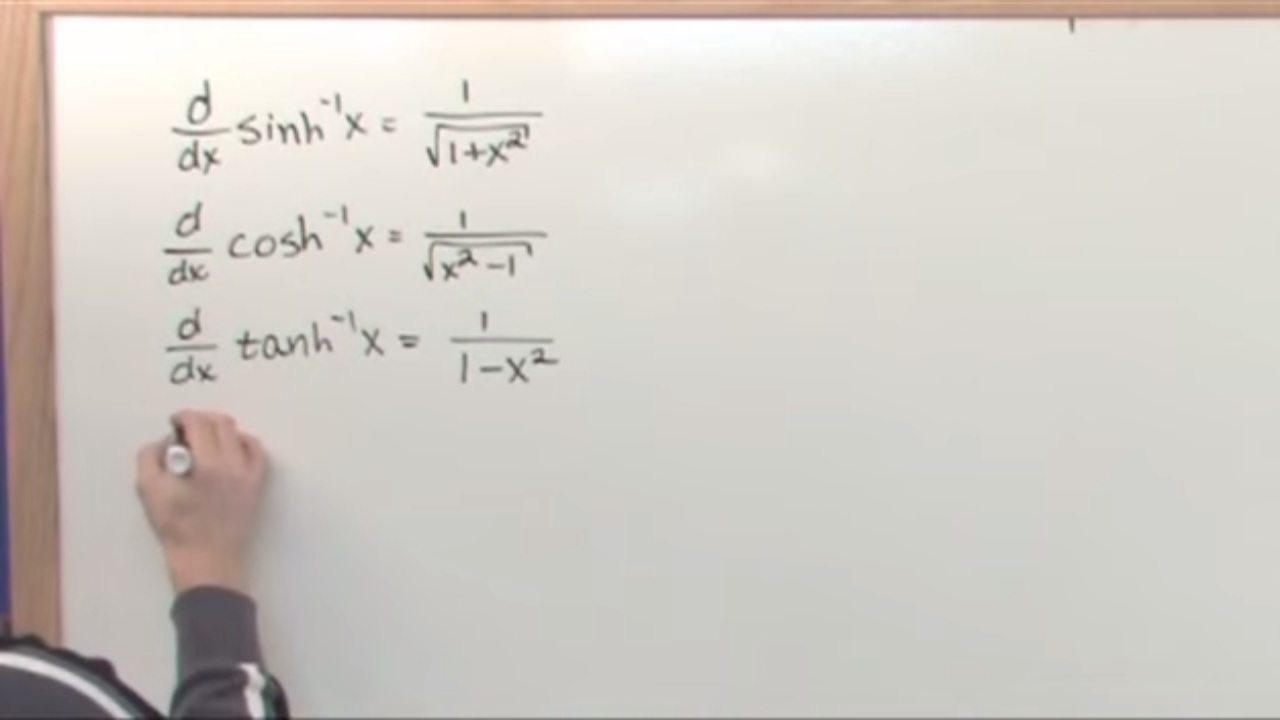
Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

Derivatives of Inverse Functions
Unit I: Lec 3 | MIT Calculus Revisited: Single Variable Calculus
How to Solve Basic Inverse Trigonometric Functions (Precalculus - Trigonometry 20)
Function inverses example 2 | Functions and their graphs | Algebra II | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: