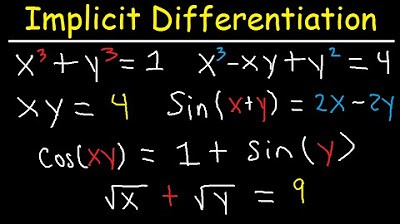Implicit Differentiation Day 1
TLDRThis educational video script introduces the concept of finding derivatives of implicitly defined functions, contrasting them with explicitly defined functions. It emphasizes the importance of identifying the independent variable and correctly applying the chain rule when differentiating with respect to that variable, which could be time or another variable. The script provides step-by-step examples of differentiating complex equations with respect to various variables, including algebraic manipulation to solve for dy/dx, demonstrating the process with equations involving trigonometric functions.
Takeaways
- 📚 The lesson is about finding derivatives of functions that are not in the form of \( y = f(x) \) and understanding the difference between explicitly and implicitly defined functions.
- 🔍 Explicitly defined functions have a clear \( y = \) or \( f(x) = \) form, while implicitly defined functions do not have \( y \) or \( x \) isolated and can have variables intertwined.
- 👀 When differentiating implicitly defined functions, it's crucial to treat every \( y \) as a function of another variable, often \( x \), and apply the chain rule when necessary.
- ⏳ If the derivative is with respect to a variable other than \( x \) or \( y \), such as time (\( t \)), both \( x \) and \( y \) are considered functions of that variable, necessitating the use of the chain rule.
- 📉 The process involves differentiating each term in the equation with respect to the chosen variable and multiplying by the derivative of the function that the variable represents.
- 📝 An example given was differentiating an implicit equation with respect to time, showing how to apply the chain rule to each term involving \( y \) and \( x \).
- 🔢 The script demonstrates algebraic manipulation to solve for \( \frac{dy}{dx} \) from the differentiated equation, emphasizing the need to isolate terms involving \( \frac{dy}{dx} \).
- 📌 It's highlighted that the independent variable determines whether or not to use the chain rule, and in the case of \( x \) being the independent variable, the chain rule is not needed.
- 📈 The script explains that derivatives involving both \( x \) and \( y \) require plugging in both coordinates to find the slope of the curve at a particular point.
- 📚 Additional examples are provided, including using the product rule for differentiation and solving for \( \frac{dy}{dx} \) algebraically.
- 📝 The final takeaway is the importance of recognizing the independent variable in the context of differentiation and correctly applying the chain rule or other differentiation techniques.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is how to find derivatives of functions that are not in the form of y = f(x), specifically dealing with implicitly defined functions and using the chain rule when necessary.
What is the difference between an explicitly defined function and an implicitly defined function?
-An explicitly defined function is one where y is expressed as an equation in terms of x, like y = f(x). An implicitly defined function does not have y isolated; instead, x's and y's are intermingled throughout the equation without being solved for y.
Why is it necessary to assume that every y is a function of x when differentiating implicitly defined functions?
-It is necessary to assume that every y is a function of x because in an implicit function, y is not isolated and can depend on x in complex ways. This assumption allows us to apply the chain rule correctly when differentiating with respect to x.
Can derivatives be taken with respect to variables other than x?
-Yes, derivatives can be taken with respect to variables other than x. For example, if the independent variable is time, both x and y would be functions of time, and the chain rule would be used in the differentiation process.
What is the chain rule, and when is it used in the context of the video script?
-The chain rule is a fundamental principle in calculus for differentiating composite functions. In the context of the video script, it is used when differentiating implicitly defined functions with respect to an independent variable other than x, such as time or another variable.
How does the process of differentiating with respect to time differ from differentiating with respect to x?
-When differentiating with respect to time, every term in the equation, including y and x, is considered to be a function of time, and derivatives are taken with respect to time (dy/dt or dx/dt). In contrast, when differentiating with respect to x, x is the independent variable, and no chain rule is applied to it directly.
What is the purpose of algebraically solving for dy/dx in the context of the video script?
-The purpose of algebraically solving for dy/dx is to isolate the derivative of y with respect to x, which allows us to understand the slope of the curve at any point in the xy-plane, given the x and y coordinates.
How does the process of differentiating an equation change when the independent variable is not x?
-When the independent variable is not x, we must assume that both x and y can be written as functions of the new independent variable, and we apply the chain rule to find the derivatives of y and x with respect to that variable.
What is the significance of the product rule in the context of the video script?
-The product rule is significant in the context of the video script when differentiating terms that are products of two functions, such as in the case of 2x * (dy/dx), where the product rule is used to differentiate the product of x and dy/dx.
Can the derivative of a function involving trigonometric functions be simplified using trigonometric identities?
-Yes, the derivative of a function involving trigonometric functions can often be simplified using trigonometric identities, such as expressing the derivative in terms of tangent functions, as shown in the script with dy/dx = tan(x) * tan(y).
Outlines
📚 Understanding Implicit Functions and Derivatives
This paragraph introduces the concept of derivatives for functions that are not explicitly defined in the form y = f(x). It distinguishes between explicitly defined functions (where y is directly expressed as a function of x) and implicitly defined functions (where x and y are intertwined in a way that y is not directly solved for). The speaker emphasizes the need to treat every y as a function of x when differentiating implicitly defined functions. They illustrate this with an example of differentiating an equation with respect to time, using the chain rule to account for the derivatives of y and x with respect to time. The explanation also covers the process of differentiating with respect to a variable other than x, such as w, and how to apply the chain rule in such cases.
🔍 Solving for dy/dx Algebraically
The speaker continues by demonstrating how to algebraically solve for the derivative dy/dx in the context of implicitly defined functions. They differentiate an equation with respect to x, treating x as the independent variable. The process involves isolating terms containing dy/dx on one side of the equation and using algebraic manipulation to solve for dy/dx. The speaker shows how to rearrange the terms, factor out dy/dx, and then divide through to isolate dy/dx. They provide examples of solving for dy/dx in different scenarios, including when the equation involves trigonometric functions. The explanation highlights the importance of recognizing the independent variable and correctly applying the chain rule or product rule as needed.
Mindmap
Keywords
💡Derivative
💡Explicitly Defined Function
💡Implicitly Defined Function
💡Chain Rule
💡Independent Variable
💡Differentiation with Respect to
💡Product Rule
💡Algebraic Manipulation
💡Slope
💡Trigonometric Functions
💡Tangent
Highlights
Introduction to the concept of derivatives of functions not in the form of y = f(x).
Difference between explicitly defined functions (y = f(x)) and implicitly defined functions.
Assumption that every y in an implicit function is a function of x when taking derivatives.
Use of the chain rule when derivatives are taken with respect to a variable other than x or y.
Differentiation of an implicit equation with respect to time, illustrating the chain rule.
Explanation of how to differentiate with respect to a variable other than x or y, such as w.
Process of differentiating an equation with respect to x, using algebra to solve for dy/dx.
Rearranging the differentiated equation to isolate terms involving dy/dx.
Factoring out dy/dx to simplify the equation and solve for it.
Derivative as a function of both x and y, and how to interpret it in terms of slope.
Differentiation of an equation involving trigonometric functions, using the product rule.
Isolating dy/dx in an equation involving trigonometric functions and solving for it.
Final expression for dy/dx in terms of trigonometric functions.
Alternative form of the derivative expression using tangent functions.
Importance of knowing the independent variable in the differentiation process.
Practical application of derivatives in finding the slope of a curve at a given point.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: