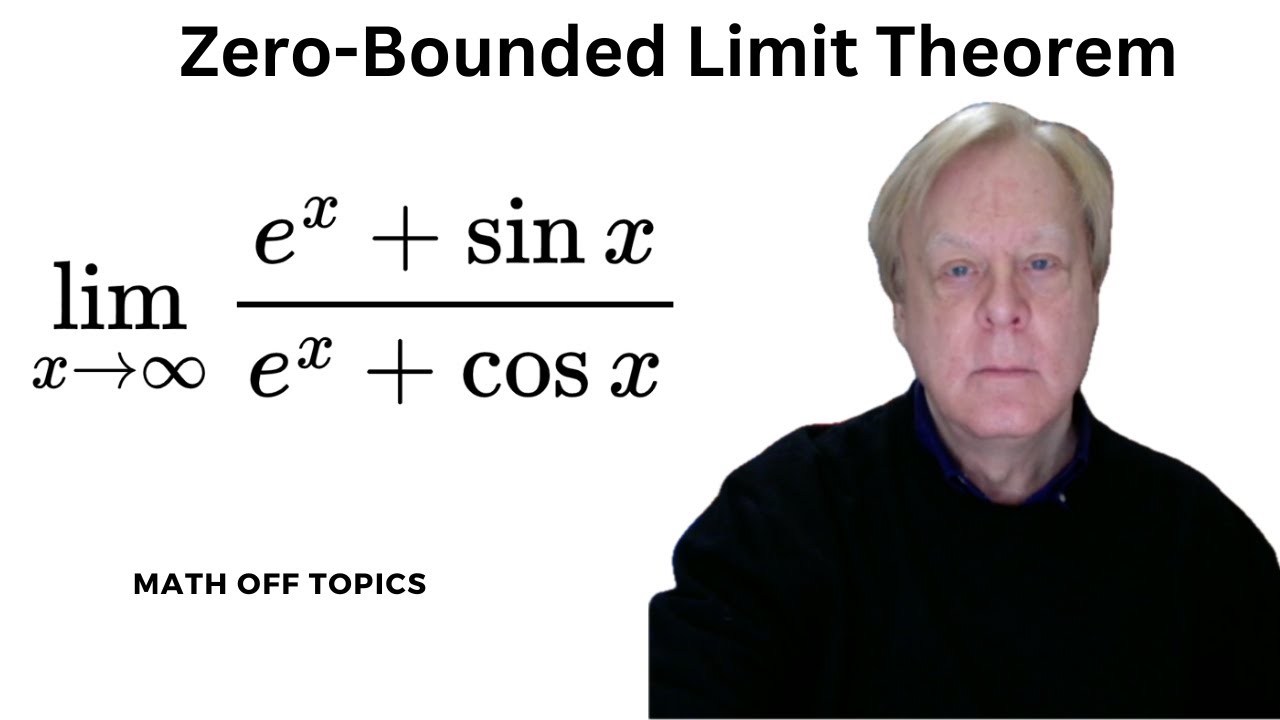
finally 0^0 approaches 0 after 6 years!
TLDRIn this video, the creator presents a unique example of a limit with an indeterminate form of zero to the zero power, which surprisingly approaches zero. The example involves a complex expression with the base approaching zero as the exponent approaches infinity, using the square root of (x + 1) minus the square root of x, raised to the power of 1 over the natural logarithm of the natural logarithm of x. Through a series of mathematical manipulations, including conjugate multiplication and application of L'Hôpital's rule, the presenter successfully demonstrates that the limit equals zero, providing a rare and intriguing insight into limits and their behavior.
Takeaways
- 📚 The video provides a unique example of a limit with an indeterminate form of '0 to the power of 0', concluding that the answer is zero.
- 🔍 The presenter has been seeking this example since 2017 and expresses a sense of achievement in finally finding it.
- 🧩 The example involves a complex limit calculation with the base approaching zero and the exponent approaching zero as x approaches infinity.
- 📉 The base of the limit is constructed using the expression 'square root of (x + 1) minus square root of x', which simplifies to a form that approaches zero.
- 📈 The exponent is carefully chosen as '1 over the natural logarithm of the natural logarithm of x', which approaches zero as x approaches infinity.
- 🤔 The script emphasizes the importance of not just assuming the limit is zero without showing the work, highlighting the need for a rigorous approach.
- 🔧 The presenter uses the conjugate multiplication technique to simplify the expression inside the limit, which is a common method in calculus to deal with square roots.
- 📌 The script mentions the use of L'Hôpital's rule, which is applicable in situations involving 'infinity over infinity', to solve the limit.
- 📚 The video script includes a step-by-step walkthrough of taking natural logarithms and differentiating to apply L'Hôpital's rule effectively.
- 📉 The differentiation process simplifies the expression to a point where the limit can be evaluated as negative infinity, which, when exponentiated, results in zero.
- 🎯 The final result is that the limit of the expression as x approaches infinity is zero, which is a significant conclusion in the context of limits with indeterminate forms.
- 💡 The presenter's thought process is shared, explaining how the idea for the base and exponent was conceived to achieve a limit that approaches zero.
Q & A
What is the main topic of the video script?
-The main topic of the video script is to provide an example of a limit with an indeterminate form of '0 to the power of 0' and to demonstrate that the result approaches zero.
Why might some viewers find the example in the script controversial?
-Some viewers might find the example controversial because the base is set to zero, which is typically not allowed in mathematical operations, making it seem like 'cheating'.
What is the function used in the video to approach the indeterminate form '0 to the power of 0'?
-The function used is the limit as X approaches infinity of (square root of (x + 1) - square root of x) raised to the power of (1 over the natural log of the natural log of x).
How does the script verify that the base of the function approaches zero?
-The script verifies that the base approaches zero by multiplying the numerator and denominator by the conjugate of the expression, simplifying, and showing that the limit results in 1 over infinity, which equals zero.
What is the approach used to show that the exponent of the function approaches zero?
-The script takes the natural log of both sides of the exponent, uses properties of logarithms, and shows that as X approaches infinity, the exponent approaches 1 over infinity, which equals zero.
Why does the script multiply by the conjugate of the expression?
-Multiplying by the conjugate is a technique used to simplify expressions involving square roots and to eliminate the indeterminate form of infinity minus infinity.
What is the significance of the script's use of the natural logarithm function?
-The natural logarithm function is used to transform the exponent into a form that can be more easily analyzed and to apply the properties of logarithms to simplify the expression.
What is the L'Hôpital's Rule mentioned in the script, and how is it applied?
-L'Hôpital's Rule is a method for solving limits of the indeterminate forms '0/0' and '∞/∞' by differentiating the numerator and denominator until a determinate form is reached. The script applies it by differentiating the top and bottom of the fraction until the limit can be determined.
How does the script ensure the limit of the function is not affected by the 'plus one' in the square root?
-The script shows that as X approaches infinity, the 'plus one' becomes insignificant compared to the rest of the expression, and thus does not affect the limit.
What is the final result of the limit presented in the script?
-The final result of the limit is zero, as the script demonstrates that both the base and the exponent approach zero in their respective ways.
How does the script justify the use of infinity and zero in the context of limits?
-The script justifies the use of infinity and zero by showing that in the context of limits, these values can represent the behavior of functions as they approach certain values, and by using mathematical properties to simplify expressions to determinate forms.
Outlines
📚 Introduction to the Zero to the Zero Power Limit Example
The speaker introduces a mathematical example they've been eager to demonstrate since 2017, involving a limit with an indeterminate form of zero to the zero's power. They aim to show that the result of this limit is zero. The example involves a complex expression with the base approaching zero and the exponent approaching zero, using the expression 'square root of (x + 1) - square root of x' raised to the power of '1 over the natural log of the natural log of x'. The speaker promises to verify the components of this expression later in the explanation.
🔍 Deep Dive into the Limit Calculation Process
The speaker delves into the process of calculating the limit, starting with the expression inside the power, which simplifies to an indeterminate form of infinity minus infinity. They then multiply the numerator and denominator by the conjugate to simplify the expression further. The speaker explains the use of the natural logarithm to handle the limit of the exponent and applies L'Hôpital's rule to differentiate the numerator and denominator, simplifying the expression to a form that can be evaluated as x approaches infinity. The process involves careful manipulation of the terms and an understanding of the behavior of logarithms and exponents at infinity.
🎯 Conclusion of the Zero to the Zero Power Limit
The speaker concludes the calculation by applying L'Hôpital's rule to the simplified expression, breaking it down into two separate limits and evaluating each. They find that the first limit simplifies to negative infinity, and the second limit evaluates to infinity. Multiplying these together, they arrive at a negative infinity result for the natural logarithm of L, which, when exponentiated, yields a base of zero. This confirms the initial claim that the limit of zero to the zero's power is zero, completing the complex example and providing a satisfying resolution to the problem.
Mindmap
Keywords
💡Limit
💡Indeterminate Form
💡Zero to the Power of Zero
💡Square Root
💡Natural Logarithm
💡Infinity
💡Conjugate
💡L'Hôpital's Rule
💡Continuous Function
💡Derivative
💡Exponentiation
Highlights
The video presents a unique example of a limit with an indeterminate form of 0 to the power of 0, resulting in zero.
The creator has been seeking this example since 2017, emphasizing its significance.
The base of the example is made to approach zero using the expression square root of (x + 1) minus square root of x.
The exponent is carefully chosen to approach zero as well, using 1 over the natural log of the natural log of x.
The video demonstrates the verification of the base approaching zero by substituting infinity and simplifying the expression.
The use of the conjugate to simplify the expression and demonstrate the approach to zero is explained.
The video clarifies the importance of differentiating between various approaches to infinity and their impact on the result.
The process of taking the natural log of both sides to simplify the limit expression is shown.
The application of L'Hôpital's rule to an 'infinity over infinity' situation is demonstrated in the video.
Differentiation of the numerator and denominator to apply L'Hôpital's rule is detailed step by step.
The video shows the simplification process by canceling terms and finding a common denominator.
The concept of breaking apart the limit into a product of limits is introduced, provided they all exist.
The limits of individual components as x approaches infinity are calculated, leading to a conclusion of negative infinity.
The video concludes that the natural log of L (the limit) is equal to negative infinity, which is a pivotal point in the example.
The final step involves raising e to the power of negative infinity, resulting in the limit approaching zero.
The creator reflects on the thought process behind choosing the base and exponent to achieve the desired limit result.
The video concludes with the successful demonstration of a 0 to the power of 0 limit approaching zero, a long-sought example.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: