Verifying Trigonometric Identities Using Half Angle Formulas
TLDRThis video script delves into verifying trigonometric identities involving half-angle formulas. It demonstrates the proof of three identities: sine squared over 2 equating to the difference between cosecant and cotangent divided by 2 cosecant, cosine squared over 2 being equal to sine plus tangent over 2 tangent, and tangent over 2 being equivalent to tangent over secant plus one. The script uses algebraic manipulation and trigonometric conversions to validate each identity, providing a clear and concise explanation for students of trigonometry.
Takeaways
- 📚 The video script discusses verifying trigonometric identities involving half-angle formulas.
- 🔍 The first identity to be proven is that \( \sin^2(x/2) = \frac{\csc(x) - \cot(x)}{2\csc(x)} \).
- 📐 The half-angle identity for sine is used as a starting point, which is \( \sin(x/2) = \pm\sqrt{\frac{1 - \cos(x)}{2}} \).
- 📈 Squaring both sides of the half-angle identity for sine is necessary to derive \( \sin^2(x/2) \).
- 🌐 The expression \( 1 - \cos(x) \) over 2 is manipulated by multiplying the numerator and denominator by \( \frac{1}{\sin(x)} \) to fit the identity.
- 🔑 The terms \( \csc(x) \) and \( \cot(x) \) are identified as the cosecant and cotangent functions of x, respectively.
- 📉 The second identity to be proven is \( \cos^2(x/2) = \frac{\sin(x) + \tan(x)}{2\tan(x)} \).
- 📝 The half-angle identity for cosine is \( \cos(x/2) = \pm\sqrt{\frac{1 + \cos(x)}{2}} \), which is squared to eliminate the square root.
- 🔄 The expression is then manipulated by multiplying the numerator and denominator by \( \frac{\sin(x)}{\cos(x)} \) to match the identity.
- 📌 The terms \( \tan(x) \) and \( \sin(x) \) are identified in the process of proving the identity.
- 📝 The third identity to be proven is \( \tan(x/2) = \frac{\tan(x)}{\sec(x) + 1} \).
- 🔍 The script uses the form \( \tan(x/2) = \frac{\sin(x)}{1 + \cos(x)} \) for its simplicity and direct relation to the identity.
- 📈 By multiplying the numerator and denominator by \( \frac{1}{\cos(x)} \), the expression is transformed to match the identity.
- 🔑 The terms \( \sec(x) \) and \( \tan(x) \) are used to complete the proof of the identity.
Q & A
What is the purpose of the video script provided?
-The video script is focused on proving trigonometric identities related to half-angle formulas and their applications in simplifying expressions involving sine, cosine, tangent, cosecant, and cotangent.
What is the first identity that the script attempts to prove?
-The first identity is that sine squared of x over 2 is equal to the expression (cosecant x - cotangent x) divided by 2 times cosecant x.
How does the script use the half-angle identity for sine to prove the first identity?
-The script squares the half-angle identity for sine, which is ±√(1 - cos x) / 2, to eliminate the square root and simplify to 1 - cos x / 2, which is then manipulated to match the form of the identity to be proved.
What is the second identity that the script discusses?
-The second identity is that cosine squared of x divided by 2 is equal to (sine x + tangent x) / (2 tangent x).
How does the script square the half-angle identity for cosine to prove the second identity?
-The script uses the half-angle identity for cosine, which is √(1 + cos x) / 2, and squares both sides to eliminate the square root, resulting in (1 + cos x) / 2, which is then manipulated to match the form of the identity to be proved.
What is the third identity that the script aims to verify?
-The third identity is that tangent of x divided by 2 is equal to tangent x divided by (secant x + 1).
What form of tangent half-angle identity does the script use for the third identity?
-The script uses the form sine x / (1 + cos x) for the half-angle identity of tangent, which simplifies the process of proving the third identity.
Why is the script multiplying the numerator and denominator by 'one over sine x' in the first identity proof?
-Multiplying by 'one over sine x' is done to convert the expression 1 - cos x / 2 into a form that includes cosecant and cotangent, which are then used to match the identity to be proved.
What is the significance of multiplying the numerator and denominator by 'sine over cosine' in the second identity proof?
-This step is crucial to transform the expression (1 + cos x) / 2 into a form involving sine and tangent, which simplifies to the identity to be proved.
How does the script ensure the transformation of the expressions in the third identity proof?
-The script multiplies the numerator and denominator by 'one over cosine' to convert sine x / (1 + cos x) into an expression involving tangent and secant, which matches the identity to be proved.
What is the common approach used in all three identity proofs in the script?
-The common approach is to start with a known half-angle identity, square both sides to eliminate the square root, and then manipulate the expressions algebraically to match the form of the identity to be proved.
Outlines
📚 Proving Half-Angle Identities for Sine and Cosine
This paragraph introduces the process of verifying trigonometric identities related to half angles. The focus is on proving that \( \sin^2(x/2) = \frac{\csc(x) - \cot(x)}{2\csc(x)} \). The explanation begins with the half-angle identity for sine, which is squared to eliminate the square root and then manipulated algebraically to express sine squared in terms of cosecant and cotangent. The process involves multiplying by the reciprocal of sine to convert the expression into the desired form, ultimately verifying the given identity.
🔍 Demonstrating the Half-Angle Identity for Cosine
The second paragraph continues the theme of half-angle identities by proving that \( \cos^2(x/2) = \frac{\sin(x) + \tan(x)}{2\tan(x)} \). It starts with the half-angle formula for cosine, which includes a square root. The process involves squaring both sides to eliminate the radical and then multiplying the numerator and denominator by \( \sin(x)/\cos(x) \) to simplify the expression. The result is an identity that relates cosine squared to sine and tangent, confirming the initial claim.
📐 Verifying the Tangent Half-Angle Identity
In this paragraph, the script discusses the verification of the tangent half-angle identity, specifically proving that \( \tan(x/2) = \frac{\tan(x)}{\sec(x) + 1} \). The explanation uses a specific form of the tangent half-angle identity that simplifies the expression to a single term in the numerator and two terms in the denominator. The process involves algebraic manipulation to express tangent in terms of sine and cosine, and then further simplifying to match the given identity, thus verifying its correctness.
Mindmap
Keywords
💡Sine squared x/2
💡Cosecant
💡Cotangent
💡Half-angle identity
💡Cosine squared x/2
💡Tangent
💡Secant
💡Trigonometric identity
💡Multiplying by one
💡Reciprocal functions
💡Squaring both sides
Highlights
Introduction to verifying identities using half-angle formulas.
Explanation of the half-angle identity for sine.
Derivation of sine squared x/2 using the half-angle identity.
Steps to square both sides to remove the radical.
Replacement of sine squared with the derived expression.
Multiplication of numerator and denominator by 1/sine x.
Simplification to obtain cosecant and cotangent terms.
Verification of the identity: sine squared x/2 = (cosecant x - cotangent x) / (2 cosecant x).
Introduction to verifying the identity for cosine squared x/2.
Explanation of the half-angle identity for cosine.
Squaring both sides to remove the radical for cosine squared.
Replacement of cosine squared with the derived expression.
Multiplication of numerator and denominator by sine/cosine.
Simplification to obtain tangent and sine terms.
Verification of the identity: cosine squared x/2 = (sine x + tangent x) / (2 tangent x).
Introduction to verifying the identity for tangent x/2.
Explanation of different forms of tangent theta/2.
Selection of the form sine theta / (1 + cosine theta) for verification.
Replacement of tangent x/2 with the selected form.
Multiplication of numerator and denominator by 1/cosine.
Simplification to obtain tangent and secant terms.
Verification of the identity: tangent x/2 = tangent x / (secant x + 1).
Transcripts
Browse More Related Video
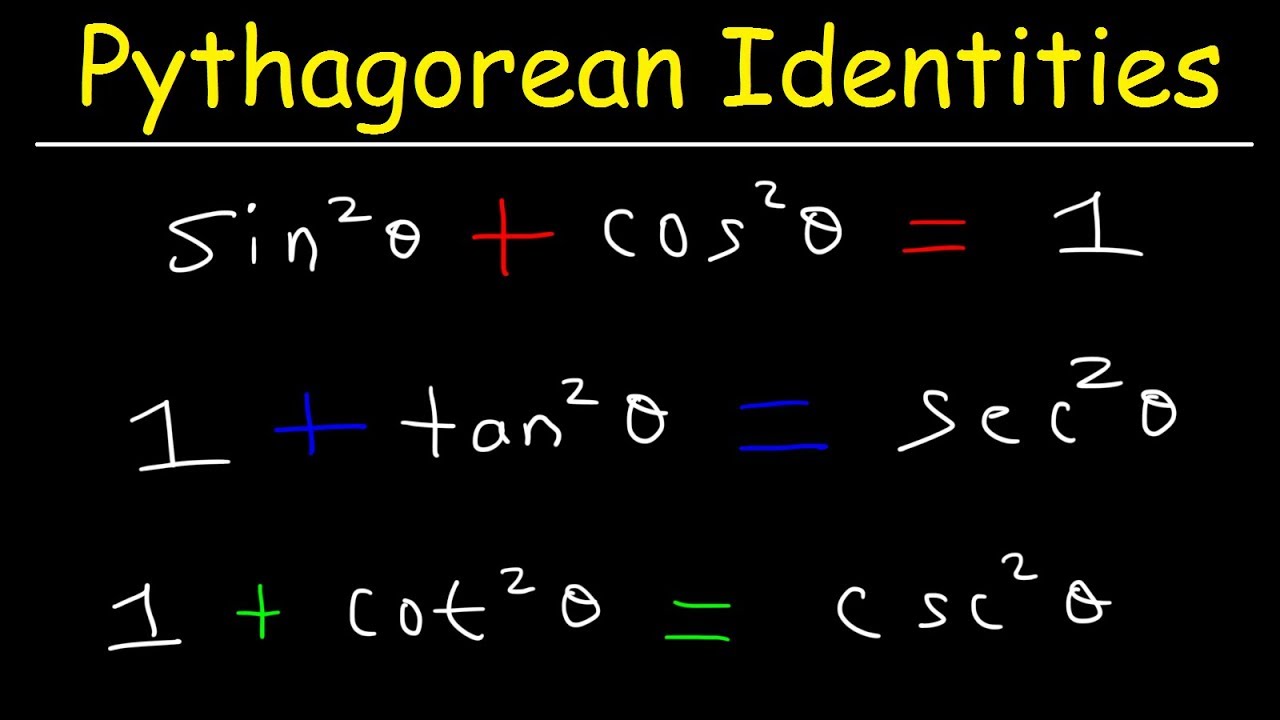
Pythagorean Identities - Examples & Practice Problems, Trigonometry

Trig - 0.4 Fundamental Trig Identities
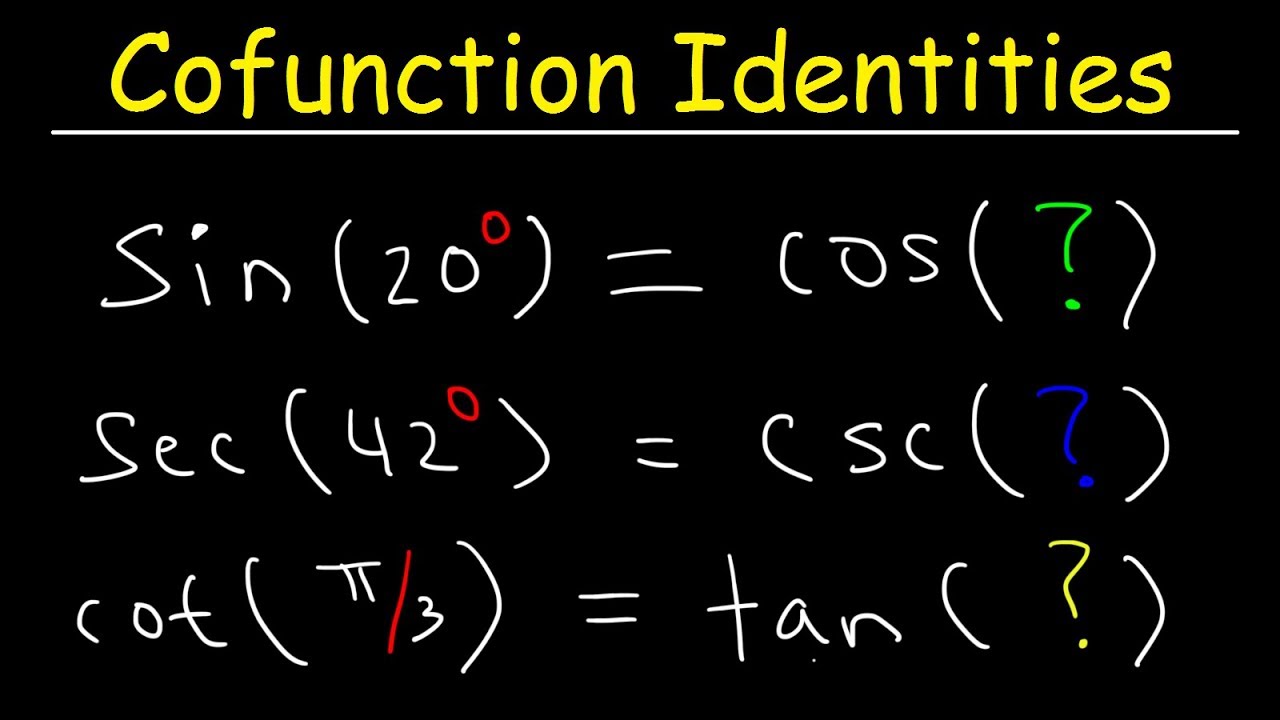
Cofunction Identities Examples & Practice Problems Trigonometry

Integrals: Trig Substitution 2
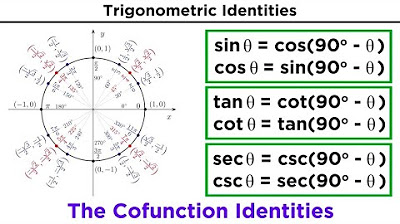
Basic Trigonometric Identities: Pythagorean Identities and Cofunction Identities
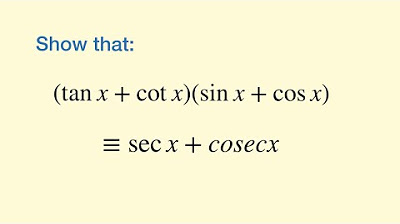
Secant, Cosecant and Cotangent - (2 of 4: Trigonometric Identities)
5.0 / 5 (0 votes)
Thanks for rating: