Lec 31: Stokes' theorem | MIT 18.02 Multivariable Calculus, Fall 2007
TLDRThis educational video script delves into the concept of curl in vector calculus, explaining its significance in measuring the rotation within a fluid flow. It illustrates how curl can be calculated using a determinant and its real-world applications, such as identifying areas of rotation in the Charles River. The script further explores Stokes' theorem, which relates the line integral of a vector field to the double integral of its curl, and discusses the importance of compatible orientations for curves and surfaces. Practical examples and the right-hand rule are used to clarify these mathematical principles, providing a comprehensive understanding of the topic.
Takeaways
- 📚 The script discusses the concept of curl in vector calculus, explaining it as a measure of rotation within a vector field, such as fluid flow.
- 🌀 The curl is defined mathematically as the cross product between the del operator and a vector field, resulting in a vector with specific components indicating the rotation.
- 🔍 The significance of curl is further clarified with examples, such as a constant vector field resulting in zero curl, indicating no rotation.
- 📈 The script differentiates between curl and divergence, noting that while curl measures rotation, divergence measures expansion or stretching in a vector field.
- 🌡️ An example of a vector field representing rotation about the z-axis is given, illustrating how to compute its curl and the resulting implications for angular velocity and axis of rotation.
- 🏗️ The concept of curl is connected to the broader understanding of vector fields, including the potential for fluid dynamics applications, such as identifying areas of swirling water in a river.
- 🔍 The script introduces Stokes' Theorem, which relates the work done by a vector field along a closed curve to a double integral of the curl of the field over a surface bounded by that curve.
- 📐 The importance of choosing compatible orientations for the curve and the surface in Stokes' Theorem is emphasized, with explanations and examples provided to clarify this concept.
- 🤔 The script presents a method for visualizing and remembering the orientation rule using the right-hand rule, which is a common technique in vector calculus.
- 📝 An example calculation using Stokes' Theorem is provided, comparing it to a direct line integral approach and demonstrating the theorem's utility.
- 📘 The script concludes with a discussion on the conditions required for applying Stokes' Theorem, such as the need for the vector field to be continuous and differentiable on the surface.
Q & A
What does the curl of a vector field measure?
-The curl of a vector field measures the rotation or vorticity within the field. If the vector field represents fluid flow, the curl indicates how much rotation is occurring in the fluid, with the direction of the curl corresponding to the axis of rotation and its magnitude being twice the angular velocity.
How is the curl of a vector field mathematically defined?
-The curl of a vector field F is mathematically defined as the cross product between the del operator (∇) and the vector field F. It can be computed by arranging the components of F into a determinant and expanding it to obtain a vector with components (Ry - Qz, Pz - Rx, Qx - Py).
What is the physical interpretation of a zero curl in a fluid flow?
-A zero curl in a fluid flow indicates that there is no rotation happening in the fluid. It suggests a uniform translation or irrotational flow, where particles of the fluid move parallel to each other without any swirling motion.
Can you provide an example of a vector field with zero curl?
-An example of a vector field with zero curl is a constant vector field where everything translates at the same speed. Another example is a field that stretches space along a single axis, such as a field that goes parallel to the x-direction and varies linearly with x (e.g., x*i), which also results in a curl of zero.
What is the difference between curl and divergence in the context of vector fields?
-Curl measures the rotation or vorticity in a vector field, while divergence measures the magnitude of a field's source or sink at a given point. Divergence indicates how much a field is expanding or contracting, and it is not concerned with rotational motion.
What is Stokes' theorem and how does it relate to the curl of a vector field?
-Stokes' theorem states that the work done by a vector field along a closed curve can be replaced by a double integral of the curl of the vector field over a suitably chosen surface bounded by that curve. It connects the line integral of a vector field around a boundary to the flux of the curl of the field through a surface.
How does the orientation of a curve and a surface affect the application of Stokes' theorem?
-The orientation of a curve and a surface must be compatible when applying Stokes' theorem. If the curve is oriented in one direction, the normal vector to the surface should point in a way that is consistent with the right-hand rule, which involves walking along the curve with the surface to one side and determining the direction of the normal vector.
What is the right-hand rule, and how does it help in determining the direction of the normal vector in Stokes' theorem?
-The right-hand rule is a common mnemonic used to determine the direction of the normal vector when the orientation of a curve and a surface is being considered. By pointing the thumb of your right hand along the curve and the index finger towards the surface, the middle finger points in the direction of the normal vector.
Can you provide an example of applying Stokes' theorem to calculate the work done by a vector field?
-An example given in the script is calculating the work done by the vector field F = (z, x, y) around the unit circle in the x, y plane. Instead of directly computing the line integral, one could use Stokes' theorem to compute the flux of the curl of F through a chosen surface, such as a flat disk or a paraboloid, bounded by the unit circle.
What is the significance of the curl being related to the angular velocity in the context of fluid dynamics?
-In fluid dynamics, the curl being related to angular velocity is significant because it quantifies the local rotational motion of the fluid. This can be used to analyze phenomena like vortices and eddies, where the curl would be non-zero, indicating regions of swirling or circulating flow.
Why is it important for a vector field to be continuous and differentiable for Stokes' theorem to be applicable?
-For Stokes' theorem to be applicable, the vector field must be continuous and differentiable everywhere on the surface S. This ensures that the mathematical operations involved in calculating the curl and the flux are well-defined and that the theorem's assumptions about the field's behavior hold true.
Outlines
📚 Introduction to MIT OpenCourseWare and Vector Field Concepts
The script opens with an introduction to MIT OpenCourseWare, highlighting its mission to provide free, high-quality educational resources, and invites donations to support the initiative. It then delves into a physics lecture about vector fields, specifically the concept of curl, which measures the rotation within a fluid. The determinant method for calculating curl is explained, along with its physical interpretation in terms of angular velocity and axis of rotation. Examples are given to illustrate the concept, such as a constant vector field with zero curl indicating no rotation, and a field that stretches along the x-axis also having zero curl, distinguishing it from divergence, which measures expansion.
🌀 Exploring Curl with Fluid Dynamics and Vector Field Analysis
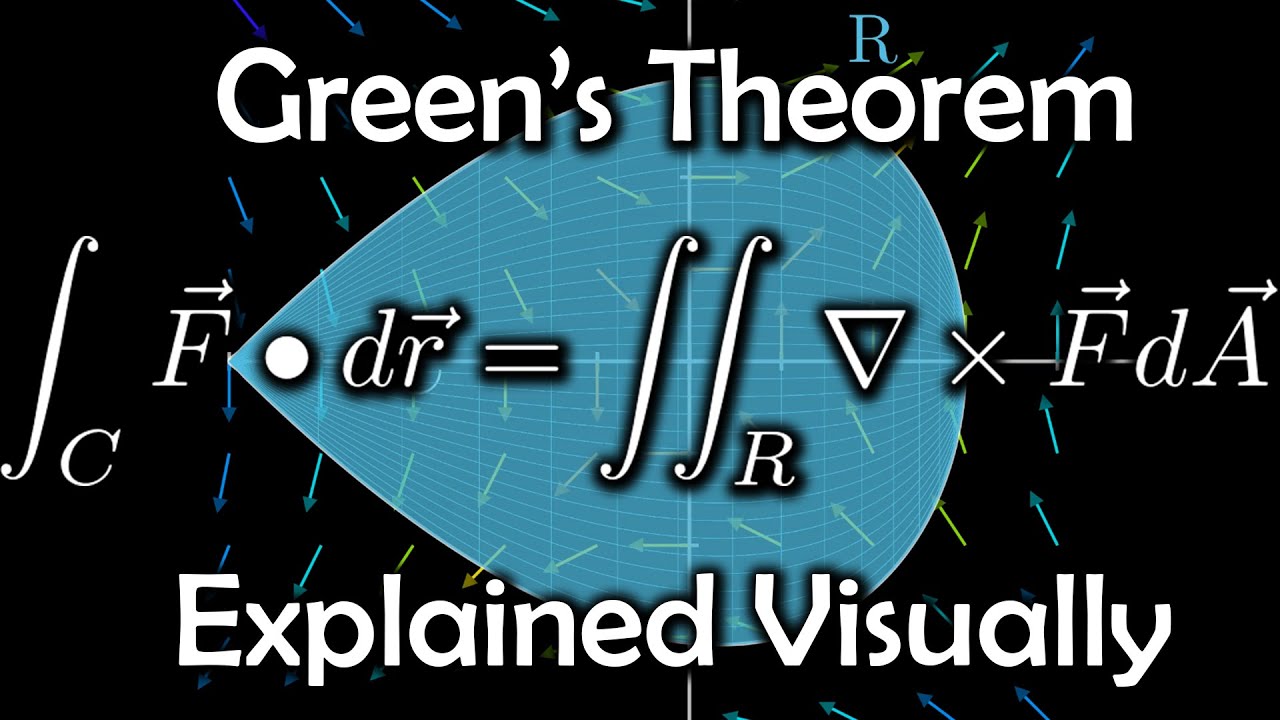
This paragraph continues the discussion on curl, using the Charles River as a real-world example to describe how water flow can have both a general direction and localized eddies with curl. The significance of curl is further explored in the context of understanding whether a vector field is conservative, a property determined by a zero curl. The paragraph also introduces Stokes' theorem, which relates the work done by a vector field along a closed curve to a double integral of the curl over a surface, and explains the theorem's application in converting line integrals to surface integrals, providing an alternative approach to calculating complex integrals.
📘 Stokes' Theorem and Surface Orientation Conventions
The explanation of Stokes' theorem is expanded upon, emphasizing the importance of compatible orientations for the curve C and the surface S. The paragraph describes how to determine the normal vector's direction using the right-hand rule and the implications of choosing different orientations for the curve and surface. It also discusses the concept of flux and how it can be calculated differently depending on the orientation, with the normal vector's direction affecting whether the flux is considered positive or negative.
🤔 Clarifying Orientation and the Right-Hand Rule for Vector Fields
The paragraph focuses on clarifying the concept of orientation for curves and surfaces in vector field analysis. It explains the right-hand rule in different scenarios, such as walking along a curve with the surface to one side and how the normal vector direction changes accordingly. The paragraph also addresses the potential confusion in understanding the rule and offers alternative ways to visualize it, such as by imagining the surface and curve in various orientations or by using one's hand to represent the directions.
🔄 Topological Considerations and the Impact of Surface Deformation
This paragraph explores the topological aspects of vector fields and surfaces, discussing how the properties of a surface, such as its normal vector, can be understood through deformation. It uses the example of a cone being flattened into a disk to illustrate how the normal vector's direction is preserved through such transformations. The paragraph also touches on the implications of having a cylinder with two boundary curves and how to choose orientations for these curves to ensure the normal vector points outward.
📐 Stokes' Theorem Application and the Importance of Consistent Orientations
The script moves on to practical applications of Stokes' theorem, emphasizing the need for consistent orientations when dealing with vector fields and surfaces. It explains how to choose orientations for curves and surfaces when they are not provided and the importance of this consistency in ensuring the accuracy of calculations. The paragraph also provides an example of how Green's theorem is a special case of Stokes' theorem when applied to the x-y plane.
📘 The Role of Vector Field Continuity and Differentiability in Stokes' Theorem
This paragraph discusses the prerequisites for applying Stokes' theorem, specifically the requirement for the vector field to be continuous and differentiable everywhere on the surface. It provides examples of how to choose an appropriate surface when the vector field is not defined at certain points, such as avoiding the origin in the example given. The paragraph also mentions that for a closed surface, the flux would be zero due to the divergence of curl being zero, a topic to be explored further in subsequent lessons.
🔍 Proof Strategy for Stokes' Theorem Using Coordinate Systems
The script outlines a proof strategy for Stokes' theorem by leveraging the known special case of the theorem in the x-y plane and extending it to other planes and coordinate systems. It explains that work, flux, and curl have geometric interpretations that are independent of the coordinate system used. The paragraph suggests that any surface can be decomposed into small, almost flat pieces, allowing the application of Stokes' theorem on each piece and summing the results to obtain the total flux and work done.
📘 Example Calculation Using Stokes' Theorem and Surface Choice
The final paragraph provides an example calculation using Stokes' theorem to find the work done by a vector field around the unit circle in the x-y plane. It contrasts the direct calculation of the line integral with the application of Stokes' theorem using a non-flat surface, a piece of a paraboloid. The paragraph details the process of finding the curl of the vector field, calculating the flux through the chosen surface, and emphasizes the flexibility in choosing the surface for the application of Stokes' theorem, noting that while any surface can be chosen, a flat disk would have been a simpler choice in this case.
Mindmap
Keywords
💡Curl
💡Vector Field
💡Divergence
💡Stokes' Theorem
💡Conservative Vector Field
💡Line Integral
💡Surface Integral
💡Orientation
💡Right-Hand Rule
💡Flux
💡Differentiability
Highlights
Introduction to the concept of curl in vector fields, defined as the cross product between the del operator and vector field F.
Explanation of curl's significance in measuring the rotation within a fluid flow, with direction indicating the axis of rotation and magnitude relating to angular velocity.
Illustration of curl's computation through determinant expansion, yielding components that signify rotation in different planes.
Examples provided to demonstrate the curl of constant and stretching vector fields, highlighting the zero curl in cases of uniform translation or expansion.
Clarification on the difference between curl, which measures rotation, and divergence, which measures expansion or stretching effects in a vector field.
Demonstration of a vector field representing rotation around the z-axis, with the curl revealing the axis of rotation and associated angular velocity.
Discussion on the practical implications of curl in understanding complex fluid motions, such as in the Charles River with its observable eddies and swirls.
Introduction to Stokes' theorem, relating the work done by a vector field along a closed curve to a double integral of the curl over a surface.
Explanation of the conditions and conventions necessary for applying Stokes' theorem, including the compatibility of orientations for the curve and surface.
Use of the right-hand rule to determine the direction of the normal vector in relation to the curve and surface orientation.
Illustration of the application of Stokes' theorem through examples, including the comparison with Green's theorem in the context of the x, y plane.
Highlighting the importance of choosing an appropriate surface for Stokes' theorem based on the vector field's definition and differentiability.
Discussion on the geometric interpretation of work, flux, and curl, and their independence from the coordinate system used.
Introduction to the proof strategy for Stokes' theorem, leveraging the known cases in coordinate planes and extending to any surface by decomposing it into small flat pieces.
Example calculation using Stokes' theorem to find the work of a vector field around the unit circle in the x, y plane, contrasting direct integration with the theorem's application.
Emphasis on the arbitrary nature of conventions in curl and orientation compatibility, rooted in the preference for right-handed coordinate systems.
Final remarks on the prerequisites for using Stokes' theorem, including the necessity for the vector field to be continuous and differentiable on the surface.
Announcement of further discussion on the relationship between curl, conservativeness, and the divergence theorem, with a preview of an upcoming practice exam.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: