Stokes' theorem proof part 6 | Multivariable Calculus | Khan Academy
TLDRThis video script delves into the application of Green's theorem to a line integral over the boundary of a surface. The presenter begins by expressing the line integral in the dt domain and then proceeds to algebraically manipulate the expression to resemble the form required for applying Green's theorem. The goal is to demonstrate that this approach yields the same result as previously obtained through surface integrals. The script outlines the process of deparametrizing the integral and transitioning from the curve C to C1 in the xy plane, setting the stage for the application of Green's theorem in the next video.
Takeaways
- 📚 The video script discusses the process of converting a line integral over a boundary into an expression in the dt domain.
- 🔍 The lecturer plans to apply algebraic manipulation and Green's theorem to the integral.
- 📉 The integral is expressed in terms of the variables dx, dt, and dy, dt, with the intention of simplifying the expression.
- 📝 The script involves grouping terms that are multiplied by dx dt and dy dt, and factoring out these terms for clarity.
- 📈 The lecturer distributes the R vector across the terms involving partial derivatives of z with respect to x and y.
- 📌 The process aims to show that the manipulated expression is equivalent to the earlier evaluated surface integral.
- 📐 The script introduces a comparison with a theoretical vector field, suggesting a template for understanding the integral.
- 📘 The lecturer identifies similarities between the integral in the t domain and the theoretical vector field, with M and N being analogous to certain terms.
- 🔄 The integral is then rewritten in terms of a line integral over a new path C1 in the xy plane, simplifying the expression further.
- 🌐 The final goal is to apply Green's theorem to convert the line integral into a double integral over a region R in the xy plane.
- 🔚 The lecturer intends to demonstrate in the next video that the results obtained from this process will match those from previous videos.
Q & A
What is the main goal of the video script?
-The main goal of the video script is to perform algebraic manipulation on a line integral over the boundary of a surface expressed in the dt domain, apply Green's theorem, and show that the result is consistent with an earlier evaluation of a surface integral.
What is the significance of expressing the line integral in the dt domain?
-Expressing the line integral in the dt domain allows for the application of Green's theorem, which simplifies the process of evaluating the integral by transforming it into a double integral over a region in the xy-plane.
What algebraic manipulations are performed on the integral?
-The algebraic manipulations include grouping terms that are multiplied by dx dt and dy dt, factoring out dx dt and dy dt, and distributing the components of the vector field R across the partial derivatives of z with respect to x and y.
What is the role of Green's theorem in this context?
-Green's theorem is used to convert the line integral over the curve C1 in the xy-plane into a double integral over the region R that the curve surrounds, simplifying the calculation.
How does the script relate the manipulated integral to the theoretical vector field?
-The script shows that after manipulation, the integral resembles the form of the theoretical vector field, with terms analogous to M and N, which are functions of x and y multiplied by dx dt and dy dt, respectively.
What is the significance of the terms M and N in the context of the script?
-M and N represent the components of the vector field that are analogous to the manipulated integral's terms after applying the algebraic manipulations and factoring out dx dt and dy dt.
What does the script imply about the relationship between the original boundary C and the new path C1?
-The script implies that the new path C1 in the xy-plane is analogous to the original boundary C, and the integral over C1 will yield the same result as the integral over the original boundary when applying Green's theorem.
How does the script suggest that the final result will be consistent with earlier videos?
-The script suggests that by manipulating the integral in a certain way after applying Green's theorem, the result will be the same as the one obtained in earlier videos, where a surface integral was evaluated.
What is the purpose of the art program mentioned in the script?
-The art program is used to visually demonstrate the process of copying and pasting parts of the work to illustrate the algebraic manipulations and the application of Green's theorem.
What is the final step mentioned in the script for the next video?
-The final step mentioned is to apply Green's theorem to the manipulated integral and show that it yields the same result as the earlier evaluation of the surface integral, which will be demonstrated in the next video.
Outlines
📚 Algebraic Manipulation and Green's Theorem Application
This paragraph discusses the continuation of a mathematical process involving line integrals over a boundary of a surface. The speaker plans to perform algebraic manipulations and apply Green's theorem to show that it results in the same outcome as a previously evaluated surface integral. The focus is on expressing the integral in terms of 'dt' in the 'dt domain', grouping terms, and factoring out 'dx dt' and 'dy dt'. The speaker also aims to demonstrate that the transformed integral resembles the theoretical vector field and can be rewritten in a parametrized form, setting the stage for applying Green's theorem in the next video.
🔍 Transition to Double Integral Using Green's Theorem
The second paragraph continues the discussion by focusing on the application of Green's theorem to the transformed line integral. The speaker intends to show that the result of this application will be identical to the results obtained in earlier videos. The process involves reverting the parametrized integral back into the xy-plane, which simplifies the integral to a form that can be directly related to the original boundary C. The speaker emphasizes the significance of this step, as it allows for the application of Green's theorem to convert the line integral into a double integral over the region R, which is surrounded by the path C1 in the xy-plane. The paragraph concludes with a promise to demonstrate this process in the next video.
Mindmap
Keywords
💡Line Integral
💡Green's Theorem
💡Algebraic Manipulation
💡Surface Integral
💡Vector Field
💡Partial Derivative
💡Differential Elements (dx, dy, dt)
💡Parametric Representation
💡Deparametrize
💡Boundary
💡Double Integral
Highlights
The line integral over the boundary of a surface is expressed in terms of dt in the dt domain.
Algebraic manipulation will be performed before applying Green's theorem.
The goal is to show the equivalence between the line integral and the surface integral evaluated earlier.
The integral is expressed as an integral from a to b, grouping terms multiplied by dx dt.
R is distributed and factored out with dx dt and dy dt.
The expression starts to resemble the theoretical vector field.
The integral can be rewritten in terms of M and N, analogous to the theoretical vector field.
The integral is deparametrized back into the xy plane.
The path of integration is now C1 in the xy plane, not the original boundary C.
Green's theorem will be applied to convert the line integral into a double integral over the region R.
The result will be the same as in earlier videos, demonstrating the consistency of the approach.
The process involves distributing and factoring out terms to simplify the integral expression.
The integral is manipulated to resemble the form seen in previous theoretical discussions.
The transition from the t domain to the xy plane is a key step in the manipulation.
The analogy between the current expression and the theoretical vector field is emphasized.
The final expression is set up for the application of Green's theorem in the next steps.
Transcripts
Browse More Related Video
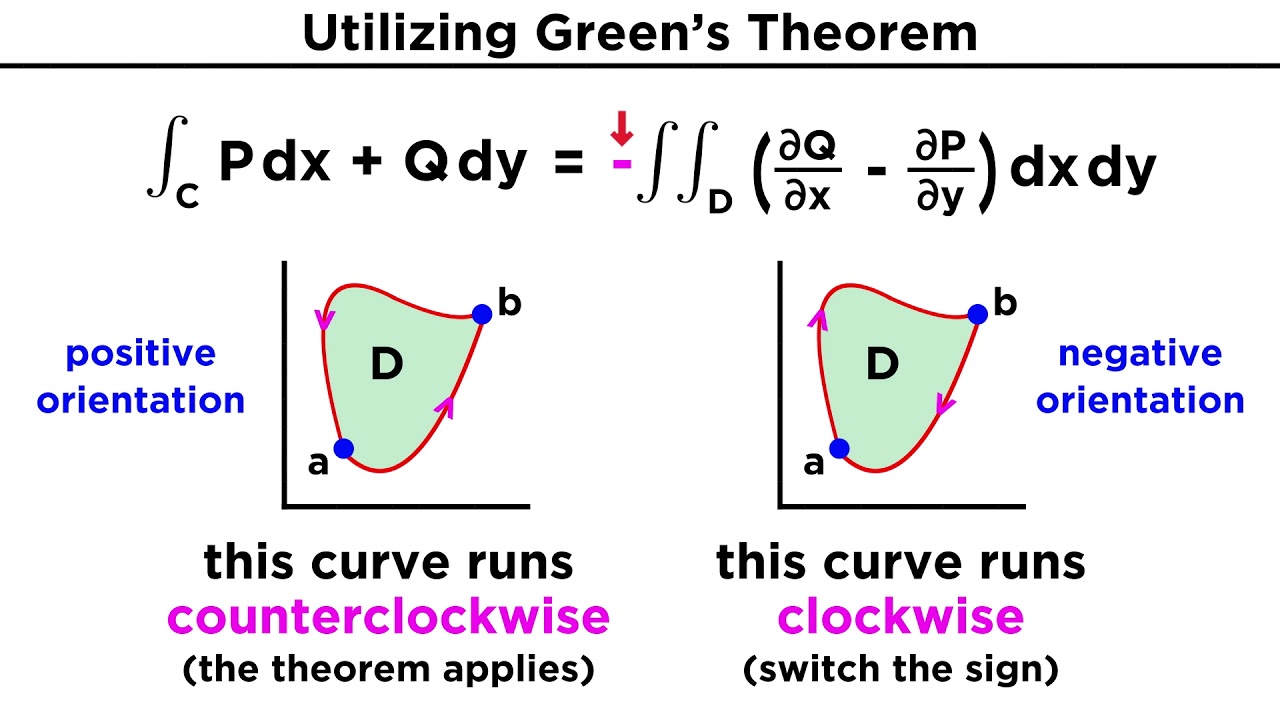
Green's Theorem

Stokes' theorem proof part 7 | Multivariable Calculus | Khan Academy

Stokes' theorem proof part 3 | Multivariable Calculus | Khan Academy

Green's and Stokes' theorem relationship | Multivariable Calculus | Khan Academy
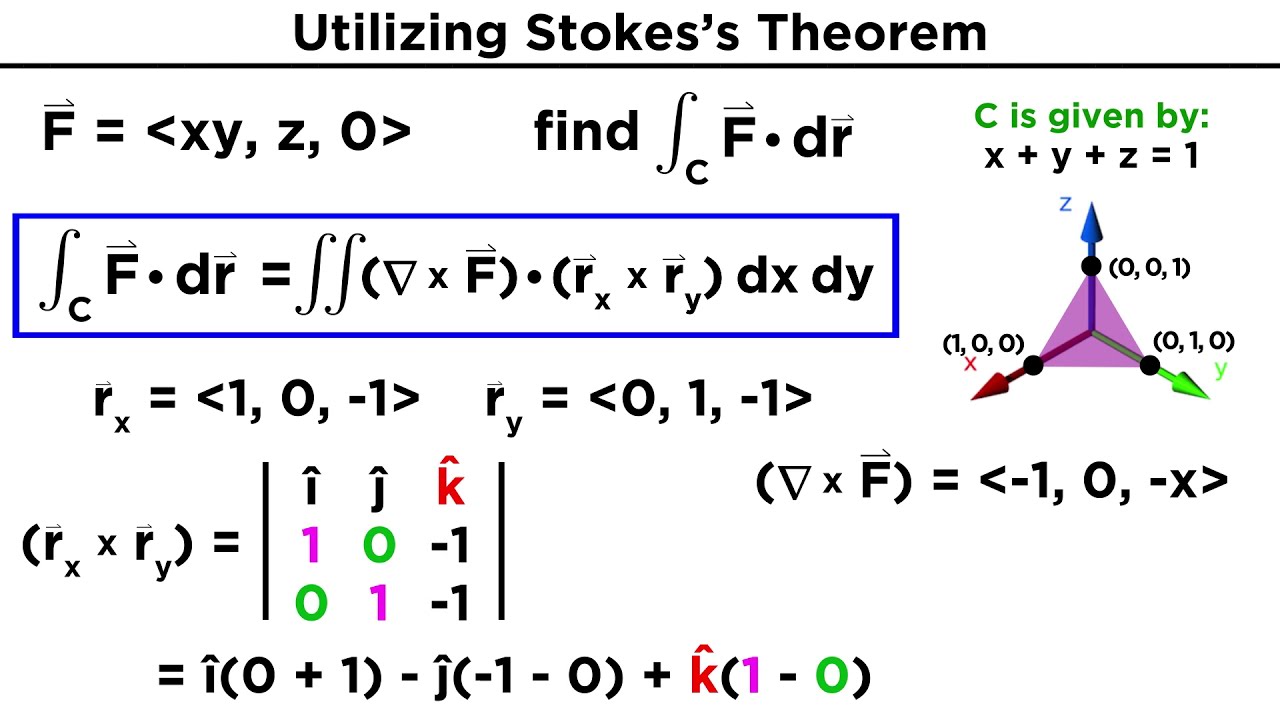
Stokes's Theorem

Green's theorem example 2 | Multivariable Calculus | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: