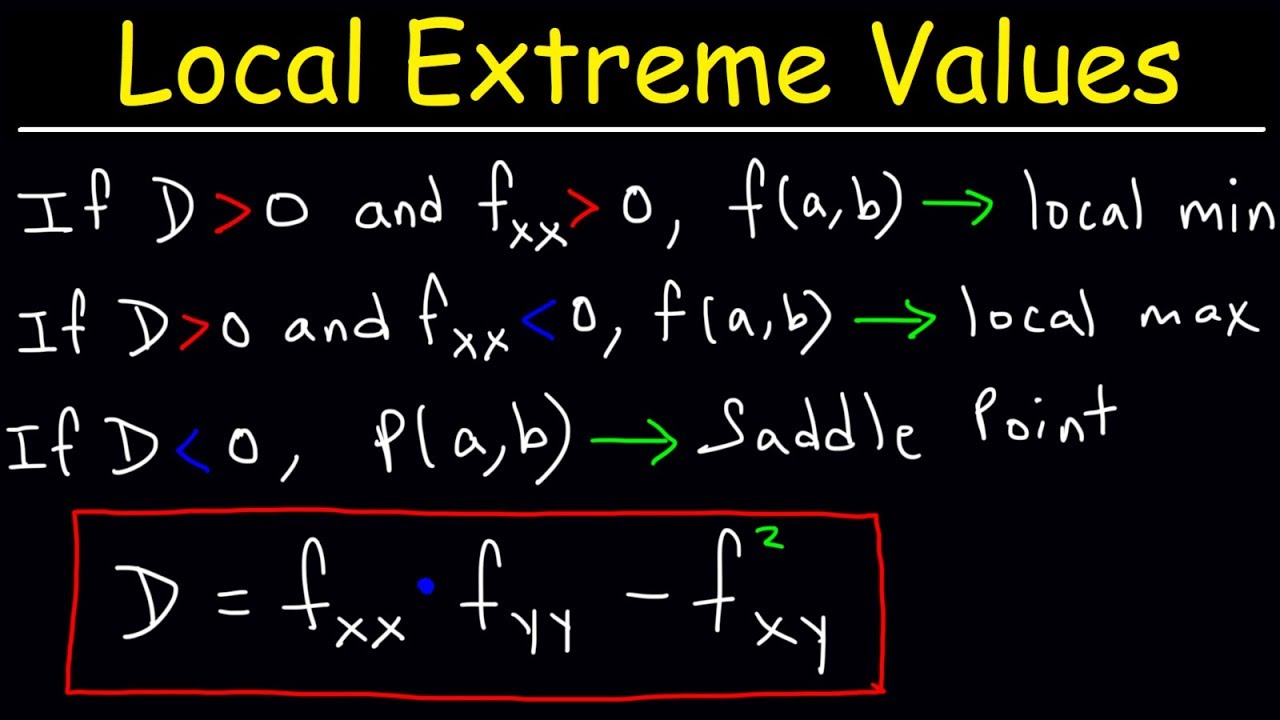
Saddle points

Khan Academy
22 Jun 201605:50
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores multi-variable calculus, focusing on finding extrema of functions by visualizing the graph and identifying flat tangent planes. It introduces the concept of saddle points, using the function f(x,y)=x^2-y^2 as an example, where the origin has a flat tangent plane but is neither a local maximum nor minimum. The script explains how to recognize saddle points by examining the function's behavior in different directions and hints at the second partial derivative test for future discussion.

Takeaways
  • 📈 The script discusses the concept of maximizing or minimizing a multi-variable function by visualizing its graph and finding points where the tangent plane is flat.
  • 🔍 It explains that a flat tangent plane represents a constant output value and is found where the function barely intersects with the plane, indicating no higher values above that point.
  • 🏞️ The script introduces the idea of local minima, where the function value is higher than all neighboring points, and one would go downhill in any direction from such points.
  • 🐎 It introduces the concept of a saddle point, a unique feature of multi-variable functions where the function behaves differently in different directions, neither a local maximum nor minimum.
  • 📚 The function f(x, y) = x^2 - y^2 is used as an example to illustrate the concept of a saddle point, where the tangent plane at the origin is flat.
  • 🧭 The partial derivatives of the function are calculated to demonstrate that at the origin, both derivatives are zero, indicating no slope in any direction.
  • 📊 By 'chopping' the graph with planes of constant X or Y values, it's shown that the function has a local maximum in one direction and a local minimum in the other, confirming the saddle point.
  • 🤔 The script emphasizes the importance of recognizing saddle points in optimization problems, such as in company profit maximization or cost functions in machine learning.
  • 🔬 It hints at the second partial derivative test, a method to determine if a point is a saddle point without visual graph analysis, which will be discussed in subsequent videos.
  • 📝 The script is educational, aiming to teach viewers about advanced calculus concepts related to multi-variable functions and their optimization.
  • 👋 The presenter signs off, indicating the end of the video and the continuation of the topic in future videos.
Q & A

  • What is the main concept discussed in the video script?

    -The main concept discussed in the video script is the process of finding extrema (maxima or minima) in multi-variable functions, specifically the concept of saddle points and how they differ from local maxima or minima.

  • What does it mean for a tangent plane to be flat?

    -A tangent plane being flat means that the partial derivatives of the function at that point are zero, indicating no slope in any direction from that point.

  • Why is the concept of a saddle point important in multi-variable calculus?

    -The concept of a saddle point is important in multi-variable calculus because it represents a point where the function does not have a local maximum or minimum, but rather behaves differently depending on the direction you consider, which is a phenomenon not present in single-variable calculus.

  • What is the function given in the script to illustrate the concept of a saddle point?

    -The function given in the script to illustrate the concept of a saddle point is f(x, y) = x^2 - y^2.

  • How do you determine the partial derivatives of the function f(x, y) = x^2 - y^2?

    -The partial derivative with respect to x is 2x, and the partial derivative with respect to y is -2y. These are found by differentiating the function with respect to each variable while treating the other variable as a constant.

  • What happens when you evaluate the partial derivatives of the function f(x, y) = x^2 - y^2 at the origin (0,0)?

    -When you evaluate the partial derivatives at the origin (0,0), both derivatives equal zero, indicating that the tangent plane at the origin is flat.

  • What is the significance of the second partial derivative test mentioned in the script?

    -The second partial derivative test is significant because it helps determine the nature of critical points in multi-variable functions, such as whether a point is a local maximum, local minimum, or a saddle point.

  • How does the script describe the saddle point visually?

    -The script describes the saddle point visually by comparing it to the shape of a saddle that you would put on a horse's back, indicating that the function behaves differently when viewed from different directions.

  • Why are saddle points important in practical applications like optimization?

    -Saddle points are important in practical applications like optimization because they represent points where the function does not have a clear maximum or minimum, which is crucial to recognize when trying to find the absolute maximum or minimum of a function.

  • What does the script suggest will be discussed in the next videos?

    -The script suggests that the next videos will discuss the second partial derivative test, which is a method to determine whether a critical point is a local maximum, local minimum, or a saddle point.

Outlines
00:00
📈 Understanding Multi-variable Function Optimization

This paragraph introduces the concept of optimizing a multi-variable function by visualizing its graph and identifying points where the tangent plane is flat, indicating potential local minima or maxima. The focus is on finding the spot where a flat plane barely intersects the graph, suggesting a local extremum. It also introduces the concept of saddle points, which are unique to multi-variable functions and represent points where the function behaves like a local maximum in one direction and a local minimum in another. The example function f(x, y) = x^2 - y^2 is used to illustrate the calculation of partial derivatives at the origin, which both equal zero, indicating a flat tangent plane. The explanation includes a visual representation of how the function behaves when 'chopped' in different directions, revealing the saddle point's nature.

05:01
🐎 The Saddle Point and Its Significance in Optimization

The second paragraph delves deeper into the concept of saddle points, likening their shape to a saddle used on a horse's back, hence the name. It discusses the importance of recognizing saddle points when optimizing functions that may represent real-world scenarios such as company profits or machine learning cost functions. The paragraph sets the stage for the next part of the series, which will cover the second partial derivative test—a method for identifying saddle points without the need for graphical representation. The speaker promises to elaborate on this test in upcoming videos, leaving the audience with an anticipation for further insights into multi-variable calculus.

Mindmap
Keywords
💡Multi-variable function
A multi-variable function is a mathematical function that depends on more than one independent variable. In the context of the video, the focus is on functions of two variables, such as f(x, y), where the graph represents a surface in three-dimensional space. The script discusses how to find extrema (maxima or minima) of such functions by visualizing the graph and identifying points where the tangent plane is flat.
💡Tangent plane
A tangent plane is a concept from calculus that represents the local behavior of a surface at a given point. It is a plane that touches the surface at that point without intersecting it elsewhere. In the video, the tangent plane is used to find points of extrema by looking for where it is 'completely flat,' indicating no change in the function's value in any direction from that point.
💡Partial derivatives
Partial derivatives are the rate at which a multi-variable function changes with respect to one variable while keeping the other variables constant. The script mentions computing the partial derivatives of the function f(x, y) = x^2 - y^2 and evaluating them at the origin to determine the slope of the tangent plane, which is essential for identifying extrema or saddle points.
💡Origin
In mathematics, the origin refers to the point (0, 0) in a two-dimensional coordinate system or (0, 0, 0) in three dimensions. The script uses the origin as an example to demonstrate how to calculate partial derivatives and to illustrate the concept of a flat tangent plane at a specific point.
💡Local minima and maxima
Local minima and maxima are points on a function's graph where the function's value is less than or greater than the values of all neighboring points, respectively. The video script uses the concept of local minima and maxima to describe points where the function's value is higher or lower than its surroundings, which can be incidentally found when looking for flat tangent planes.
💡Saddle point
A saddle point is a special type of critical point in multi-variable calculus where the tangent plane is flat, but the point is neither a local maximum nor a local minimum. The script introduces the concept by showing a function where the origin has a flat tangent plane but behaves like a local maximum in one direction and a local minimum in another, hence the name 'saddle point' due to its resemblance to a saddle on a horse.
💡Second partial derivative test
The second partial derivative test is a method used to determine the nature of critical points in multi-variable functions, specifically to distinguish between local minima, local maxima, and saddle points. The script mentions that this test will be discussed in subsequent videos as a way to identify saddle points without relying on visual graphs.
💡Optimization
Optimization refers to the process of finding the best solution or result from a set of possible alternatives, often in the context of maximizing or minimizing a function. The video script relates the concept of finding extrema in multi-variable functions to practical applications like optimizing profits or cost functions in machine learning.
💡Paraboloid
A paraboloid is a three-dimensional surface shaped like a parabola, which is a U-shaped curve. In the script, the function f(x, y) = x^2 - y^2 is discussed, and its graph is described as having parabolic shapes in both the x and y directions, which helps to illustrate the concept of a saddle point.
💡Critical point
A critical point in calculus is a point on the graph of a function where the derivative is either zero or undefined. The script discusses critical points in the context of multi-variable functions, specifically saddle points, which are a type of critical point where the tangent plane is flat but does not correspond to a local extremum.
Highlights

The concept of maximizing or minimizing a multi-variable function by visualizing its graph and identifying where the tangent plane is completely flat.

Imagining a flat plane representing a constant Z value to find points of tangency on the function's graph.

The introduction of local minima as points where the function's value is higher than all neighboring points.

The emergence of saddle points in multi-variable functions, a concept not present in single-variable calculus.

Visualizing saddle points with the function f(x,y)=x^2-y^2 and its flat tangent plane at the origin.

Computing partial derivatives of the function to confirm the flatness of the tangent plane at the origin.

The significance of both partial derivatives being zero, indicating no slope in any direction from the origin.

Chopping the graph with planes of constant X or Y values to reveal local maxima and minima from different perspectives.

The paradox of a point being both a local maximum and minimum depending on the direction viewed, defining the saddle point.

The explanation of why single-variable calculus does not have saddle points due to the lack of directional disagreement.

The saddle point's unique terminology, likened to the shape of a saddle used on a horse's back.

The practical implications of recognizing saddle points in optimization problems, such as in company profits or machine learning cost functions.

The预告 of the second partial derivative test as a method to identify saddle points without visual graph analysis.

The importance of the second partial derivative test in computationally determining the nature of critical points in functions.

The upcoming discussion on the second partial derivative test in subsequent videos to aid in function optimization.

Transcripts

Browse More Related Video

Local Extrema, Critical Points, & Saddle Points of Multivariable Functions - Calculus 3

Second partial derivative test

Increasing/Decreasing + Local Max and Mins using First Derivative Test

Use the Second Derivative Test to Find Any Extrema and Saddle Points: f(x,y) = -4x^2 + 8y^2 - 3

Analyzing a function with its derivative | AP Calculus AB | Khan Academy

Find and classify critical points

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Multi-variable CalculusSaddle PointsOptimizationPartial DerivativesTangent PlanesLocal MaximaLocal MinimaGraph AnalysisMathematical ConceptsMaximize FunctionMinimize Function