Finding Volume with Double Integrals (Rectangular Coordinates)

Houston Math Prep

23 Apr 202026:35

EducationalLearning

32 Likes 10 Comments

TLDRThis video from Houston Math Prep delves into calculating volumes using double integrals, expanding on the concept of finding areas. It explains how to interpret the double integral as summing up the value one at every point to represent a region with a height of one, conceptualizing it as volume. The instructor guides through examples, including finding the volume between the XY plane and various planes or surfaces, and demonstrates the process of setting up and computing the integrals for different solid objects. The video also covers integrating between two functions to find the volume of a solid bounded by different planes.

Takeaways

📚 The video is a tutorial on finding volume with double integrals, expanding on the concept of finding area using integrals.
🔍 It explains that a double integral over a region R with 'da' can be thought of as calculating area, with the integral being either in the order of 'dx dy' or 'dy dx'.
📈 The script clarifies that the function inside a double integral, often a constant like 1, can represent a uniform height over the region, conceptualizing the area as a volume.
📉 The importance of the height function within the integral is emphasized, as varying heights can transform the calculation of area into a calculation of volume for non-uniform shapes.
🌟 An example is given where the volume between the XY plane and the plane Z = 6 - 3x - 2y is calculated using a double integral with the height function as part of the integral.
📐 The script demonstrates setting up double integrals for different regions, such as a square for the first example, and determining the order of integration (dx dy or dy dx) based on the shape of the region.
📝 The process of evaluating the integral involves finding appropriate bounds for the integral based on the region's geometry and then performing the integration step by step.
📊 The video provides a method to find the volume of a tetrahedron in the first octant bounded by the plane 4x + 2y + z = 8, by rearranging the equation to solve for z and setting up the double integral accordingly.
📉 Another example involves finding the volume under the graph of Z = x^2 above a triangular region, showcasing the process of setting bounds and integrating with respect to y first due to the region's shape.
🏢 The final example discusses finding the volume of a solid bounded by two planes and the coordinate planes, emphasizing the need to subtract the bottom function from the top function when setting up the integral.
🎓 The tutorial concludes by highlighting the general approach to solving volume problems with double integrals, which includes understanding the region, setting up the integral with appropriate bounds, and evaluating the integral.

Q & A

What is the main topic of the video?
-The main topic of the video is finding volume with double integrals.
What is the significance of having a function inside a double integral?
-The function inside a double integral represents the height at every point in the region, which when summed over the region, allows for the calculation of volume instead of just area.
What is the difference between calculating area and volume using double integrals?
-Calculating area with double integrals involves integrating over a region to find the total area, while calculating volume involves summing up the heights (represented by a function) over the region to find the total volume.
What is the first example given in the video for calculating volume with a double integral?
-The first example is calculating the volume between the XY plane and the plane Z = 6 - 3x - 2y.
How does the shape of the base affect the order of integration in double integrals?
-The shape of the base determines whether the region is simpler to integrate in the x-direction or y-direction, which in turn affects the choice of dy dx or dx dy order for the iterated integral.
What is the process for setting up the bounds for a double integral?
-The process involves identifying the region's shape and determining the lower and upper bounds for the inner and outer integrals based on the region's limits.
How does the video script handle the calculation of the volume of a tetrahedron in the first octant?
-The script rearranges the equation 4x + 2y + z = 8 in terms of z, then sets up the double integral with the appropriate bounds for x and y to calculate the volume of the tetrahedron.
What is the importance of solving for Z explicitly in the context of double integrals for volume?
-Solving for Z explicitly allows for the correct representation of the height function, which is necessary for setting up the correct double integral to calculate volume.
How does the script approach the calculation of volume for a solid bounded by multiple planes?
-The script uses the approach of integrating the top function minus the bottom function over the region, which accounts for the volume between the two planes.
What is the final example in the script for calculating volume with double integrals?
-The final example is calculating the volume of a solid bounded above by the plane x + y + 2z = 6, below by the plane y - 2z = 8, and bounded by the XZ and YZ planes.

Outlines

00:00

📚 Introduction to Volume Calculation with Double Integrals

Houston Math Prep introduces the concept of calculating volume using double integrals, expanding on the previous topic of finding areas with double integrals. The script explains that while double integrals typically calculate area, considering a function inside the integral can represent volume. The idea is to think of the double integral of 1 over a region as summing up the value at every point, akin to a solid with a uniform height of 1. The video then transitions to calculating the volume of an object with varying heights represented by a function of x and y.

05:01

📐 Calculating the Volume of a Solid with a Given Height Function

The script presents an example of finding the volume between the XY plane and the plane Z = 6 - 3x - 2y. It sets up the double integral with the height function inside and chooses the order of integration as dy dx. The region of integration is a square from 0 to 1 on both x and y axes. The integral is computed step by step, resulting in the volume of the solid being 7/2 or 3.5 cubic units.

10:03

📏 Volume of a Tetrahedron in the First Octant

The video moves on to calculate the volume of a tetrahedron in the first octant bounded by the plane 4x + 2y + z = 8. The height function is derived by solving for z, resulting in z = 8 - 4x - 2y. The region's shape is triangular, and the script determines the order of integration as dy dx based on the triangular base. The bounds for integration are established, and the integral is computed, yielding a volume of 32/3 or approximately 10.67 cubic units.

15:04

📉 Volume Under the Graph of Z = x^2 Over a Triangular Region

The script then discusses finding the volume under the graph of Z = x^2 above a triangular region with vertices at (0, -1), (1, 0), and (0, 1). The region is determined to be Y simple, and the bounds for integration are set as y = x - 1 and y = 1 - x. The double integral is evaluated, resulting in a volume of 1/6 cubic units.

20:04

🛠 Volume of a Solid Bounded by Two Planes and Two Surfaces

The video explains how to find the volume of a solid bounded by the plane X + Y + 2Z = 6 above and the plane Y - 2Z = 8 below, with sides bounded by the XZ and YZ planes and a vertical surface X + Y = 6. The volume is calculated by integrating the difference between the top and bottom functions with respect to Z. The region is triangular, and the script sets up the double integral with the appropriate bounds, resulting in a volume of 72 cubic units.

25:08

🎓 Conclusion and Summary of Double Integrals for Volume

In conclusion, the video script summarizes the process of calculating volumes with double integrals, emphasizing the use of a height function to represent varying heights across a region or integrating between two functions to find the volume between them. The script thanks viewers for watching and hints at further topics to be covered in upcoming videos.

Mindmap

Keywords

💡Double Integrals

Double integrals are a mathematical concept used to calculate volumes, areas, and other quantities over a two-dimensional region. In the context of this video, double integrals are used to find the volume of a solid bounded by different surfaces. The script explains that double integrals can be set up as iterated integrals in either order (dy DX or DX dy), which is crucial for calculating areas or volumes by summing up infinitesimally small contributions over a region.

💡Volume

Volume refers to the amount of space occupied by a solid object. In the script, the concept of volume is central as the video aims to teach how to calculate the volume of various solids using double integrals. The video provides examples of solids with different shapes and demonstrates how to set up and compute double integrals to find their volumes.

💡Iterated Integral

An iterated integral is a type of integral that is computed in successive steps, integrating one variable at a time. The video script uses iterated integrals to explain how to calculate areas and volumes by breaking down the problem into simpler, one-dimensional integrals. The choice between dy DX and DX dy in the script illustrates the flexibility in the order of integration when setting up double integrals.

💡Region R

Region R in the script denotes a specific area in the xy-plane over which the double integral is being evaluated. The region is important because it defines the boundaries within which the volume or area calculations take place. The script mentions different shapes of regions, such as squares and triangles, and how they relate to the setup of the integrals.

💡Height Function

The height function in the context of this video is a function that describes the distance from the base of a solid to its top surface at every point within the base area. The script explains that instead of a constant height of 1, different height functions based on x or y can be used to calculate the volume of more complex shapes by summing up the product of the base area element and the corresponding height function value.

💡XY Plane

The XY plane is a two-dimensional plane defined by the x and y axes in a Cartesian coordinate system. In the script, the XY plane serves as the base from which the volume of the solid above it is calculated. The video discusses solids bounded by the XY plane and other surfaces, emphasizing the importance of the XY plane in setting up the bounds for the integrals.

💡Tetrahedron

A tetrahedron is a polyhedron with four triangular faces, and in the script, it is used as an example of a solid whose volume is to be calculated using double integrals. The video explains how to set up the double integral for a tetrahedron bounded by a plane and the XY plane, demonstrating the application of the height function and the choice of integration order.

💡Integration Bounds

Integration bounds are the limits that define the range over which an integral is computed. In the script, determining the correct bounds for the integrals is essential for accurately calculating volumes. The video provides examples of how to identify and apply these bounds based on the shape of the region and the equations of the surfaces defining the solid.

💡Area

Area is a measure of the extent of a two-dimensional surface. The script mentions area in the context of explaining how double integrals can calculate areas as well as volumes. It is noted that the integral of the constant function 1 over a region represents the area of that region, which is a foundational concept before extending to volume calculations with variable height functions.

💡Solid Object

A solid object in the script refers to a three-dimensional figure for which the volume is being calculated. The video uses the term to describe various geometric shapes whose volumes are determined through the application of double integrals. The script provides a step-by-step approach to finding the volume of different solid objects, emphasizing the practical application of mathematical concepts.

Highlights

Introduction to expanding on the previous video about finding area using double integrals to now finding volume with double integrals.

Explanation of double integrals as calculating area and the significance of the function inside the integrals, which can represent height in volume calculations.

Illustration of how a constant height function of 1 in a double integral can be thought of as summing up the value one at every point, conceptualizing it as a region with a height of 1.

Discussion on how varying heights across a region can be represented by a function of x or y, allowing for the calculation of volume with double integrals.

First example demonstrating the volume calculation between the XY plane and the plane Z = 6 - 3x - 2y using double integrals.

Selection of the order of integration (dy DX or DX dy) based on the shape of the region, and setting up the integral with the appropriate bounds.

Computation of the integral for the volume of a solid object with a given height function, including the integration process and evaluation of bounds.

Second example calculating the volume of a tetrahedron in the first octant bounded by the plane 4x + 2y + Z = 8.

Rearranging the equation to solve for Z as the height function and choosing the order of integration based on the triangular shape of the base.

Determining the bounds for the integral by analyzing the triangular region and the equations of the lines forming the base.

Integration process for the volume of the tetrahedron, including the setup of the integral and the evaluation of the bounds for both inner and outer integrals.

Third example finding the volume under the graph of Z = x^2 above a triangular region with given vertices.

Setting up the volume integral with the top surface formula and analyzing the triangular region to determine the order of integration.

Establishing the bounds for the integral based on the equations of the lines forming the triangular region and the vertices.

Integration process for the volume under the graph of Z = x^2, including simplification of terms and evaluation of the integral.

Fourth example calculating the volume of a solid bounded by two planes and the coordinate planes, requiring the integration of the top minus the bottom function.

Solving for the top and bottom functions in terms of Z and setting up the double integral with the correct order of integration.

Determining the bounds for the integral by analyzing the triangular region in the XY plane and the lines forming the region.

Final integration process for the volume of the solid, including combining like terms, evaluating the integral, and obtaining the volume.

Conclusion summarizing the method of using double integrals to calculate volumes with a height function or between two functions (top minus bottom).

Transcripts

Browse More Related Video

Triple Integrals in Rectangular Coordinates

Triple Integrals in Cylindrical Coordinates

2011 Calculus AB free response #3 (c) | AP Calculus AB | Khan Academy

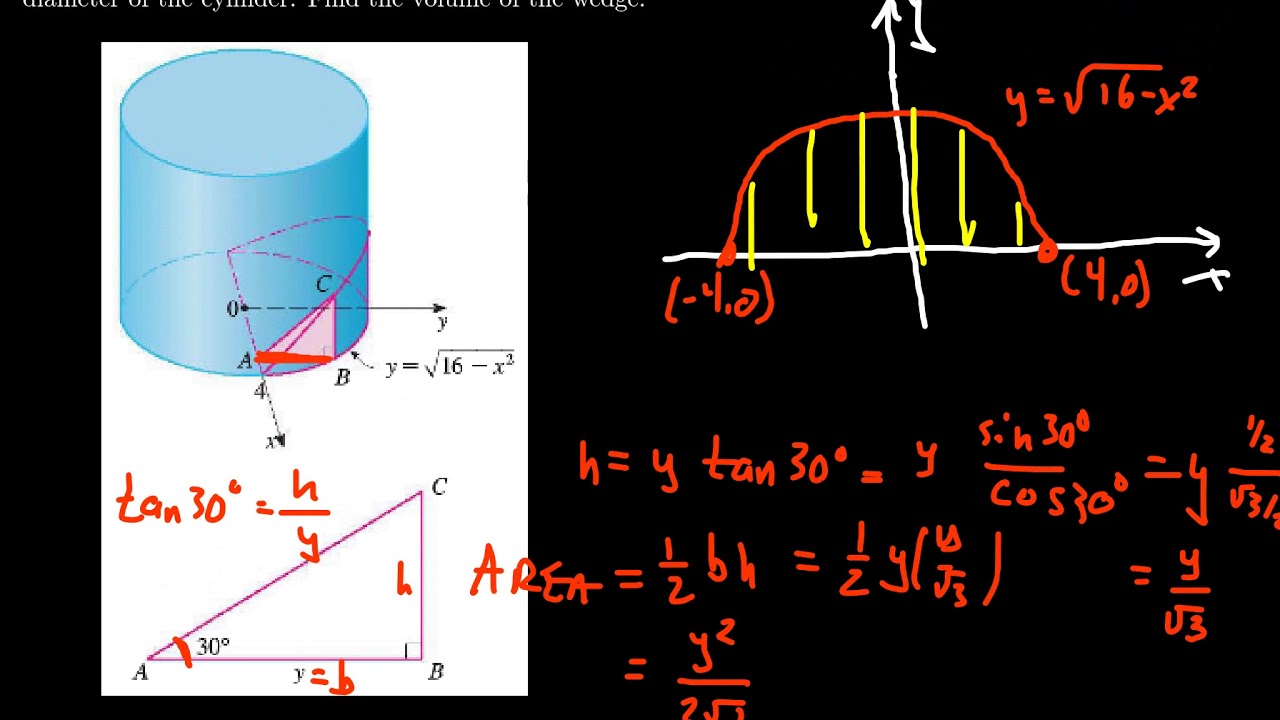
The Volume of a Cylindrical Wedge with Triangular Cross-Sections

AP Calculus AB: Lesson 7.4: Disk and Washer Method

Lec 25: Triple integrals in rectangular & cylindrical | MIT 18.02 Multivariable Calculus, Fall 2007

Related Tags

Double Integrals Volume Calculation Math Tutorial Height Function Area to Volume Integrals Explained Solid Geometry Educational Content Houston Math Prep Calculus Concepts