Calculus 3 Lecture 15.2: How to Find Divergence and Curl of Vector Fields

Professor Leonard

6 May 201671:49

EducationalLearning

32 Likes 10 Comments

TLDRThis lecture delves into the characteristics of vector fields, focusing on divergence and curl, which describe the flow and rotation of substances like fluid or electricity. The instructor uses the analogy of a pond's surface to illustrate the concept of vector fields and explains how divergence measures the flow entering and leaving a point, while curl measures the rotation. The lecture includes mathematical formulas and examples to clarify these concepts, emphasizing their importance in understanding vector field behavior.

Takeaways

📚 The lecture focuses on the characteristics of vector fields, specifically divergence and curl, and their significance in calculus.
🌀 Divergence measures how much fluid or flow enters or leaves a small neighborhood around a point in a vector field, indicating whether the field is gaining or losing fluid at that point.
🔄 Curl measures the tendency of fluid or flow to rotate within a neighborhood of a point in a vector field, indicating the presence of vorticity or spinning motion.
💧 A positive divergence indicates more fluid is entering a neighborhood than leaving, suggesting fluid is gathering at that point.
🌪️ A negative divergence indicates more fluid is leaving a neighborhood than entering, suggesting fluid is diverging away from that point.
⭕️ When the amount of fluid entering and leaving a neighborhood is equal, the divergence is zero, and the field at that point is considered incompressible.
🔧 A positive curl indicates counterclockwise rotation of the fluid or flow at a point, while a negative curl suggests clockwise rotation.
🔄 Zero curl at a point indicates no rotation or irrotational flow at that point, and if true for all points, the vector field is irrotational.
📘 The concepts of divergence and curl are applied to various problems in vector calculus, including fluid dynamics and electromagnetism.
📝 The lecture provides mathematical formulas and procedures for calculating divergence and curl, emphasizing the importance of understanding their physical interpretations.

Q & A

What are the two main characteristics of vector fields discussed in the script?
-The two main characteristics of vector fields discussed are divergence and curl, which describe how a flow (like fluid or electricity) behaves within the field.
What does divergence measure in the context of a vector field?
-Divergence measures how much fluid or flow enters or leaves a small neighborhood around a point within the vector field. It compares the amount of flow entering versus leaving to determine if there's a net gain or loss of fluid at that point.
What is the significance of a negative divergence?
-A negative divergence indicates that more fluid or flow is entering a neighborhood around a point than is leaving, suggesting that fluid is being collected or converging at that point.
How is the term 'incompressible' related to divergence?
-A vector field is considered incompressible at a point if the divergence is zero at that point, meaning the same amount of fluid enters and leaves the neighborhood around that point, indicating no net gain or loss of fluid.
What does a positive divergence signify in terms of fluid flow?
-A positive divergence signifies that more fluid is leaving the neighborhood around a point than is entering, which can be visualized as fluid diverging or spreading away from that point.
What is curl, and what does it measure?
-Curl is a measure of the tendency of the fluid or flow within a vector field to rotate around a given point. It quantifies the rotational motion or 'spinning' of the flow at that point.
What does a positive curl value indicate about the rotation of a fluid?
-A positive curl value indicates that the fluid or flow is rotating counterclockwise around the point, suggesting a left-handed rotation.
How is the maximum rate of rotation related to the curl vector?
-The maximum rate of rotation is equal to the magnitude of the curl vector. It represents the greatest possible spinning or rotational effect around the point, which occurs when a paddle or similar object is aligned parallel to the curl vector.
What is the difference between a divergent and an incompressible vector field?
-A divergent vector field has a positive divergence at every point, meaning more fluid is leaving than entering the neighborhood around each point. In contrast, an incompressible vector field has a divergence of zero at every point, indicating that the amount of fluid entering and leaving is the same, with no net gain or loss.
What does an irrotational vector field imply about the curl at every point?
-An irrotational vector field implies that the curl is zero at every point, indicating no rotation or spinning of the fluid or flow within the field.

Outlines

00:00

📚 Introduction to Vector Fields and Divergence

The script begins with an introduction to vector fields, emphasizing their role in describing the flow of substances like fluids or electricity. The main focus is on the concepts of divergence and curl, which are characteristics used to understand how vector fields behave. Divergence measures the amount of flow entering or leaving a small neighborhood around a point, indicating whether fluid is converging or diverging from that point. The explanation clarifies that a positive divergence indicates more fluid entering than leaving, while a negative divergence suggests the opposite. The concept of an incompressible vector field, where the flow neither enters nor leaves a neighborhood, is also introduced.

05:01

🔍 Calculating Divergence and Understanding Its Implications

This paragraph delves into the mathematical calculation of divergence, introducing the del operator and its application in vector fields. The explanation outlines the process of finding divergence by taking partial derivatives of the vector field's components with respect to their corresponding spatial dimensions. The paragraph also discusses the physical interpretation of divergence, such as its relation to the conservation of mass and the concept of incompressibility in fluid dynamics. The importance of divergence in understanding the behavior of fluid flow within a vector field is highlighted.

10:02

🌀 Exploring the Concept of Curl in Vector Fields

The script shifts focus to the concept of curl, which quantifies the rotation or spinning motion within a vector field. A vivid analogy of inserting a paddle into a flowing fluid is used to explain how curl measures the tendency of the flow to rotate around a point. The mathematical formulation of curl is introduced, involving the cross product of the del operator and the vector field. The summary clarifies the physical significance of positive and negative curl values, indicating clockwise and counterclockwise rotation, respectively, and the special case of zero curl, which implies no rotation or an 'irrotational' flow field.

15:02

📘 Detailed Analysis of Divergence in a Given Vector Field

The script provides a detailed analysis of a specific vector field, using visual and mathematical approaches to understand its divergence properties. It discusses the uniformity of vector magnitudes in the field and how this relates to the flow entering and leaving a neighborhood around a point. The mathematical calculation of divergence for this field is presented, leading to the conclusion that the divergence is zero everywhere, indicating an incompressible flow with no net gain or loss of fluid.

20:05

🌐 Visualizing and Calculating Curl in a Radial Vector Field

This paragraph examines the curl in a radial vector field, where vectors point away from the origin with unit magnitude. A visual representation is used to demonstrate the flow around a chosen point, highlighting the imbalance in vector strength on opposite sides of the point, which leads to rotation. The calculation of curl is performed, resulting in a non-zero vector that indicates the direction and sense of rotation. The paragraph concludes with a discussion on the physical interpretation of the curl result, relating it to the rotation of a paddle within the flow.

25:07

📊 Evaluating Divergence and Curl at Specific Points

The script discusses the evaluation of divergence and curl at specific points within a vector field. It emphasizes that these values can change depending on the point being considered and are not necessarily constant across the entire field. The process involves setting up the mathematical expressions for divergence and curl and then substituting the coordinates of the point of interest. The results provide insights into the local behavior of the flow, such as whether fluid is being gained or lost, or the direction and magnitude of rotation at that point.

30:09

🔧 Practical Application of Divergence and Curl in Vector Fields

The final paragraph of the script summarizes the practical applications of divergence and curl in understanding vector fields. It reinforces the concepts learned and encourages students to apply these mathematical tools to analyze the behavior of fluid flows and other physical phenomena represented by vector fields. The importance of careful calculation and the physical interpretation of the results is highlighted, preparing students for further studies in calculus and vector analysis.

Mindmap

Keywords

💡Vector Field

A vector field is a concept in physics and mathematics that assigns a vector to every point in space. In the context of the video, it represents the flow of a substance, such as fluid or electricity, across a field. The video discusses how vector fields can be used to describe the behavior of these flows, with the instructor using the analogy of wind across the surface of a pond to help visualize this concept.

💡Divergence

Divergence is a measure of how much a vector field is spreading out or converging at a given point. It is calculated as a scalar value that indicates whether more fluid is entering or leaving a small neighborhood around a point. The video emphasizes that a positive divergence indicates a net flow of fluid away from the point, while a negative divergence suggests a net flow towards the point, and a zero divergence means the flow is balanced.

💡Curl

Curl is a measure of the rotational tendency within a vector field. It quantifies the amount of 'spin' or rotation happening at a point in the field. The video script uses the analogy of a paddle wheel to explain curl; if the vector field were to cause the wheel to spin, it would indicate a non-zero curl. A positive curl indicates counterclockwise rotation, while a negative curl indicates clockwise rotation.

💡Incompressible

In the script, the term 'incompressible' is used to describe a situation where the amount of fluid entering and leaving a neighborhood around a point is the same, resulting in a divergence of zero. This concept is applied to vector fields to indicate that they do not gain or lose fluid in a given region, which is a key characteristic in fluid dynamics and can be used to describe certain properties of gases and liquids under certain conditions.

💡Irrotational

Irrotational refers to a vector field where the curl is zero at every point, meaning there is no rotation or 'curling' of the flow. The video script explains that if a vector field is irrotational, it implies that there is no spinning motion within the flow, which is a significant property in the study of fluid dynamics and can be related to the conservation of angular momentum.

💡Dot Product

The dot product is a mathematical operation that takes two vectors and returns a scalar. In the context of the video, the dot product is used to calculate the divergence of a vector field. The script explains that the divergence is found by taking the dot product of the del operator with the vector field, which results in a scalar that represents the fluid flow behavior at a point.

💡Cross Product

The cross product is another mathematical operation between two vectors, resulting in a third vector that is perpendicular to the plane containing the original vectors. The video script mentions the cross product in the context of calculating the curl of a vector field, where the del operator crossed with the vector field results in a vector that represents the rotational tendency of the flow.

💡Del Operator

The del operator, often represented by the vector '∇', is a vector differential operator used in vector calculus. In the video, the del operator is used in the calculation of both divergence and curl. It is applied to the vector field to find the partial derivatives with respect to each coordinate, which are essential steps in determining the properties of the flow at a point.

💡Partial Derivative

A partial derivative is a derivative of a function with multiple variables, with only one variable being considered for the derivative at a time while the others are held constant. The video script discusses partial derivatives in the context of finding both divergence and curl, where the partial derivatives with respect to x, y, and z are taken for each component of the vector field.

💡Scalar and Vector

Scalar and vector are two types of quantities in physics and mathematics. A scalar is a quantity that is fully described by its magnitude, while a vector has both magnitude and direction. The video script differentiates between the scalar nature of divergence, which is a measure of fluid flow at a point, and the vector nature of curl, which describes the rotational tendency with both direction and magnitude.

Highlights

Introduction to the concept of vector fields and their characteristics.

Explanation of how vector fields represent the flow of substances like fluid or electricity.

Divergence defined as a measure of flow entering or leaving a neighborhood around a point.

Curl introduced as a measure of the rotation or spinning of the flow within a vector field.

The significance of divergence in determining if a vector field is incompressible.

Use of the del operator to calculate divergence and its scalar nature.

Illustration of how divergence can indicate fluid gathering or dispersing at a point.

The concept of curl as it relates to the tendency of fluid to rotate, using the water wheel analogy.

Curl's calculation using the cross product and its vector outcome indicating direction and magnitude of spin.

Differentiation between positive and negative divergence and their implications on fluid dynamics.

Positive curl indicating counterclockwise rotation and negative curl indicating clockwise rotation.

The irrotational property of a vector field when curl is zero at every point.

Practical examples demonstrating the calculation of divergence and curl for specific vector fields.

The impact of the vector field's components on the resulting divergence and curl values.

Visual representation of vector fields to aid in understanding divergence and curl.

The importance of the right-hand rule in interpreting the direction of curl.

How the magnitude of the curl vector relates to the maximum rate of rotation.

Conditions under which the maximum rotation occurs in relation to the curl vector.

Transcripts

Browse More Related Video

Vector Fields, Divergence, and Curl

Divergence and Curl (Vector Fields)

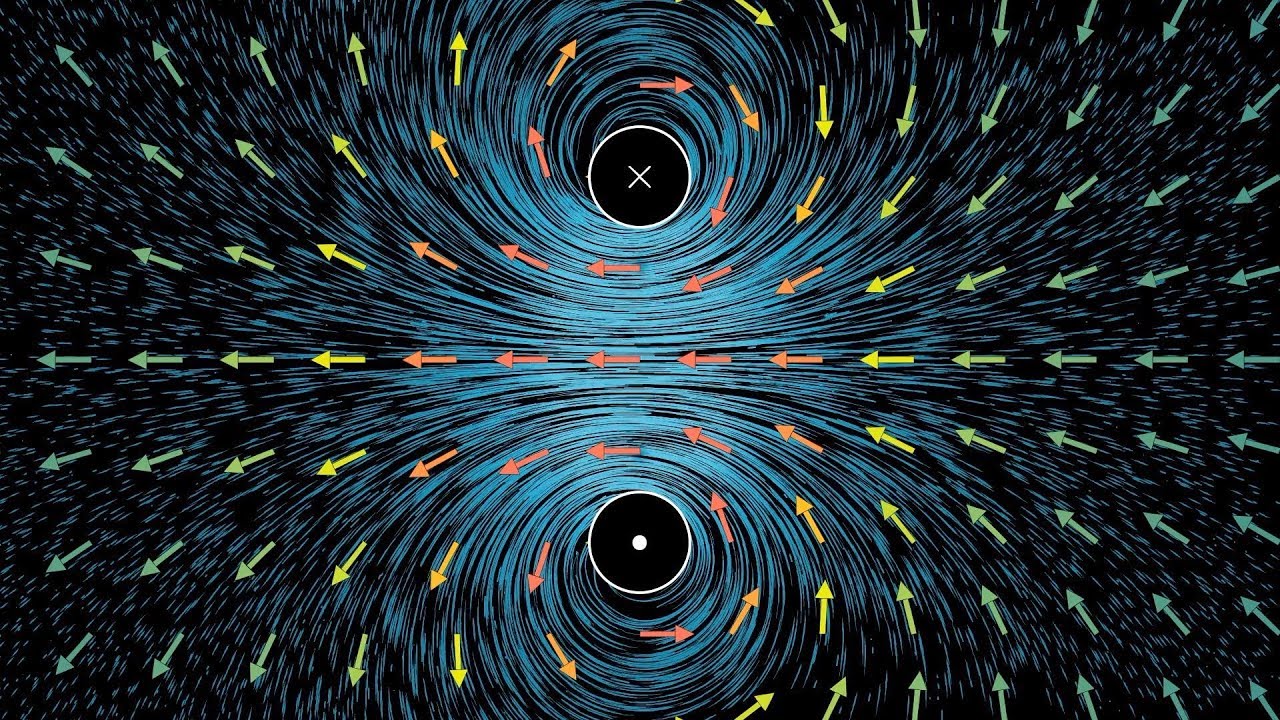
Divergence and curl: The language of Maxwell's equations, fluid flow, and more

Fluid flow and vector fields | Multivariable calculus | Khan Academy

Lec 30: Line integrals in space, curl, exactness... | MIT 18.02 Multivariable Calculus, Fall 2007

2d curl intuition

Related Tags

Vector Fields Divergence Curl Fluid Flow Electricity Flow Calculus Concepts Mathematics Education Field Behavior Scalar Measurement Vector Analysis