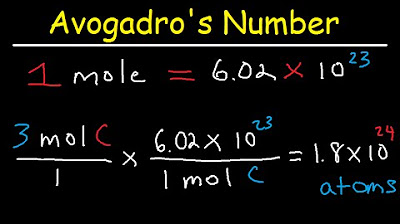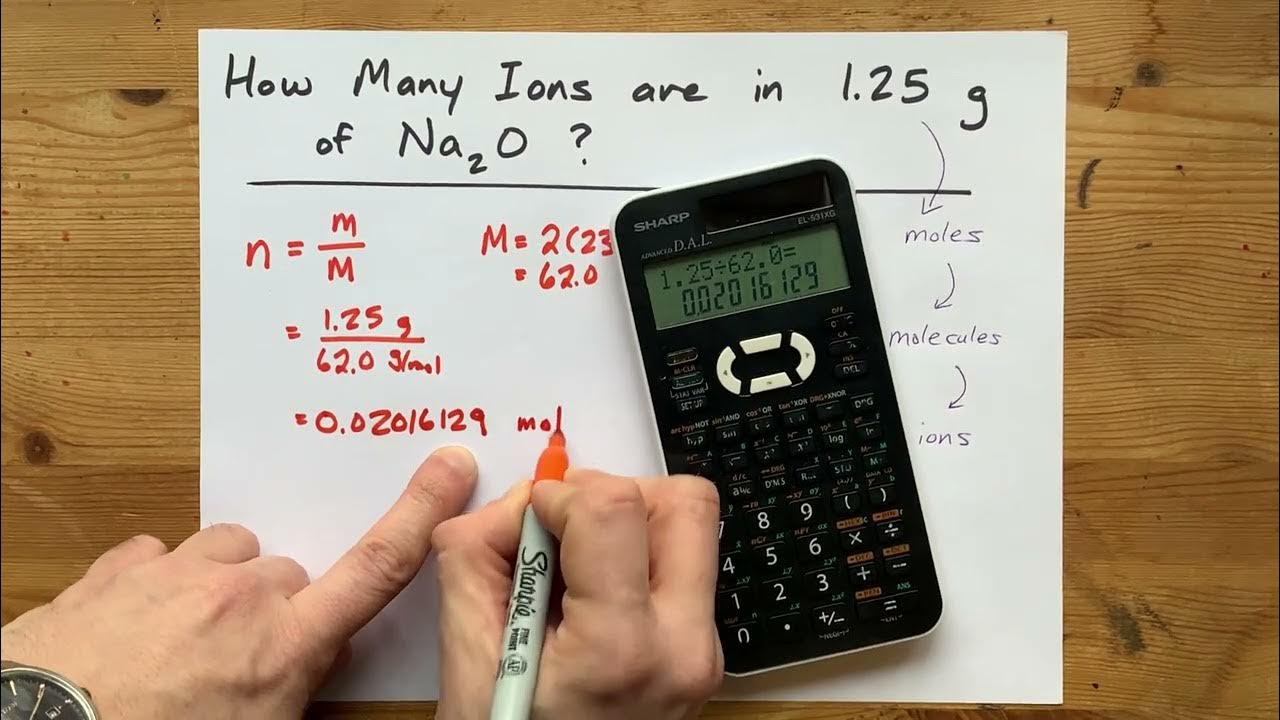How many atoms of Aluminum are in 0.250 grams of aluminum?

chemistNATE
11 Feb 202303:32
EducationalLearning
32 Likes 10 Comments

TLDRThis script demonstrates the process of calculating the number of aluminum atoms in 0.25 grams of the element. It involves converting grams to moles using the atomic mass of aluminum (27.0 g/mol), and then multiplying the resulting moles by Avogadro's number (6.022 x 10^23) to find the atom count. The video emphasizes the importance of significant figures, rounding the final result to 5.58 x 10^21 atoms, aligning with the precision of the given atomic mass.

Takeaways
  • 🔍 To determine the number of atoms in a given mass of aluminum, you must first convert grams to moles using the atomic mass of aluminum.
  • ⚖️ The molar mass of aluminum is approximately 27.0 grams per mole, which is used to divide the mass of aluminum to find moles.
  • 📐 The conversion from grams to moles is represented by the formula n = mass / molar mass.
  • 📉 The initial mass of aluminum provided in the script is 0.250 grams.
  • 🔢 After dividing 0.250 grams by the molar mass of aluminum, the result is approximately 0.00925926 moles.
  • 🌌 To find the number of atoms, multiply the number of moles by Avogadro's number, which is 6.022 × 10^23 atoms per mole.
  • 🔄 The script demonstrates the use of a calculator to perform the necessary scientific calculations, including exponentiation.
  • 📲 The script mentions the use of a calculator's 'exp' or 'e' button to input exponential notation for large numbers.
  • 📝 The final result of the calculation is approximately 5.5759 × 10^21 atoms of aluminum.
  • 📈 The importance of significant figures is emphasized, with the final answer rounded to three significant figures, resulting in 5.58 × 10^21 atoms.
  • 📚 The script suggests that the periodic table used may have inaccuracies, as it only provides three significant figures for the atomic mass of aluminum.
Q & A

  • What is the first step to determine the number of atoms in a given mass of aluminum?

    -The first step is to convert grams into moles by dividing the mass by the atomic mass of aluminum.

  • What is the atomic mass of aluminum used in the script?

    -The atomic mass of aluminum used in the script is 27.0 grams per mole.

  • How many moles of aluminum are in 0.25 grams according to the script?

    -There are approximately 0.00925926 moles of aluminum in 0.25 grams.

  • What is Avogadro's number and how is it used in this context?

    -Avogadro's number is 6.022 x 10^23, and it is used to convert moles of a substance into the number of individual atoms or molecules.

  • How does one convert moles of aluminum to atoms?

    -To convert moles to atoms, multiply the number of moles by Avogadro's number.

  • What is the significance of scientific notation in the calculation presented in the script?

    -Scientific notation is used to express very large numbers, such as the number of atoms, in a more manageable form.

  • What is the final number of aluminum atoms calculated in the script?

    -The final number of aluminum atoms calculated is 5.5759 x 10^21.

  • Why is it important to consider significant figures in scientific calculations?

    -Significant figures are important to ensure the precision of the result is consistent with the precision of the measurements and calculations involved.

  • How many significant figures are in the original number given in the script?

    -The original number given in the script has three significant figures.

  • What is the final answer rounded to in terms of significant figures?

    -The final answer is rounded to three significant figures, which is 5.58 x 10^21 atoms.

  • Why does the script mention that leading zeros do not count in significant figures?

    -Leading zeros are not counted in significant figures because they do not contribute to the precision of the number; only the non-zero digits and the first zero after a decimal point are considered significant.

Outlines
00:00
🔍 Calculating Aluminum Atoms from Grams

This paragraph explains the process of determining the number of aluminum atoms in a given mass. It starts by emphasizing the need to convert grams to moles using the atomic mass of aluminum, which is approximately 27.0 grams per mole. The molar mass is then used to find the number of moles in 0.25 grams of aluminum, resulting in approximately 0.009259 moles. The next step involves multiplying this number of moles by Avogadro's number (6.022 x 10^23) to convert moles to the number of atoms. The calculation yields approximately 5.5759 x 10^21 atoms. The paragraph concludes by discussing the importance of significant figures, rounding the final answer to three significant figures, which is 5.58 x 10^21 atoms.

Mindmap
Keywords
💡Aluminum
Aluminum is a chemical element with the symbol Al and atomic number 13. It is a silvery-white, soft, nonmagnetic, and ductile metal in the boron group. In the script, aluminum is the subject of the calculation, where the goal is to determine the number of atoms present in a given mass of aluminum.
💡Atoms
Atoms are the basic units of matter, consisting of a nucleus and electrons. They are the smallest unit of an element that retains the properties of that element. The video script focuses on counting the number of aluminum atoms in a specific mass, emphasizing the fundamental nature of atoms in chemical calculations.
💡Grams
Grams is a unit of mass in the metric system, equivalent to one-thousandth of a kilogram. In the script, grams are the initial unit of measurement for the mass of aluminum, which is then converted to moles to facilitate the calculation of the number of atoms.
💡Moles
Moles are a unit of measurement used in chemistry to express amounts of a chemical substance. One mole contains Avogadro's number of entities, which can be atoms, molecules, ions, or other particles. The script explains the conversion from grams to moles as a necessary step in determining the number of aluminum atoms.
💡Molar Mass
Molar mass is the mass of one mole of a substance, usually expressed in grams per mole (g/mol). It is numerically equivalent to the substance's atomic or molecular mass in atomic mass units (amu). In the script, the molar mass of aluminum (27.0 g/mol) is used to convert grams of aluminum to moles.
💡Avogadro's Number
Avogadro's number is the number of constituent particles, usually atoms or molecules, that are contained in one mole of a substance. It is approximately 6.022 x 10^23 particles per mole. The script uses Avogadro's number to convert moles of aluminum to the actual number of atoms.
💡Significant Figures
Significant figures are the digits in a number that carry meaningful information about its precision. The script emphasizes the importance of reporting the number of atoms with the correct number of significant figures, which is three in this case, to maintain the accuracy of the result.
💡Scientific Notation
Scientific notation is a way of expressing numbers that are too large or too small to be conveniently written in decimal form. It is typically written as a product of a number between 1 and 10 and a power of 10. The script uses scientific notation to express the large number of atoms resulting from the calculation.
💡Calculator
A calculator is an electronic device used to perform arithmetic operations and calculations. In the script, the use of a calculator is demonstrated to perform the necessary mathematical operations to convert grams to moles and then to atoms.
💡Exponent
An exponent is a number that indicates how many times a base number is multiplied by itself in an expression. In the script, the exponent is used in the context of scientific notation (e.g., 10^23) to express Avogadro's number and the resulting number of atoms.
💡Conversion
Conversion in this context refers to the process of changing one form of measurement to another, such as converting grams to moles or moles to atoms. The script outlines the steps for converting the mass of aluminum to the number of atoms by performing these conversions.
Highlights

The process of counting atoms in a given mass of aluminum involves converting grams to moles and then moles to atoms.

Conversion from grams to moles is achieved by dividing the mass by the atomic mass of aluminum.

Aluminum's molar mass is approximately 27.0 grams per mole.

Moles are then converted to atoms by multiplying by Avogadro's number.

Avogadro's number is used because aluminum is composed of atoms, not molecules.

The formula for calculating the number of particles is the number of moles times Avogadro's number.

Avogadro's number is approximately 6.022 x 10^23.

The calculation of moles of aluminum results in approximately 0.00926 moles.

Multiplying the moles of aluminum by Avogadro's number gives the number of atoms.

The result of the calculation is approximately 5.5759 x 10^21 atoms of aluminum.

Significant figures in the original number and the periodic table guide the final answer's precision.

The final answer should have three significant figures, rounded appropriately.

Leading zeros are not counted when determining significant figures, but trailing zeros after a decimal are counted.

The importance of using scientific notation for large numbers in calculations is emphasized.

The transcript provides a step-by-step guide on how to perform the calculations.

The transcript explains the use of the 'exp' button on calculators for scientific notation.

The transcript encourages asking questions for further clarification.

The transcript concludes with a summary of the process and an invitation for further engagement.

Transcripts

Browse More Related Video

How many molecules are in 76.3 g of N2O4 ?

Very Common Mole Questions

How many moles are in 27.0 g of H2O ?

Avogadro's Number, The Mole, Grams, Atoms, Molar Mass Calculations - Introduction

The Mole: Avogadro's Number and Stoichiometry

How many ions are in 1.25 grams of Na2O?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Aluminum AtomsMolar MassAvogadro's NumberScientific CalculationChemistry TutorialMole ConversionAtomic MassPeriodic TableSignificant FiguresEducational ContentSTEM Learning