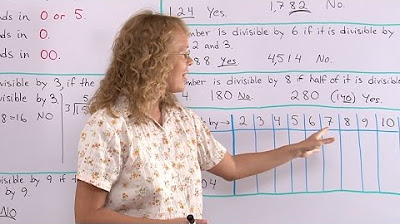You, me, and my first International Math Olympiad problem
TLDRIn this educational video, the host tackles a 1975 International Mathematical Olympiad (IMO) problem, which involves calculating the sum of digits of a number raised to a high power, specifically 4444 to the 4444 power. The host explains the concept of digit sum and demonstrates the process of finding the sum of digits iteratively. Using logarithms to estimate the number of digits and divisibility rules, the host deduces that the final digit sum is congruent to 7 modulo 9, concluding that the answer is 7. The video is a testament to the host's ability to understand and solve complex mathematical problems, inspiring viewers with a clear and engaging explanation.
Takeaways
- 📚 The speaker is attempting to solve a 1975 International Mathematical Olympiad (IMO) question involving the sum of digits of a large number.
- 🤔 The speaker admits that they usually don't understand IMO questions, but this one they were able to both understand and solve.
- 🔢 The problem involves calculating the sum of digits of the number 4...4 (with 4444 fours) raised to the power of 4...4 (also with 4444 fours).
- 📝 The speaker introduces a notation for the sum of digits function, denoted as 's', to simplify the explanation and calculations.
- 📉 The speaker explains that the digit sum function reduces the size of the number quickly, which is important for understanding the problem's complexity.
- 🔑 The speaker uses logarithms to establish an upper bound for the number of digits in the given large number, which is crucial for setting limits on the problem.
- 📈 The speaker warns about the pitfalls of assuming that a larger number has a larger digit sum, emphasizing the importance of careful consideration in inequalities.
- 🔍 The speaker investigates the digit sum function by examining the maximum digit sums for two-digit and three-digit numbers, providing a foundation for the larger calculations.
- 🔗 The speaker connects the digit sum to divisibility by 9, explaining that a number is divisible by 9 if the sum of its digits is divisible by 9.
- 🔄 The speaker uses modular arithmetic to simplify the problem by breaking it down into smaller, more manageable parts, focusing on the remainders when divided by 9.
- 🎯 The final conclusion is that the sum of the digits of the sum of the digits of the sum of the digits of the given number is 7, which is a significant insight into the problem.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is solving a 1975 International Mathematical Olympiad (IMO) question involving the sum of digits of a power of a number.
What is the specific IMO question being solved in the script?
-The specific IMO question is to find the sum of the digits of the sum of the digits of the sum of the digits of the number 4444 to the power of 4444.
What is the term 'digit sum' as used in the script?
-The term 'digit sum' refers to the sum of the individual digits of a number. For example, the digit sum of 4997 is 4 + 9 + 9 + 7 = 29.
What is the significance of the logarithm in the script?
-The logarithm is used to estimate the number of digits in the number 4444 raised to the power of 4444, which helps in setting an upper bound for the digit sum calculations.
Why is the digit sum of a number congruent to the number itself modulo 9?
-The digit sum of a number is congruent to the number itself modulo 9 because of the properties of divisibility by 9, which states that a number is divisible by 9 if the sum of its digits is divisible by 9.
What is the maximum digit sum for a two-digit number?
-The maximum digit sum for a two-digit number is 18, which occurs when the number is 99 (9 + 9).
What is the concept of 'teaching sum' used in the script?
-The 'teaching sum' is a recursive application of the digit sum operation. It refers to the sum of the digits of the digit sum of the number, and this process can be repeated multiple times as required by the problem.
Why is it important to be careful with inequalities when discussing digit sums?
-It is important to be careful with inequalities because having a larger number does not necessarily mean it has a larger digit sum. For example, 1111 has a digit sum of 4, while 17 has a digit sum of 8, even though 1111 is a larger number.
How does the script use the concept of congruence in modulo arithmetic?
-The script uses congruence in modulo arithmetic to simplify the power of 4444 to the power of 4444 by breaking it down into smaller powers and using the fact that powers of numbers cycle in their remainders when divided by 9.
What is the final answer to the IMO question posed in the script?
-The final answer to the IMO question is that the sum of the digits of the sum of the digits of the sum of the digits of the number 4444 to the power of 4444 is 7.
What is the significance of the number 9 in the digit sum calculations?
-The number 9 is significant because it is the base for the digit sum calculations. When a number is congruent to its digit sum modulo 9, it simplifies the process of finding the digit sum of large powers.
Outlines
📚 Introduction to Mathematical Olympiad Problem
The speaker introduces a Mathematical Olympiad (IMO) problem from 1975, explaining that it's unique because they not only understand it but also found a solution. The problem involves calculating the sum of the digits of the number 4^4444, and then repeatedly summing the digits of the results until a single digit is obtained. The speaker humorously admits they've never been smart enough for the IMO but will attempt to solve this problem, starting with an explanation of the 'digit sum' concept and providing an example with the number 49972.
🔍 Investigating the Digit Sum Function
The speaker delves into the properties of the digit sum function, explaining that it can be used to establish an upper bound for the problem at hand. They use logarithms to determine the number of digits in 4^4444, which helps to set an upper limit for the digit sum. The explanation includes a caution about the pitfalls of assuming that a larger number will have a larger digit sum, illustrated with examples, and emphasizes the importance of careful handling of inequalities.
🔢 Applying Bounds to the Digit Sum Problem
Building on the previous discussion, the speaker applies the concept of digit sum bounds to the original problem. They calculate an upper limit for the digit sum of 4^4444 by assuming all digits are 9, resulting in a number with 17776 digits. The speaker then iteratively applies the digit sum function to this number, being careful to replace each digit with 9 for the upper bound calculation, and finds that the digit sum of the digit sum of 4^4444 is less than 46.
🔄 Exploring Divisibility and Digit Sums
The speaker introduces a connection between digit sums and divisibility by 9, explaining that a number is divisible by 9 if the sum of its digits is also divisible by 9. They demonstrate this with the number 125 and then apply this concept to the problem of finding the digit sum of 4^4444. The process involves breaking down the exponentiation into smaller powers of 4, calculating the digit sum of each, and using modular arithmetic to find a pattern that leads to the conclusion that the digit sum is congruent to 7 modulo 9.
🧩 Piecing Together the Final Digit Sum
The speaker continues the modular arithmetic approach, breaking down the exponentiation of 4^4444 into manageable parts and calculating the congruence of each part modulo 9. They build upon previous results, squaring the base number and its powers, to find that the digit sum of 4^4444 is congruent to 7 modulo 9. The process involves careful tracking of the powers and their respective digit sums, leading to the final insight that the digit sum of the original problem is indeed 7.
🎉 Solving the IMO Problem and Conclusion
The speaker concludes the video by verifying their solution to the IMO problem, demonstrating that the digit sum of 4^4444 is 7. They express excitement and disbelief at having solved an IMO problem and show the result using a calculator. The speaker wraps up by reflecting on the satisfaction of understanding and solving the problem, ending the video on a high note.
Mindmap
Keywords
💡International Mathematical Olympiad (IMO)
💡Digit Sum
💡Powers of Numbers
💡Logarithm
💡Modular Arithmetic
💡Inequality
💡Divisibility Rule
💡Recursive Calculation
💡Congruence
💡Base-10
💡Bounding
Highlights
Introduction to solving a 1975 International Mathematical Olympiad (IMO) problem.
Explaining the task of finding the sum of digits of the sum of digits of a number, iteratively.
The choice of the number 4444 to the power of 4444 as an example for the iterative digit sum problem.
Using logarithms to estimate the number of digits in a large power, as an upper bound for the digit sum.
Clarification on the maximum digit sum for two-digit numbers and the extension to three-digit numbers.
Warning about the potential inequality fallacy when comparing digit sums of different numbers.
The process of iteratively calculating the digit sum of the power of 4444, using congruences modulo 9.
The discovery that the digit sum of powers of 4444 follows a pattern when considered modulo 9.
Building an argument for the upper bound of the digit sum based on the pattern found.
The realization that the digit sum of the digit sum of the number is congruent to 7 modulo 9.
Concluding that the final digit sum of the iterative process must be 7, based on the established pattern and bounds.
Demonstration of using a calculator to verify the solution and confirming the digit sum is indeed 7.
Reflection on the successful understanding and solving of an IMO problem, which is considered a significant achievement.
Emphasizing the importance of careful consideration in mathematical proofs and problem-solving.
The joy and satisfaction expressed in being able to solve an IMO question, highlighting the educational value of such challenges.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: