Gibbs’ phase rule
TLDRThe video script delves into the Gibbs phase rule, a fundamental principle in phase diagrams. It explains the relationship between the number of phases (P), components (C), and degrees of freedom (F) in an alloy system. The rule is articulated as F = C - P + 2 (or 1, if pressure is constant), highlighting how equilibrium conditions limit variable specification. Illustrated with examples, the script clarifies how degrees of freedom decrease with more phases, culminating in invariant reactions like the eutectic point, where all variables are fixed.
Takeaways
- 📚 The Gibbs phase rule is a fundamental principle in phase diagrams that relates the number of phases in equilibrium to the number of components in a system.
- 🔍 The degrees of freedom (F) is defined as the number of thermodynamic variables that can be independently specified without changing the phases in equilibrium.
- 🌡️ Thermodynamic variables for Gibbs phase rule include pressure and temperature, and in some cases, composition variables such as phase compositions.
- 🧩 The phase compositions are the relevant composition variables for the application of Gibbs phase rule, not the overall alloy composition.
- 📉 If there are C components in an alloy, to specify the composition of a phase, C-1 composition variables are needed, as the last component can be derived from the others.
- 🔢 The total number of variables (V) is calculated as P*(C-1) plus the number of thermodynamic variables (2 for pressure and temperature, or 1 if pressure is constant).
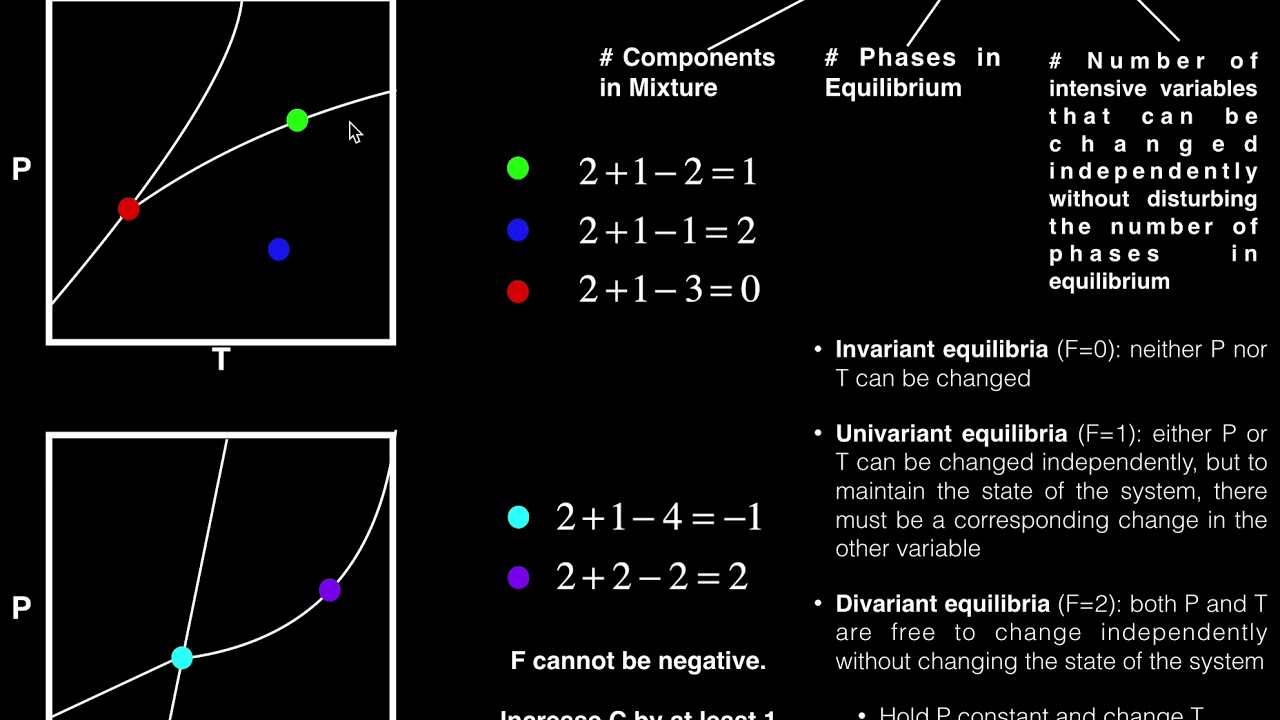
- ⚖️ The degrees of freedom (F) is calculated as the number of components minus the number of phases plus 2 (or 1 if pressure is constant).
- 💧 In a single phase equilibrium, the degrees of freedom allow for the independent specification of both the phase composition and temperature.
- 🌐 In a two-phase equilibrium, the degrees of freedom decrease to 1, meaning only one variable can be freely specified, with the others determined by equilibrium conditions.
- 🔮 In a three-phase equilibrium, the degrees of freedom are zero, indicating that all variables are fixed and cannot be independently changed.
- 📉 The eutectic reaction, where three phases coexist at a fixed temperature and composition, is an example of an invariant reaction with zero degrees of freedom, represented by a horizontal line in phase diagrams.
Q & A
What is the Gibbs phase rule?
-The Gibbs phase rule is a fundamental principle in thermodynamics that relates the number of phases in equilibrium (P), the number of components in a system (C), and the degrees of freedom (F). It helps to determine the number of independent variables that can be specified without changing the equilibrium state of a system.
What are the thermodynamic variables considered in the Gibbs phase rule?
-The thermodynamic variables considered in the Gibbs phase rule are pressure and temperature, as well as the composition variables, specifically the phase compositions. The overall alloy composition is not considered a variable unless it is also the phase composition in a single phase equilibrium.
How are the degrees of freedom defined in the context of the Gibbs phase rule?
-The degrees of freedom (F) are defined as the number of thermodynamic variables that can be specified independently without changing the phases in equilibrium. It is determined by the equilibrium conditions between the variables.
What is the formula for calculating the degrees of freedom according to the Gibbs phase rule?
-The formula for calculating the degrees of freedom (F) according to the Gibbs phase rule is F = C - P + 2, where C is the number of components and P is the number of phases in equilibrium. If pressure is fixed, the formula becomes F = C - P + 1.
How many composition variables are needed for a phase with C components?
-For a phase with C components, you need C - 1 composition variables because the composition of the last component can be found by 1 minus the sum of the other compositions.
What is the significance of the number of composition variables in relation to the number of phases?
-The total number of composition variables needed is P times (C - 1), where P is the number of phases. This is because for each phase, you need to specify the composition of C - 1 components.
What happens to the degrees of freedom when the number of phases increases?
-As the number of phases increases, the degrees of freedom generally decrease because more variables are determined by the equilibrium conditions, leaving fewer variables that can be specified independently.
What is an example of a single phase equilibrium in the context of the Gibbs phase rule?
-An example of a single phase equilibrium is a single phase liquid in equilibrium, where the variables are the liquid composition (C_L) and temperature (T). According to the Gibbs phase rule, you have 2 degrees of freedom, meaning you can independently specify both the liquid composition and the temperature.
How does the Gibbs phase rule apply to a two-phase equilibrium like liquid plus alpha?
-In a two-phase equilibrium like liquid plus alpha, you have 3 variables (compositions of both phases and temperature), but the degrees of freedom is only 1. This means you can specify one variable (either temperature or one of the compositions), and the other two will be determined by the equilibrium conditions.
What is the significance of zero degrees of freedom in a three-phase equilibrium?
-Zero degrees of freedom in a three-phase equilibrium indicates that there are no independent variables that can be specified without changing the equilibrium state. All variables, including the compositions of each phase and the temperature, are fixed at specific values, which is characteristic of an invariant reaction, such as the eutectic reaction.
Why is the eutectic reaction considered an invariant reaction in a phase diagram?
-The eutectic reaction is considered an invariant reaction because it represents a three-phase equilibrium with zero degrees of freedom. The compositions of the phases and the temperature are fixed and cannot be varied independently, which is why it is represented by a horizontal line in a phase diagram.
Outlines
🔍 Introduction to Gibbs Phase Rule
The script begins with an introduction to the Gibbs phase rule, a fundamental concept in phase diagrams. It explains the relationship between the number of phases (P), the number of components (C), and the degrees of freedom (F). The concept of thermodynamic variables, including pressure, temperature, and phase compositions, is discussed, with an emphasis on their role in phase equilibrium. The script clarifies that only phase compositions are considered as variables for the Gibbs phase rule, while overall alloy composition is not a variable unless in a single phase equilibrium.
📚 Understanding Composition Variables and Degrees of Freedom
This paragraph delves into the specifics of composition variables, explaining that for C components, one needs to specify C-1 compositions for each phase, as the last component can be derived from the others. The total number of variables (V) is calculated as P times (C-1) plus the number of thermodynamic variables, which can be either 2 (pressure and temperature) or 1 (if pressure is constant). The degrees of freedom are then introduced as the number of thermodynamic variables that can be independently specified without altering the equilibrium of phases.
📉 Defining Degrees of Freedom and Gibbs Phase Rule
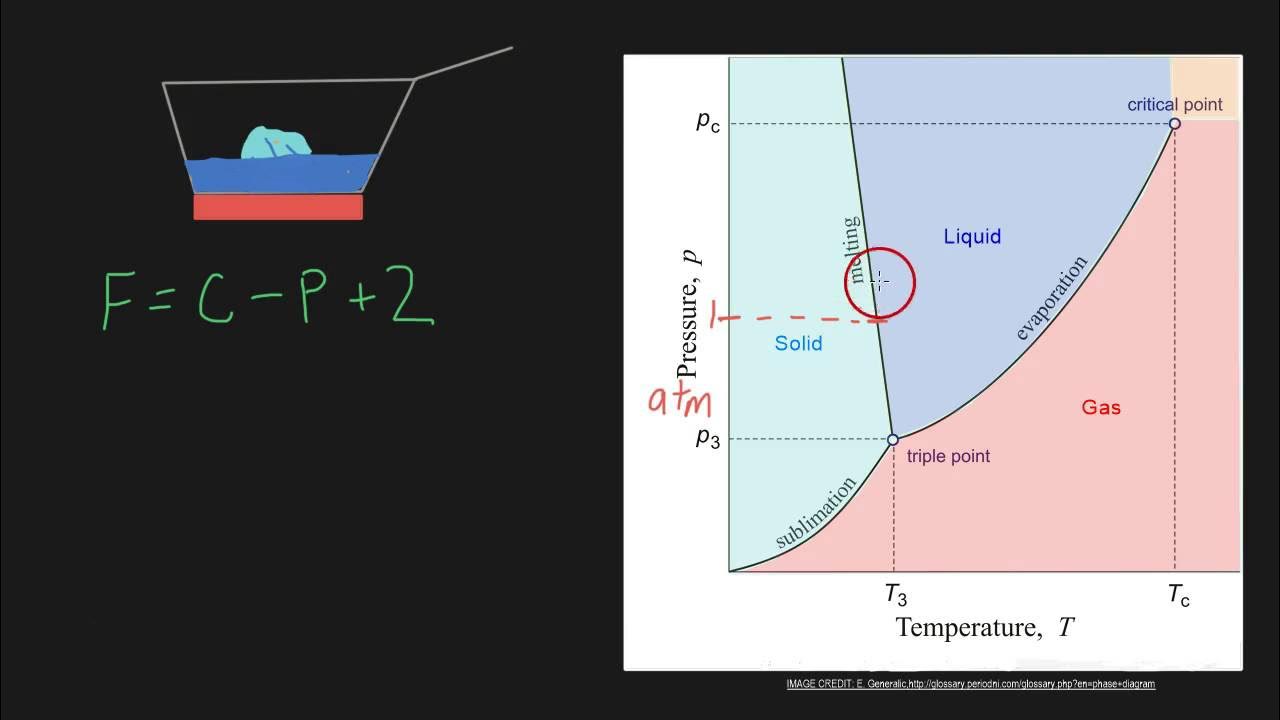
The script defines degrees of freedom as the number of thermodynamic variables that can be independently set without changing the equilibrium of phases. It explains that equilibrium conditions limit the number of variables that can be freely specified. The Gibbs phase rule is then presented in its formulaic form, F = C - P + 2, which accounts for both pressure and temperature as variables. The rule is simplified for cases where pressure is fixed, changing the formula to F = C - P + 1.
📈 Applying Gibbs Phase Rule to Phase Diagrams
The script applies the Gibbs phase rule to a binary phase diagram, specifically the lead-tin diagram, to illustrate its practical use. It discusses single phase equilibrium, where the degrees of freedom allow for the independent specification of liquid composition and temperature. The example demonstrates how the phase rule can be used to understand the conditions for phase equilibrium in a system with varying components and phases.
🔄 Transition to Multiphase Equilibrium and Its Implications
This paragraph explores the implications of the Gibbs phase rule in multiphase equilibrium, starting with a two-phase equilibrium (liquid plus alpha). It explains how the degrees of freedom decrease as the number of phases increases, limiting the variables that can be independently specified. The script uses the lead-tin diagram to illustrate how specifying temperature fixes the compositions of both phases along the tie line, highlighting the constraints imposed by phase equilibrium.
🌡️ Invariant Reactions and the Concept of Eutectic
The final paragraph discusses the concept of invariant reactions, such as the eutectic reaction, where three phases coexist in equilibrium with zero degrees of freedom. It explains that in such cases, all variables, including compositions and temperature, are fixed. The script uses the eutectic line in the phase diagram to demonstrate this point, emphasizing that invariant reactions are represented by horizontal lines in binary phase diagrams.
Mindmap
Keywords
💡Gibbs phase rule
💡Phases
💡Components
💡Degrees of freedom
💡Thermodynamic variables
💡Phase compositions
💡Equilibrium conditions
💡Binary phase diagram
💡Invariant reaction
💡Eutectic
Highlights
Introduction to Gibbs phase rule and its importance in phase diagrams.
Definition of the key terms: number of phases (P), number of components (C), and degrees of freedom (F).
Explanation of thermodynamic variables relevant to Gibbs phase rule: pressure, temperature, and phase compositions.
Clarification that overall alloy composition is not a variable in Gibbs phase rule unless it equals phase composition.
The formula for calculating the number of composition variables based on the number of components in a phase.
Total number of variables (V) calculation including composition variables and thermodynamic variables like pressure and temperature.
Definition of degrees of freedom as the number of thermodynamic variables that can be specified independently without changing the equilibrium.
The rule for degrees of freedom F: F = C - P + 2 (if both pressure and temperature are variables).
Special case of the Gibbs phase rule when pressure is fixed, leading to F = C - P + 1.
Application of Gibbs phase rule to a binary phase diagram, assuming pressure is constant at 1 atmosphere.
Example of single phase equilibrium and how the degrees of freedom allow for variation of both composition and temperature.
Analysis of two-phase equilibrium (liquid plus alpha) and the resulting decrease in degrees of freedom to one.
Explanation of how specifying temperature in a two-phase equilibrium determines the compositions of both phases.
Three-phase equilibrium scenario and the resulting zero degrees of freedom, indicating fixed compositions and temperature.
The eutectic reaction as an example of an invariant reaction with zero degrees of freedom, where all variables are fixed.
Identification of horizontal lines in binary phase diagrams as indicative of invariant reactions.
Further discussion on invariant reactions and their significance in phase diagrams.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: