Using IVT, MVT, and EVT
TLDRThis educational video script discusses the application and proof of three fundamental calculus theorems: the Intermediate Value Theorem (IVT), the Mean Value Theorem (MVT), and the Extreme Value Theorem (EVT). It emphasizes the conditions necessary for each theorem to hold, such as continuity and differentiability, and provides step-by-step explanations on how to apply them to find specific values or slopes within a given interval. The script also includes strategies for tackling related multiple-choice questions, reinforcing the importance of understanding these theorems in calculus.
Takeaways
- 📚 The script discusses theorems, particularly the Intermediate Value Theorem (IVT), Mean Value Theorem (MVT), and Extreme Value Theorem (EVT), emphasizing their conditions and applications.
- 🔍 For the IVT to apply, the function must be continuous, and the value of interest must lie between the function values at the endpoints of the interval.
- 📈 The MVT requires the function to be differentiable over an interval, and it guarantees the existence of a point where the derivative equals the average rate of change over that interval.
- 🏔 The EVT states that if a function is continuous on a closed interval, it must attain both a maximum and a minimum value within that interval.
- 🤔 The script suggests using the word 'must' as a clue to identify theorem-based questions, prompting students to think about the conditions necessary for each theorem.
- 📉 To find the average rate of change without taking a derivative, one should calculate the slope between two points on the function's graph.
- 📝 When applying the MVT, it's important to identify the interval and the average rate of change, which should equal the derivative at some point within that interval.
- 📉 The script highlights the importance of setting up a problem correctly when applying theorems, ensuring that all necessary conditions are met.
- 📊 The script mentions that EVT is the easiest theorem to prove among the three, as it only requires continuity on a closed interval.
- 🤓 The importance of graphing functions when in doubt is emphasized, as it can help visualize the conditions necessary for the theorems to apply.
- 📋 The script includes multiple-choice questions to test understanding of the theorems, focusing on identifying the correct conditions and theorems for given scenarios.
Q & A
What are the conditions required for the Intermediate Value Theorem (IVT) to hold?
-The conditions for IVT to hold are that the function must be continuous on a closed interval, and the value of interest must fall between the function values at the endpoints of the interval.
What does the Mean Value Theorem (MVT) guarantee?
-MVT guarantees that if a function is differentiable on an open interval and continuous on the closed interval containing that open interval, then there exists at least one point 'c' in the interval where the derivative of the function (f'(c)) is equal to the average rate of change of the function over that interval.
What is the average rate of change of a function over an interval and how is it found?
-The average rate of change of a function over an interval is the slope of the secant line connecting two points on the graph of the function. It is found by taking the difference in the function values at the endpoints of the interval and dividing it by the difference in the x-values of those endpoints.
What is the Extreme Value Theorem (EVT) and what conditions are necessary for it to apply?
-EVT states that if a function is continuous on a closed interval, then the function has both an absolute maximum and an absolute minimum on that interval. The necessary conditions for EVT are that the function must be continuous over the interval, which includes the endpoints.
Why can't we use IVT when the value of interest doesn't fall between the function values at the endpoints?
-We can't use IVT when the value of interest doesn't fall between the function values at the endpoints because IVT specifically states that the function must take on every value between its values at the interval endpoints, including the endpoints themselves.
How does the script differentiate between using IVT, MVT, and EVT in problem-solving?
-The script suggests that if the problem involves finding a value within a range, IVT is likely needed. If the problem is about finding a derivative that equals a certain value, MVT is used. EVT is applied when the problem is about finding an absolute maximum or minimum value within a closed interval.
What is the significance of stating the conditions before applying a theorem in the script?
-Stating the conditions before applying a theorem is important because it ensures that the prerequisites for the theorem's application are met, providing a logical foundation for the subsequent steps in the problem-solving process.
What is the role of the average rate of change in the context of MVT?
-In the context of MVT, the average rate of change is crucial because it must equal the derivative at some point 'c' within the interval. This is the value that MVT guarantees to exist, given the function's differentiability and continuity over the interval.
Why is it important to graph out a function when in doubt about its properties?
-Graphing out a function when in doubt helps visualize the function's behavior, such as continuity, differentiability, and the presence of extreme values. This visual aid can assist in determining the correct theorem to apply and in understanding the function's properties.
How does the script handle multiple-choice questions related to IVT, MVT, and EVT?
-The script advises to read the question carefully, identify the theorem being asked about, and then apply the relevant conditions and theorem guarantees to determine the correct answer. It also suggests graphing out the function if there is any doubt about the function's properties.
Outlines
📚 Introduction to Theorems and IVT Application
This paragraph introduces the concept of theorems, specifically focusing on the Intermediate Value Theorem (IVT). The speaker advises to review the IVT, Mean Value Theorem (MVT), and the Existence Theorem (EVT) if not familiar. The IVT is set up by establishing the condition of a continuous function and identifying two key points within the interval where the function's value is known. The speaker emphasizes the importance of stating these conditions upfront. The theorem guarantees the existence of a value 'r' such that the function 'f' at 'r' equals a specific value, in this case, 7, between the given endpoints. The explanation concludes by reiterating the conditions necessary for IVT to hold: continuity, endpoints, and the interval containing the desired value.
🔍 Mean Value Theorem (MVT) Explained
The second paragraph delves into the Mean Value Theorem (MVT), contrasting it with the IVT by discussing the conditions under which MVT applies. The speaker clarifies that for MVT to be valid, the function must be differentiable over the interval, not just continuous. The average rate of change is identified as a key component, calculated by the difference in function values at two points divided by the difference in their corresponding x-values. The speaker illustrates this with a specific example, aiming to find when the average rate of change is negative six. The MVT guarantees the existence of a point 'c' where the derivative of the function equals the average rate of change, provided the function is differentiable over the interval. The explanation concludes by reiterating the steps needed to apply MVT: stating the conditions, identifying the theorem, and explaining why the theorem's conclusion is valid.
📈 Extreme Value Theorem (EVT) and Its Conditions
The third paragraph introduces the Extreme Value Theorem (EVT), focusing on the conditions required for EVT to be applicable. The speaker explains that EVT is used to establish the existence of absolute maxima or minima within a closed interval where the function is continuous. The speaker emphasizes that the function being continuous over a closed interval is sufficient for EVT to guarantee the existence of extreme values. The explanation is straightforward, highlighting the simplicity of applying EVT compared to IVT and MVT, and concludes by reinforcing the importance of stating the conditions and the theorem's guarantees.
📘 Multiple Choice Questions on Theorems
The final paragraph presents a series of multiple-choice questions that test the understanding of IVT, MVT, and EVT. The speaker encourages students to discuss the questions with peers and attempt them despite their difficulty. The questions cover various scenarios, such as finding the average rate of change without needing to differentiate, identifying conditions for MVT to guarantee a derivative of zero, and using EVT to establish the existence of an absolute maximum. The speaker also addresses common misconceptions and emphasizes the importance of graphing functions when in doubt. The paragraph concludes with a brief analysis of the answers to the multiple-choice questions, ensuring a clear understanding of when each theorem is applicable.
Mindmap
Keywords
💡IVT (Intermediate Value Theorem)
💡MVT (Mean Value Theorem)
💡EVT (Extreme Value Theorem)
💡Differentiable
💡Continuous
💡Derivative
💡Average Rate of Change
💡Endpoint
💡Slope
💡Absolute Max/Min
Highlights
Introduction to theorems and the importance of understanding the conditions for the Intermediate Value Theorem (IVT), Mean Value Theorem (MVT), and Extreme Value Theorem (EVT).
Explanation of the setup for an IVT question, emphasizing the need for a continuous function and specific endpoints.
Clarification that the IVT guarantees a value between endpoints if the function is continuous and the value lies between the function values at those endpoints.
Emphasizing five key elements for theorem application: continuity, endpoints, IVT, interval, and being between endpoints.
Transition to Mean Value Theorem (MVT) discussion, focusing on finding a value 'c' where the derivative equals a given slope.
Instruction on identifying ordered pairs for calculating the average rate of change, crucial for MVT application.
Condition for MVT: the function must be differentiable on the interval, not just continuous.
MVT guarantees the existence of a derivative equal to the average rate of change within a given interval.
Differentiation between IVT and MVT based on whether one is looking for a value or a slope.
Introduction to the Extreme Value Theorem (EVT), focusing on the existence of absolute maxima and minima for continuous functions on closed intervals.
Condition for EVT: the function must be continuous on a closed interval to guarantee the existence of maxima and minima.
Explanation of how EVT is the simplest theorem to prove among IVT, MVT, and EVT.
Guidance on approaching multiple-choice questions involving IVT, MVT, and EVT, with an emphasis on understanding the theorems' conditions and guarantees.
Clarification that the average rate of change does not require differentiation, just the slope of the function between two points.
Advice on using graphical representations to aid in understanding and solving theorem-based problems.
Discussion on the application of IVT to guarantee a specific function value based on continuity and endpoints.
Final review of the key steps in applying theorems: stating the condition, what has to be true, and proving why with the necessary information.
Transcripts
Browse More Related Video

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)

2022 Live Review 5 | AP Calculus AB | Focusing on the Big Theorems Including IVT, EVT, & MVT

2021 Live Review 5 | AP Calculus AB | Understanding the Big Theorems Including IVT, EVT, & MVT
Calculus AB Homework 5.1 Rolle's and Mean Value Theorem

Calculus 1: Lecture 3.2 Rolle's Theorem and the Mean Value Theorem
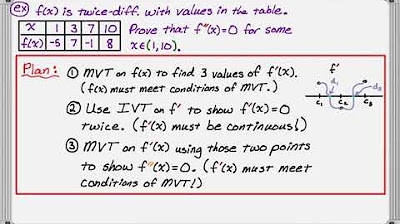
Using MVT on a Table to Prove Second Derivative Equals Zero
5.0 / 5 (0 votes)
Thanks for rating: