Tension Force Physics Problems
TLDRThis educational video script provides a detailed guide on solving tension problems in physics. It begins by calculating the tension in a rope lifting a 50 kg box with an upward acceleration of 2.3 m/s², highlighting that tension exceeds weight in such scenarios. The script then contrasts this with a situation where the box descends slowly, showing tension is less than weight. It proceeds to solve more complex problems involving two ropes and equilibrium, using free body diagrams and trigonometric relationships to find the tension forces in each rope. The importance of verifying calculations by ensuring forces in x and y directions sum to zero is emphasized, reinforcing the concepts taught.
Takeaways
- 📚 The video focuses on solving tension problems in physics, specifically calculating the tension in ropes lifting or moving objects.
- 🔍 To calculate tension, a free body diagram is used to identify forces such as tension, weight, and net force.
- ⏳ When an object is lifted with an upward acceleration, the tension in the rope is greater than the weight force of the object.
- 📈 The formula to calculate tension when lifting with acceleration is \( T = m(g + a) \), where \( m \) is mass, \( g \) is gravitational acceleration, and \( a \) is acceleration.
- 📉 Conversely, when an object descends with a downward acceleration, the tension is less than the weight force.
- 📐 For objects in equilibrium, the net force in all directions must be zero, which allows for the calculation of tension forces in multiple ropes.
- 📝 The components of tension forces (T1x, T1y, T2x, T2y) can be calculated using trigonometric functions based on the angles involved.
- 🔄 To solve for unknown tensions, equations are set up based on equilibrium conditions in both the x and y directions.
- 🔢 Example calculations in the script demonstrate how to find tension in ropes for different scenarios, including lifting with acceleration and slow descent.
- 🔄 The script also shows how to check work by ensuring that the calculated forces in both directions balance out to zero.
- 🔑 The concept of tension is emphasized as a force that acts along a rope, typically due to a pulling action.
Q & A
What is the main topic of the video?
-The main topic of the video is solving tension problems in physics, specifically calculating the tension in ropes lifting or moving objects.
What is the formula used to calculate the tension in the rope when lifting a box?
-The formula used to calculate the tension in the rope is T = m(a + g), where T is the tension, m is the mass of the object, a is the upward acceleration, and g is the gravitational acceleration.
What is the gravitational acceleration (g) in meters per second squared?
-The gravitational acceleration (g) is approximately 9.8 meters per second squared.
How does the tension in the rope compare to the weight force when lifting a box with upward acceleration?
-When lifting a box with upward acceleration, the tension in the rope is greater than the weight force of the box.
What is the weight force of a 50 kg box?
-The weight force of a 50 kg box is calculated as 50 kg * 9.8 m/s², which equals 490 Newtons.
What happens to the tension force when the box is allowed to slowly descend with a downward acceleration?
-When the box is allowed to slowly descend with a downward acceleration, the tension force becomes less than the weight force.
How can you determine the tension in two ropes when a box is in equilibrium?
-In equilibrium, the sum of all forces in the x and y directions must add to zero. By breaking down the components of the tensions in the ropes and setting up equations based on the angles and forces, you can solve for the tension in each rope.
What is the significance of cosine and sine in calculating the components of tension forces?
-Cosine and sine are used to determine the horizontal (x) and vertical (y) components of the tension forces in the ropes, based on the angles at which the ropes are pulling.
How do you check if the calculated tension forces are correct?
-You can check if the calculated tension forces are correct by ensuring that the sum of all forces in the x and y directions add up to zero, confirming that the object remains in equilibrium.
What is the tension in the rope when lifting a 100 kg box with a downward acceleration of 0.75 m/s²?
-The tension in the rope when lifting a 100 kg box with a downward acceleration of 0.75 m/s² is calculated as (100 kg * 9.8 m/s²) - (100 kg * 0.75 m/s²) = 980 N - 75 N = 905 Newtons.
What is the role of equilibrium in solving tension problems?
-Equilibrium is crucial in solving tension problems because it allows us to set up the condition that the net force in all directions must be zero, which helps in solving for unknown tension forces.
Outlines
🔍 Calculating Tension in a Rope Lifting a Box
This paragraph introduces the concept of solving tension problems in physics. It outlines a scenario where a rope is lifting a 50 kg box with an upward acceleration of 2.3 m/s². The tension force in the rope is calculated using a free body diagram, identifying forces such as the upward tension, downward weight, and net force. The net force is determined to be the tension minus the weight force, leading to the equation t - w = m * a. By rearranging and substituting the given values, the tension force is calculated to be 605 Newtons, which is greater than the weight force of 490 Newtons. The paragraph also discusses the case of a box descending with a downward acceleration, resulting in a tension force less than the weight force, calculated to be 452.5 Newtons.
📚 Analyzing Tension in Multiple Ropes with a Free Body Diagram
The second paragraph delves into a more complex problem involving two ropes supporting a box at rest. The box is in equilibrium, implying that the net forces in the x and y directions must sum to zero. The forces are broken down into their x and y components, and the relationships between the tensions t1 and t2 are established. Using trigonometric relationships for the angles involved (60° and 30°), the tensions are related by t1 * cos(60°) = t2 * cos(30°), leading to t1 being 1.732 times t2. By substituting the mass and gravitational acceleration into the equation, t2 is found to be 294 Newtons, and subsequently, t1 is calculated to be approximately 509.2 Newtons. The paragraph concludes with a verification step to ensure that the calculated forces in both directions balance out, confirming the correctness of the solution.
🔄 Verifying Tension Calculations with Force Components
This paragraph continues the verification process of the tension forces calculated in the previous paragraph. It breaks down the forces into their x and y components and checks if they sum to zero, which is a requirement for the box to remain at rest. The x components of the forces are shown to cancel each other out, and the y components are verified to balance the downward weight force of 588 Newtons. The paragraph emphasizes the importance of this verification step to ensure the accuracy of the calculated tension forces in the ropes.
🔄 Solving Tension Problems with Equilibrium Conditions
The final paragraph presents a simpler tension problem involving a 100 kg box at rest, supported by two ropes. The forces are analyzed in both the x and y directions, with the y direction being used to calculate t1, the tension in the first rope. Using the equilibrium condition and the sine of the angle (60°), t1 is found to be approximately 1132 Newtons. The x direction is then used to calculate t2, the tension in the second rope, which is found to be 566 Newtons by equating t1x to t2 and using the cosine of the angle. The paragraph concludes with a reiteration of the importance of checking the work to ensure that all forces in both directions sum to zero, confirming the equilibrium state and the correctness of the tension calculations.
Mindmap
Keywords
💡Tension Force
💡Free Body Diagram
💡Net Force
💡Weight Force
💡Equilibrium
💡Components of Force
💡Acceleration
💡Gravitational Acceleration
💡Trigonometric Functions
💡Substitution Method
Highlights
The video focuses on solving tension problems in physics.
A 50 kg box is lifted with an upward acceleration of 2.3 m/s² using a rope.
Tension force is calculated through a free body diagram identifying forces in play.
Net force is the difference between tension and weight force, directed upwards.
The equation for tension force is mass times gravitational acceleration plus vertical acceleration.
Tension force is 605 Newtons when lifting the box upwards.
Tension force is greater than weight force when an object is lifted with acceleration.
When the box descends slowly, the tension force is less than the weight force.
The tension force during slow descent is calculated to be 452.5 Newtons.
For an object in equilibrium, the sum of forces in x and y directions must be zero.
T1 and T2 are broken down into their x and y components to solve for tension forces.
T1x is equal to T2x when the object is at rest.
T1 is 1.732 times T2 based on the cosine of the angles involved.
The weight force is balanced by the sum of T1y and T2y.
T2 is calculated to be 294 Newtons using the equilibrium condition.
T1 is found to be approximately 509.2 Newtons using substitution.
Checking work involves ensuring forces in x and y directions sum to zero.
A simpler problem involves calculating tension in two ropes with a box at rest.
T1 is determined to be about 1132 Newtons using the weight force and sine of the angle.
T2 is found to be 566 Newtons by equating it to T1x.
The final step is to verify that forces in x and y directions balance out.
Tension is a force that acts along a rope, transmitted typically by pulling.
Transcripts
Browse More Related Video

Falling Box with Pulley | Physics with Professor Matt Anderson | M12-23

College Physics 1: Lecture 19 - Interacting Objects, Ropes, and Pulleys
Finding the Tension and Acceleration for a Two Mass Pulley (with Friction)
Physics 15 Torque Example 2 (2 of 7) Mass on Rod and Cable
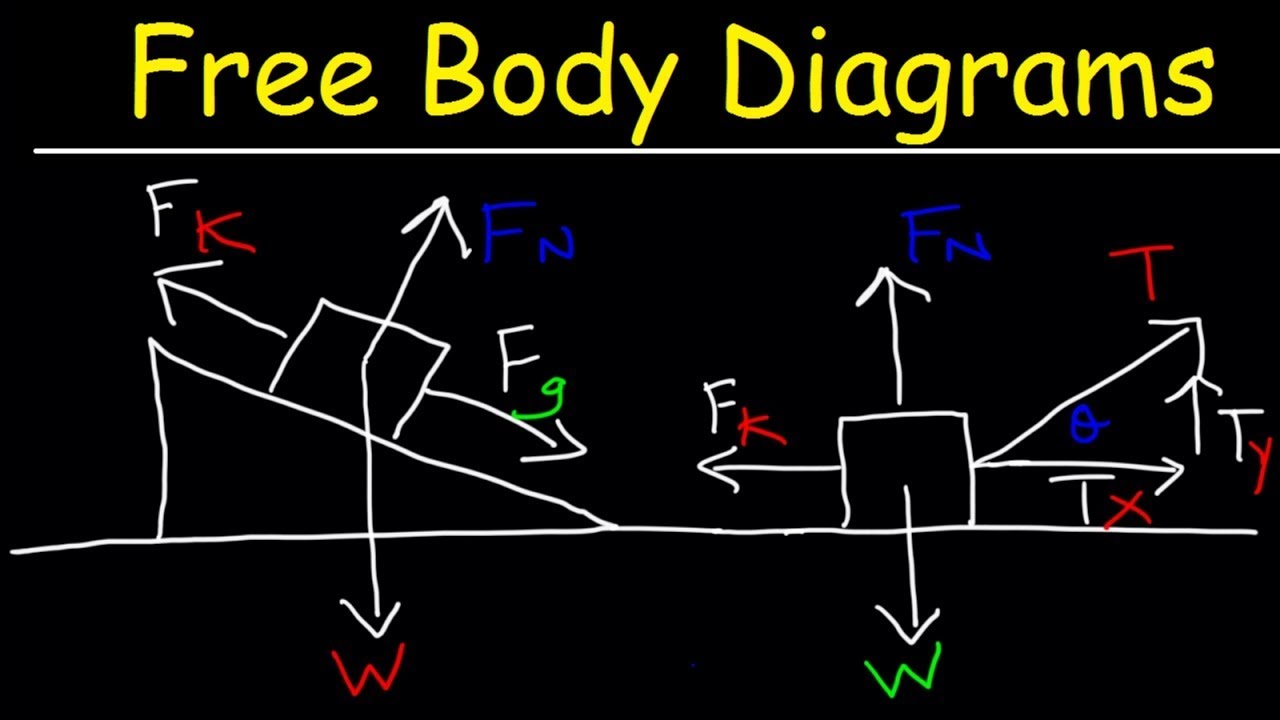
Free Body Diagrams - Tension, Friction, Inclined Planes, & Net Force

The force of tension | Forces and Newton's laws of motion | Physics | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: