How to solve percent concentration problems even if you're 🤷🏻♀️ - Dr K
TLDRThis educational video script aims to demystify percent concentration problems, guiding viewers through a step-by-step process to identify and solve them with confidence. It starts with the basics, explaining how to recognize a percent concentration problem and the importance of distinguishing between solute and solution. The script then progresses to solving problems involving both volume and mass, using simple mathematical formulas. It covers how to prepare solutions with specific concentrations and provides tips for understanding the relationship between solute, solvent, and solution. The goal is to empower viewers with the knowledge to tackle a variety of percent concentration challenges, with resources for further learning and practice.
Takeaways
- 🔍 The first indication of a percent concentration problem is the presence of words like 'percent', 'percentage', or the percent symbol.
- 📚 To solve percent concentration problems, it's helpful to remember that the solute is always on top and the solution is at the bottom in calculations.
- 🧪 In percent concentration problems, the solute is the smaller quantity and the solution is the larger quantity.
- 📉 For percent by volume problems, divide the volume of the solute by the volume of the solution and multiply by 100 to get the percentage.
- ⚗️ In mass-related problems, divide the mass of the solute by the mass of the solution and multiply by 100 to get the percentage.
- 💧 Understanding the difference between solute, solvent, and solution is crucial for solving problems correctly.
- 📝 When given a percentage, you may need to perform algebraic manipulations to find the unknown volume or mass of the solute.
- 📈 For preparing solutions, calculate the volume or mass of the solute needed based on the desired percentage concentration.
- 📐 It's important to use consistent units when calculating percent concentration, such as grams over milliliters or kilograms over liters.
- 📚 The script provides a step-by-step approach to solving percent concentration problems, starting with simpler examples and progressing to more complex ones.
- 📺 The video script suggests that viewers pause and check their work, indicating an interactive learning method.
Q & A
What is the first hint that a problem involves percent concentration?
-The first hint that a problem involves percent concentration is the presence of words like 'percent', 'percentage', or the percent symbol in the question.
What is the general approach to solving percent concentration problems?
-To solve percent concentration problems, you need to identify the solute and the solution. Remember that the solute is always on top and the solution is at the bottom, then you multiply with 100.
What is the solute in the example where 75 ml of ethanol is mixed with 375 ml of solution?
-In the example, ethanol is the solute because it has a smaller volume (75 ml) compared to the solution (375 ml).
How do you calculate the percent by volume concentration when given the volumes of solute and solution?
-To calculate the percent by volume concentration, you divide the volume of the solute by the volume of the solution, then multiply by 100.
What is the solute in the example where 30 grams of HCl is part of a 200 grams solution?
-In the example, HCl is the solute because it is the component with a smaller mass (30 grams) in comparison to the total mass of the solution (200 grams).
How do you calculate the percent mass concentration when given the masses of solute and solution?
-To calculate the percent mass concentration, you divide the mass of the solute by the mass of the solution, then multiply by 100.
What does 'solute' and 'solvent' refer to in the context of percent concentration problems?
-In percent concentration problems, 'solute' refers to the substance that is dissolved, while 'solvent' is the substance in which the solute is dissolved. Together, they form the solution.
How do you prepare a solution with a given percent concentration by volume?
-To prepare a solution with a given percent concentration by volume, you need to calculate the volume of solute required based on the total volume of the solution and the given percentage, then add the appropriate amount of solvent.
In the example involving vinegar and water, why is vinegar considered the solute and water the solvent?
-In the example, vinegar is considered the solute because it is the substance being dissolved, and water is the solvent because it is the substance in which the vinegar is dissolved.
How do you find the mass of NaCl needed to prepare a 35 ml saline solution with a 10% mass/volume concentration?
-To find the mass of NaCl needed, you use the formula where 10% (the given concentration) equals the mass of NaCl (unknown) divided by 35 ml (the volume of the solution) times 100. Solving for the mass of NaCl gives you 3.5 grams.
Why is it important to match units when calculating percent concentration by volume or mass?
-It is important to match units when calculating percent concentration to ensure consistency and accuracy. For example, if you use grams for the mass of solute, you must also use grams for the mass of the solution.
Outlines
🧪 Understanding Percent Concentration Problems
This paragraph introduces viewers to the concept of percent concentration problems, explaining that the presence of words like 'percent' or the percent symbol indicates a problem involving concentration. It guides the audience through identifying solute and solution, emphasizing that the solute is the smaller quantity and is placed on top in calculations. The paragraph provides a step-by-step solution to a problem involving ethanol and a solution, illustrating how to calculate percent by volume. It also touches on the difference between mass and volume in percent concentration calculations and offers links to introductory videos for further understanding.
📚 Advanced Percent Concentration Calculations
The second paragraph builds upon the basic understanding of percent concentration by tackling more complex problems. It explains how to identify the solute and solvent in a given solution and how to calculate the required amounts to prepare a solution with a specific percent concentration. The paragraph walks through an example of preparing a vinegar in water solution, demonstrating algebraic manipulation to find the volume of solute needed. It also addresses a scenario involving the preparation of a saline solution with a given mass percentage of NaCl, showing how to calculate the mass of solute required for a specific volume of solution. The paragraph concludes by encouraging viewers to check out a playlist for more percent concentration problems and to subscribe for additional chemistry content.
Mindmap
Keywords
💡Percent Concentration
💡Solute
💡Solution
💡Percent by Volume
💡Percent by Mass
💡Vinegar in Water Solution
💡Saline Solution
💡Mass Over Volume
💡Algebra
💡Specific Unit
Highlights
Introduction to solving percent concentration problems with confidence.
Identifying percent concentration problems by the presence of words like 'percent', 'percentage', or the percent symbol.
Understanding the roles of solute and solution in percent concentration calculations.
A tip to remember that solute is always on top and solution at the bottom in calculations.
Example problem: Calculating percent by volume with 75 ml ethanol and 375 ml solution.
Explanation of how to determine the solute as the smaller quantity in the given example.
Demonstration of calculating 20% volume concentration using the provided formula.
Transition to a second example involving mass concentration with 200 grams of solution and 30 grams of HCl.
Clarification that HCl is the solute and the calculation results in a 15% mass concentration.
Introduction of a more complex problem involving preparing a vinegar in water solution with a given percentage.
Explanation of how to prepare a 30% vinegar in water solution using algebraic methods.
Calculation of the volume of vinegar needed for the solution, resulting in 150 ml.
Determination of the volume of water needed by subtracting the volume of vinegar from the total solution.
Another example problem involving preparing a saline solution with NaCl and water.
Calculation of the mass of NaCl needed for a 10% mass/volume saline solution.
Final calculation resulting in 3.5 grams of NaCl for a 35 ml saline solution.
Encouragement for viewers to feel more confident in solving percent concentration problems.
Invitation to check out a percent concentration playlist for more chemistry videos.
Transcripts
Browse More Related Video
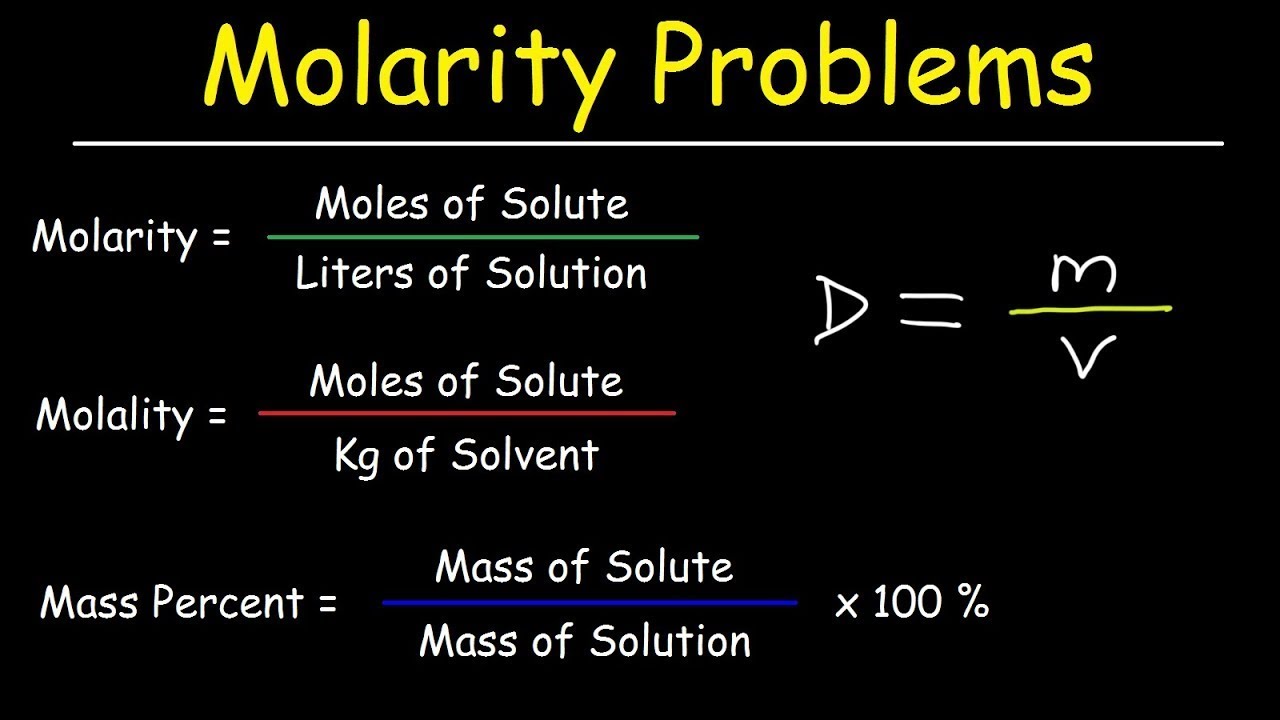
Molarity, Molality, Volume & Mass Percent, Mole Fraction & Density - Solution Concentration Problems

How to calculate percent concentration | Percent mass | Percent volume | Percent mass-volume - Dr K

Calculate %m/m (Percent by Mass of a solution)
Molarity Dilution Problems Solution Stoichiometry Grams, Moles, Liters Volume Calculations Chemistry
Mass Percent of a Solution Made Easy: How to Calculate Mass % or Make a Specific Concentration
The Percentages You'll See in Chemistry
5.0 / 5 (0 votes)
Thanks for rating: