Graphing basketball binomial distribution | Probability and Statistics | Khan Academy

Khan Academy
26 Nov 201404:22
EducationalLearning
32 Likes 10 Comments

TLDRThis video script guides viewers through visualizing a binomial probability distribution, specifically for the number of successful free throws made with a 70% success rate. The host encourages drawing a histogram to understand the distribution's shape, scales the y-axis from 0% to 40%, and the x-axis for values 0 to 6, representing the number of free throws made. The script details the probabilities for each outcome, from 0.1% for zero successful throws to 32.4% for four, and illustrates how the distribution changes with different success rates or numbers of attempts. The exercise aims to make the abstract concept of binomial distribution more tangible and interactive.

Takeaways
  • 🏀 The video discusses the probability distribution of a random variable representing the number of successful free throws made out of a certain number of attempts.
  • 🎯 The assumption is a 70% free throw percentage, which means a 0.7 probability of making a free throw and a 0.3 probability of missing.
  • 📈 The video suggests visualizing this probability distribution through a graph, specifically a binomial probability distribution graph.
  • 📊 The graph is a histogram that shows the probability of making 0 to 6 free throws, given the 70% success rate.
  • 📝 The video provides a step-by-step guide on how to draw the histogram, including setting up the axes and plotting the probabilities.
  • 📉 The probabilities for each outcome are given, starting from 0.1% for making 0 free throws to 32.4% for making 4 free throws.
  • 📚 The shape of the binomial distribution is visualized, showing how it changes with different numbers of attempts or success rates.
  • 🤔 The video encourages viewers to think about how changes in the free throw percentage or the number of shots taken would affect the distribution.
  • 🛠️ It is suggested that viewers can use a spreadsheet to experiment with different values and see how the binomial distribution changes.
  • 📊 The histogram is a useful tool for visualizing the distribution and understanding the likelihood of different outcomes.
  • 💡 The exercise is an educational demonstration of how to create and interpret a binomial distribution histogram.
Q & A

  • What is the main topic discussed in the video script?

    -The main topic discussed in the video script is the probability distribution of a binomial random variable, specifically the number of successful free throws made out of a certain number of attempts, given a 70 percent free throw percentage.

  • What is the purpose of visualizing the binomial probability distribution?

    -Visualizing the binomial probability distribution helps to make the concept more tangible and understandable, allowing viewers to see the shape of the distribution and how it changes with different parameters.

  • What is the free throw percentage used in the script for the binomial probability distribution?

    -The free throw percentage used in the script for the binomial probability distribution is 70 percent.

  • How many free throws are considered in the video script for the binomial distribution?

    -The number of free throws considered in the video script is not explicitly stated, but it is implied to be a scenario where the random variable can take on values from zero to six.

  • What does the video script suggest to do with the binomial distribution graph?

    -The video script suggests graphing the binomial distribution to visualize its shape and to understand how the distribution changes with different free throw percentages or numbers of shots taken.

  • What is the highest percentage shown on the graph in the video script?

    -The highest percentage shown on the graph in the video script is a little over 32 percent.

  • How does the video script describe the process of graphing the binomial distribution?

    -The video script describes the process of graphing the binomial distribution step by step, starting with setting up the axes for percentages and values the random variable can take, and then plotting and shading the probabilities for each value.

  • What is the significance of the percentages 18.5%, 32.4%, 30.3%, and 11.8% mentioned in the script?

    -The percentages 18.5%, 32.4%, 30.3%, and 11.8% represent the probabilities of making three, four, five, and six free throws, respectively, given the 70 percent free throw percentage and the binomial distribution.

  • What tool can be used to explore how the binomial distribution changes with different parameters?

    -A spreadsheet can be used to explore how the binomial distribution changes with different free throw percentages or numbers of shots taken.

  • What is the educational value of creating a histogram for the binomial distribution as described in the script?

    -Creating a histogram for the binomial distribution helps in visualizing the distribution, understanding the probabilities of different outcomes, and seeing how changes in parameters affect the shape and probabilities of the distribution.

Outlines
00:00
📊 Visualizing Binomial Distribution of Free Throws

The video script begins with a recap of the previous video, which covered the probability distribution for a random variable representing the number of successful free throws out of a given number of attempts, assuming a 70% success rate. The speaker encourages viewers to visualize this distribution by drawing a graph. The script then describes the process of creating a binomial probability distribution graph, including setting up axes with percentages on one and values of the random variable (number of successful free throws) on the other. The graph is populated with the probabilities of making zero to six free throws, with the highest probability at 32.4% for making three free throws. The speaker suggests that this visual exercise helps in understanding the distribution and encourages viewers to explore how changes in the free throw percentage or the number of attempts affect the distribution, hinting at the use of spreadsheets for such exploration.

Mindmap
Keywords
💡Probability Distribution
Probability distribution refers to the likelihood of different outcomes in an experiment, often represented graphically. In the video, the focus is on a binomial probability distribution, which is a discrete distribution that describes the number of successes in a fixed number of independent Bernoulli trials with the same probability of success. The script discusses visualizing this distribution for a specific scenario involving free throws.
💡Random Variable
A random variable is a variable whose value is determined by an outcome of a random phenomenon. In the context of the video, the random variable is defined as the number of successful free throws made out of a certain number of attempts, with a given success rate.
💡Free Throw Percentage
Free throw percentage is a measure of shooting accuracy, calculated as the number of successful free throws divided by the total number of free throws attempted, expressed as a percentage. The video uses a 70 percent free throw percentage to calculate the probability distribution of successful free throws.
💡Binomial Probability Distribution
Binomial probability distribution is a type of discrete probability distribution that describes the number of successes in a fixed number of independent trials with the same probability of success. The script describes drawing a graph to visualize this distribution for a specific scenario, which helps in understanding the likelihood of different outcomes.
💡Graph
A graph is a visual representation used to display data and relationships between variables. In the video, the instructor suggests graphing the binomial probability distribution to make the concept more tangible and easier to understand. The script includes a step-by-step process of drawing such a graph.
💡Histogram
A histogram is a graphical representation used to show the distribution of data. It groups the data into bins and shows the frequency of data points in each bin. The video script describes creating a histogram to represent the binomial probability distribution of making free throws.
💡Percentage
Percentage is a way of expressing a number as a fraction of 100. It is used to describe proportions or comparisons. In the script, various percentages are mentioned to represent the likelihood of making a certain number of free throws, such as 32.4 percent for making four free throws.
💡Spreadsheet
A spreadsheet is a computer application program for data organization, analysis, and storage. In the video, the instructor suggests using a spreadsheet to explore how changes in the free throw percentage or the number of shots taken would affect the binomial distribution, allowing for dynamic visualization and analysis.
💡Success Rate
Success rate is the ratio of the number of successful outcomes to the total number of attempts in a given situation. In the context of the video, the success rate is the probability of making a free throw, which is 70 percent, and it is a key factor in calculating the binomial probability distribution.
💡Visualize
To visualize means to form a mental image or picture of something. In the script, the instructor encourages visualizing the binomial probability distribution by drawing a graph, which helps in understanding the distribution's shape and characteristics.
💡Shape
In statistics, the shape of a distribution refers to the form or pattern that the distribution takes when plotted on a graph. The video script discusses the shape of the binomial distribution and how it can be visualized through a graph, which is important for understanding the distribution's properties.
Highlights

Introduction of a probability distribution for a random variable representing the number of successful free throws.

Assumption of a 70 percent free throw percentage in the model.

Suggestion to visualize the binomial probability distribution through graphing.

Explanation of the importance of graphing for visual understanding of distribution shapes.

Decision to graph the binomial distribution together with the audience.

Description of setting up the graph with percentage scales and values the random variable can take.

Identification of the highest percentage on the graph as just over 32 percent.

Detailing the process of drawing the graph with different percentages and values.

Graphing the probability of making zero free throws as barely registering on the graph.

Incremental graphing of probabilities for making one, two, and three free throws.

Highlighting the peak probability at 32.4 percent for making four free throws.

Demonstration of the decrease in probability for making five and six free throws.

Discussion on the practical application of graphing for understanding binomial distributions.

Invitation to explore how changes in free throw percentage or number of shots affect the distribution.

Suggestion to use a spreadsheet to experiment with different parameters of the binomial distribution.

Emphasis on the educational value of visualizing statistical distributions through graphing.

Transcripts

Browse More Related Video

Free throw binomial probability distribution | Probability and Statistics | Khan Academy

Generalizing k scores in n attempts | Probability and Statistics | Khan Academy

Probability of making 2 shots in 6 attempts | Probability and Statistics | Khan Academy

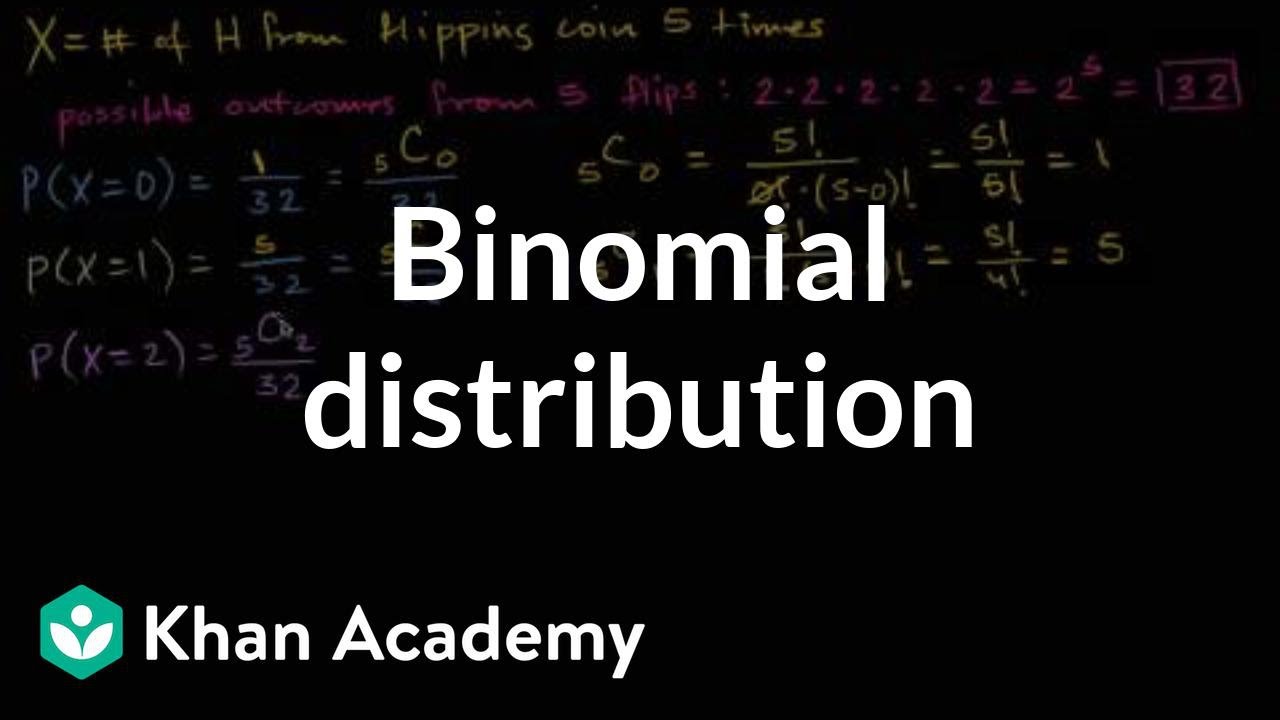
Binomial distribution | Probability and Statistics | Khan Academy

Visualizing a binomial distribution | Probability and Statistics | Khan Academy

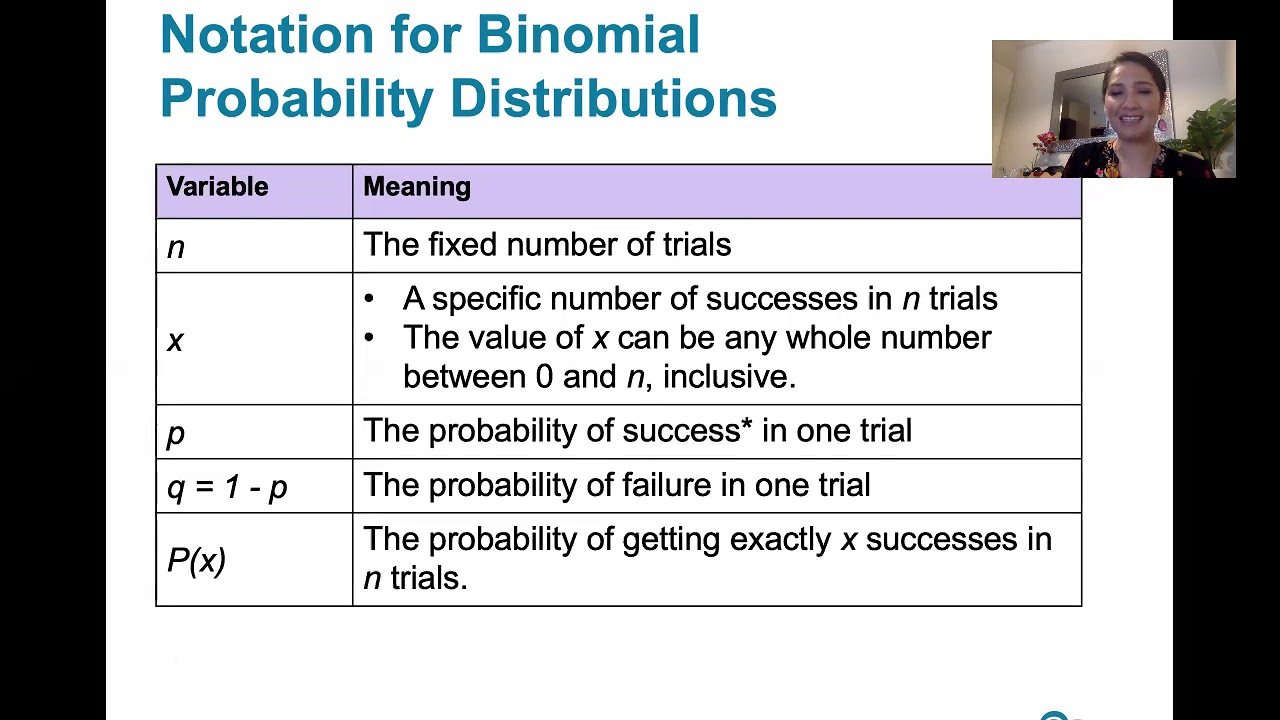
5.2.1 Binomial Probability Distributions - Is this procedure described by a binomial distribution?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ProbabilityBinomialDistributionFree ThrowsBasketballVisualizationHistogramMathematicsEducationalGraphing