Lesson 13 - Polar Coordinates (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis advanced calculus 2 tutorial introduces the concept of polar coordinates, a crucial topic for students pursuing careers in engineering, physics, or science. Polar coordinates offer a different way to represent points in a plane compared to the traditional Cartesian coordinates (X, Y). The instructor emphasizes the practical applications of polar coordinates in various fields, particularly in engineering, where they are used frequently. The lesson begins with an explanation of polar coordinates and their significance, setting the stage for more complex topics like polar equations and integrals in future sections.

Takeaways
  • 📚 The class is transitioning to a new topic: polar coordinates, which will be the focus for the next few sections.
  • 🔍 Polar coordinates are an alternative way to represent functions and points in a plane, different from the Cartesian coordinate system.
  • 📈 The polar coordinate system will be particularly important for students pursuing careers in engineering, physics, or science.
  • 📉 The instructor emphasizes the practicality and frequent use of polar coordinates in various engineering fields, unlike some other topics covered in the class.
  • 📝 The class will start with the basics of polar coordinates, not immediately jumping into integrals or derivatives.
  • 📐 Cartesian coordinates are the traditional x and y values that students are familiar with from their earlier studies.
  • 📊 In Cartesian coordinates, points are represented as ordered pairs (x, y), which define their location on a graph.
  • 📈 Cartesian coordinates are used to write equations and functions, where x is the independent variable and y is the dependent variable.
  • 🌐 The introduction of a third dimension (z) will be covered in future studies, specifically in three-dimensional calculus.
  • 📚 The current focus is on two-dimensional functions and the transition to understanding and applying polar coordinates.
Q & A

  • What is the main topic being introduced in this section of the Advanced Calculus 2 tutorial?

    -The main topic being introduced is polar coordinates and their representation in functions.

  • Why is the polar coordinate system important to learn according to the instructor?

    -The polar coordinate system is important because it is very powerful and is used frequently in various branches of engineering, physics, and science-based careers.

  • What are the typical subjects covered towards the end of an Advanced Calculus 2 course?

    -Towards the end of an Advanced Calculus 2 course, subjects such as trigonometric functions, inverses, surface areas, arc lengths, and polar coordinates are typically covered.

  • What is the significance of polar equations in calculus?

    -Polar equations are significant in calculus as they lead to the study of integrals in polar coordinates, which is a concept that can be challenging but essential for certain applications.

  • How does the instructor describe the transition to polar coordinates from Cartesian coordinates?

    -The instructor describes the transition as a switch in gears, moving from familiar concepts to a new way of thinking about coordinates that is less commonly used but very important.

  • What is the Cartesian coordinate system?

    -The Cartesian coordinate system is a two-dimensional coordinate system that uses an ordered pair (X, Y) to define the position of a point in a plane.

  • What is the difference between an independent variable and a dependent variable in the context of functions?

    -In the context of functions, the independent variable (usually X) is the input you plug into the function, while the dependent variable (usually Y) is the output that depends on the value of the independent variable and the function itself.

  • What does the instructor mean by 'integrals in polar coordinates'?

    -Integrals in polar coordinates refer to the process of calculating areas, volumes, or other quantities by integrating functions that are represented in polar coordinates rather than the standard Cartesian coordinates.

  • Why might some topics in Advanced Calculus 2 be considered 'disconnected'?

    -Some topics in Advanced Calculus 2 might be considered 'disconnected' because they cover a wide range of mathematical concepts that are not necessarily linked in a sequential learning path, and some may not be used frequently in all fields.

  • How does the instructor relate the concept of polar coordinates to real-world applications?

    -The instructor relates polar coordinates to real-world applications by emphasizing their frequent use in engineering and physics, suggesting that understanding polar coordinates is essential for many science-based careers.

  • What is the instructor's stance on the importance of learning polar coordinates compared to other techniques in calculus?

    -The instructor believes that polar coordinates are more universally important and useful compared to other techniques like integration by partial fractions, which may not be used as frequently depending on the specific problem encountered.

Outlines
00:00
📚 Introduction to Polar Coordinates in Advanced Calculus 2

The instructor introduces a new topic in the Advanced Calculus 2 course, which is polar coordinates. They explain that the next few sections will focus on the polar representation of functions, emphasizing the importance of understanding polar coordinates for those pursuing careers in engineering, physics, or science. The instructor highlights that while some topics in calculus may not be frequently used, polar representation and integration are essential tools across various engineering disciplines. The summary also contrasts polar coordinates with the more familiar Cartesian coordinates, which are defined by two numbers, X and Y, on a graph. The instructor prepares the students for a shift in perspective, moving away from discussing integrals and derivatives to focusing solely on the concept of polar coordinates in this section.

Mindmap
Keywords
💡Polar Coordinates
Polar coordinates are a two-dimensional coordinate system where each point on a plane is determined by a distance from a reference point and an angle from a reference direction. In the video, polar coordinates are introduced as a new topic, emphasizing their importance in various scientific and engineering fields. The script mentions that polar coordinates will be the focus for the next few sections, indicating their significance in understanding advanced calculus concepts.
💡Advanced Calculus 2
Advanced Calculus 2 is a higher-level course in calculus that builds upon the foundational concepts introduced in Calculus 1. The script positions the discussion of polar coordinates within the context of this advanced course, suggesting that the topic is a sophisticated and integral part of the curriculum. It is implied that understanding polar coordinates is crucial for students pursuing careers in engineering, physics, or other science-based fields.
💡Trigonometric Functions
Trigonometric functions are mathematical functions that relate angles to the ratios of the sides of a right-angled triangle. The script briefly mentions these functions in the context of the diverse topics covered in Calculus 2, indicating that they are part of the broader mathematical landscape that students are expected to navigate.
💡Surface Areas and Arc Lengths
Surface areas and arc lengths are concepts that deal with the measurement of two-dimensional surfaces and the lengths of curves, respectively. The script mentions these topics as part of the 'disconnected' topics that students encounter towards the end of Calculus 2, suggesting that they are specialized areas of study within the broader field of calculus.
💡Integrals in Polar Coordinates
Integrals in polar coordinates refer to the process of calculating areas or volumes using the polar coordinate system. The script highlights this as a significant aspect of polar coordinates in Calculus 2, noting that it can be a challenging concept for students because it represents a shift from the more familiar Cartesian coordinate system.
💡Cartesian Coordinate System
The Cartesian coordinate system, also known as the XY coordinate system, is a method of representing points on a plane using two perpendicular axes, labeled X and Y. The script contrasts this with polar coordinates, explaining that Cartesian coordinates are based on two numbers (X and Y) that define a point's location, whereas polar coordinates use a radial distance and an angle.
💡Independent Variable
In the context of functions, the independent variable is the input that can be freely chosen and does not depend on other variables. The script uses the term when discussing functions in the Cartesian coordinate system, stating that 'X is the independent variable' because it is the value that is plugged into the function to obtain the dependent variable, Y.
💡Dependent Variable
The dependent variable is a variable that depends on the value of the independent variable. In the script, Y is described as the dependent variable because its value is determined by the value of X and the specific function being used, such as 'Y is equal to f of X'.
💡Function Representation
Function representation refers to the graphical or algebraic depiction of a function's behavior. The script provides a review of how functions are represented in the Cartesian coordinate system, where Y is shown as a function of X, and the shape of the graph is determined by the function's formula.
💡Engineering and Physics
Engineering and physics are fields of study that apply scientific principles to design, build, and understand various systems and phenomena. The script emphasizes the importance of polar coordinates in these fields, suggesting that the concepts learned in Calculus 2, particularly polar coordinates and their applications, are highly relevant and practically useful in these disciplines.
Highlights

Introduction to polar coordinates as a new topic in Advanced Calculus 2.

Emphasis on the importance of polar coordinates for future studies in engineering, physics, or science-based careers.

Explanation that polar coordinates are less familiar but very powerful for problem-solving in various fields.

Comparison between Cartesian coordinates (X, Y) and the concept of polar coordinates.

Description of Cartesian coordinates as the traditional method of representing points on a graph.

Discussion on how Cartesian coordinates are taught and understood with X and Y values.

Introduction of three-dimensional functions and the addition of Z coordinates in future studies.

Clarification that the current discussion is limited to two-dimensional plane for simplicity.

Explanation of Cartesian points and their representation on a graph.

Review of writing equations in Cartesian coordinate systems with X and Y variables.

Illustration of functions where Y is a dependent variable determined by the function of X.

Highlighting that X is the independent variable because it is input into the function.

Clarification that Y is the dependent variable as it relies on the value of X and the function itself.

The significance of learning polar coordinates for engineering and scientific applications.

The potential for polar representation and integration to be used frequently across different branches of engineering.

A comparison of the utility of polar coordinates versus other integration techniques that may not be as commonly used.

Transcripts

Browse More Related Video

Lesson 14 - Polar Equations (Calculus 2 Tutor)

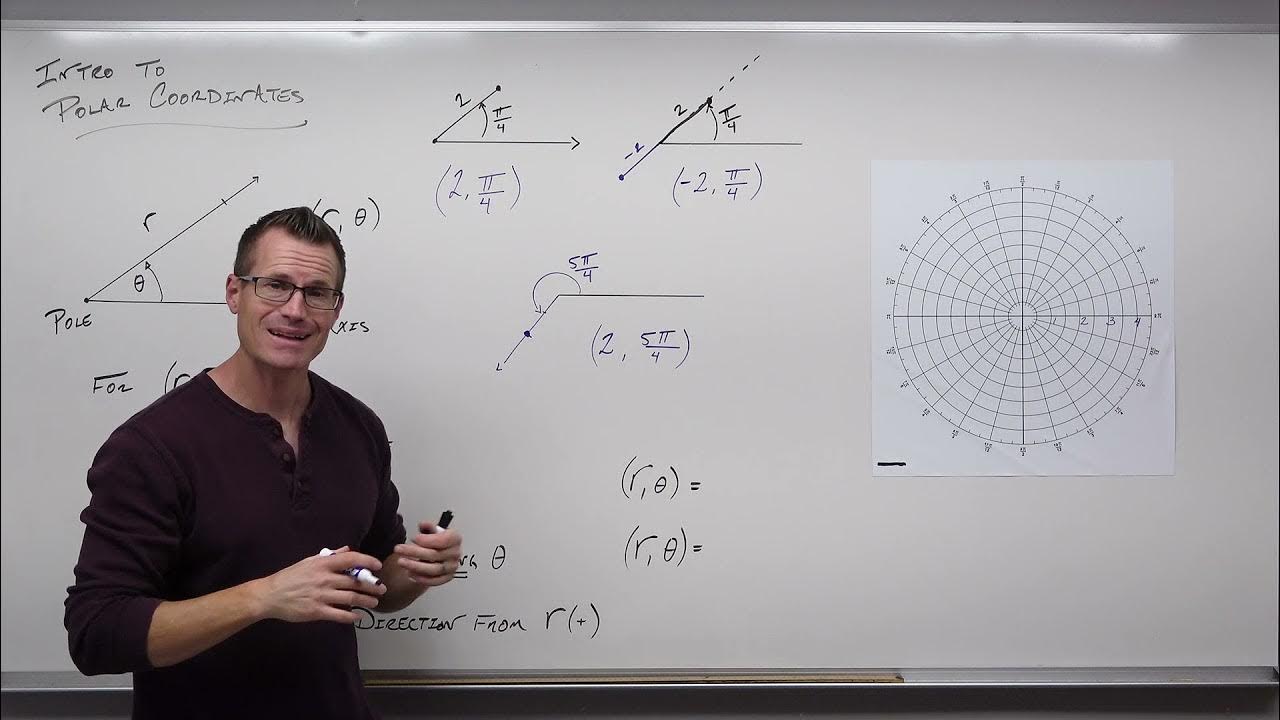
Introduction to Polar Coordinates (Precalculus - Trigonometry 36)

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Calculus 2: Polar Coordinates (Video #30) | Math with Professor V

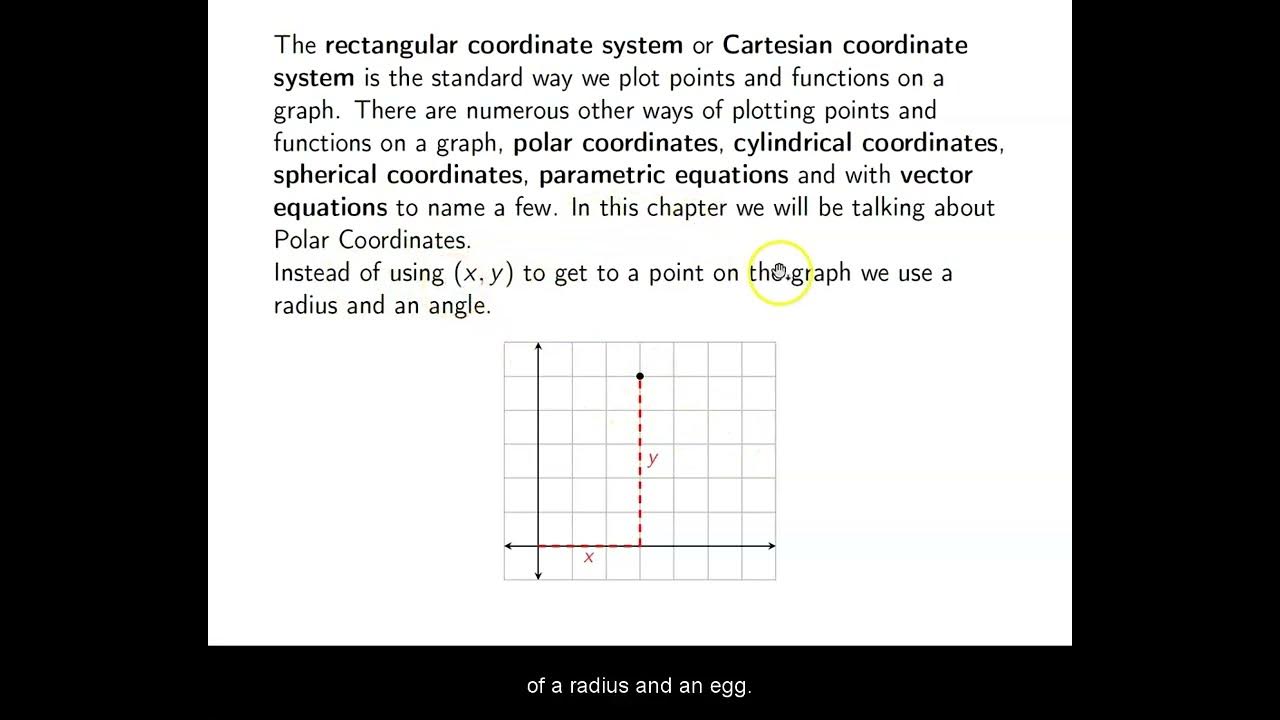
Ch. 8.1 Polar Coordinates

Double Integrals in Polar Coordinates

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Polar CoordinatesAdvanced CalculusTrigonometric FunctionsEngineeringPhysicsScienceIntegralsDerivativesCartesian SystemEducational Content